No CrossRef data available.
Article contents
SUBSETS OF VERTICES GIVE MORITA EQUIVALENCES OF LEAVITT PATH ALGEBRAS
Part of:
Modules, bimodules and ideals
Published online by Cambridge University Press: 30 March 2017
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
We show that every subset of vertices of a directed graph 
$E$ gives a Morita equivalence between a subalgebra and an ideal of the associated Leavitt path algebra. We use this observation to prove an algebraic version of a theorem of Crisp and Gow: certain subgraphs of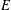 
$E$ can be contracted to a new graph 
$G$ such that the Leavitt path algebras of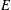 
$E$ and 
$G$ are Morita equivalent. We provide examples to illustrate how desingularising a graph, and in- or out-delaying of a graph, all fit into this setting.
MSC classification
- Type
- Research Article
- Information
- Bulletin of the Australian Mathematical Society , Volume 96 , Issue 2 , October 2017 , pp. 212 - 222
- Copyright
- © 2017 Australian Mathematical Publishing Association Inc.
Footnotes
This research has been supported by a University of Otago Research Grant.
References
Abrams, G. and Aranda Pino, G., ‘The Leavitt path algebras of arbitrary graphs’, Houston J. Math.
34 (2008), 423–442.Google Scholar
Abrams, G., Louly, A., Pardo, E. and Smith, C., ‘Flow invariants in the classification of Leavitt path algebras’, J. Algebra
333 (2011), 202–231.CrossRefGoogle Scholar
Aranda Pino, G., Martín Barquero, D., Martín González, C. and Siles Molina, M., ‘The socle of a Leavitt path algebra’, J. Pure Appl. Algebra
212 (2008), 500–509.CrossRefGoogle Scholar
Arklint, S., Gabe, J. and Ruiz, E., ‘Hereditary
$C^{\ast }$
-subalgebras of graph algebras’, Preprint, 2016, arXiv:1604.03085.Google Scholar
$C^{\ast }$
-subalgebras of graph algebras’, Preprint, 2016, arXiv:1604.03085.Google ScholarBates, T., ‘Applications of the gauge-invariant uniqueness theorem for graph algebras’, Bull. Aust. Math. Soc.
66 (2002), 57–67.CrossRefGoogle Scholar
Bates, T. and Pask, D., ‘Flow equivalence of graph algebras’, Ergodic Theory Dynam. Systems
24 (2004), 367–382.CrossRefGoogle Scholar
Clark, L. O., Flynn, C. and an Huef, A., ‘Kumjian–Pask algebras of locally convex higher-rank graphs’, J. Algebra
399 (2014), 445–474.CrossRefGoogle Scholar
Clark, L. O. and Pangalela, Y. E. P., ‘Kumjian–Pask algebras of finitely-aligned higher-rank graphs’, Preprint, 2016, arXiv:1512.06547.CrossRefGoogle Scholar
Clark, L. O. and Sims, A., ‘Equivalent groupoids have Morita equivalent Steinberg algebras’, J. Pure Appl. Algebra
219 (2015), 2062–2075.CrossRefGoogle Scholar
Crisp, T. and Gow, D., ‘Contractible subgraphs and Morita equivalence of graph C
∗ -algebras’, Proc. Amer. Math. Soc.
134 (2006), 2003–2013.CrossRefGoogle Scholar
Drinen, D. and Sieben, N., ‘
C
∗ -equivalences of graphs’, J. Operator Theory
45 (2001), 209–229.Google Scholar
Drinen, D. and Tomforde, M., ‘The C
∗ -algebras of arbitrary graphs’, Rocky Mountain J. Math.
35 (2005), 105–135.CrossRefGoogle Scholar
Johansen, R. and Sørensen, A. P. W., ‘The Cuntz splice does not preserve ∗-isomorphism of Leavitt path algebras over ℤ’, J. Pure Appl. Algebra
220 (2016), 3966–3983.CrossRefGoogle Scholar
Nasr-Isfahani, A. R., ‘Singular equivalence of finite dimensional algebras with radical square zero’, J. Pure Appl. Algebra
220 (2016), 3948–3965.CrossRefGoogle Scholar
Raeburn, I., Graph Algebras, CBMS Regional Conference Series in Mathematics, 103 (American Mathematical Society, Providence, RI, 2005).CrossRefGoogle Scholar
Sørensen, A. P. W., ‘Geometric classification of simple graph algebras’, Ergodic Theory Dynam. Systems
33 (2013), 1199–1220.CrossRefGoogle Scholar
Tomforde, M., ‘Leavitt path algebras with coefficients in a commutative ring’, J. Pure Appl. Algebra
215 (2011), 471–484.CrossRefGoogle Scholar
You have
Access