Hostname: page-component-848d4c4894-ndmmz Total loading time: 0 Render date: 2024-06-02T13:56:36.967Z Has data issue: false hasContentIssue false
  • English
  • Français

Krivine’s Function Calculus and Bochner Integration

Part of: Topological linear spaces and related structures Normed linear spaces and Banach spaces; Banach lattices

Published online by Cambridge University Press:  15 October 2018

V. G. Troitsky  and
M. S. Türer
V. G. Troitsky
Affiliation:
Department of Mathematical and Statistical Sciences, University of Alberta, Edmonton, AB, T6G 2G1, Canada Email: troitsky@ualberta.ca
M. S. Türer
Affiliation:
Department of Mathematics and Computer Science, İstanbul Kültür University, Bakırköy 34156, İstanbul, Turkey Email: m.turer@iku.edu.tr
Get access Rights & Permissions [Opens in a new window]

Abstract

We prove that Krivine’s Function Calculus is compatible with integration. Let $(\unicode[STIX]{x1D6FA},\unicode[STIX]{x1D6F4},\unicode[STIX]{x1D707})$ be a finite measure space, 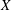$X$ a Banach lattice, 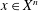$\mathbf{x}\in X^{n}$, and $f:\mathbb{R}^{n}\times \unicode[STIX]{x1D6FA}\rightarrow \mathbb{R}$ a function such that 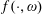$f(\cdot ,\unicode[STIX]{x1D714})$ is continuous and positively homogeneous for every $\unicode[STIX]{x1D714}\in \unicode[STIX]{x1D6FA}$, and $f(\mathbf{s},\cdot )$ is integrable for every 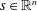$\mathbf{s}\in \mathbb{R}^{n}$. Put 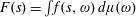$F(\mathbf{s})=\int f(\mathbf{s},\unicode[STIX]{x1D714})\,d\unicode[STIX]{x1D707}(\unicode[STIX]{x1D714})$ and define 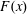$F(\mathbf{x})$ and 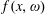$f(\mathbf{x},\unicode[STIX]{x1D714})$ via Krivine’s Function Calculus. We prove that under certain natural assumptions $F(\mathbf{x})=\int f(\mathbf{x},\unicode[STIX]{x1D714})\,d\unicode[STIX]{x1D707}(\unicode[STIX]{x1D714})$, where the right hand side is a Bochner integral.

Keywords

Banach latticefunction calculusBochner integral

MSC classification

Primary: 46B42: Banach lattices
Secondary: 46A40: Ordered topological linear spaces, vector lattices
Type
Article
Information
Canadian Mathematical Bulletin , Volume 62 , Issue 3 , September 2019 , pp. 663 - 669
Copyright
© Canadian Mathematical Society 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The first author was supported by an NSERC grant.

References

Abramovich, Y. A. and Aliprantis, C. D., An invitation to operator theory . Graduate Studies in Mathematics, 50, American Mathematical Society, Providence, RI, 2002.Google Scholar
Aliprantis, C. D. and Burkinshaw, O., Positive operators . Springer, Dordrecht, 2006. Reprint of the 1985 original.Google Scholar
Davis, W. J., Garling, D. J. H., and Tomczak-Jaegermann, N., The complex convexity of quasinormed linear spaces . J. Funct. Anal. 55(1984), no. 1, 110–150.Google Scholar
Diestel, J. and Uhl, J. J. Jr., Vector measures . Mathematical Surveys, 15, American Mathematical Society, Providence, RI, 1977.Google Scholar
Kalton, N. J., Hermitian operators on complex Banach lattices and a problem of Garth Dales . J. Lond. Math. Soc. (2) 86(2012), no. 3, 641–656.Google Scholar
Lindenstrauss, J. and Tzafriri, L., Classical Banach spaces. II . Function spaces, Springer-Verlag, Berlin, 1979.Google Scholar