Hostname: page-component-848d4c4894-ndmmz Total loading time: 0 Render date: 2024-05-28T03:12:38.762Z Has data issue: false hasContentIssue false
  • English
  • Français

WEAK ADMISSIBILITY, PRIMITIVITY, O-MINIMALITY, AND DIOPHANTINE APPROXIMATION

Part of: Noncompact transformation groups Diophantine approximation, transcendental number theory Model theory Probabilistic theory: distribution modulo $1$; metric theory of algorithms Geometry of numbers Additive number theory; partitions

Published online by Cambridge University Press:  17 April 2018

Martin Widmer
Martin Widmer*
Affiliation:
Department of Mathematics, Royal Holloway, University of London, Egham, Surrey TW20 0EX, U.K. email martin.widmer@rhul.ac.uk
Get access

Abstract

We generalize Skriganov’s notion of weak admissibility for lattices to include standard lattices occurring in Diophantine approximation and algebraic number theory, and we prove estimates for the number of lattice points in sets such as aligned boxes. Our result improves on Skriganov’s celebrated counting result if the box is sufficiently distorted, the lattice is not admissible, and, e.g., symplectic or orthogonal. We establish a criterion under which our error term is sharp, and we provide examples in dimensions 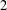$2$ and 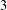$3$ using continued fractions. We also establish a similar counting result for primitive lattice points, and apply the latter to the classical problem of Diophantine approximation with primitive points as studied by Chalk, Erdős, and others. Finally, we use o-minimality to describe large classes of sets to which our counting results apply.

MSC classification

Primary: 11H06: Lattices and convex bodies 11P21: Lattice points in specified regions 11K60: Diophantine approximation 11J20: Inhomogeneous linear forms
Secondary: 03C64: Model theory of ordered structures; o-minimality 22F30: Homogeneous spaces
Type
Research Article
Information
Mathematika , Volume 64 , Issue 2 , 2018 , pp. 475 - 496
Copyright
Copyright © University College London 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Barroero, F., Counting algebraic integers of fixed degree and bounded height. Monatsh. Math. 175(1) 2014, 25–41.CrossRefGoogle Scholar
Barroero, F., Algebraic s-integers of fixed degree and bounded height. Acta Arith. 167(1) 2015, 67–90.CrossRefGoogle Scholar
Barroero, F. and Widmer, M., Counting lattice points and o-minimal structures. Int. Math. Res. Not. IMRN 2014 2014, 4932–4957.CrossRefGoogle Scholar
Chalk, J. H. H. and Erdős, P., On the distribution of primitive lattice points in the plane. Canad. Math. Bull. 2 1959, 91–96.CrossRefGoogle Scholar
Dani, S. G., Laurent, M. and Nogueira, A., Multi-dimensional metric approximation by primitive points. Math. Z. 279(3–4) 2015, 1081–1101.CrossRefGoogle Scholar
Frei, C., Loughran, D. and Sofos, E., Rational points of bounded height on general conic bundle surfaces. Proc. Lond. Math. Soc. (3) 2018, doi: 10.1112/plms.12134.CrossRefGoogle Scholar
Frei, C. and Pieropan, M., O-minimality on twisted universal torsors and Manin’s conjecture over number fields. Ann. Sci. Éc. Norm. Supér. 49(4) 2016, 757–811.CrossRefGoogle Scholar
Frei, C. and Sofos, E., Counting rational points on smooth cubic surfaces. Math. Res. Lett. 23(1) 2016, 127–143.CrossRefGoogle Scholar
Frei, C. and Sofos, E., Generalised divisor sums of binary forms over number fields. J. Inst. Math. Jussieu (to appear).Google Scholar
Laurent, M. and Nogueira, A., Inhomogeneous approximation with coprime integers and lattice orbits. Acta Arith. 154(4) 2012, 413–427.CrossRefGoogle Scholar
Pila, J. and Wilkie, A., The rational points of a definable set. Duke Math. J. 133 2006, 591–616.CrossRefGoogle Scholar
Scanlon, T., A proof of the André–Oort conjecture using mathematical logic [after Pila, Wilkie and Zannier]. In Séminaire Bourbaki, Vol. 2010/2011, Exposés 1027–1042 (Astérisque 348 ), Société Mathématique de France (2012), Exp. No. 1037, ix, 299–315.Google Scholar
Scanlon, T., O-minimality as an approach to the André-Oort conjecture. In Around the Zilber–Pink Conjecture (Panoramas et Synthéses 52 ), Société Mathématique de France (2017), 111–165.Google Scholar
Schmidt, W. M., Badly approximable systems of linear forms. J. Number Theory 1 1969, 139–154.CrossRefGoogle Scholar
Schmidt, W. M., Irregularities of distribution. VII. Acta Arith. 21 1972, 45–50.CrossRefGoogle Scholar
Skriganov, M. M., Constructions of uniform distributions in terms of geometry of numbers. Algebra Analiz. 6(3) 1994, 200–230.Google Scholar
Skriganov, M. M., Ergodic theory on SL(n), diophantine approximations and anomalies in the lattice point problem. Invent. Math. 132 1998, 1–72.CrossRefGoogle Scholar
Spain, P. G., Lipschitz: a new version of an old principle. Bull. Lond. Math. Soc. 27 1995, 565–566.CrossRefGoogle Scholar
Technau, N. and Widmer, M., A note on Skriganov’s counting theorem. Submitted.Google Scholar
van den Dries, L., Tame Topology and o-Minimal Structures (London Mathematical Society Lecture Note Series 248 ), Cambridge University Press (Cambridge, 1998).CrossRefGoogle Scholar
Widmer, M., Counting primitive points of bounded height. Trans. Amer. Math. Soc. 362 2010, 4793–4829.CrossRefGoogle Scholar
Widmer, M., Lipschitz class, narrow class, and counting lattice points. Proc. Amer. Math. Soc. 140(2) 2012, 677–689.CrossRefGoogle Scholar
Wilkie, A. J., o-minimal structures. In Séminaire Bourbaki, Vol. 2007/2008 (Astérisque 326 ), Société Mathématique de France (2009), Exp. No. 985, vii, 131–142.Google Scholar