Hostname: page-component-848d4c4894-2pzkn Total loading time: 0 Render date: 2024-05-18T02:30:12.569Z Has data issue: false hasContentIssue false
  • English
  • Français

TOPICS SURROUNDING THE COMBINATORIAL ANABELIAN GEOMETRY OF HYPERBOLIC CURVES IV: DISCRETENESS AND SECTIONS

Part of: Curves

Published online by Cambridge University Press:  18 January 2024

YUICHIRO HOSHI Open the ORCID record for YUICHIRO HOSHI [Opens in a new window]  and
SHINICHI MOCHIZUKI Open the ORCID record for SHINICHI MOCHIZUKI [Opens in a new window]
YUICHIRO HOSHI*
Affiliation:
Research Institute for Mathematical Sciences Kyoto University Kyoto 606-8502 Japan
SHINICHI MOCHIZUKI
Affiliation:
Research Institute for Mathematical Sciences Kyoto University Kyoto 606-8502 Japan motizuki@kurims.kyoto-u.ac.jp
*
yuichiro@kurims.kyoto-u.ac.jp
Get access Rights & Permissions [Opens in a new window]

Abstract

Let $\Sigma $ be a nonempty subset of the set of prime numbers which is either equal to the entire set of prime numbers or of cardinality one. In the present paper, we continue our study of the pro-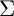$\Sigma $ fundamental groups of hyperbolic curves and their associated configuration spaces over algebraically closed fields in which the primes of 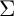$\Sigma $ are invertible. The present paper focuses on the topic of comparison between the theory developed in earlier papers concerning pro-$\Sigma $ fundamental groups and various discrete versions of this theory. We begin by developing a theory concerning certain combinatorial analogues of the section conjecture and Grothendieck conjecture. This portion of the theory is purely combinatorial and essentially follows from a result concerning the existence of fixed points of actions of finite groups on finite graphs (satisfying certain conditions). We then examine various applications of this purely combinatorial theory to scheme theory. Next, we verify various results in the theory of discrete fundamental groups of hyperbolic topological surfaces to the effect that various properties of (discrete) subgroups of such groups hold if and only if analogous properties hold for the closures of these subgroups in the profinite completions of the discrete fundamental groups under consideration. These results make possible a fairly straightforward translation, into discrete versions, of pro-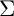$\Sigma $ results obtained in previous papers by the authors. Finally, we discuss a construction that was considered previously by M. Boggi in the discrete case from the point of view of the present paper.

Keywords

combinatorial anabelian geometrycombinatorial section conjecturefixed pointscombinatorial Grothendieck conjecturediscrete/profinite comparison

MSC classification

Primary: 14H30: Coverings, fundamental group
Secondary: 14H10: Families, moduli (algebraic)
Type
Article
Information
Nagoya Mathematical Journal , First View , pp. 1 - 101
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press on behalf of Foundation Nagoya Mathematical Journal

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

André, Y., On a geometric description of $Gal\left({\overline{\mathbb{Q}}}_p/{\mathbb{Q}}_p\right)$ and a $p$ -adic avatar of $\hat{GT}$ , Duke Math. J. 119 (2003), 1–39.Google Scholar
Berkovich, V. G., Spectral theory and analytic geometry over non-Archimedean fields, Mathematical Surveys and Monographs, Vol. 33, American Mathematical Society, Providence, RI, 1990.Google Scholar
Berkovich, V. G., Smooth $p$ -adic analytic spaces are locally contractible , Invent. Math. 137 (1999), 1–84.CrossRefGoogle Scholar
Boggi, M., Profinite Teichmüller theory , Math. Nachr. 279 (2006), 953–987.CrossRefGoogle Scholar
Boggi, M., The congruence subgroup property for the hyperelliptic modular group, preprint, arXiv:math.0803.3841v4, 2013.Google Scholar
Boggi, M., On the procongruence completion of the Teichmüller modular group , Trans. Amer. Math. Soc. 366 (2014), 5185–5221.CrossRefGoogle Scholar
Deligne, P. and Mumford, D., The irreducibility of the space of curves of given genus , Inst. Hautes Études Sci. Publ. Math. 36 (1969), 75–109.CrossRefGoogle Scholar
Hoshi, Y. and Mochizuki, S., On the combinatorial anabelian geometry of nodally nondegenerate outer representations , Hiroshima Math. J. 41 (2011), 275–342.CrossRefGoogle Scholar
Hoshi, Y. and Mochizuki, S., “Topics surrounding the combinatorial anabelian geometry of hyperbolic curves I: Inertia groups and profinite Dehn twists” in Galois–Teichmüller theory and arithmetic geometry, Advanced Studies in Pure Mathematics, Vol. 63, Mathematical Society of Japan, Tokyo, 2012, pp. 659–811.CrossRefGoogle Scholar
Hoshi, Y. and Mochizuki, S., Topics surrounding the combinatorial anabelian geometry of hyperbolic curves II: Tripods and combinatorial cuspidalization, Lecture Notes in Mathematics, Vol. 2299, Springer, Singapore, 2022.CrossRefGoogle Scholar
Hoshi, Y. and Mochizuki, S., Topics surrounding the combinatorial anabelian geometry of hyperbolic curves III: Tripods and tempered fundamental groups, to appear in Kyoto J. Math. Google Scholar
Kato, K. and Nakayama, C., Log Betti cohomology, log étale cohomology, and log de Rham cohomology of log schemes over C , Kodai Math. J. 22 (1999), 161–186.CrossRefGoogle Scholar
Mochizuki, S., The local pro- $p$ anabelian geometry of curves , Invent. Math. 138 (1999), 319–423.CrossRefGoogle Scholar
Mochizuki, S., Extending families of curves over log regular schemes , J. Reine Angew. Math. 511 (1999), 43–71.CrossRefGoogle Scholar
Mochizuki, S., The absolute anabelian geometry of canonical curves , Doc. Math. Extra Volume: Kazuya Kato’s Fiftieth Birthday (2003), 609–640.Google Scholar
Mochizuki, S., The geometry of anabelioids , Publ. Res. Inst. Math. Sci. 40 (2004), 819–881.CrossRefGoogle Scholar
Mochizuki, S., Semi-graphs of anabelioids , Publ. Res. Inst. Math. Sci. 42 (2006), 221–322.CrossRefGoogle Scholar
Mochizuki, S., A combinatorial version of the Grothendieck conjecture , Tohoku Math. J. 2 (2007), no. 59, 455–479.Google Scholar
Mochizuki, S., Absolute anabelian cuspidalizations of proper hyperbolic curves , J. Math. Kyoto Univ. 47 (2007), 451–539.Google Scholar
Mochizuki, S., On the combinatorial cuspidalization of hyperbolic curves , Osaka J. Math. 47 (2010), 651–715.Google Scholar
Mochizuki, S., Topics in absolute anabelian geometry II: Decomposition groups and endomorphisms , J. Math. Sci. Univ. Tokyo 20 (2013), 171–269.Google Scholar
Mochizuki, S., Inter-universal Teichmüller theory I: Construction of Hodge theaters , Publ. Res. Inst. Math. Sci. 57 (2021), 3–207.CrossRefGoogle Scholar
Mochizuki, S. and Tamagawa, A., The algebraic and anabelian geometry of configuration spaces , Hokkaido Math. J. 37 (2008), 75–131.CrossRefGoogle Scholar
Nakayama, C. and Ogus, A., Relative rounding in toric and logarithmic geometry , Geom. Topol. 14 (2010), 2189–2241.CrossRefGoogle Scholar
Paris, L., Residual $p$ properties of mapping class groups and surface groups , Trans. Amer. Math. Soc. 361 (2009), 2487–2507.CrossRefGoogle Scholar
Pop, F. and Stix, J., Arithmetic in the fundamental group of a $p$ -adic curve. On the $p$ -adic section conjecture for curves , J. Reine Angew. Math. 725 (2017), 1–40.CrossRefGoogle Scholar
Ribes, L. and Zalesskii, P., Profinite groups, second ed. Ergebnisse der Mathematik Und Ihrer Grenzgebiete. 3. Folge. A Series of Modern Surveys in Mathematics, Vol. 40, Springer, Berlin, 2010.CrossRefGoogle Scholar
Tamagawa, A., The Grothendieck conjecture for affine curves , Compos. Math. 109 (1997), 135–194.CrossRefGoogle Scholar
Tamagawa, A., Resolution of nonsingularities of families of curves , Publ. Res. Inst. Math. Sci. 40 (2004), 1291–1336.CrossRefGoogle Scholar
Zalesskii, P. A. and Mel’nikov, O. V., Subgroups of profinite groups acting on trees , Math. USSR-Sb. 63 (1989), 405–424.CrossRefGoogle Scholar