No CrossRef data available.
Article contents
The boundedness of the bilinear oscillatory integral along a parabola
Published online by Cambridge University Press: 03 April 2023
Abstract
In this paper, the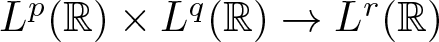 
$L^p(\mathbb{R})\times L^q(\mathbb{R})\rightarrow L^r(\mathbb{R})$ boundedness of the bilinear oscillatory integral along parabolais set up, where β > 1 or β < 0, 
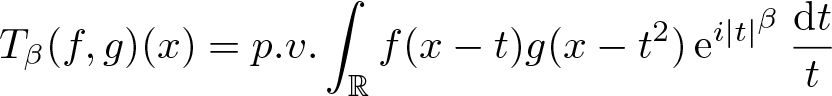\begin{equation*}T_\beta(f, g)(x)=p.v.\int_{{\mathbb R}} f(x-t)g(x-t^{2})\,{\rm e}^{i |t|^{\beta}}\,\frac{{\rm d}t}{t}\end{equation*}
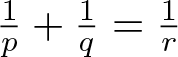$\frac{1}{p}+\frac{1}{q}=\frac{1}{r}$ and 
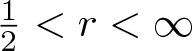$\frac{1}{2}\lt r\lt\infty$, p > 1 and q > 1. The result for the case β < 0 extends the 
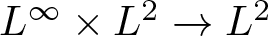$L^\infty\times L^2\to L^2$ boundedness obtained by Fan and Li (D. Fan and X. Li, A bilinear oscillatory integral along parabolas, Positivity 13(2) (2009), 339–366) by confirming an open question raised in it.
MSC classification
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 66 , Issue 1 , February 2023 , pp. 54 - 88
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press on Behalf of The Edinburgh Mathematical Society.
References
Carleson, L., On convergence and growth of partial sums of Fourier series, Acta Math. 116 (1966), 125–157.10.1007/BF02392815CrossRefGoogle Scholar
Coifman, R. and Meyer, Y., On commutators of singular integrals and bilinear singular integrals, Trans. Amer. Math. Soc. 212 (1975), 315–331.10.1090/S0002-9947-1975-0380244-8CrossRefGoogle Scholar
Coifman, R. and Meyer, Y., Ondelettes et opéRatrs III. OpéRateurs multilineaires (Hermann, Paris, 1991).Google Scholar
Fan, D. and Li, X., A bilinear oscillatory integral along parabolas, Positivity 13(2) (2009), 339–366.10.1007/s11117-008-2270-3CrossRefGoogle Scholar
Fefferman, C., Pointwise convergence of Fourier series, Ann. Math. 98(2) (1973), 551–571.10.2307/1970917CrossRefGoogle Scholar
Fefferman, C. and Stein, E., H p spaces of several variables, Acta Math. 229(3–4) (1972), 137–193.10.1007/BF02392215CrossRefGoogle Scholar
Hörmander, L., Oscillatory integrals and multipliers on 
${FL^p}$, Ark. Mat. 11 (1973), 1–11.10.1007/BF02388505CrossRefGoogle Scholar
${FL^p}$, Ark. Mat. 11 (1973), 1–11.10.1007/BF02388505CrossRefGoogle ScholarLacey, M. and Thiele, C., L p estimates on the bilinear Hilbert transform for
$2 \lt p \lt \infty$, Ann. Math. 146 (1997), 693–724.10.2307/2952458CrossRefGoogle Scholar
$2 \lt p \lt \infty$, Ann. Math. 146 (1997), 693–724.10.2307/2952458CrossRefGoogle ScholarLacey, M. and Thiele, C., On Calderón’s conjecture, Ann. Math. 149(2) (1999), 475–496.10.2307/120971CrossRefGoogle Scholar
Li, X., Bilinear Hilbert transforms along curves I: the monomial case, Anal. PDE. 6(1) (2013), 197–220.10.2140/apde.2013.6.197CrossRefGoogle Scholar
Li, X. and Xiao, L., Uniform estimates for bilinear Hilbert transforms and bilinear maximal functions associated to polynomials, Amer. J. Math. 138(4) (2016), 907–962.10.1353/ajm.2016.0030CrossRefGoogle Scholar
Li, J. and Yu, H., Bilinear Hilbert transforms and (sub)bilinear maximal functions along convex curves, Pacific J. Math. 310(2) (2021), 375–446.10.2140/pjm.2021.310.375CrossRefGoogle Scholar
Phong, D. H. and Stein, E. M., On a stopping process for oscillatory integrals, J. Geom. Anal. 4 (1994), 104–120.10.1007/BF02921595CrossRefGoogle Scholar
Sjölin, P., Convolution with oscillating kernels, Indiana Univ. Math. J. 30(1) (1981), 47–55.10.1512/iumj.1981.30.30004CrossRefGoogle Scholar
Wainger, S., Special trigonometric series in k dimensions, Mem. Amer. Math Soc. 59 (1965).Google Scholar