Hostname: page-component-848d4c4894-ndmmz Total loading time: 0 Render date: 2024-06-11T06:03:10.689Z Has data issue: false hasContentIssue false
  • English
  • Français

Fractional interpolation inequality and radially symmetric ground states

Part of: Partial differential equations Linear function spaces and their duals General mathematical topics and methods in quantum theory Miscellaneous topics - Partial differential equations

Published online by Cambridge University Press:  12 May 2022

Li Ma  and
Fangyuan Dong
Li Ma
Affiliation:
School of Mathematics and Physics, University of Science and Technology Beijing, 30 Xueyuan Road, Haidian District, Beijing 100083, China (lma17@ustb.edu.cn,dfangyuan20@163.com)
Fangyuan Dong
Affiliation:
School of Mathematics and Physics, University of Science and Technology Beijing, 30 Xueyuan Road, Haidian District, Beijing 100083, China (lma17@ustb.edu.cn,dfangyuan20@163.com)
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we establish a new fractional interpolation inequality for radially symmetric measurable functions on the whole space $R^{N}$ and a new compact imbedding result about radially symmetric measurable functions. We show that the best constant in the new interpolation inequality can be achieved by a radially symmetric function. As applications of this compactness result, we study the existence of ground states of the nonlinear fractional Schrödinger equation on the whole space $R^{N}$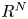. We also prove an existence result of standing waves and prove their orbital stability.

Keywords

Interpolation inequalityfractional Laplaciancompactness resultground statesstanding waves

MSC classification

Primary: 35R11: Fractional partial differential equations
Secondary: 46E35: Sobolev spaces and other spaces of “smooth” functions, embedding theorems, trace theorems 35B65: Smoothness and regularity of solutions 81Q05: Closed and approximate solutions to the Schrödinger, Dirac, Klein-Gordon and other equations of quantum mechanics
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 153 , Issue 3 , June 2023 , pp. 937 - 957
Check for updates
Copyright
Copyright © The Author(s), 2022. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adams, R. A.. Sobolev spaces. Pure and Applied Mathematics vol. 65 (New York-London: Academic Press (A subsidiary of Harcourt Brace Jovanovich Publishers), 1975).Google Scholar
Ao, W. W., Chan, H., Delatorre, A., Fontelos, M., Gonzalez, M. and Wei, J. C.. ODE methods in non-local equations. J. Math. Study 53 (2020), 370–401. A special volume for Prof. A. Chang's 70th birthday.10.4208/jms.v53n4.20.01CrossRefGoogle Scholar
Byeon, J. and Wang, Z.-Q.. On the Hénon equation: asymptotic profile of ground states, II. J. Differ. Equ. 216 (2005), 78–108.10.1016/j.jde.2005.02.018CrossRefGoogle Scholar
Cazenave, T.. Semilinear Schrödinger equations. Lecture Notes in Mathematics vol. 10 (New York: New York University Courant Institute of Mathematical Sciences, 2003).10.1090/cln/010CrossRefGoogle Scholar
Chen, J. and Guo, B.. Sharp constant of an improved Gagliardo-Nirenberg inequality and its application. Ann. Math. Pura Appl. 190 (2011), 341–354.10.1007/s10231-010-0152-3CrossRefGoogle Scholar
Chen, W., Li, Y. and Ma, P.. The fractional Laplacian (Singapore: World Scientific Publishing, 2020). p. 35R11.10.1142/10550CrossRefGoogle Scholar
Chhetri, M. and Girg, P.. Some bifurcation results for fractional Laplacian problems. Nonlinear Anal. 191 (2020), 111642.10.1016/j.na.2019.111642CrossRefGoogle Scholar
Cho, Y. and Ozawa, T.. Sobolev inequalities with symmetry. Commun. Contemp. Math. 11 (2009), 355–365.10.1142/S0219199709003399CrossRefGoogle Scholar
Cho, Y., Hwang, G., Kwon, S. and Lee, S.. On the finite time blowup for mass-critical Hartree equations. Proc. R. Soc. Edinburgh: Sect. A Math. 145 (2015), 467–479.10.1017/S030821051300142XCrossRefGoogle Scholar
Costa, D. G.. An invitation to variational methods in differential equations (Boston: Birkhauser, 2007).CrossRefGoogle Scholar
Di Nezza, E., Palatucci, G. and Valdinoci, E.. Hitchhiker's guide to the fractional Sobolev spaces. Bull. Sci. Math. 136 (2012), 521–573.10.1016/j.bulsci.2011.12.004CrossRefGoogle Scholar
Dyda, B., Ihnatsyeva, L. and Vähäkangas, A. V.. On improved fractional Sobolev-Poincaré inequalities. Ark. Mat. 54 (2016), 437–454.10.1007/s11512-015-0227-xCrossRefGoogle Scholar
Frank, R. L. and Seiringer, R.. Non-linear ground state representations and sharp Hardy inequalities. J. Funct. Anal. 255 (2008), 3407–3430.10.1016/j.jfa.2008.05.015CrossRefGoogle Scholar
Frank, R. L., Lenzmann, E. and Silvestre, L.. Uniqueness of radial solutions for the fractional Laplacian. Commun. Pure Appl. Math. 69 (2016), 1671–1726.10.1002/cpa.21591CrossRefGoogle Scholar
Hajaiej, H., Molica Bisci, G. and Vilasi, L.. Existence results for a critical fractional equation. Asymptot. Anal. 100 (2016), 209–225.Google Scholar
Hajaiej, H., Molinet, L., Ozawa, T. and Wang, B.. Necessary and sufficient conditions for the fractional Gagliardo-Nirenberg inequalities and applications to Navier-Stokes and generalized boson equations. In Harmonic Analysis and Nonlinear Partial Differential Equations, Res. Inst. Math. Sci. (RIMS) vol. B26, pp. 159–175 (Kyoto: RIMS Kôkyûroku Bessatsu, 2011).Google Scholar
Hezzi, H., Marzouk, A. and Saanouni, T.. A note on the inhomogeneous Schrödinger equation with mixed power nonlinearity. Commun. Math. Anal. 18 (2015), 34–53.Google Scholar
Laskin, N.. Fractional quantum mechanics (Hackensack, NJ: World Scientific Publishing Co. Pte. Ltd., 2018).10.1142/10541CrossRefGoogle Scholar
Le Coz, S.. A note on Berestycki-Cazenave's classical instability result for nonlinear Schrödinger equations. Adv. Nonlinear Stud. 8 (2008), 455–463.10.1515/ans-2008-0302CrossRefGoogle Scholar
Li, J. J. and Ma, L.. Extremals to new Gagliardo-Nirenberg inequality and ground states. Appl. Math. Lett. 120 (2021), 107266.10.1016/j.aml.2021.107266CrossRefGoogle Scholar
Li, Y., Wang, Z.-Q. and Zeng, J.. Ground states of nonlinear Schrödinger equations with potentials. Ann. Inst. H. Poincaré Anal. Non Linéaire 23 (2006), 829–837.Google Scholar
Lions, P.-L.. The concentration-compactness principle in the calculus of variations. The limit case. II. Rev. Mat. Iberoam. 1 (1985), 45–121.10.4171/RMI/12CrossRefGoogle Scholar
Lions, P.-L.. Symetrie et compacité dans les espaces de Sobolev. J. Funct. Anal. 49 (1982), 315–334.10.1016/0022-1236(82)90072-6CrossRefGoogle Scholar
Luo, H. and Zhang, Z.. Normalized solutions to the fractional Schrödinger equations with combined nonlinearities. Calc. Var. Partial Differ. Equ. 59 (2020), 143.10.1007/s00526-020-01814-5CrossRefGoogle Scholar
Ma, L.. On nonlocal nonlinear elliptic problems with the fractional Laplacian. Glasgow Math. J. 62 (2020), 75–84.10.1017/S0017089518000538CrossRefGoogle Scholar
Ma, L.. On nonlocal Hénon type problems with the fractional Laplacian. Nonlinear Anal. 203 (2021), 112190.10.1016/j.na.2020.112190CrossRefGoogle Scholar
Ma, L. and Zhang, K.. Ground states of a nonlinear drifting Schrödinger equation. Appl. Math. Lett. 105 (2020), 106324.10.1016/j.aml.2020.106324CrossRefGoogle Scholar
Mallick, A.. Extremals for fractional order Hardy-Sobolev-Maz'ya inequality. Calc. Var. Partial Differ. Equ. 58 (2019), 45.10.1007/s00526-019-1492-5CrossRefGoogle Scholar
Moroz, V. and Van Schaftingen, J.. Groundstates of nonlinear Choquard equations: Hardy-Littlewood-Sobolev critical exponent. Commun. Contemp. Math. 17 (2015), 1550005.10.1142/S0219199715500054CrossRefGoogle Scholar
Musina, R. and Nazarov, A. I.. Fractional Hardy-Sobolev inequalities on half spaces. Nonlinear Anal. 178 (2019), 32–40.CrossRefGoogle Scholar
Nguyen, H. M. and Squassina, M.. Fractional Caffarelli-Kohn-Nirenberg inequalities. J. Funct. Anal. 274 (2018), 2661–2672.10.1016/j.jfa.2017.07.007CrossRefGoogle Scholar
Paul, S. and Santra, S.. On the ground state solution of a fractional Schrödinger equation. Proc. Am. Math. Soc. 147 (2019), 5275–5290.10.1090/proc/14632CrossRefGoogle Scholar
Saanouni, T.. Remarks on the inhomogeneous fractional nonlinear Schrödinger equation. J. Math. Phys. 57 (2016), 081503.10.1063/1.4960045CrossRefGoogle Scholar
Smets, D., Su, J. and Willem, M.. Non-radial ground states for the Hénon equation. Commun. Contemp. Math. 4 (2002), 467–480.10.1142/S0219199702000725CrossRefGoogle Scholar
Tao, T.. Nonlinear dispersive equations, local and global analysis. In CBMS, Regional Conference Series in Math. Number 106 (Providence, RI: American Mathematical Society, 2006).CrossRefGoogle Scholar
Strauss, W. A.. Existence of solitary waves in higher dimensions. Commun. Math. Phys. 55 (1977), 149–162.10.1007/BF01626517CrossRefGoogle Scholar
Zhang, J.. Stability of attractive Bose-Einstein condensate. J. Stat. Phys. 101 (2000), 731–746.10.1023/A:1026437923987CrossRefGoogle Scholar