Hostname: page-component-77c89778f8-gvh9x Total loading time: 0 Render date: 2024-07-24T15:37:03.555Z Has data issue: false hasContentIssue false
  • English
  • Français

Stability of maxima of random variables defined on a Markov chain

Published online by Cambridge University Press:  01 July 2016

Sidney I. Resnick
Sidney I. Resnick*
Affiliation:
Technion—Israel Institute of Technology, Haifa
*
∗ Present address: Stanford University.
Get access Rights & Permissions [Opens in a new window]

Abstract

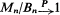Consider maxima Mn of a sequence of random variables defined on a finite Markov chain. Necessary and sufficient conditions for the existence of normalizing constants Bn such that are given. The problem can be reduced to studying maxima of i.i.d. random variables drawn from a finite product of distributions πi=1mHi(x). The effect of each factor Hi(x) on the behavior of maxima from πi=1mHi is analyzed. Under a mild regularity condition, Bn can be chosen to be the maximum of the m quantiles of order (1 - n-1) of the H's.

Keywords

SEMI-MARKOV PROCESSESRELATIVE STABILITY OF MAXIMADISTRIBUTIONS WITH RAPIDLY VARYING TAILSPRODUCTS OF DISTRIBUTIONSMARKOV CHAINS
Type
Research Article
Information
Advances in Applied Probability , Volume 4 , Issue 2 , August 1972 , pp. 285 - 295
Copyright
Copyright © Applied Probability Trust 1972 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Research carried out at Purdue University and supported by the National Science Foundation Graduate Traineeship Program and by N.S.F. Contract GP-7631.

References

[1] Berman, S. (1962) A law of large numbers for the maximum in a stationary Gaussian sequence. Ann. Math. Statist. 33, 93–97.CrossRefGoogle Scholar
[2] de Haan, L. (1970) On Regular Variation and its Applications to the Weak Convergence of Sample Extremes. Mathematical Centre Tracts, No. 32, Amsterdam.Google Scholar
[3] Gnedenko, B. V. (1943) Sur la distribution limite du terme maximum d'une série aléatoire. Ann. Math. 44, 423–453.CrossRefGoogle Scholar
[4] Juncosa, M. L. (1949) The asymptotic behavior of the minimum in a sequence of random variables. Duke Math. J. 16, 609–618.CrossRefGoogle Scholar
[5] Kolmogorov, A. N. and Gnedenko, B. V. (1954) Limit Distributions for Sums of Independent Random Variables. Addison-Wesley, Reading, Massachusetts.Google Scholar
[6] Pyke, R. (1961) Markov renewal processes: definitions and preliminary properties. Ann. Math. Statist. 32, 1231–1242.CrossRefGoogle Scholar
[7] Pyke, R. and Schaufele, R. A. (1964) Limit theorems for Markov renewal processes. Ann. Math. Statist. 35, 1746–1764.CrossRefGoogle Scholar
[8] Resnick, S. I. and Neuts, M. F. (1970) Limit laws for maxima of a sequence of random variables defined on a Markov chain. Adv. Appl. Prob. 2, 323–343.CrossRefGoogle Scholar
[9] Resnick, S. I. (1971) Tail equivalence and its applications. J. Appl. Prob. 8, 136–156.CrossRefGoogle Scholar
[10] Varga, R. S. (1962) Matrix Iterative Analysis. Prentice-Hall, Princeton, New Jersey.Google Scholar