Article contents
A REMARK ON THE SEPARABLE QUOTIENT PROBLEM FOR TOPOLOGICAL GROUPS
Published online by Cambridge University Press: 03 June 2019
Abstract
The Banach–Mazur separable quotient problem asks whether every infinite-dimensional Banach space  $B$ has a quotient space that is an infinite-dimensional separable Banach space. The question has remained open for over 80 years, although an affirmative answer is known in special cases such as when
$B$ has a quotient space that is an infinite-dimensional separable Banach space. The question has remained open for over 80 years, although an affirmative answer is known in special cases such as when  $B$ is reflexive or even a dual of a Banach space. Very recently, it has been shown to be true for dual-like spaces. An analogous problem for topological groups is: Does every infinite-dimensional (in the topological sense) connected (Hausdorff) topological group
$B$ is reflexive or even a dual of a Banach space. Very recently, it has been shown to be true for dual-like spaces. An analogous problem for topological groups is: Does every infinite-dimensional (in the topological sense) connected (Hausdorff) topological group 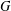 $G$ have a quotient topological group that is infinite dimensional and metrisable? While this is known to be true if
$G$ have a quotient topological group that is infinite dimensional and metrisable? While this is known to be true if 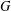 $G$ is the underlying topological group of an infinite-dimensional Banach space, it is shown here to be false even if
$G$ is the underlying topological group of an infinite-dimensional Banach space, it is shown here to be false even if  $G$ is the underlying topological group of an infinite-dimensional locally convex space. Indeed, it is shown that the free topological vector space on any countably infinite
$G$ is the underlying topological group of an infinite-dimensional locally convex space. Indeed, it is shown that the free topological vector space on any countably infinite 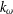 $k_{\unicode[STIX]{x1D714}}$-space is an infinite-dimensional toplogical vector space which does not have any quotient topological group that is infinite dimensional and metrisable. By contrast, the Graev free abelian topological group and the Graev free topological group on any infinite connected Tychonoff space, both of which are connected topological groups, are shown here to have the tubby torus
$k_{\unicode[STIX]{x1D714}}$-space is an infinite-dimensional toplogical vector space which does not have any quotient topological group that is infinite dimensional and metrisable. By contrast, the Graev free abelian topological group and the Graev free topological group on any infinite connected Tychonoff space, both of which are connected topological groups, are shown here to have the tubby torus 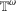 $\mathbb{T}^{\unicode[STIX]{x1D714}}$, which is an infinite-dimensional metrisable group, as a quotient group.
$\mathbb{T}^{\unicode[STIX]{x1D714}}$, which is an infinite-dimensional metrisable group, as a quotient group.
Keywords
MSC classification
- Type
- Research Article
- Information
- Bulletin of the Australian Mathematical Society , Volume 100 , Issue 3 , December 2019 , pp. 453 - 457
- Copyright
- © 2019 Australian Mathematical Publishing Association Inc.
References
- 2
- Cited by


