No CrossRef data available.
Article contents
CHARACTERISATION OF PRIMES DIVIDING THE INDEX OF A CLASS OF POLYNOMIALS AND ITS APPLICATIONS
Published online by Cambridge University Press: 01 April 2024
Abstract
Let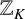 
${\mathbb {Z}}_{K}$ denote the ring of algebraic integers of an algebraic number field 
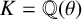$K = {\mathbb Q}(\theta )$, where 
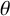$\theta $ is a root of a monic irreducible polynomial 
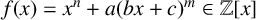$f(x) = x^n + a(bx+c)^m \in {\mathbb {Z}}[x]$, 
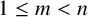$1\leq m<n$. We say 
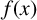$f(x)$ is monogenic if 
$\{1, \theta , \ldots , \theta ^{n-1}\}$ is a basis for 
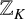${\mathbb {Z}}_K$. We give necessary and sufficient conditions involving only 
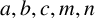$a, b, c, m, n$ for 
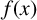$f(x)$ to be monogenic. Moreover, we characterise all the primes dividing the index of the subgroup 
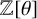${\mathbb {Z}}[\theta ]$ in 
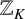${\mathbb {Z}}_K$. As an application, we also provide a class of monogenic polynomials having non square-free discriminant and Galois group 
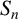$S_n$, the symmetric group on n letters.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.
Footnotes
The author is thankful to IIT Madras for NFIG grant RF/22-23/1035/MA/NFIG/009034.
Dedicated to Professor Sudesh Kaur Khanduja
References
Bishnoi, A. and Khanduja, S. K., ‘A class of trinomials with Galois group
${S}_n$
’, Algebra Colloq. 19(1) (2012), 905–911.CrossRefGoogle Scholar
${S}_n$
’, Algebra Colloq. 19(1) (2012), 905–911.CrossRefGoogle ScholarCohen, H., A Course in Computational Algebraic Number Theory (Springer-Verlag, Berlin–Heidelberg, 1993).CrossRefGoogle Scholar
Dedekind, R., ‘Über den Zusammenhang zwischen der Theorie der Ideale und der Theorie der höheren Kongruenzen’, Götttingen Abh. 23 (1878), 1–23.Google Scholar
Filaseta, M. and Moy, R., ‘On the Galois group over
$\mathbb{Q}$
of a truncated binomial expansion’, Colloq. Math. 154 (2018), 295–308.CrossRefGoogle Scholar
$\mathbb{Q}$
of a truncated binomial expansion’, Colloq. Math. 154 (2018), 295–308.CrossRefGoogle ScholarGaál, I., Diophantine Equations and Power Integral Bases: Theory and Algorithms, 2nd edn (Birkhäuser/Springer, Cham, 2019).CrossRefGoogle Scholar
Jakhar, A., Khanduja, S. K. and Sangwan, N., ‘On prime divisors of the index of an algebraic integer’, J. Number Theory 166 (2016), 47–61.CrossRefGoogle Scholar
Jakhar, A., Khanduja, S. K. and Sangwan, N., ‘Characterisation of primes dividing the index of a trinomial’, Int. J. Number Theory 13(10) (2017), 2505–2514.CrossRefGoogle Scholar
Jones, L., ‘A brief note on some infinite families of monogenic polynomials’, Bull. Aust. Math. Soc. 100 (2019), 239–244.CrossRefGoogle Scholar
Jones, L., ‘On necessary and sufficient conditions for the monogenity of a certain class of polynomials’, Math. Slovaca 72(3) (2022), 591–600.CrossRefGoogle Scholar
Jones, L., ‘Infinite families of monogenic quadrinomials, quintinomials and sextinomials’, Colloq. Math. 169 (2022), 1–10.CrossRefGoogle Scholar
Kaur, S. and Kumar, S., ‘On a conjecture of Lenny Jones about certain monogenic polynomials’, Bull. Aust. Math. Soc., to appear. Published online (21 November 2023).CrossRefGoogle Scholar