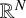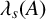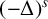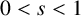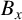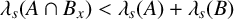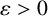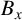1 Introduction
Let
![]() $\Omega $
be an open subset of
$\Omega $
be an open subset of
![]() $\mathbb {R}^N$
. Denote by
$\mathbb {R}^N$
. Denote by
![]() $\lambda (\Omega )$
the lowest eigenvalue of the Laplace operator
$\lambda (\Omega )$
the lowest eigenvalue of the Laplace operator
![]() $-\Delta $
in
$-\Delta $
in
![]() $\Omega $
with Dirichlet boundary conditions. It is well known that if
$\Omega $
with Dirichlet boundary conditions. It is well known that if
![]() $\lambda (\Omega )$
is small, then
$\lambda (\Omega )$
is small, then
![]() $\Omega $
must be ‘large’. For example, when
$\Omega $
must be ‘large’. For example, when
![]() $\Omega $
is empty, then
$\Omega $
is empty, then
![]() $\lambda (\Omega )=+\infty $
. The Faber–Krahn inequality for the Laplace operator states that
$\lambda (\Omega )=+\infty $
. The Faber–Krahn inequality for the Laplace operator states that
where
![]() $C_N$
is the lowest eigenvalue of a ball with unit volume and
$C_N$
is the lowest eigenvalue of a ball with unit volume and
![]() $\lvert \Omega \rvert $
is the volume of
$\lvert \Omega \rvert $
is the volume of
![]() $\Omega $
[Reference Faber3, Reference Krahn5]. In other words, among all domains with fixed volume, the ball has the smallest
$\Omega $
[Reference Faber3, Reference Krahn5]. In other words, among all domains with fixed volume, the ball has the smallest
![]() $\lambda $
.
$\lambda $
.
Geometrically, when
![]() $\lambda (\Omega )$
is small,
$\lambda (\Omega )$
is small,
![]() $\Omega $
is not only large, but also ‘fat’ in some sense. As shown in [Reference Lieb6], the inequality
$\Omega $
is not only large, but also ‘fat’ in some sense. As shown in [Reference Lieb6], the inequality
![]() $\lambda (\Omega )\geq \alpha _NR^{-2}$
, where R is the radius of the largest ball contained in
$\lambda (\Omega )\geq \alpha _NR^{-2}$
, where R is the radius of the largest ball contained in
![]() $\Omega $
, is not true when
$\Omega $
, is not true when
![]() $N>1$
. This implies that
$N>1$
. This implies that
![]() $\Omega $
need not contain any ball of fixed radius R, even if
$\Omega $
need not contain any ball of fixed radius R, even if
![]() $\lambda (\Omega )$
is sufficiently small. Nevertheless, if
$\lambda (\Omega )$
is sufficiently small. Nevertheless, if
![]() $\lambda (\Omega )$
is small, then
$\lambda (\Omega )$
is small, then
![]() $\Omega $
contains ‘most of’ a ball of radius
$\Omega $
contains ‘most of’ a ball of radius
![]() $R\sim \lambda ^{-1/2}(\Omega )$
. This assertion is derived from the following inequality: for any
$R\sim \lambda ^{-1/2}(\Omega )$
. This assertion is derived from the following inequality: for any
![]() $\varepsilon \in (0,1)$
, there exists
$\varepsilon \in (0,1)$
, there exists
![]() $\alpha _N(\varepsilon )$
, with
$\alpha _N(\varepsilon )$
, with
![]() $\alpha _N(\varepsilon )\to 0$
as
$\alpha _N(\varepsilon )\to 0$
as
![]() $\varepsilon \to 1$
, such that
$\varepsilon \to 1$
, such that
where R is the largest radius such that
![]() $\lvert \Omega \cap B_R\rvert \geq \varepsilon \lvert B_R\rvert $
for some ball
$\lvert \Omega \cap B_R\rvert \geq \varepsilon \lvert B_R\rvert $
for some ball
![]() $B_R$
. More precisely, fix
$B_R$
. More precisely, fix
![]() $\varepsilon \in (0,1)$
(
$\varepsilon \in (0,1)$
(
![]() $\varepsilon $
may be close to
$\varepsilon $
may be close to
![]() $1$
) and let
$1$
) and let
![]() $R>0$
be the largest radius such that
$R>0$
be the largest radius such that
![]() $\lvert \Omega \cap B_R\rvert \geq \varepsilon \lvert B_R\rvert $
for some ball
$\lvert \Omega \cap B_R\rvert \geq \varepsilon \lvert B_R\rvert $
for some ball
![]() $B_R$
. By (1.1),
$B_R$
. By (1.1),
This means that
![]() $\Omega $
contains ‘most of’ the ball
$\Omega $
contains ‘most of’ the ball
![]() $B_{\sqrt {\alpha _N(\varepsilon )}\lambda ^{-{1}/{2}}(\Omega )}$
of large radius when
$B_{\sqrt {\alpha _N(\varepsilon )}\lambda ^{-{1}/{2}}(\Omega )}$
of large radius when
![]() $\lambda (\Omega )$
is small.
$\lambda (\Omega )$
is small.
The inequality (1.1) follows from [Reference Lieb6, Corollary 2] which is a consequence of the following theorem.
Theorem 1.1. Let A and B be nonempty open sets in
![]() $\mathbb R^N$
,
$\mathbb R^N$
,
![]() $N\geq 1$
, and let
$N\geq 1$
, and let
![]() $\lambda (A)$
,
$\lambda (A)$
,
![]() $\lambda (B)$
be the corresponding lowest eigenvalues of the Laplacian
$\lambda (B)$
be the corresponding lowest eigenvalues of the Laplacian
![]() $-\Delta $
with Dirichlet boundary conditions. Let
$-\Delta $
with Dirichlet boundary conditions. Let
![]() $B_x=x+B$
denote B translated by
$B_x=x+B$
denote B translated by
![]() $x\in \mathbb R^N$
. Let
$x\in \mathbb R^N$
. Let
![]() $\varepsilon>0$
. Then, there exists an x such that
$\varepsilon>0$
. Then, there exists an x such that
If A and B are both bounded, then there is an
![]() $x\in \mathbb R^N$
such that
$x\in \mathbb R^N$
such that
Moreover, the mapping
![]() $x\mapsto \lambda (A\cap B_x)$
is upper-semicontinuous, so that the set of x for which (1.2) or (1.3) holds is open.
$x\mapsto \lambda (A\cap B_x)$
is upper-semicontinuous, so that the set of x for which (1.2) or (1.3) holds is open.
Lieb [Reference Lieb6] used Theorem 1.1 to prove two important corollaries: a lower bound for
![]() $\sup _x\{\mathrm {Volume}(A\cap B_x) \}$
in terms of
$\sup _x\{\mathrm {Volume}(A\cap B_x) \}$
in terms of
![]() $\lambda (A)$
when B is a ball (see [Reference Lieb6, Corollary 2]) and a compactness lemma for certain sequences in
$\lambda (A)$
when B is a ball (see [Reference Lieb6, Corollary 2]) and a compactness lemma for certain sequences in
![]() $W^{1,p}(\mathbb R^N) $
that under some conditions, a bounded sequence in
$W^{1,p}(\mathbb R^N) $
that under some conditions, a bounded sequence in
![]() $W^{1,p}(\mathbb R^N) $
has a nonzero weak limit (see [Reference Lieb6, Lemma 6]).
$W^{1,p}(\mathbb R^N) $
has a nonzero weak limit (see [Reference Lieb6, Lemma 6]).
Inspired by [Reference Lieb6], our purpose in this paper is to generalise the main result in [Reference Lieb6] for the fractional Laplacian
![]() $(-\Delta )^s$
,
$(-\Delta )^s$
,
![]() $0<s<1$
. The fractional Laplacian
$0<s<1$
. The fractional Laplacian
![]() $(-\Delta )^s$
is defined as a nonlocal pseudodifferential operator on the space of rapidly decreasing functions by
$(-\Delta )^s$
is defined as a nonlocal pseudodifferential operator on the space of rapidly decreasing functions by
 $$ \begin{align*} (-\Delta)^su(x)=c_{N,s}P.V.\int_{\mathbb{R}^N}\frac{u(x)-u(\xi)}{\lvert x-\xi\rvert^{N+2s}}\,d\xi=c_{N,s} \lim_{\varepsilon\to 0}\int_{\mathbb{R}^N\setminus B(x,\varepsilon)}\frac{u(x)-u(\xi)}{\lvert x-\xi\rvert^{N+2s}}\,d\xi, \end{align*} $$
$$ \begin{align*} (-\Delta)^su(x)=c_{N,s}P.V.\int_{\mathbb{R}^N}\frac{u(x)-u(\xi)}{\lvert x-\xi\rvert^{N+2s}}\,d\xi=c_{N,s} \lim_{\varepsilon\to 0}\int_{\mathbb{R}^N\setminus B(x,\varepsilon)}\frac{u(x)-u(\xi)}{\lvert x-\xi\rvert^{N+2s}}\,d\xi, \end{align*} $$
where
![]() $c_{N,s}$
is a normalisation constant. By using the Fourier transform, the fractional Laplacian can also be defined as
$c_{N,s}$
is a normalisation constant. By using the Fourier transform, the fractional Laplacian can also be defined as
In the distributional sense, the fractional Laplacian can be extended on
![]() $\mathcal L_s(\mathbb R^N)$
, where
$\mathcal L_s(\mathbb R^N)$
, where
by
Some elementary properties of the fractional Laplacian can be found in [Reference Di Nezza, Palatucci and Valdinoci2]. Recent progress on the eigenvalue bounds for the fractional Laplacian and the fractional Schrödinger operator can be found in the survey [Reference Frank, Palatucci and Kuusi4] and the references given therein.
Denote by
![]() $\overset {.}{H^s}(\mathbb {R}^N)$
the space of functions
$\overset {.}{H^s}(\mathbb {R}^N)$
the space of functions
![]() $f\in L^2(\mathbb R^N)$
such that
$f\in L^2(\mathbb R^N)$
such that
 $$ \begin{align} \lVert f\rVert^2_{\overset{.}{H^s}(\mathbb R^N)}=\iint_{\mathbb R^{2N}}\frac{(f(x)-f(y))^2}{\lvert x-y\rvert^{N+2s}}\,dx\,dy<\infty. \end{align} $$
$$ \begin{align} \lVert f\rVert^2_{\overset{.}{H^s}(\mathbb R^N)}=\iint_{\mathbb R^{2N}}\frac{(f(x)-f(y))^2}{\lvert x-y\rvert^{N+2s}}\,dx\,dy<\infty. \end{align} $$
For
![]() $A\subset \mathbb {R}^N$
, we define the space
$A\subset \mathbb {R}^N$
, we define the space
![]() $H_0^s(A)$
as the completion of
$H_0^s(A)$
as the completion of
![]() $C_0^\infty (A)$
with norm
$C_0^\infty (A)$
with norm
![]() $ \lVert \cdot \rVert _{\overset {.}{H^s}(\mathbb R^N)}$
and
$ \lVert \cdot \rVert _{\overset {.}{H^s}(\mathbb R^N)}$
and
We can now state our main result.
Theorem 1.2. Let
![]() $0<s<1$
. Suppose that A and B are two nonempty open sets in
$0<s<1$
. Suppose that A and B are two nonempty open sets in
![]() $\mathbb R^N$
,
$\mathbb R^N$
,
![]() $N\geq 1$
. Let
$N\geq 1$
. Let
![]() $\lambda _s(A)$
and
$\lambda _s(A)$
and
![]() $\lambda _s(B)$
be the corresponding lowest eigenvalues of the fractional Laplacian
$\lambda _s(B)$
be the corresponding lowest eigenvalues of the fractional Laplacian
![]() $(-\Delta )^s$
with Dirichlet boundary conditions. Then, the following assertions hold.
$(-\Delta )^s$
with Dirichlet boundary conditions. Then, the following assertions hold.
-
(i) For any
 $\varepsilon>0$
, there exists an
$\varepsilon>0$
, there exists an
 $x\in \mathbb R^N$
such that
$x\in \mathbb R^N$
such that  $$ \begin{align*} \lambda_s(A\cap B_x)<\lambda_s(A)+\lambda_s(B)+\varepsilon, \end{align*} $$
$$ \begin{align*} \lambda_s(A\cap B_x)<\lambda_s(A)+\lambda_s(B)+\varepsilon, \end{align*} $$
where
 $B_x$
is the translate of B by x.
$B_x$
is the translate of B by x. -
(ii) In addition, if A and B are bounded, then there is an
 $x\in \mathbb R^N$
such that
$x\in \mathbb R^N$
such that  $$ \begin{align*} \lambda_s(A\cap B_x)<\lambda_s(A)+\lambda_s(B). \end{align*} $$
$$ \begin{align*} \lambda_s(A\cap B_x)<\lambda_s(A)+\lambda_s(B). \end{align*} $$
-
(iii) The mapping
 $x\mapsto \lambda _s(A\cap B_x)$
is upper-semicontinuous.
$x\mapsto \lambda _s(A\cap B_x)$
is upper-semicontinuous.
As applications of Theorem 1.2, by the same arguments as [Reference Lieb6, Corollary 2], we can first prove a lower bound for
![]() $\sup _x\{\mathrm {Volume}(A\cap B_x) \}$
in terms of
$\sup _x\{\mathrm {Volume}(A\cap B_x) \}$
in terms of
![]() $\lambda _s (A)$
when B is a ball.
$\lambda _s (A)$
when B is a ball.
Corollary 1.3. Let A be a nonempty open set in
![]() $\mathbb R^N$
,
$\mathbb R^N$
,
![]() $N\geq 1$
. Let
$N\geq 1$
. Let
![]() $B_r$
be a ball of radius r. Given
$B_r$
be a ball of radius r. Given
![]() $\delta $
with
$\delta $
with
![]() $0<\delta <1$
, put
$0<\delta <1$
, put
where
![]() $w_N$
is the volume of the unit ball and
$w_N$
is the volume of the unit ball and
![]() $\alpha _{N,s}$
is the lowest eigenvalue of
$\alpha _{N,s}$
is the lowest eigenvalue of
![]() $(-\Delta )^s$
on a ball of unit volume. Suppose that for
$(-\Delta )^s$
on a ball of unit volume. Suppose that for
![]() $R>0$
,
$R>0$
,
Then, for every r with
![]() $0<r<R$
, there is an
$0<r<R$
, there is an
![]() $x\in \mathbb R^N$
and a ball
$x\in \mathbb R^N$
and a ball
![]() $B(x,r)$
of radius r centred at x such that
$B(x,r)$
of radius r centred at x such that
Second, we can use Theorem 1.2 to give a different proof of the compactness lemma (see [Reference Bellazzini, Frank and Visciglia1, Lemma 2.1]) in the space
![]() $H^s(\mathbb R^N)$
with
$H^s(\mathbb R^N)$
with
![]() $0<s<1$
. The proof is similar to the one of [Reference Lieb6, Lemma 6] and we omit the details. The proof of Theorem 1.2 is inspired by the approach in [Reference Lieb6]. However, because of the presence of the fractional Laplacian, some difficulties arise. For instance, the product rule for derivatives cannot be applied and one needs to use delicate integral estimates (see the proof below).
$0<s<1$
. The proof is similar to the one of [Reference Lieb6, Lemma 6] and we omit the details. The proof of Theorem 1.2 is inspired by the approach in [Reference Lieb6]. However, because of the presence of the fractional Laplacian, some difficulties arise. For instance, the product rule for derivatives cannot be applied and one needs to use delicate integral estimates (see the proof below).
The rest of this paper is devoted to the proof of Theorem 1.2.
2 Proof of Theorem 1.2
In this section, we give the proof of our main result. We begin with the first assertion in Theorem 1.2.
2.1 Proof of assertion (i)
By the definition,
 $$ \begin{align*} \lambda_s(A)&=\inf\{\lVert f\rVert^2_{\overset{.}{H^s}(\mathbb R^N)} : f\in H_0^s(A), \lVert f\rVert_{L^2(A)}=1 \mbox{ and } f\not\equiv 0\}\\ &=\inf\{\lVert f\rVert^2_{\overset{.}{H^s}(\mathbb R^N)}: f\in C_0^\infty(A), \lVert f\rVert_{L^2(A)}=1 \mbox{ and } f\not\equiv 0\}, \end{align*} $$
$$ \begin{align*} \lambda_s(A)&=\inf\{\lVert f\rVert^2_{\overset{.}{H^s}(\mathbb R^N)} : f\in H_0^s(A), \lVert f\rVert_{L^2(A)}=1 \mbox{ and } f\not\equiv 0\}\\ &=\inf\{\lVert f\rVert^2_{\overset{.}{H^s}(\mathbb R^N)}: f\in C_0^\infty(A), \lVert f\rVert_{L^2(A)}=1 \mbox{ and } f\not\equiv 0\}, \end{align*} $$
where we have used the density of
![]() $C_0^{\infty }(A)$
in
$C_0^{\infty }(A)$
in
![]() $H_0^s(A)$
.
$H_0^s(A)$
.
For
![]() $\varepsilon>0$
, there are real-valued functions
$\varepsilon>0$
, there are real-valued functions
![]() $f\in C^\infty _0(A)$
and
$f\in C^\infty _0(A)$
and
![]() $g\in C^\infty _0(B)$
such that
$g\in C^\infty _0(B)$
such that
Following [Reference Lieb6], given
![]() $x\in \mathbb R^N$
, we define
$x\in \mathbb R^N$
, we define
![]() $h_x(y)=f(y)g(y-x)$
, for
$h_x(y)=f(y)g(y-x)$
, for
![]() $y\in \mathbb R^N$
, and note that
$y\in \mathbb R^N$
, and note that
![]() $h_x(y)$
belongs to
$h_x(y)$
belongs to
![]() $C^\infty _0(A\cap B_x)$
. Put
$C^\infty _0(A\cap B_x)$
. Put
By Fubini’s theorem,
We next estimate
![]() $\lVert h_x\rVert _{\overset {.}{H^s}(\mathbb R^N)}$
. In the local case
$\lVert h_x\rVert _{\overset {.}{H^s}(\mathbb R^N)}$
. In the local case
![]() $s=1$
, by using the product rule
$s=1$
, by using the product rule
![]() $\nabla h_x(y)=\nabla f(y)g(y-x)+f(y) \nabla g(y-x)$
, Lieb proved that
$\nabla h_x(y)=\nabla f(y)g(y-x)+f(y) \nabla g(y-x)$
, Lieb proved that
This is the key estimate in the proof of Lieb [Reference Lieb6]. However, in the case of the fractional Laplacian, one cannot use the product rule as above. Instead, we use the integral representation of the fractional norm and some integral estimates. We shall establish the following inequality:
which is also the key in our proof. From the definition (1.4),
 $$ \begin{align} \begin{split} \lVert h_x\rVert^2_{\overset{.}{H^s}(\mathbb R^N)} &=\iint_{\mathbb R^{2N}}\frac{(f(y)g(y-x)-f(z)g(z-x))^2}{\lvert y-z\rvert^{N+2s}}\,dz\,dy\\ &=\iint_{\mathbb R^{2N}}\frac{(f(y)-f(z))g(y-x)+f(z)(g(y-x)-g(z-x))^2}{\lvert y-z\rvert^{N+2s}}\,dz\,dy\\ &=I_1(x)+I_2(x)+2I_3(x), \end{split} \end{align} $$
$$ \begin{align} \begin{split} \lVert h_x\rVert^2_{\overset{.}{H^s}(\mathbb R^N)} &=\iint_{\mathbb R^{2N}}\frac{(f(y)g(y-x)-f(z)g(z-x))^2}{\lvert y-z\rvert^{N+2s}}\,dz\,dy\\ &=\iint_{\mathbb R^{2N}}\frac{(f(y)-f(z))g(y-x)+f(z)(g(y-x)-g(z-x))^2}{\lvert y-z\rvert^{N+2s}}\,dz\,dy\\ &=I_1(x)+I_2(x)+2I_3(x), \end{split} \end{align} $$
where
 $$ \begin{align*} &I_1(x):=\iint_{\mathbb R^{2N}}\frac{(f(y)-f(z))^2g^2(y-x)}{\lvert y-z\rvert^{N+2s}}\,dz\,dy,\\ &I_2(x):=\iint_{\mathbb R^{2N}}\frac{f^2(z)(g(y-x)-g(z-x))^2}{\lvert y-z\rvert^{N+2s}}\,dz\,dy,\\ &I_3(x):=\iint_{\mathbb R^{2N}}\frac{(f(y)-f(z))f(z)g(y-x)(g(y-x)-g(z-x))}{\lvert y-z\rvert^{N+2s}}\,dz\,dy. \end{align*} $$
$$ \begin{align*} &I_1(x):=\iint_{\mathbb R^{2N}}\frac{(f(y)-f(z))^2g^2(y-x)}{\lvert y-z\rvert^{N+2s}}\,dz\,dy,\\ &I_2(x):=\iint_{\mathbb R^{2N}}\frac{f^2(z)(g(y-x)-g(z-x))^2}{\lvert y-z\rvert^{N+2s}}\,dz\,dy,\\ &I_3(x):=\iint_{\mathbb R^{2N}}\frac{(f(y)-f(z))f(z)g(y-x)(g(y-x)-g(z-x))}{\lvert y-z\rvert^{N+2s}}\,dz\,dy. \end{align*} $$
By Fubini’s theorem,
 $$ \begin{align*} \int_{\mathbb R^N}I_1(x)\,dx= \iint_{\mathbb R^{2N}}\frac{(f(y)-f(z))^2}{\lvert y-z\rvert^{N+2s}}\,dz\,dy\int_{\mathbb R^N}g^2(y-x)\,dx=\lVert f\rVert^2_{\overset{.}{H^s}(\mathbb R^N)}. \end{align*} $$
$$ \begin{align*} \int_{\mathbb R^N}I_1(x)\,dx= \iint_{\mathbb R^{2N}}\frac{(f(y)-f(z))^2}{\lvert y-z\rvert^{N+2s}}\,dz\,dy\int_{\mathbb R^N}g^2(y-x)\,dx=\lVert f\rVert^2_{\overset{.}{H^s}(\mathbb R^N)}. \end{align*} $$
However, by a change of variable,
 $$ \begin{align*} I_2(x)&=\iint_{\mathbb R^{2N}}\frac{f^2(z)(g(y-x)-g(z-x))^2}{\lvert y-z\rvert^{N+2s}}\,dz\,dy\\ &=\iint_{\mathbb R^{2N}}\frac{f^2(z+x)(g(y)-g(z))^2}{\lvert y-z\rvert^{N+2s}}\,dz\,dy. \end{align*} $$
$$ \begin{align*} I_2(x)&=\iint_{\mathbb R^{2N}}\frac{f^2(z)(g(y-x)-g(z-x))^2}{\lvert y-z\rvert^{N+2s}}\,dz\,dy\\ &=\iint_{\mathbb R^{2N}}\frac{f^2(z+x)(g(y)-g(z))^2}{\lvert y-z\rvert^{N+2s}}\,dz\,dy. \end{align*} $$
Using Fubini’s theorem again,
For
![]() $I_3$
, after a change of variable
$I_3$
, after a change of variable
![]() $y=z+h$
, we obtain
$y=z+h$
, we obtain
It follows from Fubini’s theorem that
where
and
Applying Hölder’s inequality,
 $$ \begin{align*} \int_{\mathbb R^N}g(x)g(x-h)\,dx\leq \bigg(\int_{\mathbb R^N}g^2(x)\,dx\int_{\mathbb R^N}g^2(x-h)\,dx\bigg)^{1/2}=\int_{\mathbb R^N}g^2(x)\,dx. \end{align*} $$
$$ \begin{align*} \int_{\mathbb R^N}g(x)g(x-h)\,dx\leq \bigg(\int_{\mathbb R^N}g^2(x)\,dx\int_{\mathbb R^N}g^2(x-h)\,dx\bigg)^{1/2}=\int_{\mathbb R^N}g^2(x)\,dx. \end{align*} $$
This implies that
![]() $K(h)\geq 0$
. In the same way, we also obtain
$K(h)\geq 0$
. In the same way, we also obtain
![]() $L(h)\leq 0.$
Consequently,
$L(h)\leq 0.$
Consequently,
Combining the integral estimates of
![]() $I_1,I_2, I_3$
and (2.2), we arrive at the estimate (2.1). Using (2.1) and the choice of f and g, we obtain
$I_1,I_2, I_3$
and (2.2), we arrive at the estimate (2.1). Using (2.1) and the choice of f and g, we obtain
Hence,
which yields
![]() $0\leq \lVert h_x\rVert ^2_{\overset {.}{H^s}(\mathbb R^N)}<(\lambda _s(A)+\lambda _s(B)+\varepsilon )D(x)$
on a set of positive measure. By the definition, we get
$0\leq \lVert h_x\rVert ^2_{\overset {.}{H^s}(\mathbb R^N)}<(\lambda _s(A)+\lambda _s(B)+\varepsilon )D(x)$
on a set of positive measure. By the definition, we get
for x in a set of positive measure. The existence of x is proved.
2.2 Proof of assertion (ii)
Suppose that A and B are bounded. Then the embedding
![]() $L^2(A)\to H^s_0(A)$
is compact (see, for example, [Reference Di Nezza, Palatucci and Valdinoci2]). It follows that there is a function
$L^2(A)\to H^s_0(A)$
is compact (see, for example, [Reference Di Nezza, Palatucci and Valdinoci2]). It follows that there is a function
![]() $\tilde {f}\in H^s_0(A)$
such that
$\tilde {f}\in H^s_0(A)$
such that
![]() $\lVert \tilde {f}\rVert _{L^2(A)}=1$
and
$\lVert \tilde {f}\rVert _{L^2(A)}=1$
and
Similarly, there exists
![]() $\tilde {g}\in H^s_0(B)$
satisfying
$\tilde {g}\in H^s_0(B)$
satisfying
![]() $\lVert \tilde {g}\rVert _{L^2(B)}=1$
and
$\lVert \tilde {g}\rVert _{L^2(B)}=1$
and
As above, we also define
Applying Fubini’s theorem,
This implies that
![]() $\tilde {D}(x)<\infty $
for almost all
$\tilde {D}(x)<\infty $
for almost all
![]() $x\in \mathbb R^N$
or
$x\in \mathbb R^N$
or
![]() $\tilde {h}_x\in L^2(\mathbb R^N)$
for almost all
$\tilde {h}_x\in L^2(\mathbb R^N)$
for almost all
![]() $x\in \mathbb R^N$
.
$x\in \mathbb R^N$
.
Define again
![]() $\lVert \tilde {h}_x\rVert ^2_{\overset {.}{H^s}(\mathbb R^N)}$
as in (2.2). Using the same argument as for
$\lVert \tilde {h}_x\rVert ^2_{\overset {.}{H^s}(\mathbb R^N)}$
as in (2.2). Using the same argument as for
![]() $\lVert h_x\rVert ^2_{\overset {.}{H^s}(\mathbb R^N)}$
above, we also obtain
$\lVert h_x\rVert ^2_{\overset {.}{H^s}(\mathbb R^N)}$
above, we also obtain
This implies that
![]() $\lVert \tilde {h}_x\rVert _{\overset {.}{H^s}(\mathbb R^N)}<\infty $
for almost all
$\lVert \tilde {h}_x\rVert _{\overset {.}{H^s}(\mathbb R^N)}<\infty $
for almost all
![]() $x\in \mathbb R^N$
or
$x\in \mathbb R^N$
or
![]() $\tilde {h}_x\in \overset {.}{H^s}(\mathbb R^N)$
for almost all
$\tilde {h}_x\in \overset {.}{H^s}(\mathbb R^N)$
for almost all
![]() $x\in \mathbb R^N$
.
$x\in \mathbb R^N$
.
Combining (2.3) and (2.4), we arrive at
which gives
on a set of positive measure. Consequently,
It remains to show the strict inequality. It is sufficient to prove that
is not true for almost all x. Indeed, denote by
![]() $\chi _A$
the characteristic function of the set A. Put
$\chi _A$
the characteristic function of the set A. Put
Then,
![]() $\chi \in C_0(\mathbb R^N)$
. Given any
$\chi \in C_0(\mathbb R^N)$
. Given any
![]() $\varepsilon>0$
, there exists an open set C such that
$\varepsilon>0$
, there exists an open set C such that
![]() $0<\chi (x)<\varepsilon $
and both
$0<\chi (x)<\varepsilon $
and both
![]() $\tilde {f}(y)$
and
$\tilde {f}(y)$
and
![]() $\tilde {g}(y-x)$
are positive in
$\tilde {g}(y-x)$
are positive in
![]() $A\cap B_x$
for all
$A\cap B_x$
for all
![]() $x\in C$
(we can choose connected components of A and B if necessary). Hence,
$x\in C$
(we can choose connected components of A and B if necessary). Hence,
![]() $\tilde {D}(x)>0$
on C and if
$\tilde {D}(x)>0$
on C and if
![]() $\lVert \tilde {h}_x\rVert ^2_{\overset {.}{H^s}(\mathbb R^N)}= (\lambda _s(A)+\lambda _s(B))\tilde {D}(x)$
on C, then
$\lVert \tilde {h}_x\rVert ^2_{\overset {.}{H^s}(\mathbb R^N)}= (\lambda _s(A)+\lambda _s(B))\tilde {D}(x)$
on C, then
However, by the fractional Faber–Krahn inequality (see, for example, [Reference Frank, Palatucci and Kuusi4]),
Letting
![]() $\varepsilon \downarrow 0$
, we obtain a contradiction from (2.5) and (2.6).
$\varepsilon \downarrow 0$
, we obtain a contradiction from (2.5) and (2.6).
2.3 Proof of assertion (iii)
It is not difficult to see that
 $$ \begin{align*} \lambda_s(A\cap B_x)=\inf\bigg\{\frac{\lVert h_x\rVert^2_{\overset{.}{H^s}(\mathbb R^N)}}{D(x)}: h_x=f(\cdot)g(\cdot-x), f\in C^\infty_0(A), g\in C^\infty_0(B)\bigg\}, \end{align*} $$
$$ \begin{align*} \lambda_s(A\cap B_x)=\inf\bigg\{\frac{\lVert h_x\rVert^2_{\overset{.}{H^s}(\mathbb R^N)}}{D(x)}: h_x=f(\cdot)g(\cdot-x), f\in C^\infty_0(A), g\in C^\infty_0(B)\bigg\}, \end{align*} $$
where the function
![]() ${\lVert h_x\rVert ^2_{\overset {.}{H^s}(\mathbb R^N)}}/{D(x)}$
is the quotient of two continuous functions and then is extended to an upper-semicontinuous function by putting
${\lVert h_x\rVert ^2_{\overset {.}{H^s}(\mathbb R^N)}}/{D(x)}$
is the quotient of two continuous functions and then is extended to an upper-semicontinuous function by putting
![]() ${\lVert h_x\rVert ^2_{\overset {.}{H^s}(\mathbb R^N)}}/{D(x)}=\infty $
if
${\lVert h_x\rVert ^2_{\overset {.}{H^s}(\mathbb R^N)}}/{D(x)}=\infty $
if
![]() $D(x)=0$
. Hence,
$D(x)=0$
. Hence,
![]() $x\mapsto \lambda _s(A\cap B_x)$
is upper-semicontinuous.
$x\mapsto \lambda _s(A\cap B_x)$
is upper-semicontinuous.
Acknowledgement
This work was initiated and done when the second author visited Vietnam Institute for Advanced Study in Mathematics (VIASM) in 2022. He would like to thank the institute for hospitality and support during the visit.