No CrossRef data available.
Article contents
SOME COUNTING FORMULAE FOR 
$\lambda $-QUIDDITIES OVER THE RINGS 
${\mathbb {Z}}/2^{m}{\mathbb {Z}}$
Part of:
Combinatorics
Published online by Cambridge University Press: 16 May 2024
Abstract
The 
$\lambda $-quiddities of size n are n-tuples of elements of a fixed set, solutions of a matrix equation appearing in the study of Coxeter’s friezes. Their number and properties are closely linked to the structure and the cardinality of the chosen set. Our main objective is an explicit formula giving the number of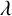 
$\lambda $-quiddities of odd size, and a lower and upper bound for the number of 
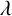$\lambda $-quiddities of even size, over the rings 
${\mathbb {Z}}/2^{m}{\mathbb {Z}}$ (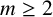
$m \geq 2$). We also give explicit formulae for the number of 
$\lambda $-quiddities of size n over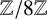 
${\mathbb {Z}}/8{\mathbb {Z}}$.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.
References
Conley, C. and Ovsienko, V., ‘Quiddities of polygon dissections and the Conway–Coxeter frieze equation’, Ann. Sc. Norm. Super. Pisa Cl. Sci. (5) 24(4) (2023), 2125–2170.Google Scholar
Cuntz, M. and Holm, T., ‘Frieze patterns over integers and other subsets of the complex numbers’, J. Comb. Algebra 3(2) (2019), 153–188.CrossRefGoogle Scholar
Cuntz, M. and Mabilat, F., ‘Comptage des quiddités sur les corps finis et sur quelques anneaux
$\mathbb{Z}/N\mathbb{Z}$
’, Ann. Fac. Sci. Toulouse Math. (6), to appear, arXiv:2304.03071.Google Scholar
$\mathbb{Z}/N\mathbb{Z}$
’, Ann. Fac. Sci. Toulouse Math. (6), to appear, arXiv:2304.03071.Google ScholarMabilat, F., ‘Combinatoire des sous-groupes de congruence du groupe modulaire’, Ann. Math. Blaise Pascal 28(1) (2021), 7–43.CrossRefGoogle Scholar
Mabilat, F., ‘
$\lambda$
-quiddité sur
$\mathbb{Z}[\alpha ]$
avec
$\alpha$
transcendant’, Math. Scand. 128(1) (2022), 5–13.CrossRefGoogle Scholar
$\lambda$
-quiddité sur
$\mathbb{Z}[\alpha ]$
avec
$\alpha$
transcendant’, Math. Scand. 128(1) (2022), 5–13.CrossRefGoogle ScholarMabilat, F., ‘Solutions monomiales minimales irréductibles dans
${SL}_2(\mathbb{Z}/{p}^n\mathbb{Z})$
’, Bull. Sci. Math., to appear, arXiv:2202.07279.Google Scholar
${SL}_2(\mathbb{Z}/{p}^n\mathbb{Z})$
’, Bull. Sci. Math., to appear, arXiv:2202.07279.Google ScholarMorier-Genoud, S., ‘Coxeter’s frieze patterns at the crossroad of algebra, geometry and combinatorics’, Bull. Lond. Math. Soc. 47(6) (2015), 895–938.CrossRefGoogle Scholar
Morier-Genoud, S., ‘Counting Coxeter’s friezes over a finite field via moduli spaces’, Algebr. Comb. 4(2) (2021), 225–240.Google Scholar
Ovsienko, V., ‘Partitions of unity in
$\mathrm{SL}(2,\mathbb{Z})$
, negative continued fractions, and dissections of polygons’, Res. Math. Sci. 5(2) (2018), Article no. 21.CrossRefGoogle Scholar
$\mathrm{SL}(2,\mathbb{Z})$
, negative continued fractions, and dissections of polygons’, Res. Math. Sci. 5(2) (2018), Article no. 21.CrossRefGoogle ScholarShort, I., Van Son, M. and Zabolotskii, A., ‘Frieze patterns and Farey complexes’, Preprint, 2023, arXiv:2312.12953.Google Scholar