Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ehrig, Michael
and
Stroppel, Catharina
2016.
Diagrammatic description for the categories of perverse sheaves on isotropic Grassmannians.
Selecta Mathematica,
Vol. 22,
Issue. 3,
p.
1455.
Wilbert, Arik
2017.
Topology of two-row Springer fibers for the even orthogonal and symplectic group.
Transactions of the American Mathematical Society,
Vol. 370,
Issue. 4,
p.
2707.
Ehrig, Michael
and
Stroppel, Catharina
2018.
Nazarov–Wenzl algebras, coideal subalgebras and categorified skew Howe duality.
Advances in Mathematics,
Vol. 331,
Issue. ,
p.
58.
Stroppel, Catharina
and
Wilbert, Arik
2019.
Two-block Springer fibers of types C and D: a diagrammatic approach to Springer theory.
Mathematische Zeitschrift,
Vol. 292,
Issue. 3-4,
p.
1387.
Li, Yiqiang
2019.
Quiver varieties and symmetric pairs.
Representation Theory of the American Mathematical Society,
Vol. 23,
Issue. 1,
p.
1.
KIM, D.
2019.
EULER CHARACTERISTIC OF SPRINGER FIBERS.
Transformation Groups,
Vol. 24,
Issue. 2,
p.
403.
Russell, Heather M
and
Tymoczko, Julianna S
2019.
The Transition Matrix between the Specht and Web Bases Is Unipotent with Additional Vanishing Entries.
International Mathematics Research Notices,
Vol. 2019,
Issue. 5,
p.
1479.
Tubbenhauer, Daniel
2020.
𝔤𝔩n-webs, categorification and Khovanov–Rozansky homologies.
Journal of Knot Theory and Its Ramifications,
Vol. 29,
Issue. 11,
p.
2050074.
Eberhardt, Jens Niklas
Naisse, Grégoire
and
Wilbert, Arik
2021.
Real Springer fibers and odd arc algebras.
Journal of the London Mathematical Society,
Vol. 103,
Issue. 4,
p.
1415.
EHRIG, M.
and
TUBBENHAUER, D.
2021.
RELATIVE CELLULAR ALGEBRAS.
Transformation Groups,
Vol. 26,
Issue. 1,
p.
229.
Im, Mee Seong
Lai, Chun-Ju
and
Wilbert, Arik
2022.
Irreducible components of two-row Springer fibers for all classical types.
Proceedings of the American Mathematical Society,
Li, Yanbo
and
Shi, Xiaolin
2022.
Semi-simplicity of Temperley-Lieb Algebras of type D.
Algebras and Representation Theory,
Vol. 25,
Issue. 5,
p.
1133.
SAUNDERS, N.
and
WILBERT, A.
2022.
EXOTIC SPRINGER FIBERS FOR ORBITS CORRESPONDING TO ONE-ROW BIPARTITIONS.
Transformation Groups,
Vol. 27,
Issue. 3,
p.
1111.
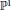 ${{\mathbb{P}}^{1}}$-bundles. Using results of Kumar and Procesi we compute the cohomology ring with its action of the Weyl group. The main tool is a type
${{\mathbb{P}}^{1}}$-bundles. Using results of Kumar and Procesi we compute the cohomology ring with its action of the Weyl group. The main tool is a type  $\text{D}$ diagram calculus labelling the irreducible components in a convenient way that relates to a diagrammatical algebra describing the category of perverse sheaves on isotropic Grassmannians based on work of Braden. The diagram calculus generalizes Khovanov's arc algebra to the type
$\text{D}$ diagram calculus labelling the irreducible components in a convenient way that relates to a diagrammatical algebra describing the category of perverse sheaves on isotropic Grassmannians based on work of Braden. The diagram calculus generalizes Khovanov's arc algebra to the type  $\text{D}$ setting and should be seen as setting the framework for generalizing well-known connections of these algebras in type
$\text{D}$ setting and should be seen as setting the framework for generalizing well-known connections of these algebras in type  $\text{A}$ to other types.
$\text{A}$ to other types.



