Hostname: page-component-5b777bbd6c-w9n4q Total loading time: 0 Render date: 2025-06-24T11:30:47.499Z Has data issue: false hasContentIssue false
  • English
  • Français

The Asymptotic Behaviour of Equidistant Permutation Arrays

Published online by Cambridge University Press:  20 November 2018

S. A. Vanstone
S. A. Vanstone*
Affiliation:
St. Jerome's College, University of Waterloo, Waterloo, Ontario
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

An equidistant permutation array (EPA) A(r λ v) is a v × r array defined on a set V of r symbols such that every row is a permutation of V and any two distinct rows have precisely λ common column entries. Define R(r, λ) to be the largest value of v for which there exists an A (r, λ; v). Deza [2] has shown that

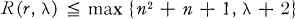
where n = r – λ. Bolton [1] has shown that

(*)

In this paper, we show that equality holds in (*) for λ > ┌n/3┐(n2 + n). In order to do this we require several more definitions.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 31 , Issue 1 , 01 February 1979 , pp. 45 - 48
Copyright
Copyright © Canadian Mathematical Society 1979

References

1. Bolton, D. W., unpublished manuscript.Google Scholar
2. Deza, M., Matrices dont deux lignes quelconques coincident dans un nombre donne de positions communes, Journal of Combinatorial Theory, Series A. 20 (1976), 306–318.Google Scholar
3. Deza, M., Mullin, R. C. and Vanstone, S. A., Room squares and equidistant permutation arrays, Ars Combinatori. 2 (1976), 235–244.Google Scholar
4. Heinrich, K., van Rees, J., and Wallis, W. D., A general construction jor equidistant permutation arrays, (preprint).Google Scholar
5. Mullin, R. C., An asymptotic property of (r, λ)-systems, Utilitas Math. 3 (1973), 139–152.Google Scholar
6. Schellenberg, P. J., and Vanstone, S. A., Some results on equidistant permutation arrays, Proc. 6th Manitoba Conference on Numerical Math (1976), 389–410.Google Scholar
7. Vanstone, S. A., Pairwise orthogonal generalized Room squares and equidistant permutation arrays, Journal of Combinatorial Theory, Series A. 25 (1978), 84–89.Google Scholar