Hostname: page-component-848d4c4894-ttngx Total loading time: 0 Render date: 2024-06-08T23:08:47.757Z Has data issue: false hasContentIssue false
  • English
  • Français

Constructions of Chiral Polytopes of Small Rank

Published online by Cambridge University Press:  20 November 2018

Antonio Breda D’Azevedo ,
Gareth A. Jones  and
Egon Schulte
Antonio Breda D’Azevedo
Affiliation:
Departamento de Matemática, Universidade de Aveiro, P 3800 Aveiro, Portugal email: breda@ua.pt
Gareth A. Jones
Affiliation:
School of Mathematics, University of Southampton, Southampton SO17 1BJ, United Kingdom email: g.a.jones@soton.ac.uk
Egon Schulte
Affiliation:
Department of Mathematics, Northeastern University, Boston, Massachusetts 02115, USA email: schulte@neu.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

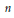An abstract polytope of rank $n$ is said to be chiral if its automorphism group has precisely two orbits on the flags, such that adjacent flags belong to distinct orbits. This paper describes a general method for deriving new finite chiral polytopes from old finite chiral polytopes of the same rank. In particular, the technique is used to construct many new examples in ranks 3, 4, and 5.

Keywords

51M2052B1505C25abstract regular polytopechiral polytopechiral maps
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 63 , Issue 6 , 01 December 2011 , pp. 1254 - 1283
Copyright
Copyright © Canadian Mathematical Society 2011

References

[1] Artin, E., Geometric algebra. Interscience Publishers, Inc., New York-London, 1957.Google Scholar
[2] Breda, A., Breda D’Azevedo, A., and Nedela, R., Chirality group and chirality index of the Coxeter chiral maps. Ars Combin. 81(2006), 147–160.Google Scholar
[3] Breda D’Azevedo, A. J. and Jones, G. A., Double coverings and reflexible abelian hypermaps. Beiträge Algebra Geom. 41(2000), no. 2, 371–389.Google Scholar
[4] Breda D’Azevedo, A. J. and Nedela, R., Join and intersection of hypermaps. Acta Univ. M. Belli Ser. Math. 9(2001), 13–28.Google Scholar
[5] Breda D’Azevedo, A. J., Nedela, R., and Širáň, J., Classification of regular maps of negative prime Euler characteristic. Trans. Amer. Math. Soc. 357(2005), no. 10, 4175–4190. doi:10.1090/S0002-9947-04-03622-0Google Scholar
[6] Breda D’Azevedo, A. J., Jones, G., Nedela, R., and M. Škoviera, Chirality groups of maps and hypermaps. J. Algebraic Combin. 29(2009), no. 3, 337–355. doi:10.1007/s10801-008-0138-zGoogle Scholar
[7] Conder, M., Hurwitz groups: a brief survey. Bull. Amer. Math. Soc. (N.S.) 23(1990), no. 2, 359–370. doi:10.1090/S0273-0979-1990-15933-6Google Scholar
[8] Conder, M. D. E., Regular maps and hypermaps of Euler characteristic −1 to −200. J. Combin. Theory Ser. B 99(2009), no. 2, 455–459. (with associated lists of computational data available at http://www.math.auckland.ac.nz/_conder/hypermaps.html.) doi:10.1016/j.jctb.2008.09.003 Google Scholar
[9] Conder, M. D. E., Širáň, J., and Tucker, T.W., The genera, reflexibility and simplicity of regular maps. J. Eur. Math. Soc. 12(2010), no. 2, 343–364. doi:10.4171/JEMS/200Google Scholar
[10] Conder, M., Hubard, I., and Pisanski, T., Constructions for chiral polytopes. J. London Math. Soc. (2) 77(2008), no. 1, 115–129. doi:10.1112/jlms/jdm093Google Scholar
[11] Conway, J. H., Curtis, R. T., Norton, S .P., Parker, R. A., and Wilson, R. A., Atlas of finite groups. Maximal subgroups and ordinary characters for simple groups. Oxford University Press, Eynsham, 1985.Google Scholar
[12] Coxeter, H. S. M., Twisted honeycombs. Conference Board of the Mathematical Sciences Regional Conference Series in Mathematics, 4, American Mathematical Society, Providence, RI, 1970.Google Scholar
[13] Coxeter, H. S. M., Regular polytopes. Third ed., Dover Publications, New York, 1973.Google Scholar
[14] Coxeter, H. S. M., Ten toroids and fifty-seven hemidodecahedra. Geom. Dedicata 13(1982), no. 1, 87–99.Google Scholar
[15] Coxeter, H. S. M., A symmetrical arrangement of eleven hemi-icosahedra. In: Convexity and graph theory (Jerusalem 1981), North-HollandMath. Stud., 87, North-Holland, Amsterdam, 1984, pp. 103–114.Google Scholar
[16] Coxeter, H. S. M. and Moser, W. O. J., Generators and relations for discrete groups. Fourth ed., Ergebnisse der Mathematik und ihrer Grenzgebiete, 14, Springer-Verlag, Berlin-New York, 1980.Google Scholar
[17] Coxeter, H. S. M. and Weiss, A. I., Twisted honeycombs ﹛3, 5, 3﹜t and their groups. Geom. Dedicata 17(1984), no. 2, 169–179.Google Scholar
[18] Dickson, L. E., Linear groups: With an exposition of the Galois field theory. Dover Publications Inc., New York, 1958.Google Scholar
[19] Doro, S. and Wilson, S. E., Rotary maps of type ﹛6, 6﹜4. Quarterly J. Math. Oxford Ser. (2) 31(1980), no. 124, 403–414. doi:10.1093/qmath/31.4.403Google Scholar
[20] The GAP Group, GAP—Groups, Algorithms, and Programming. Version 4.4.9; 2006, http://www.gap-system.org. Google Scholar
[21] Grünbaum, B., Regularity of graphs, complexes and designs. In: Problèmes combinatoires et théorie des graphes (Colloq. Internal. CNRS, Univ. Orsay, Orsay, 1976), Colloq. Internat. CNRS, 260, CNRS, Paris, 1977, pp. 191–197.Google Scholar
[22] Hartley, M. I., All polytopes are quotients, and isomorphic polytopes are quotients by conjugate subgroups. Discrete Comput. Geom. 21(1999), no. 2, 289–298. doi:10.1007/PL00009422Google Scholar
[23] Hartley, M. I. and Leemans, D., On locally spherical polytopes of type ﹛5, 3, 5﹜. Discrete Math. 309(2009), no. 1, 247–254. doi:10.1016/j.disc.2007.12.084Google Scholar
[24] Hartley, M. I., McMullen, P., and Schulte, E., Symmetric tessellations on Euclidean space-forms. Canad. J. Math. 51(1999), no. 6, 1230–1239. doi:10.4153/CJM-1999-055-6Google Scholar
[25] Heffter, L., Über metazyklische Gruppen und Nachbarkonfigurationen. Math. Ann. 50(1898), no. 2–3, 261–268. doi:10.1007/BF01448067Google Scholar
[26] Hubard, I., A. Orbanıc, and Weiss, A. I., Monodromy groups and self-invariance. Canad. J. Math. 61(2009), no. 6, 1300–1324. doi:10.4153/CJM-2009-061-5Google Scholar
[27] Hubard, I. and Weiss, A. I., Self-duality of chiral polytopes. J. Combin. Theory Ser. A 111(2005), no. 1, 128–136. doi:10.1016/j.jcta.2004.11.012Google Scholar
[28] Huppert, B., Endliche Gruppen. I. Die Grundlehren der Mathematischen Wissenschaften, 134, Springer-Verlag, Berlin-New York, 1967.Google Scholar
[29] Huppert, B. and Blackburn, N., Finite groups. III. Grundlehren der Mathematischen Wissenschaften, 243, Springer-Verlag, Berlin-New York, 1982.Google Scholar
[30] Jones, G. A. and Long, C. D., Epimorphic images of the [5, 3, 5] Coxeter group. Math. Z., to appear.Google Scholar
[31] Jones, G. A. and Mednykh, A., Three-dimensional hyperbolic manifolds with a large isometry group. Math. Proc. Cambridge Phil. Soc., to appear.Google Scholar
[32] Jones, G. A. and Silver, S. A., Suzuki groups and surfaces. J. London Math. Soc. (2) 48(1993), no. 1, 117–125. doi:10.1112/jlms/s2-48.1.117Google Scholar
[33] Jones, G. A. and Singerman, D., Complex functions. An algebraic and geometric viewpoint. Cambridge University Press, Cambridge, 1987.Google Scholar
[34] Jones, G. A. and Singerman, D., Maps, hypermaps and triangle groups. In: The Grothendieck theory of dessins d’enfants (Luminy, 1993), London Math. Soc. Lecture Note Ser., 200, Cambridge University Press, Cambridge, 1994, pp. 115–145.Google Scholar
[35] Jones, G. A., Long, C. D., and Mednykh, A., Hyperbolic manifolds and tessellations of type ﹛3, 5, 3﹜ associated with L2(q). arxiv:1106.0867Google Scholar
[36] Leemans, D. and Schulte, E., Groups of type L2(q) acting on polytopes. Adv. Geom. 7(2007), no. 4, 529–539. doi:10.1515/ADVGEOM.2007.031Google Scholar
[37] Long, C. D., Epimorphic images of simplicial Coxeter groups and some associated hyperbolic manifolds, Ph.D. Thesis, University of Southampton, 2007.Google Scholar
[38] Macbeath, A. M., Generators of the linear fractional groups. In: Number theory (Proc. Sympos. Pure Math., Vol. XII, Houston, Texas, 1967), American Mathematical Society, Providence, RI, 1969, pp. 14–32.Google Scholar
[39] McMullen, P., The groups of the regular star-polytopes. Canad. J. Math. 50(1998), no. 2, 426–448. doi:10.4153/CJM-1998-023-7Google Scholar
[40] McMullen, P. and Schulte, E., Abstract regular polytopes. Encyclopedia of Mathematics and its Applications, 92, Cambridge University Press, Cambridge, 2002.Google Scholar
[41] McMullen, P. and Schulte, E., Hermitian forms and locally toroidal regular polytopes. Adv. Math. 82(1990), no. 1, 88–125. doi:10.1016/0001-8708(90)90084-ZGoogle Scholar
[42] McMullen, P. and Schulte, E., Regular polytopes of type ﹛4, 4, 3﹜ and ﹛4, 4, 4﹜. Combinatorica 12(1992), no. 2, 203–220. doi:10.1007/BF01204723Google Scholar
[43] Monson, B. and Schulte, E., Reflection groups and polytopes over finite fields. I. Adv. in Appl. Math. 33(2004), no. 2, 290–317. doi:10.1016/j.aam.2003.11.002Google Scholar
[44] Monson, B. and Schulte, E., Reflection groups and polytopes over finite fields. II. Adv. in Appl. Math. 38(2007), no. 3, 327–356. doi:10.1016/j.aam.2005.12.001Google Scholar
[45] Monson, B. and Schulte, E., Modular reduction in abstract polytopes. Canad. Math. Bull. 52(2009), no. 3, 435–450. doi:10.4153/CMB-2009-047-7Google Scholar
[46] Monson, B. and Weiss, A. I., Regular 4-polytopes related to general orthogonal groups. Mathematika 37(1990), no. 1, 106–118. doi:10.1112/S0025579300012845Google Scholar
[47] Monson, B. and Weiss, A. I., Eisenstein integers and related C-groups. Geom. Dedicata 66(1997), no. 1, 99–117. doi:10.1023/A:1004953220074Google Scholar
[48] Nostrand, B. and Schulte, E., Chiral polytopes from hyperbolic honeycombs. Discrete Comput. Geom. 13(1995), no. 2, 17–39. doi:10.1007/BF02574026Google Scholar
[49] Orbanic, A., F-actions and parallel-product decomposition of reflexible maps. J. Algebraic Combin. 26(2007), no. 4, 507–527. doi:10.1007/s10801-007-0069-0Google Scholar
[50] Pellicer, D., A construction of higher rank chiral polytopes. Discrete Math. 310(2010), no. 6–7. 1222–1237. doi:10.1016/j.disc.2009.11.034Google Scholar
[51] Pellicer, D. and Weiss, A. I., Generalized CPR-graphs and applications. Contrib. Discrete Math. 5(2010), no. 2, 76–105.Google Scholar
[52] Ree, R., A family of simple groups associated with the simple Lie algebra of type (G2). Bull. Amer. Math. Soc. 66(1960), 508–510. doi:10.1090/S0002-9904-1960-10523-XGoogle Scholar
[53] Ree, R., A family of simple groups associated with the simple Lie algebra of type (G2). Amer. J. Math. 83(1961), 432–462. doi:10.2307/2372888Google Scholar
[54] Schulte, E. and Weiss, A. I., Chiral polytopes. In: Applied geometry and discrete mathematics, DIMACS Ser. Discrete Math. Theoret. Comput. Sci., 4, American Mathematical Society, Providence, RI, 1991, pp. 493–516.Google Scholar
[55] Schulte, E. and Weiss, A. I., Chirality and projective linear groups. Discrete Math. 131(1994), no. 1–3, 221–261. doi:10.1016/0012-365X(94)90387-5Google Scholar
[56] Schulte, E. and Weiss, A. I., Free extensions of chiral polytopes. Canad. J. Math. 47(1995), no. 3, 641–654. doi:10.4153/CJM-1995-033-7Google Scholar
[57] Suzuki, M., On a class of doubly transitive groups. Ann. of Math. 75(1962), 105–145. doi:10.2307/1970423Google Scholar
[58] Weiss, A. I., Twisted honeycombs ﹛3, 5, 3﹜t . C. R. Math. Rep. Acad. Sci. Canada 5(1983), no. 5, 211–215.Google Scholar
[59] Wilson, S., The smallest nontoroidal chiral maps. J. Graph Theory 2(1978), no. 4, 315–318. doi:10.1002/jgt.3190020405Google Scholar
[60] Wilson, S., Parallel products in groups and maps. J. Algebra 167(1994), no. 3, 539–546. doi:10.1006/jabr.1994.1200Google Scholar