Hostname: page-component-848d4c4894-m9kch Total loading time: 0 Render date: 2024-06-09T03:02:11.325Z Has data issue: false hasContentIssue false
  • English
  • Français

Idempotent Multipliers of H1(T)

Published online by Cambridge University Press:  20 November 2018

I. Klemes
I. Klemes*
Affiliation:
McGill University, Montréal, Québec
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let as usual T = R/2πZ be the circle, and H1 the subspace of L1(T) of all f such that 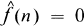for all integers n < 0. The norm

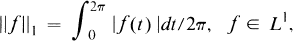
restricted to H1, makes it a Banach space. By a multiplier of H1 we mean a bounded linear operator m:H1 → H1 such that there is a sequence 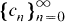in C with

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 39 , Issue 5 , 01 October 1987 , pp. 1223 - 1234
Copyright
Copyright © Canadian Mathematical Society 1987

References

1. Fournier, J. J. F., On a theorem of Paley and the Littlewood conjecture, Arkiv for Mathematik 17(1979), 199–216.Google Scholar
2. Graham, C. C. and McGehee, O. C., Essays in commutative harmonic analysis, (Springer Verlag, New York, 1979).CrossRefGoogle Scholar
3. Helson, H., Note on harmonic functions, Proc. Amer. Math. Soc. 4 (1953), 686–691.Google Scholar
4. Helson, H., On a theorem of Szegö, Proc. Amer. Math. Soc. 6 (1955), 235–242.Google Scholar
5. Klemes, I., The idempotent multipliers of H1(T), Abstracts of Amer. Math. Soc. 5 (1984), 379.Google Scholar
6. Klemes, I. I. Idempotent multipliers of H1 on the circle, II. A mean oscillation inequality for rearrangements, Ph.D. thesis, California Institute of Technology (1985).Google Scholar
7. McGehee, O. C., Pigno, L. and Smith, B., Hardy's inequality and the L1 norm of exponential sums, Ann. of Math. 113 (1981), 613–618.Google Scholar
8. Paley, R. E. A. C., On the lacunary coefficients of power series, Ann. of Math. 34 (1933), 615–616.Google Scholar