Let $h_{\vee }^{\infty }\,\left( \text{B} \right)$ and 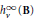$h_{\vee }^{\infty }\,\left( \text{B} \right)$ be the spaces of harmonic functions in the unit disk and multidimensional unit ball admitting a two-sided radial majorant 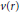$v\left( r \right)$. We consider functions $v$ that fulfill a doubling condition. In the two-dimensional case let

$$u\left( r{{e}^{i\theta }},\xi \right)\,=\,\sum\limits_{j=0}^{\infty }{\left( {{a}_{j0}}{{\xi }_{j0}}{{r}^{j}}\,\cos \,j\theta \,+\,{{a}_{j1}}{{\xi }_{j1}}{{r}^{j}}\,\sin \,j\theta \right)}$$

where 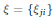$\xi \,=\,\left\{ {{\xi }_{ji}} \right\}$ is a sequence of random subnormal variables and 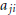${{a}_{ji}}$ are real. In higher dimensions we consider series of spherical harmonics. We will obtain conditions on the coefficients 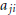${{a}_{ji}}$ that imply that $u$ is in 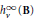$h_{\vee }^{\infty }\,\left( \text{B} \right)$ almost surely. Our estimate improves previous results by Bennett, Stegenga, and Timoney, and we prove that the estimate is sharp. The results for growth spaces can easily be applied to Bloch-type spaces, and we obtain a similar characterization for these spaces that generalizes results by Anderson, Clunie, and Pommerenke and by Guo and Liu.