Hostname: page-component-77c89778f8-m8s7h Total loading time: 0 Render date: 2024-07-22T13:32:58.728Z Has data issue: false hasContentIssue false
  • English
  • Français

Rings Satisfying (x, y, z) = (y, z, x)

Published online by Cambridge University Press:  20 November 2018

Nicholas J. Sterling
Nicholas J. Sterling*
Affiliation:
Harpur College, Binghamton, New York
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let R be a ring satisfying the identity

1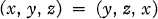

for all x, y, z ϵ R, where (x,y,z) = (xy)z — x(yz). If R also satisfies the identity (x, x, x) = 0 for all x ϵ R, then R is alternative. It is known that if R satisfies (1), it need not be an alternative (see 6). Thus, the class of rings satisfying (1) is a non-trivial extension of the class of alternative rings. P. Jordan remarked that (x, x, x)2 = 0 is an identity in R (see 9).

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 20 , 1968 , pp. 913 - 918
Copyright
Copyright © Canadian Mathematical Society 1968

References

1. Bruck, R. H. and Kleinfeld, E., The structure of alternative division rings, Proc. Amer. Math. Soc. 2 (1951), 878–890.Google Scholar
2. Kleinfeld, E., Extension of a theorem on alternative division rings, Proc. Amer. Math. Soc. 3 1952), 348–35.Google Scholar
3. Kleinfeld, E., Simple alternative rings, Ann. of Math. (2), 58 (1953), 544–547.Google Scholar
4. Kleinfeld, E., On simple alternative rings, Amer. J. Math. 75 (1953), 98–104.Google Scholar
5. Kleinfeld, E., Alternative nil rings, Ann. of Math. (2) 66 (1957), 395–399.Google Scholar
6. Kleinfeld, E., Assosymmetric rings, Proc. Amer. Math. Soc. 8 (1957), 983–986.Google Scholar
7. Outcalt, D. L., An extension of the class of alternative rings, Can. J. Math. 17 (1965), 130–141.Google Scholar
8. San Soucie, R. L. Weakly standard rings, Amer. J. Math. 79 (1957), 80–86.Google Scholar
9. Zorn, M., Alter nativkor per und quadratische Système, Abh. Math. Sem. Hamburg Univ. 9 1933), 395–402.Google Scholar