Hostname: page-component-848d4c4894-cjp7w Total loading time: 0 Render date: 2024-06-26T18:02:17.591Z Has data issue: false hasContentIssue false
  • English
  • Français

Noncommutative Symmetric Bessel Functions

Published online by Cambridge University Press:  20 November 2018

Jean-Christophe Novelli  and
Jean-Yves Thibon
Jean-Christophe Novelli
Affiliation:
Institut Gaspard Monge, Université de Marne-la-Vallée, Champs-sur-Marne, 77454 Marne-la-Vallée Cedex 2, France. e-mail: novelli@univ-mlv.fr, e-mail: jyt@univ-mlv.fr
Jean-Yves Thibon
Affiliation:
Institut Gaspard Monge, Université de Marne-la-Vallée, Champs-sur-Marne, 77454 Marne-la-Vallée Cedex 2, France. e-mail: novelli@univ-mlv.fr, e-mail: jyt@univ-mlv.fr
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The consideration of tensor products of 0-Hecke algebramodules leads to natural analogs of the Bessel 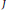$J$-functions in the algebra of noncommutative symmetric functions. This provides a simple explanation of various combinatorial properties of Bessel functions.

Keywords

05E0516W3005A15
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 51 , Issue 3 , 01 September 2008 , pp. 424 - 438
Copyright
Copyright © Canadian Mathematical Society 2008

References

[1] Bousquet-Mélou, M. and Viennot, X. G., Empilements de segments et q-énumération de polyominos convexes dirigés. J. Combin. Theory Ser. A 60(1992), no. 2, 196–224.Google Scholar
[2] Carlitz, L., The coefficients of the reciprocal of J 0 (z). Arch. Math. 6(1955), 121–127.Google Scholar
[3] Carlitz, L., Scoville, R., and Vaughan, T., Enumeration of pairs of permutations. Discrete Math. 14(1976), no. 3, 215–239.Google Scholar
[4] Carlitz, L., Scoville, R., and Vaughan, T., Enumeration of pairs of sequences by rises, falls and levels. Manuscripta Math. 19(1976), no. 3, 211–243.Google Scholar
[5] Cartier, P. and Foata, D., Problèmes combinatoires de commutation et réarrangements. Lecture Notes in Mathematics 85, Springer-Verlag, Berlin, 1969.Google Scholar
[6] Delest, M. P. and Fédou, J.-M., Enumeration of skew Ferrers’ diagrams. Discrete Math. 112(1993), no. 1–3, 65–69.Google Scholar
[7] Désarménien, J., Fonctions symétriques associées à des suites classiques de nombres. Ann. Sci. École Norm. Sup. 16(1983), no. 2, 271–304.Google Scholar
[8] Duchamp, G., Hivert, F., and Thibon, J.-Y., Noncommutative symmetric functions. VI. Free quasi-symmetric functions and related algebras. Internat. J. Alg. Comput. 12(2002), no. 5, 671–717.Google Scholar
[9] Fédou, J.-M. and Rawlings, D. P., Statistics on pairs of permutations. Discrete Math. 143(1995), no. 1,3, 31–45.Google Scholar
[10] Fédou, J.-M. and Rawlings, D. P., Adjacencies in words. Adv. in Appl. Math. 16(1995), no. 2, 206–218.Google Scholar
[11] Fédou, J.-M. and Rawlings, D. P., More statistics on permutation pairs. Electronic J. Comb. 1(1994), Research Paper 11.Google Scholar
[12] Gelfand, I. M., Krob, D., Lascoux, A., Leclerc, B., Retakh, V. S., and Thibon, J.-Y., Noncommutative symmetric functions. Adv. in Math. 112(1995), no. 2, 218–348.Google Scholar
[13] Hivert, F. and Thiéry, N. M., Representation theories of some towers of algebras related to the symmetric groups and their Hecke algebras. Proc. FPSAC’06, San-Diego (eletronic), http://fpsac-sfca.org/FPSAC06/SITE06/papers/75.pdf.Google Scholar
[14] Krob, D., Leclerc, B., and Thibon, J.-Y., Noncommutative symmetric functions. II. Transformations of alphabets. Internat. J. Algebra Comput. 7(1997), no. 2, 181–264.Google Scholar
[15] Krob, D. and Thibon, J.-Y., Noncommutative symmetric functions. IV. Quantum linear groups and Hecke algebras at q = 0. J. Algebraic Combin. 6(1997), no. 4, 339–376.Google Scholar
[16] Lascoux, A., Symmetric functions and combinatorial operators on polynomials. CBMS Regional Conference Series in Mathematics 99, American Mathematical Socoety, Providence, RI, 2003 Google Scholar
[17] Macdonald, I. G., Symmetric functions and Hall polynomials, Second edition, Oxford University Press, New York, 1995.Google Scholar
[18] Novelli, J.-C and Thibon, J.-Y., Noncommutative symmetric functions and Lagrange inversion. Adv. in Appl. Math. 40(2008), no. 1, 8–35.Google Scholar
[19] Priddy, S. B., Koszul resolutions. Trans. Amer. Math. Soc. 152(1970), 39–60.Google Scholar
[20] Viennot, X. G., Heaps of pieces I: Basic definitions and combinatorial lemmas. In: Combinatoire Énumérative. Lecture Notes in Mathematica 1234, Springer, Berlin, 1986, pp. 321–350.Google Scholar