Hostname: page-component-77c89778f8-9q27g Total loading time: 0 Render date: 2024-07-20T07:57:27.319Z Has data issue: false hasContentIssue false
  • English
  • Français

Random Matrices and Brownian Motion†

Published online by Cambridge University Press:  12 September 2008

William M. Y. Goh  and
Eric Schmutz
William M. Y. Goh
Affiliation:
Department of Mathematics, Drexel University, Philadelphia, Pa. 19104
Eric Schmutz
Affiliation:
Department of Mathematics, Drexel University, Philadelphia, Pa. 19104
Get access Rights & Permissions [Opens in a new window]

Abstract

For T ∈ GLn (Fq), let Ωn (t, T) be the number of irreducible factors of degree less than or equal to nt in the characteristic polynomial of T. Let

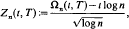
and suppose T is chosen from G Ln(Fq) at random uniformly. We prove that the stochastic process ≺Zn(t)≻t∈[0, 1] converges to the standard Brownian motion process W(t), as n → ∞.

Type
Research Article
Information
Combinatorics, Probability and Computing , Volume 2 , Issue 2 , June 1993 , pp. 157 - 180
Copyright
Copyright © Cambridge University Press 1993

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1]Billingsley, P. (1968) Convergence of probability Measures, John Wiley and Sons.Google Scholar
[2]De Laurentis, J. and Pittel, B. (1985) Random permutations and Brownian motion. Pacific Journal of Mathematics 119 287–301.Google Scholar
[3]Gihman, I. I. and Skorohod, A. V. (1974) The theory of stochastic processes, Springer-Verlag.Google Scholar
[4]Goh, W. and Schmutz, E. (1991) A central Limit theorem on G Ln(Fq). Random Structures and Algorithms 2 47–53.CrossRefGoogle Scholar
[5]Graham, R. and Diaconis, P. (1992) Affine walk on the hypercube. Journal of Computational and Applied Mathematics 41 215–235.Google Scholar
[6]Hansen, J. C. (preprint) A functional central limit theorem for random mappings.Google Scholar
[7]Hansen, J. C. (to appear) Combinatorics Probability and Computing.Google Scholar
[8]Kung, J. (1981) The cycle structure of a linear transformation over a finite field. Linear Algebra Appl. 36 141–155.CrossRefGoogle Scholar
[9]Lidl, R. and Niederreiter, H. (1983) Finite fields. Encyclopedia of Mathematics and its Applications 20, Addison-Wesley Pub.Google Scholar
[10]MacWilliams, F. and Sloane, N. (1981) The theory of error correcting codes, North-Holland, 115.Google Scholar
[11]Stong, R. (1988) Some asymptotic results on finite vector spaces. Advances in Applied Mathematics 9 167–199.CrossRefGoogle Scholar
[12]Whitaker, E. T. and Watson, G. N. (1961) A course of modern analysis, Cambridge University Press, p. 242.Google Scholar
[13]Zygmund, (1959) Trigonometric series, Cambridge University Press.Google Scholar