Hostname: page-component-7bb8b95d7b-dtkg6 Total loading time: 0 Render date: 2024-09-17T18:07:17.863Z Has data issue: true hasContentIssue false
  • English
  • Français

On the integral part of A-motivic cohomology

Part of: (Co)homology theory Algebraic number theory: global fields Arithmetic algebraic geometry

Published online by Cambridge University Press:  16 September 2024

Q. Gazda Open the ORCID record for Q. Gazda [Opens in a new window]
Q. Gazda*
Affiliation:
CMLS, École Polytechnique, Palaiseau 91120, France quentin@gazda.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

The deepest arithmetic invariants attached to an algebraic variety defined over a number field 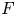$F$ are conjecturally captured by the integral part of its motivic cohomology. There are essentially two ways of defining it when 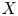$X$ is a smooth projective variety: one is via the 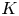$K$-theory of a regular integral model, the other is through its 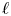$\ell$-adic realization. Both approaches are conjectured to coincide. This paper initiates the study of motivic cohomology for global fields of positive characteristic, hereafter named 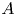$A$-motivic cohomology, where classical mixed motives are replaced by mixed Anderson 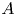$A$-motives. Our main objective is to set the definitions of the integral part and the good 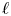$\ell$-adic part of the 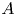$A$-motivic cohomology using Gardeyn's notion of maximal models as the analogue of regular integral models of varieties. Our main result states that the integral part is contained in the good 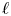$\ell$-adic part. As opposed to what is expected in the number field setting, we show that the two approaches do not match in general. We conclude this work by introducing the submodule of regulated extensions of mixed Anderson 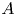$A$-motives, for which we expect the two approaches to match, and solve some particular cases of this expectation.

Keywords

Anderson t-motivemotivic cohomologyextensions groupmaximal model

MSC classification

Primary: 11R58: Arithmetic theory of algebraic function fields 14F42: Motivic cohomology; motivic homotopy theory 11G09: Drinfel'd modules; higher-dimensional motives, etc.
Type
Research Article
Information
Compositio Mathematica , Volume 160 , Issue 8 , August 2024 , pp. 1715 - 1783
Check for updates
Copyright
© 2024 The Author(s). The publishing rights in this article are licensed to Foundation Compositio Mathematica under an exclusive licence

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anderson, G. W., $t$-motives, Duke Math. J. 53 (1986), 457–502.Google Scholar
André, Y., Slope filtrations, Confluentes Mathematici 1 (2009), 1–85.Google Scholar
Beilinson, A. A., Higher regulators and values of $L$-functions, J. Sov. Math. 30 (1985), 2036–2070.Google Scholar
Beilinson, A. A., Notes on absolute Hodge cohomology, in Applications of algebraic K-theory to algebraic geometry and number theory: Part I, Contemporary Mathematics, vol. 55 (American Mathematical Society, Providence, RI, 1986), 35–68.Google Scholar
Böckle, G., Goss, D., Hartl, U. and Papanikolas, M. A. (eds), t-Motives: Hodge structures, transcendence and other motivic aspects, EMS Series of Congress Reports (European Mathematical Society, Zürich, 2020).Google Scholar
Bornhofen, M. and Hartl, U., Pure Anderson motives and abelian $\tau$-sheaves, Math. Z. 268 (2011), 247–283.Google Scholar
Bourbaki, N., Algèbre (A), Chapitres I à III; Algèbre commutative (AC), Chapitres I à IV, Éléments de Mathématique (Hermann, Paris, 1970).Google Scholar
Bühler, T., Exact categories, Expo. Math. 28 (2010), 1–69.Google Scholar
Brownawell, W. D. and Papanikolas, M. A., A rapid introduction to Drinfeld modules, t-modules and t-motives, in t-Motives: Hodge structures, transcendence, and other motivic aspects (European Mathematical Society, Zürich, 2020), 3–30.Google Scholar
Deligne, P., Le Groupe Fondamental de la Droite Projective Moins Trois Points, in Galois groups over $\mathbb {Q}$, Mathematical Sciences Research Institute Publications, vol. 16 (Springer, New York, 1989).Google Scholar
Eisenbud, D., Commutative algebra with a view toward algebraic geometry, Graduate Texts in Mathematics (Springer, 1995).Google Scholar
Gardeyn, F., A Galois criterion for good reduction of $\tau$-sheaves, J. Number Theory 97 (2002), 447–471.Google Scholar
Gardeyn, F., The structure of analytic $\tau$-sheaves, J. Number Theory 100 (2003), 332–362.Google Scholar
Gazda, Q., Regulators in the arithmetic of function fields, Preprint (2022), arXiv:2207.03461.Google Scholar
Gazda, Q. and Maurischat, A., Carlitz twists: their motivic cohomology, regulators, zeta values and polylogarithms, Preprint (2023), arXiv:2212.02972.Google Scholar
Hartl, U., A dictionary between Fontaine-theory and its analogue in equal characteristic, J. Number Theory 129 (2009), 1734–1757.Google Scholar
Hartl, U., Period spaces for Hodge structures in equal characteristic, Ann. of Math. (2) 173 (2011), 1241–1358.Google Scholar
Hartl, U., Isogenies of abelian Anderson $A$-modules and $A$-motives, Ann. Sc. Norm. Super. Pisa Cl. Sci. (5), XIX (2019), 1429–1470.Google Scholar
Hartl, U. and Juschka, A.-K., Pink's theory of Hodge structures and the Hodge conjecture over function fields, in t-motives: Hodge structures, transcendence and other motivic aspects, EMS Series of Congress Reports (European Mathematical Society, Zürich, 2020), 31–182.Google Scholar
Jannsen, U., Mixed motives and algebraic $K$-theory, Lecture Notes in Mathematics, vol. 1400 (Springer, 1990).Google Scholar
Katz, N., Une nouvelle formule de congruence pour la fonction $\zeta$, Éxposé XXII SGA7 t. II, Lecture Notes in Mathematics 340 (Springer, 1973).Google Scholar
Katz, N., $p$-adic properties of modular schemes and modular forms, Lecture Notes in Mathematics 350 (Springer, 1973).Google Scholar
Katz, N., Slope filtration of $F$-crystals, Astérisque 63 (1979), 113–163.Google Scholar
Laumon, G., Cohomology of Drinfeld modular varieties. Part I, Cambridge Studies in Advanced Mathematics, vol. 41 (Cambridge University Press, Cambridge, 1996).Google Scholar
Mornev, M., Shtuka cohomology and Goss $L$-values, PhD thesis (2018), arXiv:1808.00839.Google Scholar
Mornev, M., Tate modules of isocrystals and good reduction of Drinfeld modules, Algebra Number Theory 15 (2021), 909–970.Google Scholar
Papanikolas, M. A., Tannakian duality for Anderson–Drinfeld motives and algebraic independence of Carlitz logarithms, Invent. Math. 171 (2008), 123–174.Google Scholar
Papanikolas, M. A. and Ramachandran, N., A Weil–Barsotti formula for Drinfeld modules, J. Number Theory 98 (2003), 407–431.Google Scholar
Pink, R., Hodge structures over function fields, Preprint (1997), https://people.math.ethz.ch/~pink/ftp/HS.pdf.Google Scholar
Quillen, D., Higher algebraic K-theory. I, in Algebraic K-theory, I: Higher K-theories, Lecture Notes in Mathematics, vol. 341 (Springer, 1973), 167–171.Google Scholar
Quillen, D., Projective modules over polynomial rings, Invent. Math. 36 (1976).Google Scholar
Rosen, M., Number theory in function fields, Graduate Texts in Mathematics, vol. 210 (Springer, 2002).Google Scholar
Scholl, A. J., Remarks on special values of L-functions, in L-functions and arithmetic, eds J. Coates and M. J. Taylor, London Mathematics Society Lecture Notes Series, vol. 153 (Cambridge University Press, 1991).Google Scholar
Serre, J.-P., Corps locaux, Publications de l'Université de Nancago, No. VIII (Hermann, Paris, 1968).Google Scholar
The Stacks Project Authors, Stacks Project, https://stacks.math.columbia.edu.Google Scholar
Taelman, L., Artin $t$-motifs, J. Number Theory 129 (2009), 142–157.Google Scholar
Taelman, L., A Dirichlet unit theorem for Drinfeld modules, Math. Ann. 348 (2010), 899–907.Google Scholar
Taelman, L., Sheaves and functions modulo p, lectures on the Woods Hole trace formula, London Mathematical Society Lecture Note Series, vol. 429 (Cambridge University Press, 2015).Google Scholar
Taelman, L., 1-t-motifs, in t-motives: Hodge structures, transcendence and other motivic aspects, EMS Series of Congress Reports (European Mathematical Society, Zürich, 2020), 417–439.Google Scholar
Taguchi, Y., The Tate conjecture for $t$-motives, Proc. Amer. Math. Soc. 123 (1995), 3285–3287.Google Scholar
Tagushi, Y. and Wan, D., $L$-functions of $\varphi$-sheaves and Drinfeld modules, J. Amer. Math. Soc. 9 (1996), 755–781.Google Scholar
Tamagawa, A., Generalization of Anderson's t-motives and Tate conjecture, in Moduli spaces, Galois representations and L-functions, RIMS Kôkyûroku, vol. 884 (Research Institute for Mathematical Sciences, 1994).Google Scholar
Weibel, C., An introduction to homological algebra, Cambridge Studies in Advanced Mathematics (Cambridge University Press, 1994).Google Scholar