Hostname: page-component-cb9f654ff-pvkqz Total loading time: 0 Render date: 2025-09-04T08:31:52.324Z Has data issue: false hasContentIssue false
  • English
  • Français

Period relations for Rankin–Selberg convolutions for 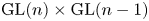${\mathrm {GL}}(n)\times {\mathrm {GL}}(n-1)$

Part of: Lie groups Discontinuous groups and automorphic forms

Published online by Cambridge University Press:  11 September 2024

Jian-Shu Li ,
Dongwen Liu  and
Binyong Sun
Jian-Shu Li
Affiliation:
Institute for Advanced Study in Mathematics, Zhejiang University, Hangzhou 310058, China jianshu@zju.edu.cn
Dongwen Liu
Affiliation:
School of Mathematical Sciences, Zhejiang University, Hangzhou 310058, China maliu@zju.edu.cn
Binyong Sun
Affiliation:
Institute for Advanced Study in Mathematics and New Cornerstone Science Laboratory, Zhejiang University, Hangzhou 310058, China sunbinyong@zju.edu.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

We formulate and prove the archimedean period relations for Rankin–Selberg convolutions for ${\mathrm {GL}}(n)\times {\mathrm {GL}}(n-1)$. As a consequence, we prove the period relations for critical values of the Rankin–Selberg L-functions for 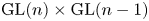${\mathrm {GL}}(n)\times {\mathrm {GL}}(n-1)$ over arbitrary number fields.

Keywords

cohomological representationRankin–Selberg convolutioncritical valueL-functionperiod relation

MSC classification

Primary: 11F67: Special values of automorphic $L$-series, periods of modular forms, cohomology, modular symbols
Secondary: 11F70: Representation-theoretic methods; automorphic representations over local and global fields 11F75: Cohomology of arithmetic groups 22E45: Representations of Lie and linear algebraic groups over real fields

Information

Type
Research Article
Information
Compositio Mathematica , Volume 160 , Issue 8 , August 2024 , pp. 1871 - 1915
Check for updates
Copyright
© 2024 The Author(s). The publishing rights in this article are licensed to Foundation Compositio Mathematica under an exclusive licence

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Aizenbud, A. and Gourevitch, D., Schwartz functions on Nash manifolds, Int. Math. Res. Not. IMRN 2008 (2008), rnm155.CrossRefGoogle Scholar
Aizenbud, A. and Gourevitch, D., Multiplicity one theorem for $({{\mathrm {GL}}}_{n+1}(\mathbb {R}),{{\mathrm {GL}}}_n(\mathbb {R}))$, Selecta Math. (N.S.) 15 (2009), 271–294.CrossRefGoogle Scholar
Blasius, D., Period relations and critical values of L-functions, Pacific J. Math. (1997), 53–83, Special issue: Olga Taussky-Todd: in memoriam.CrossRefGoogle Scholar
Borel, A., Stable real cohomology of arithmetic groups II, Progress in Mathematics, vol. 14 (Birkhäuser, 1981), 21–35.Google Scholar
Bott, R., Homogeneous vector bundles, Ann. of Math. (2) 66 (1957), 203–248.CrossRefGoogle Scholar
Chen, S.-Y., Algebraicity of ratios of special values of Rankin–Selberg L-functions and applications to Deligne's conjecture, Preprint (2022), arXiv:2205.15382.Google Scholar
Chen, S.-Y., On Deligne's conjecture for symmetric fifth L-functions of modular forms, Forum Math. 34 (2022), 831–849.CrossRefGoogle Scholar
Chen, S.-Y., On Deligne's conjecture for symmetric fourth L-functions of Hilbert modular forms, Adv. Math. 414 (2023), 108860.CrossRefGoogle Scholar
Clozel, L., Motifs et formes automorphes: applications du principe de fonctorialité. [Motives and automorphic forms: applications of the functoriality principle], in Automorphic forms, Shimura varieties, and L-functions, Vol. I (Ann Arbor, MI, 1988), Perspectives in Mathematics, vol. 10 (Academic Press, Boston, MA, 1990), 77–159.Google Scholar
Deligne, P., Valeurs de fonctions L et périodes d'intégrales, in Automorphic forms, representations and L-functions. Part 2, Proceedings of Symposia in Pure Mathematics, vol. 33 (American Mathematical Society, Providence, RI, 1979), 313–346.CrossRefGoogle Scholar
Du Cloux, F., Sur les représentations différentiables des groupes de Lie algébriques, Ann. Sci. Éc. Norm. Supér. (4) 24 (1991), 257–318.CrossRefGoogle Scholar
Grobner, H., Rationality for isobaric automorphic representations: the CM-case, Monatsh. Math. 187 (2018), 79–94.CrossRefGoogle Scholar
Grobner, H. and Harris, M., Whittaker periods, motivic periods, and special values of tensor product L-functions, J. Inst. Math. Jussieu 15 (2016), 711–769.CrossRefGoogle Scholar
Grobner, H. and Lin, J., Special values of $L$-functions and the refined Gan–Gross–Prasad conjecture, Amer. J. Math. 143 (2021), 859–937.CrossRefGoogle Scholar
Grobner, H. and Zunar, S., On the notion of the parabolic and the cuspidal support of smooth-automorphic forms and smooth-automorphic representations, Monatsh. Math. 204 (2024), 455–500.CrossRefGoogle Scholar
Hara, T. and Namikawa, K., A cohomological interpretation of archimedean zeta integrals for ${\mathrm {GL}}_3\times {\mathrm {GL}}_2$, Res. Number Theory 7 (2021), article 68.CrossRefGoogle Scholar
Hara, T. and Namikawa, K., A motivic interpretation of Whittaker periods for ${\mathrm {GL}}_n$, Manuscripta Math. 174 (2024), 303–353.CrossRefGoogle Scholar
Harder, G., General aspects in the theory of modular symbols, in Seminar on number theory, Paris 1981–82 (Paris, 1981/1982), Progress in Mathematics, vol. 38 (Birkhäuser, Boston, MA, 1983), 73–88.Google Scholar
Harder, G. and Raghuram, A., Eisenstein cohomology for GLN and the special values of Rankin–Selberg L-functions, Annals of Mathematics Studies, vol. 203 (Princeton University Press, Princeton, NJ, 2020).Google Scholar
Harris, M. and Lin, J., Period relations and special values of Rankin–Selberg L-functions, in Representation theory, number theory, and invariant theory, Progress in Mathematics, vol. 323 (Birkhäuser/Springer, Cham, 2017), 235–264.CrossRefGoogle Scholar
Hida, H., On the critical values of $L$-functions of ${\mathrm {GL}}(2)$ and ${\mathrm {GL}}(2) \times {\mathrm {GL}}(2)$, Duke Math. J. 74 (1994), 431–529.Google Scholar
Hirano, M., Ishii, T. and Miyazaki, T., Archimedean zeta integrals for ${\mathrm {GL}}(3)\times {\mathrm {GL}}(2)$, Mem. Amer. Math. Soc. 278 (2022).Google Scholar
Hochschild, G. and Mostow, G., Cohomology of Lie groups, Illinois J. Math. 6 (1962), 367–401.CrossRefGoogle Scholar
Ishii, T. and Miyazaki, T., Calculus of archimedean Rankin–Selberg integrals with recurrence relations, Represent. Theory 26 (2022), 714–763.CrossRefGoogle Scholar
Jacquet, H., Principal L-functions of the linear group, in Automorphic forms, representations and L-functions. Part 2, Proceedings of Symposia in Pure Mathematics, vol. 33 (American Mathematical Society, Providence, RI, 1979), 63–86.CrossRefGoogle Scholar
Jacquet, H., Archimedean Rankin–Selberg integrals, in Automorphic forms and L-functions II: local aspects, Contemporary Mathematics, vol. 489 (AMS and BIU, 2009), 57–172.Google Scholar
Jacquet, H. and Langlands, R., Automorphic forms on GL(2), Lecture Notes in Mathematics, vol. 114 (Springer, Berlin–New York, 1970).CrossRefGoogle Scholar
Jacquet, H., Piatetskii-Shapiro, I. I. and Shalika, J. A., Rankin–Selberg convolutions, Amer. J. Math. 105 (1983), 367–464.CrossRefGoogle Scholar
Jacquet, H. and Shalika, J. A., On Euler products and the classification of automorphic representations. I, Amer. J. Math. 103 (1981), 499–558.CrossRefGoogle Scholar
Jacquet, H. and Shalika, J. A., On Euler products and the classification of automorphic forms. II, Amer. J. Math. 103 (1981), 777–815.CrossRefGoogle Scholar
Januszewski, F., Modular symbols for reductive groups and $p$-adic Rankin–Selberg convolutions over number fields, J. Reine Angew. Math. 653 (2011), 1–45.CrossRefGoogle Scholar
Januszewski, F., On $p$-adic L-functions for ${\mathrm {GL}}(n)\times {\mathrm {GL}}(n-1)$ over totally real fields, Int. Math. Res. Not. IMRN 2015 (2015), 7884–7949.CrossRefGoogle Scholar
Januszewski, F., $p$-adic L-functions for Rankin–Selberg convolutions over number fields, Ann. Math. Qué. 40 (2016), 453–489.CrossRefGoogle Scholar
Januszewski, F., On period relations for automorphic L-functions I, Trans. Amer. Math. Soc. 371 (2019), 6547–6580.CrossRefGoogle Scholar
Januszewski, F., Non-abelian p-adic Rankin–Selberg L-functions and non-vanishing of central L-values, Amer. J. Math. 146 (2024), 495–578.CrossRefGoogle Scholar
Jiang, D., Sun, B. and Tian, F., Period relations for standard $L$-functions of symplectic type, Preprint (2019), arXiv:1909.03476.Google Scholar
Kasten, H. and Schmidt, C.-G., On critical values of Rankin–Selberg convolutions, Int. J. Number Theory 9 (2013), 205–256.CrossRefGoogle Scholar
Kazhdan, D., Mazur, B. and Schmidt, C.-G., Relative modular symbols and Rankin–Selberg convolutions, J. Reine Angew. Math. 519 (2000), 97–141.Google Scholar
Knapp, A., Local Langlands correspondence: the archimedean case, in Motives (Seattle, WA, 1991), Proceedings of Symposia in Pure Mathematics, vol. 55, Part 2 (American Mathematical Society, Providence, RI, 1994), 393–410.Google Scholar
Kudla, S., Tate's thesis, in An introduction to the Langlands Program (Birkhäuser, Boston, MA, 2003), 109–131.Google Scholar
Langlands, R. P., On the notion of an automorphic representation. A supplement to the preceding paper, in Automorphic forms, representations and L-functions. Part 1, Proceedings of Symposia in Pure Mathematics, vol. 33 (American Mathematical Society, Providence, RI, 1979), 203–207.CrossRefGoogle Scholar
Li, J.-S. and Sun, B., Low degree cohomologies of congruence groups, Sci. China Math. 62 (2019), 2287–2308.CrossRefGoogle Scholar
Li, J.-S., Liu, D., Su, F. and Sun, B., Rankin–Selberg convolutions for ${\mathrm {GL}}(n)\times {\mathrm {GL}}(n)$ and ${\mathrm {GL}}(n)\times {\mathrm {GL}}(n-1)$ for principal series representations, Sci. China Math. 66 (2023), 2203–2218.CrossRefGoogle Scholar
Mahnkopf, J., Cohomology of arithmetic groups, parabolic subgroups and the special values of L-functions of ${\mathrm {GL}}_n$, J. Inst. Math. Jussieu. 4 (2005), 553–637.CrossRefGoogle Scholar
Manin, Y. I., Parabolic points and zeta functions of modular curves, Izv. Akad. Nauk SSSR Ser. Mat. 36 (1972), 19–66.Google Scholar
Manin, Y. I., Periods of cusp forms, and $p$-adic Hecke series, Mat. Sb. (N.S.) 92 (1973), 378–401, 503.Google Scholar
Manin, Y. I., Non-archimedean integration and Jacquet–Langlands $p$-adic $L$-functions, Russian Math. Surveys 31 (1976), 5–57.CrossRefGoogle Scholar
Raghuram, A., On the special values of certain Rankin–Selberg L-functions and applications to odd symmetric power L-functions of modular forms, Int. Math. Res. Not. IMRN 2010 (2010), 334–372.CrossRefGoogle Scholar
Raghuram, A., Critical values of Rankin–Selberg L-functions for ${\mathrm {GL}}(n) \times {\mathrm {GL}}(n-1)$ and the symmetric cube L-functions for ${\mathrm {GL}}(2)$, Forum Math. 28 (2016), 457–489.CrossRefGoogle Scholar
Raghuram, A., Eisenstein cohomology for ${\mathrm {GL}}(N)$ and the special values of Rankin–Selberg L-functions over a totally imaginary number field, Preprint (2022), arXiv:2207.03393.Google Scholar
Raghuram, A. and Shahidi, F., Functoriality and special values of L-functions, in Eisenstein series and applications, eds W. T. Gan, S. Kudla and Y. Tschinkel, Progress in Mathematics, vol. 258 (Birkhäuser, Boston, MA, 2008), 271–294.CrossRefGoogle Scholar
Raghuram, A. and Shahidi, F., On certain period relations for cusp forms on ${\mathrm {GL}}_n$, Int. Math. Res. Not. IMRN 2008 (2008), rnn077.Google Scholar
Schmidt, C.-G., $p$-adic measures attached to automorphic representations of ${\mathrm {GL}}(3)$, Invent. Math. 92 (1988), 597–631.CrossRefGoogle Scholar
Schmidt, C.-G., Relative modular symbols and $p$-adic Rankin–Selberg convolutions, Invent. Math. 112 (1993), 31–76.CrossRefGoogle Scholar
Schmidt, C.-G., Period relations and $p$-adic measures, Manuscripta Math. 106 (2001), 177–201.CrossRefGoogle Scholar
Shimura, G., Sur les intégrales attachées aux formes automorphes, J. Math. Soc. Japan 11 (1959), 291–311.Google Scholar
Shimura, G., The special values of the zeta functions associated with cusp forms, Comm. Pure Appl. Math. 29 (1976), 783–804.CrossRefGoogle Scholar
Shimura, G., On the periods of modular forms, Math. Ann. 229 (1977), 211–221.CrossRefGoogle Scholar
Shimura, G., The special values of the zeta functions associated with Hilbert modular forms, Duke Math. J. 45 (1978), 637–679.CrossRefGoogle Scholar
Springer, T. A., Linear algebraic groups, Progress in Mathematics, vol. 9, second edition (Birkhäuser, Boston, MA, 1998).CrossRefGoogle Scholar
Sun, B., The nonvanishing hypothesis at infinity for Rankin–Selberg convolutions, J. Amer. Math. Soc. 30 (2017), 1–25.CrossRefGoogle Scholar
Sun, B. and Zhu, C.-B., Multiplicity one theorems: the archimedean case, Ann. of Math. (2) 175 (2012), 23–44.CrossRefGoogle Scholar
Tate, J., Number theoretic background, in Automorphic forms, representations and L-functions. Part 2, Proceedings of Symposia in Pure Mathematics, vol. 33 (American Mathematical Society, Providence, RI, 1979), 3–26.CrossRefGoogle Scholar
Trèves, F., Topological vector spaces, distributions and kernels (Academic Press, New York–London, 1967.Google Scholar
Vogan, D., Gelfand–Kirillov dimension for Harish-Chandra modules, Invent. Math. 48 (1978), 75–98.CrossRefGoogle Scholar
Vogan, D. and Zuckerman, G., Unitary representations with non-zero cohomology, Compos. Math. 53 (1984), 51–90.Google Scholar
Waldspurger, J.-L., Quelques propriétés arithmétiques de certaines formes automorphes sur ${\mathrm {GL}}(2)$, Compos. Math. 54 (1985), 121–171.Google Scholar
Wallach, N., Real reductive groups I, Pure and Applied Mathematics, vol. 132 (Academic Press, Boston, MA, 1988.Google Scholar
Wallach, N., Real reductive groups II, Pure and Applied Mathematics, vol. 132-II (Academic Press, Boston, MA, 1992.Google Scholar
Yoshida, H., Motives and Siegel modular forms, Amer. J. Math. 123 (2001), 1171–1197.CrossRefGoogle Scholar