No CrossRef data available.
Article contents
Sturmian words and Cantor sets arising from unique expansions over ternary alphabets
Published online by Cambridge University Press: 25 January 2018
Abstract
Over a finite alphabet  $A$ of real numbers, unique expansions in base
$A$ of real numbers, unique expansions in base 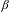 $\unicode[STIX]{x1D6FD}$ are considered. A real number
$\unicode[STIX]{x1D6FD}$ are considered. A real number 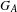 $G_{A}$ called the generalized golden ratio is a critical point of a situation of unique expansions. If
$G_{A}$ called the generalized golden ratio is a critical point of a situation of unique expansions. If 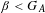 $\unicode[STIX]{x1D6FD}<G_{A}$, then there are only trivial unique expansions in base
$\unicode[STIX]{x1D6FD}<G_{A}$, then there are only trivial unique expansions in base 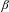 $\unicode[STIX]{x1D6FD}$, while there are non-trivial unique expansions in base
$\unicode[STIX]{x1D6FD}$, while there are non-trivial unique expansions in base 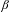 $\unicode[STIX]{x1D6FD}$ whenever
$\unicode[STIX]{x1D6FD}$ whenever  $\unicode[STIX]{x1D6FD}>G_{A}$. Komornik, Lai and Pedicini [Generalized golden ratios of ternary alphabets. J. Eur. Math. Soc.13(4) (2011), 1113–1146] investigated the case where
$\unicode[STIX]{x1D6FD}>G_{A}$. Komornik, Lai and Pedicini [Generalized golden ratios of ternary alphabets. J. Eur. Math. Soc.13(4) (2011), 1113–1146] investigated the case where  $A$ consists of three real numbers, and demonstrated that Sturmian words curiously emerge out of the generalized golden ratio. The present paper focuses on Sturmian words under this context. For a given alphabet
$A$ consists of three real numbers, and demonstrated that Sturmian words curiously emerge out of the generalized golden ratio. The present paper focuses on Sturmian words under this context. For a given alphabet 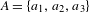 $A=\{a_{1},a_{2},a_{3}\}$ with
$A=\{a_{1},a_{2},a_{3}\}$ with  $a_{1}<a_{2}<a_{3}$, we give a complete characterization of the corresponding Sturmian words effectively and algorithmically, which reveals interesting structures behind the generalized golden ratios.
$a_{1}<a_{2}<a_{3}$, we give a complete characterization of the corresponding Sturmian words effectively and algorithmically, which reveals interesting structures behind the generalized golden ratios.
- Type
- Original Article
- Information
- Copyright
- © Cambridge University Press, 2018




