No CrossRef data available.
Article contents
Asymptotic distribution for pairs of linear and quadratic forms at integral vectors
Published online by Cambridge University Press: 17 April 2024
Abstract
We study the joint distribution of values of a pair consisting of a quadratic form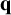 
${\mathbf q}$ and a linear form 
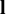${\mathbf l}$ over the set of integral vectors, a problem initiated by Dani and Margulis [Orbit closures of generic unipotent flows on homogeneous spaces of 
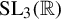$\mathrm{SL}_3(\mathbb{R})$. Math. Ann. 286 (1990), 101–128]. In the spirit of the celebrated theorem of Eskin, Margulis and Mozes on the quantitative version of the Oppenheim conjecture, we show that if 
$n \ge 5$, then under the assumptions that for every 
$(\alpha , \beta ) \in {\mathbb {R}}^2 \setminus \{ (0,0) \}$, the form 
$\alpha {\mathbf q} + \beta {\mathbf l}^2$ is irrational and that the signature of the restriction of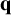 
${\mathbf q}$ to the kernel of 
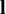${\mathbf l}$ is 
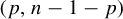$(p, n-1-p)$, where 
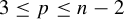${3\le p\le n-2}$, the number of vectors 
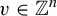$v \in {\mathbb {Z}}^n$ for which 
$\|v\| < T$, 
$a < {\mathbf q}(v) < b$ and 
$c< {\mathbf l}(v) < d$ is asymptotically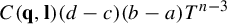 
$ C({\mathbf q}, {\mathbf l})(d-c)(b-a)T^{n-3}$ as 
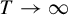$T \to \infty $, where 
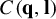$C({\mathbf q}, {\mathbf l})$ only depends on 
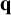${\mathbf q}$ and 
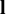${\mathbf l}$. The density of the set of joint values of 
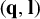$({\mathbf q}, {\mathbf l})$ under the same assumptions is shown by Gorodnik [Oppenheim conjecture for pairs consisting of a linear form and a quadratic form. Trans. Amer. Math. Soc. 356(11) (2004), 4447–4463].
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press
References
Abbaspour, H. and Moskowitz, M.. Basic Lie Theory. World Scientific Publishing Co. Pte. Ltd., Hackensack, NJ, 2007.CrossRefGoogle Scholar
Bandi, P., Ghosh, A. and Han, J.. A generic effective Oppenheim theorem for systems of forms. J. Number Theory 218 (2021), 311–333.CrossRefGoogle Scholar
Benoist, Y. and Quint, J.-F.. Random walks on finite volume homogeneous spaces. Invent. Math. 187(1) (2012), 37–59.CrossRefGoogle Scholar
Borel, A.. Linear Algebraic Groups (Graduate Texts in Mathematics, 126), 2nd edn. Springer-Verlag, New York, 1991.CrossRefGoogle Scholar
Borel, A. and -Chandra, Harish. Arithmetic subgroups of algebraic groups. Ann. of Math. (2) 75 (1962), 485–535.CrossRefGoogle Scholar
Dani, S. and Margulis, G.. Orbit closures of generic unipotetn flows on homogeneous spaces of
$\mathrm{SL}_3(\mathbb{R})$
. Math. Ann. 286 (1990), 101–128.CrossRefGoogle Scholar
$\mathrm{SL}_3(\mathbb{R})$
. Math. Ann. 286 (1990), 101–128.CrossRefGoogle ScholarDani, S. and Margulis, G.. Limit distributions of orbits of unipotent flows and values of quadratic forms. Seminar (Advances in Soviet Mathematics, 16, Part 1). Ed. Gel’fand, I. M.. American Mathematical Society, Providence, RI, 1993, pp. 91–137.Google Scholar
Dani, S. G.. Invariant measures and minimal sets of horospherical flows. Invent. Math. 64 (1981), 357–385.CrossRefGoogle Scholar
Eskin, A., Margulis, G. and Mozes, S.. Upper bounds and asymptotics in a quantitative version of the Oppenheim conjecture. Ann. of Math. (2) 147(1) (1998), 93–141.CrossRefGoogle Scholar
Eskin, A., Margulis, G. and Mozes, S.. Quadratic forms of signature
$\left(2,2\right)$
and eigenvalue spacings on rectangular 2-tori. Ann. of Math. (2) 161(2) (2005), 679–725.CrossRefGoogle Scholar
$\left(2,2\right)$
and eigenvalue spacings on rectangular 2-tori. Ann. of Math. (2) 161(2) (2005), 679–725.CrossRefGoogle ScholarGorodnik, A.. Oppenheim conjecture for pairs consisting of a linear form and a quadratic form. Trans. Amer. Math. Soc. 356(11) (2004), 4447–4463.CrossRefGoogle Scholar
Han, J.. Rogers’ mean value theorem for
$S$
-arithmetic Siegel transforms and applications to the geometry of numbers. J. Number Theory 240 (2022), 74–106.CrossRefGoogle Scholar
$S$
-arithmetic Siegel transforms and applications to the geometry of numbers. J. Number Theory 240 (2022), 74–106.CrossRefGoogle ScholarHan, J.. Weight decomposition of
$\mathfrak{sl}_{d}(\mathbb{R})$
with respect to the adjoint representation of
$\mathfrak{so}(p,q)$
. Preprint, 2024, arXiv:2402.12929.Google Scholar
$\mathfrak{sl}_{d}(\mathbb{R})$
with respect to the adjoint representation of
$\mathfrak{so}(p,q)$
. Preprint, 2024, arXiv:2402.12929.Google ScholarMalcev, A.. On the representation of an algebra as a direct sum of the radical and a semi-simple subalgebra. C. R. (Doklady) Acad. Sci. URSS (N.S.) 36 (1942), 42–45.Google Scholar
Margulis, G. A.. Formes quadratriques indéfinies et flots unipotents sur les espaces homogénes. C. R. Acad. Sci. Paris. Sér. I Math. 304(10) (1987), 249–253 (in French summary).Google Scholar
Oppenheim, A.. The minima of indefinite quaternary quadratic forms. Proc. Natl. Acad. Sci. USA 15(9) (1929), 724–727.CrossRefGoogle ScholarPubMed
Platonov, V. and Rapinchuk, A.. Algebraic Groups and Number Theory (Pure and Applied Mathematics, 139). Academic Press, Inc., Boston, MA, 1994, translated from the 1991 Russian original by R. Rowen.Google Scholar
Ratner, M.. Raghunathan’s topological conjecture and distributions of unipotent flows. Duke Math. J. 63(1) (1991), 235–280.CrossRefGoogle Scholar
Sargent, O.. Equidistribution of values of linear forms on quadratic surfaces. Algebra Number Theory 8(4) (2014), 895–932.CrossRefGoogle Scholar
Shah, N.. Uniformly distributed orbits of certain flows on homogeneous spaces. Math. Ann. 289(2) (1991), 315–334.CrossRefGoogle Scholar
Siegel, C.. A mean value theorem in geometry of numbers. Ann. of Math. (2) 46 (1945), 340–347.CrossRefGoogle Scholar
Whitney, H.. Elementary structure of real algebraic varieties. Ann. of Math. (2) 66 (1957), 545–556.CrossRefGoogle Scholar