Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Aspenberg, Magnus
2024.
Slowly recurrent Collet–Eckmann maps on the Riemann sphere.
Mathematische Annalen,
Article contents
Critical recurrence in the real quadratic family
Published online by Cambridge University Press: 08 November 2022
Abstract
We study recurrence in the real quadratic family and give a sufficient condition on the recurrence rate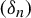 
$(\delta _n)$ of the critical orbit such that, for almost every non-regular parameter a, the set of n such that 
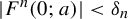$\vert F^n(0;a) \vert < \delta _n$ is infinite. In particular, when 
$\delta _n = n^{-1}$, this extends an earlier result by Avila and Moreira [Statistical properties of unimodal maps: the quadratic family. Ann. of Math. (2) 161(2) (2005), 831–881].
MSC classification
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press
References
Avila, A. and Moreira, C. G.. Statistical properties of unimodal maps: the quadratic family. Ann. of Math. (2) 161(2) (2005), 831–881.CrossRefGoogle Scholar
Aspenberg, M.. Slowly recurrent Collet–Eckmann maps on the Riemann sphere. Preprint, 2022, arXiv:2103.14432.Google Scholar
Baladi, V., Benedicks, M. and Schnellmann, D.. Whitney–Hölder continuity of the SRB measure for transversal families of smooth unimodal maps. Invent. Math. 201(3) (2015), 773–844.CrossRefGoogle Scholar
Benedicks, M. and Carleson, L.. On iterations of
$1-a{x}^2$
on
$(-1,1)$
. Ann. of Math. (2) 122(1) (1985), 1–25.CrossRefGoogle Scholar
$1-a{x}^2$
on
$(-1,1)$
. Ann. of Math. (2) 122(1) (1985), 1–25.CrossRefGoogle ScholarBenedicks, M. and Carleson, L.. The dynamics of the Hénon map. Ann. of Math. (2) 133(1) (1991), 73–169.CrossRefGoogle Scholar
Baladi, V. and Viana, M.. Strong stochastic stability and rate of mixing for unimodal maps. Ann. Sci. Éc. Norm. Supér. (4) 29(4) (1996), 483–517.CrossRefGoogle Scholar
Collet, P. and Eckmann, J.-P.. On the abundance of aperiodic behaviour for maps on the interval. Comm. Math. Phys. 73(2) (1980), 115–160.CrossRefGoogle Scholar
de Melo, W and van Strien, S.. One-Dimensional Dynamics (Ergebnisse der Mathematik und ihrer Grenzgebiete (3) [Results in Mathematics and Related Areas (3)], 25). Springer-Verlag, Berlin, 1993.CrossRefGoogle Scholar
Graczyk, J. and Światek, G.. Generic hyperbolicity in the logistic family. Ann. of Math. (2) 146(1) (1997), 1–52.CrossRefGoogle Scholar
Gao, B. and Shen, W.. Summability implies Collet–Eckmann almost surely. Ergod. Th. & Dynam. Sys. 34(4) (2014), 1184–1209.CrossRefGoogle Scholar
Jakobson, M. V.. Absolutely continuous invariant measures for one-parameter families of one-dimensional maps. Comm. Math. Phys. 81(1) (1981), 39–88.CrossRefGoogle Scholar
Keller, G. and Nowicki, T.. Spectral theory, zeta functions and the distribution of periodic points for Collet–Eckmann maps. Comm. Math. Phys. 149(1) (1992), 31–69.CrossRefGoogle Scholar
Kozlovski, O., Shen, W. and van Strien, S.. Density of hyperbolicity in dimension one. Ann. of Math. (2) 166(1) (2007), 145–182.CrossRefGoogle Scholar
Levin, G.. Perturbations of weakly expanding critical orbits. Frontiers in Complex Dynamics (Princeton Mathematical Series, 51). Eds. A. Bonifant, M. Lyubich and S. Sutherland. Princeton University Press, Princeton, NJ, 2014, pp. 163–196.CrossRefGoogle Scholar
Lyubich, M.. Dynamics of quadratic polynomials. I, II. Acta Math. 178(2) (1997), 185–247, 247–297.CrossRefGoogle Scholar
Lyubich, M.. Dynamics of quadratic polynomials. III. Parapuzzle and SBR measures. Astérisque 261 (2000), 239–252.Google Scholar
Lyubich, M.. The quadratic family as a qualitatively solvable model of chaos. Notices Amer. Math. Soc. 47(9) (2000), 1042–1052.Google Scholar
Lyubich, M.. Almost every real quadratic map is either regular or stochastic. Ann. of Math. (2) 156(1) (2002), 1–78.CrossRefGoogle Scholar
Martens, M. and Nowicki, T.. Invariant measures for typical quadratic maps. Astérisque 261 (2000), 239–252.Google Scholar
Nowicki, T. and Sands, D.. Non-uniform hyperbolicity and universal bounds for
$S$
-unimodal maps. Invent. Math. 132(3) (1998), 633–680.CrossRefGoogle Scholar
$S$
-unimodal maps. Invent. Math. 132(3) (1998), 633–680.CrossRefGoogle ScholarPrzytycki, F., Rivera-Letelier, J. and Smirnov, S.. Equivalence and topological invariance of conditions for non-uniform hyperbolicity in the iteration of rational maps. Invent. Math. 151(1) (2003), 29–63.CrossRefGoogle Scholar
Przytycki, F.. On the Perron–Frobenius–Ruelle operator for rational maps on the Riemann sphere and for Hölder continuous functions. Bol. Soc. Brasil Mat. (N.S.) 20(2) (1990), 95–125.CrossRefGoogle Scholar
Świa̧tek, G.. Collet–Eckmann condition in one-dimensional dynamics. Smooth Ergodic Theory and Its Applications (Seattle, WA, 1999) (Proceedings of Symposia in Pure Mathematics, 69). Eds. A. Katok, R. de la Llave, Y. Pesin and H. Weiss. American Mathematical Society, Providence, RI, 2001, pp. 489–498.CrossRefGoogle Scholar
Schlömilch, O.. Ueber die gleichzeitige Convergenz oder Divergenz zweier Reihen, Z. Math. Phys., Zeitschrift für Mathematik und Physik, 18 (1873), 425–426.Google Scholar
Smale, S.. Mathematical problems for the next century. Math. Intelligencer 20(2) (1998), 7–15.CrossRefGoogle Scholar
Tsujii, M.. Positive Lyapunov exponents in families of one-dimensional dynamical systems. Invent. Math. 111(1) (1993), 113–137.CrossRefGoogle Scholar
Tsujii, M.. A simple proof for monotonicity of entropy in the quadratic family. Ergod. Th. & Dynam. Sys. 20(3) (2000), 925–933.CrossRefGoogle Scholar
Young, L.-S.. Decay of correlations for certain quadratic maps. Comm. Math. Phys. 146(1) (1992), 123–138.CrossRefGoogle Scholar
- 1
- Cited by