Hostname: page-component-77c89778f8-gvh9x Total loading time: 0 Render date: 2024-07-17T13:29:59.927Z Has data issue: false hasContentIssue false
  • English
  • Français

Singular analytic linear cocycles with negative infinite Lyapunov exponents

Published online by Cambridge University Press:  17 August 2017

CHRISTIAN SADEL  and
DISHENG XU
CHRISTIAN SADEL
Affiliation:
Institute of Science and Technology, 3400 Klosterneuburg, Austria and Facultad de Matemáticas, Pontificia Universidad Católica, Santiago de Chile, Chile email chsadel@mat.uc.cl
DISHENG XU
Affiliation:
Université Paris Diderot, Sorbonne Paris Cité, Institut de Mathématiques de Jussieu-Paris Rive Gauche, UMR 7586, CNRS, Sorbonne Universités, UPMC Université Paris 06, F-75013, Paris, France email disheng.xu@imj-prg.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

We show that linear analytic cocycles where all Lyapunov exponents are negative infinite are nilpotent. For such one-frequency cocycles we show that they can be analytically conjugated to an upper triangular cocycle or a Jordan normal form. As a consequence, an arbitrarily small analytic perturbation leads to distinct Lyapunov exponents. Moreover, in the one-frequency case where the 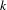$k$th Lyapunov exponent is finite and the 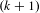$(k+1)$st negative infinite, we obtain a simple criterion for domination in which case there is a splitting into a nilpotent part and an invertible part.

Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 39 , Issue 4 , April 2019 , pp. 1082 - 1098
Copyright
© Cambridge University Press, 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Avila, A.. Density of positive Lyapunov exponents for SL(2, ℝ)-cocycles. J. Amer. Math. Soc. 24 (2011), 999–1014.Google Scholar
Avila, A.. Global theory of one-frequency Schrödinger operators. Acta Math. 215 (2015), 1–54.Google Scholar
Arnold, L., Cong, N. D. and Oseledets, V. I.. Jordan normal form for linear cocycles. Random Oper. Stoch. Equ. 7 (1999), 301–356.Google Scholar
Avila, A., Jitomirskaya, S. and Sadel, C.. Complex one-frequency cocycles. J. Eur. Math. Soc. (JEMS) 16(9) (2014), 1915–1935.Google Scholar
Avila, A. and Krikorian, R.. Monotonic cocycles. Invent. Math. 202 (2015), 271–331.Google Scholar
Avila, A. and Viana, M.. Simplicity of Lyapunov spectra: proof of the Zorich–Kontsevich conjecture. Acta Math. 198(1) (2007), 1–56.Google Scholar
Bourgain, J. and Jitomirskaya, S.. Continuity of the Lyapunov exponent for quasiperiodic operators with analytic potential. J. Stat. Phys. 108 (2002), 1203–1218.Google Scholar
Bochi, J.. Genericity of zero Lyapunov exponents. Ergod. Th. & Dynam. Sys. 22 (2002), 1667–1696.Google Scholar
Bourgain, J.. Positivity and continuity of the Lyapounov exponent for shifts on T d with arbitrary frequency vector and real analytic potential. J. Anal. Math. 96 (2005), 313–355.Google Scholar
Bochi, J. and Viana, M.. Lyapunov exponents: how frequently are dynamical systems hyperbolic? Modern Dynamical Systems and Applications. Eds. Brin, M., Hasselblatt, B. and Pesin, Y.. Cambridge University Press, Cambridge, UK, 2004, pp. 271–297.Google Scholar
Duarte, P. and Klein, S.. Positive Lyapunov exponents for higher dimensional quasiperiodic cocycles. Comm. Math. Phys. 332 (2014), 189–219.Google Scholar
Duarte, P. and Klein, S.. Continuity, positivity and simplicity of the Lyapunov exponents for quasi-periodic cocycles. J. Eur. Math. Soc., to appear, Preprint, 2016, arXiv:1603.06851 (2016).Google Scholar
Furstenberg, H. and Kesten, H.. Products of random matrices. Ann. Math. Stat. 31 (1960), 457–469.Google Scholar
Gol’dsheid, I. Y. and Margulis, G. A.. Lyapunov indices of a product of random matrices. Russian Math. Surveys 44(5) (1989), 11–71.Google Scholar
Guivarc’h, Y. and Raugi, A.. Frontiére de Furstenberg, propriétés de contraction et théoremes de convergence. Probab. Theory Related Fields 69(2) (1985), 187–242.Google Scholar
Goldstein, M. and Schlag, W.. Hölder continuity of the integrated density of states for quasi-periodic Schrödinger equations. Ann. of Math. (2) 154 (2001), 155–203.Google Scholar
Oseledets, V. I.. A multiplicative ergodic theorem. Lyapunov characteristic numbers for dynamical systems. Trans. Moscow Math. Soc. 19 (1968), 197–231.Google Scholar
Ruelle, D.. Ergodic theory of differentiable dynamical systems. Publ. Math. Inst. Hautes Études Sci. 50 (1979), 27–58.Google Scholar
Sadel, C.. A Herman–Avila–Bochi formula for higher-dimensional pseudo-unitary and Hermitian-symplectic cocycles. Ergod. Th. & Dynam. Sys. 35 (2015), 1582–1591.Google Scholar
Simon, B.. Kotani theory for one dimensional stochastic Jacobi matrices. Commun. Math. Phys. 89 (1983), 227–234.Google Scholar
Viana, M.. Almost all cocycles over any hyperbolic system have nonvanishing Lyapunov exponents. Ann. of Math. (2) 167 (2008), 643–680.Google Scholar
Xu, D.. Density of positive Lyapunov exponents for higher dimensional cocycles and Schrödinger cocycles on the strips. J. Eur. Mah. Soc., to appear, Preprint, 2015, arXiv:1506.05403.Google Scholar