No CrossRef data available.
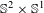 $\mathbb{S}^{2}\times \mathbb{S}^{1}$
$\mathbb{S}^{2}\times \mathbb{S}^{1}$Published online by Cambridge University Press: 13 April 2015
In this paper, we use abstract Lyapunov graphs as a combinatorial tool to obtain a complete classification of Smale flows on  $\mathbb{S}^{2}\times \mathbb{S}^{1}$. This classification gives necessary and sufficient conditions that must be satisfied by an (abstract) Lyapunov graph in order for it to be associated to a Smale flow on
$\mathbb{S}^{2}\times \mathbb{S}^{1}$. This classification gives necessary and sufficient conditions that must be satisfied by an (abstract) Lyapunov graph in order for it to be associated to a Smale flow on 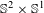 $\mathbb{S}^{2}\times \mathbb{S}^{1}$.
$\mathbb{S}^{2}\times \mathbb{S}^{1}$.