Hostname: page-component-7479d7b7d-wxhwt Total loading time: 0 Render date: 2024-07-13T16:05:28.894Z Has data issue: false hasContentIssue false
  • English
  • Français

INTEGRABLE SYSTEMS IN SYMPLECTIC GEOMETRY

Published online by Cambridge University Press:  01 February 2009

E. ASADI  and
J. A. SANDERS
E. ASADI
Affiliation:
Department of Mathematics, Faculty of Sciences, Vrije Universiteit, Amsterdam, The Netherlands
J. A. SANDERS
Affiliation:
Department of Mathematics, Faculty of Sciences, Vrije Universiteit, Amsterdam, The Netherlands
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Quaternionic vector mKDV equations are derived from the Cartan structure equation in the symmetric space = Sp(n+1)/Sp(1) × Sp(n). The derivation of the soliton hierarchy utilizes a moving parallel frame and a Cartan connection 1-form ω related to the Cartan geometry on modelled on 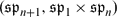. The integrability structure is shown to be geometrically encoded by a Poisson–Nijenhuis structure and a symplectic operator.

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 51 , Issue A , February 2009 , pp. 5 - 23
Copyright
Copyright © Glasgow Mathematical Journal Trust 2009

References

REFERENCES

1.Anco, S. C., Group-invariant soliton equations and bi-Hamiltonian geometric curve flows in Riemannian symmetric spaces, J. Geom. Phys. 58 (2008), 1–37.CrossRefGoogle Scholar
2.Arvanitoyeorgos, A., An introduction to Lie groups and the geometry of homogeneous spaces, volume 22 of Student Mathematical Library. American Mathematical Society, Providence, RI, 2003. Translated from the 1999 Greek original and revised by the author.CrossRefGoogle Scholar
3.Asadi, E., Integrable system in symplectic geometry, PhD thesis (vrije Universiteit Amsterdam, 2008).CrossRefGoogle Scholar
4.Doliwa, A. and Santini, P. M., An elementary geometric characterization of the integrable motions of a curve, Phys. Lett. A, 185 (4) (1994), 373–384.CrossRefGoogle Scholar
5.Dorfman, I., Dirac structures and integrability of nonlinear evolution equations, in Nonlinear science: Theory and applications (John Wiley & Sons Ltd., Chichester, 1993).Google Scholar
6.Hasimoto, R., A soliton on vortex filament, J. Fluid Mechanics, 51 (1972), 477–485.CrossRefGoogle Scholar
7.Sigurdur, Helgason, Differential geometry, Lie groups, and symmetric spaces, in Pure and applied mathematics, vol 80 (Academic Press Inc., New York, 1978).Google Scholar
8.Kosmann-Schwarzbach, Y. and Magri, F., Lax-Nijenhuis operators for integrable systems, J. Math. Phys., 37 (12) (1996), 6173–6197.CrossRefGoogle Scholar
9.Magri, F., Morosi, C. and Ragnisco, O., Reduction techniques for infinite-dimensional Hamiltonian systems: some ideas and applications, Comm. Math. Phys., 99 (1) (1985), 115–140.CrossRefGoogle Scholar
10.Magri, F. and Morosi, C., On the reduction theory of the Nijenhuis operators and its applications to Gel′fand-Dikiĭ equations, in: IUTAM-ISIMM symposium on modern developments in analytical mechanics, Vol. II (Proc. Sympos. Torino, 1982), (1983), 599–626.Google Scholar
11.Marí Beffa, G., Sanders, J. A. and Wang, J. P., Integrable systems in 3-dimensional Riemannian geometry, J. Nonlinear Sci., 12 (2) (2002), 143–167.CrossRefGoogle Scholar
12.Olver, P. J., Applications of Lie groups to Differential Equations (Springer, New York, 1986).CrossRefGoogle Scholar
13.Jan, A. Sanders and Wang, Jing Ping, Integrable systems in n-dimensional Riemannian geometry, Mosc. Math. J., 3 (4) (2003), 1369–1393.Google Scholar
14.Jan, A. Sanders and Wang, Jing Ping, Integrable systems in n-dimensional conformal geometry, J. Difference Equ. Appl., 12 (10) (2006), 983–995.Google Scholar
15.Sharpe, R. W., Differential geometry, in Graduate Texts in Mathematics, vol. 166 (Springer-Verlag, New York, 1997). Cartan's generalization of Klein's Erlangen program, With a foreword by S. S. Chern.Google Scholar
16.Terng, Chuu-Lian, Soliton equations and differential geometry, J. Differential Geom., 45 (2) (1997), 407–445.CrossRefGoogle Scholar
17.Terng, Chuu-Lian and Thorbergsson, Gudlaugur, Completely integrable curve flows on adjoint orbits. Results Math., 40 (1–4) (2001) 286–309. (Dedicated to Shiing-Shen Chern on his 90th birthday.)CrossRefGoogle Scholar
18.Terng, Chuu-Lian and Uhlenbeck, Karen, Schrödinger flows on Grassmannians in Integrable systems, geometry, and topology, vol. 36 of AMS/IP Stud. Adv. Math., Amer. Math. Soc., Providence, RI, (2006), 235–253.CrossRefGoogle Scholar