Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-23T20:51:11.603Z Has data issue: false hasContentIssue false
  • English
  • Français

A model for the spreading of fake news

Part of: Limit theorems Combinatorial probability

Published online by Cambridge University Press:  04 May 2020

Hosam Mahmoud
Hosam Mahmoud*
Affiliation:
The George Washington University
*
*Postal address: Department of Statistics, The George Washington University, Washington, D.C.20052, U.S.A. Email address: hosam@gwu.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

We introduce a model for the spreading of fake news in a community of size n. There are $j_n = \alpha n - g_n$ active gullible persons who are willing to believe and spread the fake news, the rest do not react to it. We address the question ‘How long does it take for 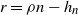$r = \rho n - h_n$ persons to become spreaders?’ (The perturbation functions $g_n$ and 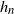$h_n$ are o(n), and 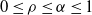$0\le \rho \le \alpha\le 1$ .) The setup has a straightforward representation as a convolution of geometric random variables with quadratic probabilities. However, asymptotic distributions require delicate analysis that gives a somewhat surprising outcome. 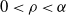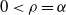Normalized appropriately, the waiting time has three main phases: (a) away from the depletion of active gullible persons, when $0< \rho < \alpha$ , the normalized variable converges in distribution to a Gumbel random variable; (b) near depletion, when $0< \rho = \alpha$ , with $h_n - g_n \to \infty$ , the normalized variable also converges in distribution to a Gumbel random variable, but the centering function gains weight with increasing perturbations; (c) at almost complete depletion, when 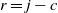$r = j -c$ , for integer 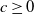$c\ge 0$ , the normalized variable converges in distribution to a convolution of two independent generalized Gumbel random variables. The influence of various perturbation functions endows the three main phases with an infinite number of phase transitions at the seam lines.

Keywords

fake newsrumorsGumbel distributionphase transition

MSC classification

Primary: 60C05: Combinatorial probability
Secondary: 60F05: Central limit and other weak theorems
Type
Research Papers
Information
Journal of Applied Probability , Volume 57 , Issue 1 , March 2020 , pp. 332 - 342
Copyright
© Applied Probability Trust 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Belen, S., Kropat, E. and Weber, G. (2009). On the classical Maki–Thompson rumour model in continuous time. Cent. Eur. J. Oper. Res. 19, 1–17.CrossRefGoogle Scholar
Daley, D. and Kendall, D. (1965). Stochastic rumours. J. Inst. Math. Appl. 1, 42–55.CrossRefGoogle Scholar
Doumas, A. and Papanicolaou, V. (2012). The coupon collector’s problem revisited: Asymptotics of the variance. Adv. Appl. Prob. 44, 166–195.CrossRefGoogle Scholar
Ferrante, M. and Saltalamacchia, M. (2014). The coupon collector’s problem. MATerials MATemàtics 2, 1887–1907.Google Scholar
Frauenthal, J. (1980). Mathematical Modeling in Epidemiology. Springer, Berlin.CrossRefGoogle Scholar
Junior, V., Machado, F. and Zuluaga, M. (2011). Rumor processes on ${\mathbb N}$ . J. Appl. Prob. 48, 624–636.CrossRefGoogle Scholar
Karr, A. (1993). Probability. Springer, New York.CrossRefGoogle Scholar
Maki, D. and Thomson, M. (1973). Mathematical Models and Applications. Prentice-Hall, Englewood Cliff, NJ.Google Scholar
Molchanov, S. and Whitmeyer, M. (2010). Two Markov models of the spread of rumors. J. Math. Sociol. 34, 157–166.CrossRefGoogle Scholar
Ojo, M. (2001). Some relationships between the generalized Gumbel and other distributions. Kragujevac J. Math. 23, 101–106.Google Scholar
Pittel, B. (1987). On spreading a rumor. SIAM J. Appl. Math. 47, 213–223.CrossRefGoogle Scholar
Pittel, B. (1990). On a Daley–Kendall model of random rumours. J. Appl. Prob. 27, 14–27.CrossRefGoogle Scholar
Sudbury, A. (1985). The proportion of the population never hearing a rumour. J. Appl. Prob. 22, 443–446.CrossRefGoogle Scholar
Svensson, A. (1993). On the duration of a Maki–Thompson epidemic. Math. Biosci. 117, 211–220.CrossRefGoogle ScholarPubMed
Watson, R. (1987). On the size of rumour. Stoch. Proc. Appl. 27, 141–149.CrossRefGoogle Scholar
Whittaker, E. and Watson, G. (1990). A Course in Modern Analysis, 4th edn. Cambridge University Press.Google Scholar