Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-18T01:28:55.579Z Has data issue: false hasContentIssue false
  • English
  • Français

Clean versus contaminated bubbles in a solid-body rotating flow

Published online by Cambridge University Press:  13 October 2017

Marie Rastello Open the ORCID record for Marie Rastello [Opens in a new window] ,
Jean-Louis Marié  and
Michel Lance
Marie Rastello*
Affiliation:
Laboratoire de Mécanique des Fluides et d’Acoustique, CNRS, Université de Lyon, Ecole Centrale de Lyon, 36 avenue Guy de Collongue, 69134 Ecully CEDEX, France Univ. Grenoble Alpes, CNRS, Grenoble INP, LEGI, 38000 GrenobleFrance
Jean-Louis Marié
Affiliation:
Laboratoire de Mécanique des Fluides et d’Acoustique, CNRS, Université de Lyon, Ecole Centrale de Lyon, 36 avenue Guy de Collongue, 69134 Ecully CEDEX, France
Michel Lance
Affiliation:
Laboratoire de Mécanique des Fluides et d’Acoustique, CNRS, Université de Lyon, Ecole Centrale de Lyon, 36 avenue Guy de Collongue, 69134 Ecully CEDEX, France
*
†Email address for correspondence: marie.rastello@legi.cnrs.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

The behaviour of clean and contaminated bubbles in solid-body rotating flows is compared in terms of drag and lift forces. Both spherical and deformed bubbles are considered. For that comparison, we have completed the data published in Rastello et al. (J. Fluid Mech., vol. 624, 2009, pp. 159–178; J. Fluid Mech., vol. 682, 2011, pp. 434–459) by a new series of measurements. When they are contaminated, bubbles are subject to an additional lift force due to the spinning of their surfaces, while the clean ones are not. A detailed description of this spinning motion is presented and an expression for the Magnus-like lift it induces is given in the light of the new information. 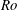The component of the lift induced by flow rotation depends on the Rossby number $Ro$, contrary to the case of clean bubbles. Including the ‘spin’ induced lift component in the dynamical equations provides a better prediction of the bubble’s trajectory in contaminated fluid. The presence of contaminants immobilizes the rear part of the bubble and reduces significantly the deformation. The laws of deformation according to the nature of the surface are presented. The way deformation influences the drag and lift coefficients in pure and contaminated fluids is quantified and discussed. Expressions for these various coefficients are proposed.

JFM classification

Drops and Bubbles: Drops and Bubbles
Type
Papers
Information
Journal of Fluid Mechanics , Volume 831 , 25 November 2017 , pp. 592 - 617
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adoua, R., Legendre, D. & Magnaudet, J. 2009 Reversal of the lift force on an oblate bubble in a weakly viscous shear flow. J. Fluid Mech. 628, 23–41.Google Scholar
Adoua, S. R.2007 Hydrodynamique d’une bulle déformée dans un écoulement cisaillé. PhD thesis, Institut National Polytechnique de Toulouse.Google Scholar
Aoyama, S., Hayashi, K., Hosokawa, S., Lucas, D. & Tomiyama, A. 2017 Lift force acting on single bubbles in linear shear flows. Intl J. Multiphase Flow 96, 113–122.CrossRefGoogle Scholar
Aoyama, S., Hayashi, K., Hosokawa, S. & Tomiyama, A. 2016 Shape of ellipsoidal bubbles in infinite stagnant liquids. Intl J. Multiphase Flow 79, 23–30.Google Scholar
Bagchi, P. & Balachandar, S. 2002 Effect of free rotation on the motion of a solid sphere in linear shear flow at moderate Re . Phys. Fluids 14 (8), 2719–2737.CrossRefGoogle Scholar
Bel-Fdhila, R. & Duineveld, P. C. 1996 The effect of surfactant on the rise of a spherical bubble at high Reynolds and Peclet numbers. Phys. Fluids 8, 310–321.Google Scholar
Blanco, A. & Magnaudet, J. 1995 The structure of the axisymmetric high-Reynolds number flow around an ellipsoidal bubble of fixed shape. Phys. Fluids 7, 1265–1274.Google Scholar
Bluemink, J. J., Lohse, D., Prosperetti, A. & Van Wijngaarden, L. 2008 A sphere in a uniformly rotating or shearing flow. J. Fluid Mech. 600, 201–233.Google Scholar
Bluemink, J. J., Lohse, D., Prosperetti, A. & Van Wijngaarden, L. 2010 Drag and lift forces on particles in a rotating flow. J. Fluid Mech. 643, 1–31.Google Scholar
Clift, R., Grace, J. R. & Weber, M. E. 1978 Bubbles, Drops and Particles. Academic.Google Scholar
Cuenot, B., Magnaudet, J. & Spennato, B. 1997 The effects of slightly soluble surfactants on the flow around a spherical bubble. J. Fluid Mech. 339, 25–53.Google Scholar
Duineveld, P. C. 1995 The rise velocity and shape of bubbles in pure water at high Reynolds number. J. Fluid Mech. 292, 325–332.Google Scholar
Giacobello, M., Ooi, A. & Balanchandar, S. 2009 Wake structure of a transversely rotating sphere at moderate Reynolds numbers. J. Fluid Mech. 621, 103–130.Google Scholar
Haberman, W. L. & Morton, R. K.1953 An experimental investigation of the drag and shape of air bubbles rising in various liquids. Tech. Rep. 802, David W. Taylor Model Basin.Google Scholar
Johnson, T. A. & Patel, V. C. 1999 Flow past a sphere up to a Reynolds number of 300. J. Fluid Mech. 378, 19–70.Google Scholar
Joseph, D. D. 2006 Rise velocity of a spherical cap bubble. J. Fluid Mech. 488, 213–223.Google Scholar
Lamb, H. 1932 Hydrodynamics, 6th edn. Dover.Google Scholar
Legendre, D. & Magnaudet, J. 1998 The lift force on a spherical bubble in a viscous linear shear flow. J. Fluid Mech. 368, 81–126.CrossRefGoogle Scholar
Legendre, D., Zenit, R. & Velez-Cordero, J. R. 2012 On the deformation of gas bubbles in liquids. Phys. Fluids 24, 043303.CrossRefGoogle Scholar
Loth, E. 2008 Quasi-steady shape and drag of deformable bubbles and drops. Intl J. Multiphase Flow 34 (6), 523–546.CrossRefGoogle Scholar
Magnaudet, J. & Eames, I. 2000 The motion of high-Reynolds-number bubbles in inhomogeneous flows. Annu. Rev. Fluid Mech. 32, 659–708.Google Scholar
Magnaudet, J. & Legendre, D. 1998 Some aspects of the lift force on a spherical bubble. Appl. Sci. Res. 58, 441–461.Google Scholar
Magnaudet, J., Rivero, M. & Fabre, J. 1995 Accelerated flows past a rigid sphere or a spherical bubble. J. Fluid Mech. 284, 97–135.Google Scholar
McLaughlin, J. B. 1996 Numerical simulation of bubble motion in water. J. Colloid Interface Sci. 184, 613–625.Google Scholar
Mei, R., Klausner, J. & Lawrence, C. 1994 A note on the history force on a spherical bubble at finite Reynolds number. Phys. Fluids 6, 418–420.CrossRefGoogle Scholar
Moore, D. W. 1959 The rise of a gas bubble in a viscous liquid. J. Fluid Mech. 6, 113–130.Google Scholar
Moore, D. W. 1965 The velocity rise of distorted gas bubbles in a liquid of small viscosity. J. Fluid Mech. 23, 749–766.CrossRefGoogle Scholar
Naciri, A.1992 Contribution à l’étude des forces exercées par un liquide sur une bulle de gaz: portance, masse ajoutée et interactions hydrodynamiques. PhD thesis, Ecole Centrale de Lyon.Google Scholar
van Nierop, E. A., Luther, S., Bluemink, J. J., Magnaudet, J., Prosperetti, A. & Lohse, D. 2007 Drag and lift forces on bubbles in a rotating flow. J. Fluid Mech. 571, 439–454.Google Scholar
Rastello, M., Marié, J. L., Grosjean, N. & Lance, M. 2009 Drag and lift forces on interface-contaminated bubbles spinning in a rotating flow. J. Fluid Mech. 624, 159–178.Google Scholar
Rastello, M., Marié, J. L. & Lance, M. 2011 Drag and lift forces on clean spherical and ellipsoidal bubbles in a solid-body rotating flow. J. Fluid Mech. 682, 434–459.Google Scholar
Schiller, L. & Naumann, A. Z. 1933 Uber die grundlegenden Berechnungen bei der Schwerkraftaufbereitung. Ver. Deut. Ing. 77, 318–320.Google Scholar
Taylor, T. D. & Acrivos, A. 1964 On the deformation and drag of a falling viscous drop at low Reynolds number. J. Fluid Mech. 18, 466–476.CrossRefGoogle Scholar
Zhang, Z. & Prosperetti, A. 2005 A second-order method for three-dimensional particle simulation. J. Comput. Phys. 210, 292–324.Google Scholar