Hostname: page-component-84b7d79bbc-g5fl4 Total loading time: 0 Render date: 2024-07-29T10:54:38.274Z Has data issue: false hasContentIssue false
  • English
  • Français

Inertio-gravity waves under the non-traditional 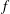$f$-plane approximation: singularity in the large-scale limit

Published online by Cambridge University Press:  01 December 2016

Jun-Ichi Yano
Jun-Ichi Yano*
Affiliation:
CNRM UMR 3589, Météo-France, 42 av Coriolis, 31057 Toulouse CEDEX, France CNRS, 31057 Toulouse CEDEX, France
*
†Email address for correspondence: jiy.gfder@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

The low horizontal wavenumber limit of waves on a plane under a constant rotation rate (the so-called $f$-plane) is degenerate: all wave frequencies asymptotically approach the inertial frequency. This degeneracy has no serious consequence when the rotation axis is perpendicular to the plane (traditional $f$-plane approximation). However, when the rotation axis is tilted from the vertical direction (non-traditional $f$-plane approximation), we encounter a type of ‘singularity’ in the sense that each term of the Taylor expansion of the wave frequency in the horizontal Coriolis parameter diverges in the limit of low horizontal wavenumber. Such a drastic change of the solution behaviour by adding the horizontal Coriolis parameter in the low horizontal wavenumber limit is rather counter-intuitive, because the conventional scale analysis suggests that the horizontal Coriolis effect is negligible in this limit. However, the degeneracy of the system makes this effect critical with a need for considering higher-order terms. Two possible asymptotic limits are proposed for resolving this degeneracy. One of them, as it turns out, amounts to a representation of the wave frequency by a Taylor expansion in the horizontal wavenumber.

JFM classification

Geophysical and Geological Flows: Geophysical and Geological Flows Geophysical and Geological Flows: Internal waves Geophysical and Geological Flows: Waves in rotating fluids
Type
Papers
Information
Journal of Fluid Mechanics , Volume 810 , 10 January 2017 , pp. 475 - 488
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bender, C. M. & Orszag, S. A. 1978 Advanced Mathematical Methods for Scientists and Engineers. McGraw-Hill.Google Scholar
Colin de Verdière, A. & Schopp, R. 1994 Flows in a rotating spherical shell: the equatorial case. J. Fluid Mech. 276, 233–260.CrossRefGoogle Scholar
Dellar, P. J. 2011 Variations on a beta-plane: derivation of non-traditional beta-plane equations from Hamilton’s principle on a sphere. J. Fluid Mech. 674, 174–195.Google Scholar
Dellar, P. J. & Salmon, R. 2005 Shallow water equations with a complete Coriolis force and topography. Phys. Fluids 17, 106601.CrossRefGoogle Scholar
Durran, D. R. & Bretherton, C. 2004 Comments on ‘The roles of the horizontal component of the Earth’s angular velocity in nonhydrostatic linear models. J. Atmos. Sci. 61, 1982–1986.Google Scholar
Eckart, C. 1960 Hydrodynamics of Oceans and Atmospheres. Pergamon.Google Scholar
Fruman, M. D. 2009 Equatorially bounded zonally propagating linear waves on a generalized 𝛽 plane. J. Atmos. Sci. 66, 2937–2945.Google Scholar
Gerkema, T. & Shrira, V. I. 2005 Near-inertial waves in the ocean: beyond the ‘traditional approximation’. J. Fluid Mech. 529, 195–219.Google Scholar
Gerkema, T., Zimmerman, J. T. F., Maas, L. R. M. & van Haren, H. 2008 Geophysical and astrophysical fluid dynamics beyond the traditional approximation. Rev. Geophys. 46, 2006RG000220.Google Scholar
Glazunov, A. V. 2010 On the effect that the direction of geostrophic wind has on turbulence and quasiordered large-scale structures in the atmospheric boundary layer. Isv. Atmos. Ocan. Phys. 46, 727–747.Google Scholar
Greenspan, H. P. 1968 The Theory of Rotating Fluids. Cambridge University Press.Google Scholar
Harlander, U. & Maas, L. R. M. 2007 Internal boundary layers in a well-mixed equatorial atmosphere/ocean. Dyn. Atmos. Oceans 44, 1–28.CrossRefGoogle Scholar
Hayashi, M. & Itoh, H. 2012 The importance of the nontraditional Coriolis terms in large-scale motions in the tropics forced by prescribed cumulus heating. J. Atmos. Sci. 69, 2699–2716.Google Scholar
Holton, J. R. 2004 An Introduction to Dynamic Meteorology, 4th edn. International Geophysical Series, vol. 88. Academic.Google Scholar
Kasahara, A. 2003a The role of the horizontal component of the Earth’s angular velocity in nonhydrostatic linear models. J. Atmos. Sci. 60, 1085–1095.Google Scholar
Kasahara, A. 2003b On the nonhydrostatic atmospheric models with inclusion of the horizontal component of the Earth’s angular velocity. J. Met. Soc. Japan 81, 935–950.Google Scholar
Kasahara, A. 2007 Initial-value approach to study the inertio-gravity waves without the ‘traditional approximation’. J. Comput. Phys. 225, 2175–2197.Google Scholar
Kasahara, A. 2009 A mechanism of deep-ocean mixing due to near-inertial waves generated by flow over topography. Dyn. Atmos. Ocean 49, 124–140.Google Scholar
Maas, L. R. M. & Harlander, U. 2007 Equatorial wave attractors and inertial oscillations. J. Fluid Mech. 570, 46–67.Google Scholar
Pedlosky, J. 1987 Geophysical Fluid Dynamics, 2nd edn. Springer.CrossRefGoogle Scholar
Rabitti, A. & Maas, L. R. M. 2013 Meridional trapping and zonal propagation of inertial waves in a rotating fluid shell. J. Fluid Mech. 729, 445–470.CrossRefGoogle Scholar
Rabitti, A. & Maas, L. R. M. 2014 Inertial wave rays in rotating spheric fluid domains. J. Fluid Mech. 758, 621–654.Google Scholar
Reznik, G. M. 2013 Linear dynamics of a stably-neutrally stratified ocean. J. Mar. Res. 71, 253–288.Google Scholar
Reznik, G. M. 2014a Geostrophic adjustment with gyroscopic waves: barotropic fluid without the traditional approximation. J. Fluid Mech. 743, 585–605.Google Scholar
Reznik, G. M. 2014b Geostrophic adjustment with gyroscopic waves: stably neutrally stratified fluid without the traditional approximation. J. Fluid Mech. 747, 605–634.Google Scholar
Reznik, G. M. 2015 Wave motions in a stably-neutrally stratified ocean. Oceanology 55, 789–795.Google Scholar
Schopp, R. & Colin de Verdière, A. 1997 Taylor column between concentric spheres. Geophys. Astrophys. Fluid Dyn. 86, 43–73.CrossRefGoogle Scholar
Stern, M. E. 1963 Trapping of low frequency oscillations in an equatorial ‘boundary layer’. Tellus 15, 246–250.CrossRefGoogle Scholar
Stewart, A. L. & Dellar, P. J. 2011a The rôle of the complete Coriolis force in cross-equatorial flow of abyssal ocean currents. Ocean Model. 38, 187–202.Google Scholar
Stewart, A. L. & Dellar, P. J. 2011b Cross-equatorial flow through an abyssal channel under the complete Coriolis force: two-dimensional solutions. Ocean Model. 40, 87–104.Google Scholar
Stewart, A. L. & Dellar, P. J. 2012 Cross-equatorial channel flow with zero potential vorticity under the complete Coriolis force. IMA J. Appl. Maths 77, 626–651.Google Scholar
Stewartson, K. 1971 On trapped oscillations of a rotating fluid in a thin spherical shell. Tellus 23, 506–510.Google Scholar
Stewartson, K. 1972 On trapped oscillations of a rotating fluid in a thin spherical shell II. Tellus 24, 283–287.CrossRefGoogle Scholar
Thuburn, J., Wood, N. & Staniforth, A. 2002 Normal modes of deep atmospheres. II: f–F-plane geometry. Q. J. R. Meteorol. Soc. 128, 1793–1806.Google Scholar
Tort, M., Ribstein, B. & Zeitlin, V. 2016 Symmetric and asymmetric inertial instability of zonal jets on the f-plane with complete Coriolis force. J. Fluid Mech. 788, 274–302.Google Scholar
White, A. A. & Bromley, R. A. 1995 Dynamically consistent, quasi-hydrostatic equations for global models with a complete representation of the Coriolis force. Q. J. R. Meteorol. Soc. 121, 399–418.Google Scholar