Hostname: page-component-54dcc4c588-sdd8f Total loading time: 0 Render date: 2025-10-01T17:23:13.808Z Has data issue: false hasContentIssue false
  • English
  • Français

A macrotransport equation for the particle distribution in the flow of a concentrated, non-colloidal suspension through a circular tube

Published online by Cambridge University Press:  08 October 2013

Arun Ramachandran
Arun Ramachandran*
Affiliation:
University of Toronto, Chemical Engineering and Applied Chemistry, 200 College Street, Toronto, Ontario, Canada M5S 3E5
*
†Email address for correspondence: engrarun@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

A two-time-scale perturbation expansion is used to derive a cross-section-averaged convection–dispersion equation for the particle distribution in the flow of a concentrated suspension of neutrally buoyant, non-colloidal particles through a straight, circular tube. 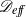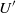Since the cross-streamline motion of particles is governed by shear-induced migration, the Taylor-dispersion coefficient ${\mathscr{D}}_{eff} $ scales as ${U}^{\prime } {R}^{3} / {a}^{2} $ , ${U}^{\prime } $ , $R$ and $a$ being the characteristic velocity scale, the tube radius and the particle radius, respectively. Here ${\mathscr{D}}_{eff} $ is found to decrease monotonically with an increase in the particle concentration. The linear dependence of ${\mathscr{D}}_{eff} $ on ${U}^{\prime } $ implies that changes in the cross-section averaged axial concentration profile are dependent only on the total axial strain experienced by the suspension. This stipulates that the spatial evolution of a fluctuation in the concentration of particles in the flowing suspension, or the width of the mixing zone between two regions of different concentrations in the tube will be independent of the suspension velocity in the tube. A second interesting feature in particulate dispersion is that the effective velocity of the particulate phase is concentration-dependent, which, by itself (i.e. without considering Taylor dispersion), can produce either sharpening or relaxation of concentration gradients. In particular, shocks with positive concentration gradients along the flow direction can asymptotically evolve into time-independent distributions in an appropriately chosen frame of reference, and concentration pulses relax asymmetrically. These trends are contrasted with those expected from the classical problem of Taylor dispersion of a passive tracer in the same geometry. The results in this paper are especially relevant for suspension flows through microfluidic geometries, where the induction lengths for shear-induced migration are short.

JFM classification

Complex Fluids: Suspensions Micro-/Nano-fluid dynamics: Microfluidics

Information

Type
Papers
Information
Journal of Fluid Mechanics , Volume 734 , 10 November 2013 , pp. 219 - 252
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Acrivos, A. 1994 Shear-induced particle diffusion in concentrated suspensions of noncolloidal particles. J. Rheol. 39 (5), 813–826.Google Scholar
Aris, R. 1956 On the dispersion of a solute in a fluid flowing through a tube. Proc. R. Soc. Lond. A 235 (1200), 67–77.Google Scholar
Brenner, H. & Edwards, D. A. 1993 Macrotransport Processes. Butterworth-Heinemann.Google Scholar
Bricker, J. M. & Butler, J. E. 2006 Oscillatory shear of suspensions of non-colloidal particles. J. Rheol. 50 (5), 711–728.CrossRefGoogle Scholar
Chapman, B. K. 1990 Shear-induced migration phenomena in suspensions. PhD thesis, University of Notre Dame.Google Scholar
Deboeuf, A., Gauthier, G., Martin, J., Morris, J. F. & Yurkovetsky, Y. 2009 Particle pressure in a sheared suspension: a bridge from osmosis to granular dilatancy. Phys. Rev. Lett. 102, 108301.Google Scholar
Delhaye, N., Poitou, A. & Chaouche, M. 2000 Squeeze flow of highly concentrated suspensions of spheres. J. Non-Newtonian Fluid Mech. 94, 67–74.Google Scholar
Eckstein, E. C., Bailey, D. G. & Shapiro, A. H. 1976 Self diffusion of particles in shear flow of a suspension. J. Fluid Mech. 79, 191–208.Google Scholar
Fahraeus, R. 1928 The suspension stability of blood. Physiol. Rev. 9 (2), 241–274.CrossRefGoogle Scholar
Fahraeus, R. & Lindqvist, T. 1930 The viscosity of the blood in narrow capillary tubes. Am. J. Physiol. 96 (3), 562–568.Google Scholar
Fang, Z., Mammoli, A. A., Brady, J. F., Ingber, M. S., Mondy, L. A. & Graham, A. L. 2002 Flow-aligned tensor models for suspension flows. Intl J. Multiphase Flow 28 (1), 137–166.Google Scholar
Frank, M., Anderson, D., Weeks, E. R. & Morris, J. F. 2003 Particle migration in pressure-driven flow of a Brownian suspension. J. Fluid Mech. 493, 363–378.Google Scholar
Gadala-Maria, F. & Acrivos, A. 1980 Shear-induced structure in a concentrated suspension of solid spheres. J. Rheol. 24 (6), 799–814.Google Scholar
Graham, A. L., Altobelli, S. A., Fukushima, E., Mondy, L. A. & Stephens, T. S. 1991 NMR imaging of shear-induced diffusion and structure in concentrated suspensions undergoing Couette flow. J. Rheol. 35 (1), 191–201.Google Scholar
Griffiths, I. M. & Stone, H. A. 2012 Axial dispersion via shear-enhanced diffusion in colloidal suspensions. Euro. Phys. Lett. 97, 58005.Google Scholar
Hampton, R. E., Mammoli, A. A., Graham, A. L. & Tetlow, N. 1997 Migration of particles undergoing pressure-driven flow in a circular conduit. J. Rheol. 41 (3), 621–640.Google Scholar
Hinch, E. J. 1991 Perturbation Methods. Cambridge University Press.Google Scholar
Holloway, W., Aristoff, J. M. & Stone, H. A. 2011 Imbibition of concentrated suspensions in capillaries. Phys. Fluids 23 (8), 081701.Google Scholar
Jana, S. C., Kapoor, B. & Acrivos, A. 1995 Apparent wall slip velocity coefficients in concentrated suspensions of noncolloidal particles. J. Rheol. 39 (6), 1123–1132.Google Scholar
Johansson, H., Olgard, G. & Jernqvist, A. 1970 Radial particle migration in plug flow – a method for solid–liquid separation and fractionation. Chem. Engng Sci. 25, 365–372.Google Scholar
Karnis, A. & Mason, S. G. 1967 The flow of suspensions through tubes. VI. Meniscus effects. J. Colloid Interface Sci. 23, 120–133.Google Scholar
Kolli, V. G., Pollauf, E. J. & Gadala-Maroa, F. 2002 Transient normal stress response in a concentrated suspension of spherical particles. J. Rheol. 46, 321–334.Google Scholar
Leighton, D. & Acrivos, A. 1987a Measurement of shear-induced self-diffusion in concentrated suspensions of spheres. J. Fluid Mech. 177, 109–131.Google Scholar
Leighton, D. & Acrivos, A. 1987b The shear-induced migration of particles in concentrated suspensions. J. Fluid Mech. 181, 415–439.CrossRefGoogle Scholar
Lighthill, M. J. & Whitham, G. B. 1955 On kinematic waves. II. A theory of traffic flow on long crowded roads. Proc. R. Soc. Lond. A 229 (1178), 317–345.Google Scholar
Miller, R. M. & Morris, J. F. 2006 Normal stress-driven migration and axial development in pressure-driven flow of concentrated suspensions. J. Non-Newtonian Fluid Mech. 135 (2–3), 149–165.Google Scholar
Mills, P. & Snabre, P. 1995 Rheology and structure of concentrated suspension of hard spheres: shear-induced particle migration. J. Phys. II 5, 1597–1608.Google Scholar
Morris, J. F. 2009 A review of microstructure in concentrated suspensions and its implications for rheology and bulk flow. Rheol. Acta 48, 909–923.Google Scholar
Morris, J. F. & Boulay, F. 1999 Curvilinear flows of non-colloidal suspensions: the role of normal stresses. J. Rheol. 43 (5), 1213–1237.Google Scholar
Narumi, T., See, H., Honma, Y., Hasegawa, T., Takahashi, T. & Phan-Thien, N. 2002 Transient response of concentrated suspensions after shear reversal. J. Rheol. 46, 295–305.Google Scholar
Nott, P. R. & Brady, J. F. 1994 Pressure-driven flow of suspensions: simulations and theory. J. Fluid Mech. 275, 157–199.Google Scholar
Pagitsas, M., Nadim, A. & Brenner, H. 1986 Multiple time scale analysis of macrotransport processes. Physica A 135, 533–550.CrossRefGoogle Scholar
Probstein, R. F. 1994 Physicochemical Hydrodynamics. John Wiley & Sons.Google Scholar
Ramachandran, A. & Leighton, D. T. 2007a The effect of gravity on the meniscus accumulation phenomenon in a tube. J. Rheol. 51 (5), 1073–1098.CrossRefGoogle Scholar
Ramachandran, A. & Leighton, D. T. 2007b Viscous resuspension in a tube: the impact of secondary flows resulting from second normal stress differences. Phys. Fluids 19 (5), 055301.CrossRefGoogle Scholar
Ramachandran, A. & Leighton, D. T. 2008 The influence of secondary flows induced by normal stress differences on the shear-induced migration of particles in concentrated suspensions. J. Fluid Mech. 603, 207–243.Google Scholar
Semwogerere, D., Morris, J. F. & Weeks, E. R. 2007 Development of particle migration in pressure-driven flow of a Brownian suspension. J. Fluid Mech. 581, 437–451.CrossRefGoogle Scholar
Sierou, A. & Brady, J. F. 2002 Rheology and microstructure in concentrated non-colloidal suspensions. J. Rheol. 46 (5), 1031–1056.Google Scholar
Taylor, G. 1953 Dispersion of soluble matter in solvent flowing slowly through a tube. Proc. R. Soc. Lond. A 219 (1137), 186–203.Google Scholar
Tetlow, N., Graham, A. L., Ingber, M. S., Subia, S. R., Mondy, L. A. & Altobelli, S. A. 1998 Particle migration in a Couette apparatus: experiment and modelling. J. Rheol. 42 (2), 307–327.Google Scholar
Yurkovetsky, Y. & Morris, J. F. 2008 Particle pressure in sheared Brownian suspensions. J. Rheol. 44 (2), 141–164.Google Scholar
Zarraga, I. E., Hill, D. A. & Leighton, D. T. 2000 The characterization of the total stress of concentrated suspensions of noncolloidal spheres in Newtonian fluids. J. Rheol. 52 (2), 185–220.Google Scholar
Zarraga, I. E. & Leighton, D. T. 2001 Normal stress and diffusion in a dilute suspension of hard spheres undergoing simple shear. Phys. Fluids 13 (3), 565–577.Google Scholar
Zhou, R. & Chang, H. C. 2005 Capillary penetration failure of blood suspensions. J. Colloid Interface Sci. 287 (2), 647–656.Google Scholar