Hostname: page-component-7bb8b95d7b-5mhkq Total loading time: 0 Render date: 2024-09-13T14:17:45.333Z Has data issue: true hasContentIssue false
  • English
  • Français

Magnetohydrodynamic flow control in Hele-Shaw cells

Published online by Cambridge University Press:  13 September 2024

Kyle I. McKee Open the ORCID record for Kyle I. McKee [Opens in a new window]
Kyle I. McKee*
Affiliation:
Department of Mathematics, Massachusetts Institute of Technology, Cambridge, MA 02139, USA Gulliver Laboratory, ESPCI Paris, PSL University, 75231 Paris CEDEX 05, France
*
†Email address for correspondence: kimckee@mit.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

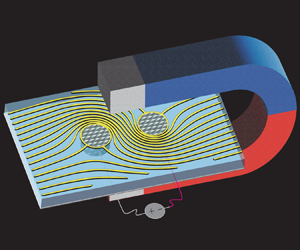
Consider the motion of a thin layer of electrically conducting fluid, between two closely spaced parallel plates, in a classical Hele-Shaw geometry. Furthermore, let the system be immersed in a uniform external magnetic field (normal to the plates) and let electrical current be driven between conducting probes immersed in the fluid layer. In the present paper, we analyse the ensuing fluid flow at low Hartmann numbers. Physically, the system is particularly interesting because it allows for circulation in the flow, which is not possible in the standard pressure-driven Hele-Shaw cell. We first elucidate the mechanism of flow generation both physically and mathematically. After formulating the problem using complex variables, we present mathematical solutions for a class of canonical multiply connected geometries in terms of the prime function framework developed by Crowdy (Solving Problems in Multiply Connected Domains, SIAM, 2020). We then demonstrate how recently developed fast numerical methods may be applied to accurately determine the flow field in arbitrary geometries.

JFM classification

Low-Reynolds-number flows: Hele-Shaw flows Micro-/Nano-fluid dynamics: Microfluidics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 993 , 25 August 2024 , A11
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlfors, L.V. 2010 Conformal Invariants: Topics in Geometric Function Theory, vol. 371. American Mathematical Society.Google Scholar
Alfvén, H. 1942 Existence of electromagnetic-hydrodynamic waves. Nature 150 (3805), 405–406.Google Scholar
Baddoo, P.J. 2020 Lightning solvers for potential flows. Fluids 5 (4), 227.Google Scholar
Baddoo, P.J. & Ayton, L.J. 2021 A calculus for flows in periodic domains. Theor. Comput. Fluid Dyn. 35 (2), 145–168.Google Scholar
Baddoo, P.J., Kurt, M., Ayton, L.J. & Moored, K.W. 2020 Exact solutions for ground effect. J. Fluid Mech. 891, R2.Google Scholar
Batchelor, G.K. 1967 An Introduction to Fluid Dynamics. Cambridge University Press.Google Scholar
Bau, H.H. 2022 Applications of magneto electrochemistry and magnetohydrodynamics in microfluidics. Magnetochemistry 8 (11), 140.Google Scholar
Bau, H.H., Zhong, J. & Yi, M. 2001 A minute magneto hydro dynamic (MHD) mixer. Sensors Actuators B: Chem. 79 (2-3), 207–215.Google Scholar
Booth, D.J., Griffiths, I.M. & Howell, P.D. 2023 Circular bubbles in a Hele-Shaw channel: a Hele-Shaw Newton's cradle. J. Fluid Mech. 954, A21.Google Scholar
Brubeck, P.D., Nakatsukasa, Y. & Trefethen, L.N. 2021 Vandermonde with Arnoldi. SIAM Rev. 63 (2), 405–415.Google Scholar
Costa, S. & Trefethen, L.N. 2023 AAA-least squares rational approximation and solution of Laplace problems. In European Congress of Mathematics Portorož, 20–26 June 2021, pp. 511–534. EMS.Google Scholar
Crowdy, D. 2006 a Calculating the lift on a finite stack of cylindrical aerofoils. Proc. R. Soc. Lond. A 462 (2069), 1387–1407.Google Scholar
Crowdy, D. 2007 Schwarz–Christoffel mappings to unbounded multiply connected polygonal regions. In Mathematical Proceedings of the Cambridge Philosophical Society, vol. 142, pp. 319–339. Cambridge University Press.Google Scholar
Crowdy, D. 2020 Solving Problems in Multiply Connected Domains. SIAM.Google Scholar
Crowdy, D.G., Kropf, E.H., Green, C.C. & Nasser, M.M.S. 2016 The Schottky–Klein prime function: a theoretical and computational tool for applications. IMA J. Appl. Maths 81 (3), 589–628, https://github.com/ehkropf/SKPrime.Google Scholar
Crowdy, D.G. 2006 b Analytical solutions for uniform potential flow past multiple cylinders. Eur. J. Mech. (B/Fluids) 25 (4), 459–470.Google Scholar
Crowdy, D.G. & Marshall, J.S. 2007 Computing the Schottky–Klein prime function on the Schottky double of planar domains. Comput. Meth. Funct. Theor. 7, 293–308.Google Scholar
David, C.S., Hester, E.W., Xu, Y. & Aurnou, J.M. 2023 Magneto-Stokes flow in a shallow free-surface annulus. J. Fluid Mech. (in press).Google Scholar
Davidson, P.A. 2001 An Introduction to Magnetohydrodynamics. Cambridge University Press.Google Scholar
Gonzalez, C. & Taha, H.E. 2022 A variational theory of lift. J. Fluid Mech. 941, A58.Google Scholar
Gopal, A. & Trefethen, L.N. 2019 a Representation of conformal maps by rational functions. Numer. Math. 142, 359–382.Google Scholar
Gopal, A. & Trefethen, L.N. 2019 b Solving Laplace problems with corner singularities via rational functions. SIAM J. Numer. Anal. 57 (5), 2074–2094.Google Scholar
Homsy, A., Koster, S., Eijkel, J.C.T., van den Berg, A., Lucklum, F., Verpoorte, E. & de Rooij, N.F. 2005 A high current density dc magnetohydrodynamic (MHD) micropump. Lab on a Chip 5 (4), 466–471.Google Scholar
Homsy, A.L.V.L.F, Linder, V., Lucklum, F. & de Rooij, N.F. 2007 Magnetohydrodynamic pumping in nuclear magnetic resonance environments. Sensors Actuators B: Chem. 123 (1), 636–646.Google Scholar
Levi, M. 2023 Conformal deformation of conductors. SIAM News, vol. 56 (April 2023).Google Scholar
Mirzadeh, M., Zhou, T., Amooie, M.A., Fraggedakis, D., Ferguson, T.R. & Bazant, M.Z. 2020 Vortices of electro-osmotic flow in heterogeneous porous media. Phys. Rev. Fluids 5 (10), 103701.Google Scholar
Miyoshi, H. & Crowdy, D.G. 2023 Generalized Schwarz integral formulas for multiply connected domains. SIAM J. Appl. Maths 83 (3), 966–984.Google Scholar
Moffatt, H.K. 1991 Electromagnetic stirring. Phys. Fluids A 3 (5), 1336–1343.Google Scholar
Moffatt, H.K. 1978 Field Generation in Electrically Conducting Fluids, vol. 2, p. 5–1. Cambridge University Press.Google Scholar
Moffatt, K. & Dormy, E. 2019 Self-Exciting Fluid Dynamos. Cambridge Texts in Applied Mathematics. Cambridge University Press.Google Scholar
Rossow, V.J. 1958 On flow of electrically conducting fluids over a flat plate in the presence of a transverse magnetic field. Tech. Rep. 1358. National Advisory Commitee For Aeronautics.Google Scholar
Taylor, G.I. 1967 Film Notes for Low Reynolds Number Flow, Illustrated Experiments in Fluid Mechanics: The National Committee for Fluid Mechanics Films Book of Film Notes. Educational Services Inc.Google Scholar
Trefethen, L.N. 2018 Series solution of Laplace problems. ANZIAM J. 60 (1), 1–26.Google Scholar
Vasconcelos, G.L., Marshall, J.S. & Crowdy, D.G. 2015 Secondary Schottky–Klein prime functions associated with multiply connected planar domains. Proc. R. Soc. Lond. A 471 (2173), 20140688.Google Scholar
Xue, Y., Waters, S.L. & Trefethen, L.N. 2024 Computation of two-dimensional Stokes flows via lightning and AAA rational approximation. SIAM J. Sci. Comput. 46 (2), A1214–A1234.Google Scholar
Yi, M., Qian, S. & Bau, H.H. 2002 A magnetohydrodynamic chaotic stirrer. J. Fluid Mech. 468, 153–177.Google Scholar
Zaltzman, B. & Rubinstein, I. 2007 Electro-osmotic slip and electroconvective instability. J. Fluid Mech. 579, 173–226.Google Scholar
Zhong, J., Yi, M. & Bau, H.H. 2002 Magneto hydrodynamic (MHD) pump fabricated with ceramic tapes. Sensors Actuators A: Phys. 96 (1), 59–66.Google Scholar