Hostname: page-component-848d4c4894-2xdlg Total loading time: 0 Render date: 2024-07-06T11:58:03.424Z Has data issue: false hasContentIssue false
  • English
  • Français

Nonlinear aspects of focusing internal waves

Published online by Cambridge University Press:  11 January 2019

Natalia D. Shmakova  and
Jan-Bert Flór Open the ORCID record for Jan-Bert Flór [Opens in a new window]
Natalia D. Shmakova
Affiliation:
Laboratoire des Écoulements Géophysiques et Industriels (LEGI), CNRS–Université Grenoble Alpes, F38000, Grenoble, France Lavrentyev Institute of Hydrodynamics, Siberian Branch of the Russian Academy of Sciences, Prospekt Lavrentyev 15, Novosibirsk 630090, Russia
Jan-Bert Flór*
Affiliation:
Laboratoire des Écoulements Géophysiques et Industriels (LEGI), CNRS–Université Grenoble Alpes, F38000, Grenoble, France
*
†Email address for correspondence: flor@legi.cnrs.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

When a torus oscillates horizontally in a linearly stratified fluid, the wave rays form a double cone, one upward and one downward, with two focal points where the wave amplitude has a maximum due to wave focusing. Following a former study on linear aspects of wave focusing (Ermanyuk et al., J. Fluid Mech., vol. 813, 2017, pp. 695–715), we here consider experimental results on the nonlinear aspects that occur in the focal region below the torus for higher-amplitude forcing. A new non-dimensional number that is based on heuristic arguments for the wave amplitude in the focal area is presented. This focusing number is defined as $Fo=(A/a)\unicode[STIX]{x1D716}^{-1/2}f(\unicode[STIX]{x1D703})$, with oscillation amplitude 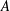$A$, 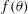$f(\unicode[STIX]{x1D703})$ a function for the variation of the wave amplitude with wave angle 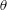$\unicode[STIX]{x1D703}$, and $\unicode[STIX]{x1D716}^{1/2}=\sqrt{b/a}$ the increase in amplitude due to the focusing, with $a$ and 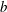$b$, respectively, the minor and major radius of the torus. Nonlinear effects occur for 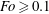$Fo\geqslant 0.1$, with the shear stress giving rise to a mean flow which results in the focal region in a central upward motion partially surrounded by a downward motion. With increasing $Fo$, the Richardson number 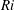$Ri$ measured from the wave steepness monotonically decreases. Wave breaking occurs at 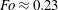$Fo\approx 0.23$, corresponding to 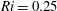$Ri=0.25$. In this regime, the focal region is unstable due to triadic wave resonance. For the different tori sizes under consideration, the triadic resonant instability in these three-dimensional flows resembles closely the resonance observed by Bourget et al. (J. Fluid Mech., vol. 723, 2013, pp. 1–20) for a two-dimensional flow, with only minor differences. Application to internal tidal waves in the ocean are discussed.

JFM classification

Geophysical and Geological Flows: Internal waves Geophysical and Geological Flows: Stratified flows
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 862 , 10 March 2019 , R4
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bourget, B., Dauxois, T., Joubaud, S. & Odier, P. 2013 Experimental study of parametric subharmonic instability for internal plane waves. J. Fluid Mech. 723, 1–20.Google Scholar
Brouzet, C., Sibgatullin, I. N., Scolan, H., Ermanyuk, E. V. & Dauxois, T. 2016 Internal wave attractors examined using laboratory experiments and 3d numerical simulations. J. Fluid Mech. 793, 109–131.Google Scholar
Bühler, O. & Muller, C. J. 2007 Instability and focusing of internal tides in the deep ocean. J. Fluid Mech. 588, 1–28.Google Scholar
Buijsman, M. C., Legg, S. & Klymak, J. 2012 Double-ridge internal tide interference and its effect on dissipation in Luzon Strait. J. Phys. Oceanogr. 42, 1337–1356.Google Scholar
Dale, A. C. & Inall, M. E. 2015 Tidal mixing processes amid small-scale, deep-ocean topography. Geophys. Res. Lett. 42, 484–491.Google Scholar
Dauxois, T., Joubaud, S., Odier, Ph. & Venaille, A. 2018 Instabilities of internal gravity wave beams. Ann. Rev. Fluid Mech. 50, 131–156.Google Scholar
Duran-Matute, M., Flór, J.-B., Godeferd, F. S. & Jause-Labert, C. 2013 Turbulence and columnar vortex formation through inertial-wave focusing. Phys. Rev. E 87, 041001(R).Google Scholar
Ermanyuk, E. V., Flór, J.-B. & Voisin, B. 2011 Spatial structure of first and higher harmonic internal waves from a horizontally oscillating sphere. J. Fluid Mech. 671, 364–383.Google Scholar
Ermanyuk, E. V., Shmakova, N. D. & Flór, J.-B. 2017 Internal wave focusing by a horizontally oscillating torus. J. Fluid Mech. 813, 695–715.Google Scholar
Fan, B., Kataoka, T. & Akylas, T. R. 2018 On the interaction of an internal wavepacket with its induced mean flow and the role of streaming. J. Fluid Mech. 838, R1.Google Scholar
Flandrin, P. 1998 Time–Frequency/Time–Scale Analysis. Academic Press.Google Scholar
Flynn, M. R., Onu, K. & Sutherland, B. R. 2003 Internal wave excitation by a vertically oscillating sphere. J. Fluid Mech. 494, 65–93.Google Scholar
Hurley, D. G. & Keady, G. 1997 The generation of internal waves by vibrating elliptic cylinders. Part 2. Approximate viscous solution. J. Fluid Mech. 351, 119–138.Google Scholar
Kataoka, T. & Akylas, T. R. 2016 Three-dimensional instability of internal gravity wave beams. Proc. International Symposium on Stratified Flows, 8th, 29 August–1 September, San Diego. University of California San Diego.Google Scholar
King, B., Zhang, H. P. & Swinney, H. L. 2009 Tidal flow over three-dimensional topography in a stratified fluid. Phys. Fluids 21, 116601.Google Scholar
Mercier, M. J., Garnier, N. B. & Dauxois, T. 2008 Reflection and diffraction of internal waves analyzed with the Hilbert transform. Phys. Fluids 20, 086601.Google Scholar
Miles, J. W. 1961 On the stability of heterogeneous shear flows. J. Fluid Mech. 10, 496–508.Google Scholar
Onu, K., Flynn, M. R. & Sutherland, B. R. 2003 Schlieren measurement of axisymmetric internal wave amplitudes. Exp. Fluids 35, 24–31.Google Scholar
Peliz, A., Le Cann, B. & Mohn, C. 2009 Circulation and mixing in a deep submerged crater: tore seamount. Geophys. Res. Abstr. 11, EGU2009–7567–1.Google Scholar
Shmakova, N., Ermanyuk, E. & Flór, J.-B. 2017 Generation of higher harmonic internal waves by oscillating spheroids. Phys. Rev. Fluids 2, 114801.Google Scholar
Vlasenko, V., Stashchuk, N., Inall, M. E., Porter, M. & Aleynik, D. 2016 Focusing of baroclinic tidal energy in a canyon. J. Geophys. Res. 121, 2824–2840.Google Scholar
Voisin, B. 2003 Limit states of internal wave beams. J. Fluid Mech. 496, 243–293.Google Scholar
Voisin, B., Ermanyuk, E. V. & Flór, J.-B. 2011 Internal wave generation by oscillation of a sphere, with application to internal tides. J. Fluid Mech. 666, 308–357.Google Scholar