No CrossRef data available.
A ‘Hardy–Littlewood’ approach to the norm form equation
Published online by Cambridge University Press: 24 October 2008
Extract
Suppose that K is a number field and n = [K: ℚ]. Write S∞(K) for the set of archimedean places of K, i.e. the set of all embeddings σ: K → ℂ. Suppose that 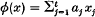 is a linear form in t variables x1, …, xt, where the aj ∈ K. This gives rise to a norm form N(x), where
is a linear form in t variables x1, …, xt, where the aj ∈ K. This gives rise to a norm form N(x), where

- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 104 , Issue 3 , November 1988 , pp. 421 - 427
- Copyright
- Copyright © Cambridge Philosophical Society 1988




