Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-01T20:32:06.595Z Has data issue: false hasContentIssue false
  • English
  • Français

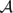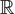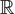Real double-points of deformations of -simple map-germs from n to 2n

Published online by Cambridge University Press:  10 April 2007

CARSTEN KLOTZ ,
OTILIA POP  and
JOACHIM H. RIEGER
CARSTEN KLOTZ
Affiliation:
Institut für Algebra und Geometrie, Universität Halle, D-06099 Halle, Germany.
OTILIA POP
Affiliation:
Institut für Algebra und Geometrie, Universität Halle, D-06099 Halle, Germany.
JOACHIM H. RIEGER
Affiliation:
Institut für Algebra und Geometrie, Universität Halle, D-06099 Halle, Germany.
Get access Rights & Permissions [Opens in a new window]

Abstract

The only stable singularities of a real map-germ 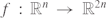are isolated transverse double-points. All 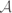-simple germs f have a deformation with the maximal number d(f) of real double-points (this is a partial generalization to higher n of the result of A'Campo [1] and Gusein-Zade [13] that all plane curve-germs have a deformation with δ real double points, with the extra hypothesis of 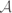-simplicity). The proof of this result is based on a classification of all 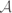-simple orbits.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 142 , Issue 2 , March 2007 , pp. 341 - 363
Copyright
Copyright © Cambridge Philosophical Society 2007

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] A'Campo, N.. Le groupe de monodromie du déploiement des singularités isolées de courbes planes. I. Math. Ann. 213 (1975), 1–32.CrossRefGoogle Scholar
[2] Arnol'd, V. I.. Simple singularities of curves. Proc. Steklov Inst. Math. 226 (1999), 20–28.Google Scholar
[3] Artin, M. and Nagata, M.. Residual intersections in Cohen-Macaulay rings. J. Math. Kyoto Univ. 12 (1972), 307–323.Google Scholar
[4] Bruce, J. W.. Classifications in singularity theory and their applications, in New Developments in Singularity Theory. Eds. Siersma, D. et al. . (Kluwer Academic Publishers, 2001), 3–33.Google Scholar
[5] Bruce, J. W. and Gaffney, T.. Simple singularities of mappings from to 2 . J. London Math. Soc. 24 (1982), 465–474.CrossRefGoogle Scholar
[6] Bruce, J. W., Plessis, A. A. du and Wall, C. T. C.. Determinacy and unipotency. Invent. Math. 88 (1987), 521–554.CrossRefGoogle Scholar
[7] Bruce, J. W., Kirk, N. and Plessis, A. A. du. Complete transversals and the classification of singularities. Nonlinearity 10 (1997), 253–275.CrossRefGoogle Scholar
[8] Cooper, T., Mond, D. and Atique, R. Wik. Vanishing topology of codimension 1 multi-germs over and . Compositio Math. 131 (2002), 121–160.CrossRefGoogle Scholar
[9] Damon, J.. The unfolding and determinacy theorems for subgroups of and . Mem. Amer. Math. Soc. 50 (1984), no. 306.Google Scholar
[10] Domitrz, W. and Rieger, J. H.. Volume-preserving diffeomorphisms on varieties and Ω-equivalence of maps. Preprint 2006.Google Scholar
[11] Gaffney, T.. 0-equivalence of maps. Math. Proc. Camb. Phil. Soc. 128 (2000), 479–496.CrossRefGoogle Scholar
[12] Gibson, C. G. and Hobbs, C. A.. Simple singularities of space curves. Math. Proc. Camb. Phil. Soc. 113 (1993), 297–310.CrossRefGoogle Scholar
[13] Gusein–Zade, S. M.. Dynkin diagrams of singularities of functions of two variables. Funct. Anal. Appl. 8 (1974), 295–300.CrossRefGoogle Scholar
[14] Houston, K.. Local topology of images of finite complex analytic maps. Topology 36 (1997), 1077–1121.CrossRefGoogle Scholar
[15] Houston, K. and Kirk, N. P.. On the classification and geometry of corank 1 map-germs from three-space to four-space. In Singularity Theory, 325–351, London Math. Soc. Lecture Note Series 263 (Cambridge University Press, 1999).CrossRefGoogle Scholar
[16] Klotz, C.. -simple germs of maps from 3 to 6 and their deformations. Diplomarbeit (MSc thesis) (Universität Halle, 2004).Google Scholar
[17] Marar, W. L. and Tari, F.. On the geometry of simple germs of corank 1 maps from 3 to 3 . Math. Proc. Camb. Phil. Soc. 119 (1996), 469–481.CrossRefGoogle Scholar
[18] Marar, W. L. and Mond, D.. Real map-germs with good perturbations. Topology 35 (1996), 157–165.CrossRefGoogle Scholar
[19] Martinet, J.. Singularities of Smooth Functions and Maps. London Math. Soc. Lecture Note Series 58 (Cambridge University Press, 1982).Google Scholar
[20] Mond, D.. On the classification of germs of maps from 2 to 3 . Proc. London Math. Soc. 50 (1985), 333–369.CrossRefGoogle Scholar
[21] Mather, J. N.. Stability of C ∞-mappings IV: classification of stable germs by -algebras. Publ. Math. Inst. Hautes Études Sci. 37 (1970), 223–248.CrossRefGoogle Scholar
[22] Rieger, J. H.. Families of maps from the plane to the plane. J. London Math. Soc. 36 (1987), 351–369.CrossRefGoogle Scholar
[23] Rieger, J. H.. Invariants of equidimensional corank-1 maps, in Geometry and Topology of Caustics – Caustics '02 (Banach Center Publ. 62, 2004), 239–248.CrossRefGoogle Scholar
[24] Rieger, J. H. and Ruas, M. A. S.. Classification of -simple germs from k n to k 2 . Compositio Math. 79 (1991), 99–108.Google Scholar
[25] Rieger, J. H. and Ruas, M. A. S.. M-deformations of -simple Σ n-p+1-germs from n to p , n≥ p . Math. Proc. Camb. Phil. Soc. 139 (2005), 333–349.CrossRefGoogle Scholar
[26] Wall, C. T. C.. Finite determinacy of smooth map-germs. Bull. London Math. Soc. 13 (1981), 481–539.CrossRefGoogle Scholar
[27] Zhitomirskii, M.. Fully simple singularities of plane and space curves. Preprint 2005.Google Scholar