Hostname: page-component-84b7d79bbc-c654p Total loading time: 0 Render date: 2024-07-26T00:34:12.345Z Has data issue: false hasContentIssue false
  • English
  • Français

Locally linear actions on 3-manifolds

Published online by Cambridge University Press:  24 October 2008

SŁawomir Kwasik  and
Kyung Bai Lee
SŁawomir Kwasik
Affiliation:
Department of Mathematics, University of Oklahoma, Norman, OK 73019, U.S.A.
Kyung Bai Lee
Affiliation:
Department of Mathematics, University of Oklahoma, Norman, OK 73019, U.S.A.
Get access Rights & Permissions [Opens in a new window]

Extract

Let a finite group G act topologically on a closed smooth manifold Mn. One of the most natural and basic questions is whether such an action can be smoothed. More precisely, let γ:G × Mn → Mn be a topological action of G on Mn. The action γ can be smoothed if there exists a smooth action 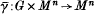and an equivariant homeomorphism 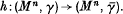It is well known that for n ≤ 2 every finite topological group action on Mn is smoothable. However already for n = 3 there are examples of topological actions on 3-manifolds which cannot be smoothed (see [1, 2] and references there). All these actions fail to be smoothable because of bad local behaviour.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 104 , Issue 2 , September 1988 , pp. 253 - 260
Copyright
Copyright © Cambridge Philosophical Society 1988

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Bing, R.. A homeomorphism between the 3-sphere and sum of the solid horned spheres. Ann. of Math. (2) 56 (1952), 354–362.Google Scholar
[2]Bing, R.. Inequivalent families of periodic homeomorphisms of E 3. Ann. of Math. (2) 80 (1964), 78–93.CrossRefGoogle Scholar
[3]Bredon, G.. Introduction to Compact Transformation Groups (Academic Press, 1972).Google Scholar
[4]Cappell, S. and Shaneson, J.. Pseudo-free actions, I. In Algebraic Topology, Aarhus 1978, Lecture Notes in Math. vol. 763 (Springer-Verlag, 1979), pp. 395–447.CrossRefGoogle Scholar
[5]Freedman, M.. The topology of four-dimensional manifolds. J. Differential Geom. 17 (1982). 357–453.Google Scholar
[6]Freedman, M.. The disk theorem for 4-dimensional manifolds. In Proc. International. Cong. Math. (Warsaw 1983) (PWN Warsaw, 1984), pp. 647–663.Google Scholar
[7]Illman, S.. Smooth equivariant triangulations of G-manifolds for G a finite group. Math. Ann. 233 (1978), 199–220.Google Scholar
[8]Kirby, R. and Siebenmann, L.. Normal bundles for codimension 2 locally flat embeddings. In Geometric Topology, Lecture Notes in Math. vol. 438 (Springer-Verlag, 1975), pp. 310–324.Google Scholar
[9]Kirby, R. and Siebenmann, L.. Foundational essays on topological manifolds. smoothings and triangulations. Ann. of Math. Stud., no. 88 (Princeton University Press, 1977).Google Scholar
[10]Kwasik, S.. On symmetries of the fake CP 2. Math. Ann. 274 (1986), 385–389.Google Scholar
[11]Kwasik, S. and Schultz, R.. Pseudofree group actions on S 4 (To appear.)Google Scholar
[12]Kwasik, S. and Vogel, P.. Asymmetric four-dimensional manifolds. Duke Math. J. 53 (1986), 759–764.Google Scholar
[13]Lashof, R. and Rothenberg, M.. G-smoothing theory. Proc. Symp. Pure Math. vol. XXXII, part 1 (American Mathematical Society, 1978), 211–266.Google Scholar
[14]Lees, A.. The surgery obstruction groups of C. T. C. Wall. Adv. in Math. 11(1973), 113–156.Google Scholar
[15]Meeks, W. III and Scott, P.. Finite group actions on 3-manifolds. Invent. Math. 86 (1986) 287–346.Google Scholar
[16]Moise, E.. Statically tame periodic homeomorphisms of compact connected 3-manifolds, II. Statically tame implies tame. Trans. Amer. Math. Soc. 259 (1980), 255–280.Google Scholar
[17]Oliver, R.. SK 1 for finite group rings: III. In Algebraic K-Theory. Evanston 1980, Lecture Notes in Math. vol. 854 (Springer-Verlag, 1981), pp. 299–337.CrossRefGoogle Scholar
[18]Raymond, F.. Classification of the actions of the circle on 3-manifolds. Trans. Amer. Math. Soc. 131 (1968), 51–78.CrossRefGoogle Scholar
[19]Rolfsen, D.. Knots and Links (Publish or Perish. 1976).Google Scholar
[20]Schultz, R.. Homotopy invariants and G-manifolds: a look at the past fifteen years. Contemp. Math. 36 (1985), 17–81.Google Scholar
[21]Wall, C. T. C.. Surgery on Compact Manifolds (Academic Press, 1970).Google Scholar
[22]Wall, C. T. C.. Classification of Hermitian forms. VI. Group rings. Ann. of Math. (2) 103 (1976), 1–80.Google Scholar