Hostname: page-component-84b7d79bbc-g7rbq Total loading time: 0 Render date: 2024-07-26T00:07:04.530Z Has data issue: false hasContentIssue false
  • English
  • Français

Multiplicative functions at consecutive integers

Published online by Cambridge University Press:  24 October 2008

Adolf Hildebrand
Adolf Hildebrand
Affiliation:
School of Mathematics, Institute for Advanced Study, Princeton, NJ 08540, U.S.A.
Get access Rights & Permissions [Opens in a new window]

Extract

Let λ(n) denote the Liouville function, i.e. λ(n) = 1 if n has an even number of prime factors, and λ(n) = − 1 otherwise. It is natural to expect that the sequence λ(n) (n ≥ 1) behaves like a random sequence of ± signs. In particular, it seems highly plausible that for any choice of εi = ± 1 (i = 0,…, k) we have

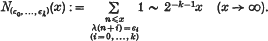
Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 100 , Issue 2 , September 1986 , pp. 229 - 236
Copyright
Copyright © Cambridge Philosophical Society 1986

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] Elliott, P. D. T. A.. Arithmetic Functions and Integer Products (Springer-Verlag, 1985).CrossRefGoogle Scholar
[2] Graham, S. W. and Hensley, D.. Problem E 3025 in Amer. Math. Monthly 90 (1983), 707.CrossRefGoogle Scholar
[3] Harman, G., Pintz, J. and Wolke, D.. A note on the Möbius and the Liouville function. Studia Sci. Math. Hungar. (in the Press).Google Scholar
[4] Hildebrand, A.. Multiplicative functions in short intervals. (Preprint.)Google Scholar
[5] Huxley, M. N.. On the difference between consecutive primes. Invent. Math. 15 (1972), 164–170.CrossRefGoogle Scholar
[6] Ramachandra, K.. Some problems of analytic number theory. Acta Arith. 31 (1976), 313–323.CrossRefGoogle Scholar
[7] Wirsing, E.. Das asymptotische Verhalten von Summen über multiplikative Funktionen II. Acta Math. Acad. Sci. Hungar. 18 (1967), 411–467.CrossRefGoogle Scholar