Hostname: page-component-848d4c4894-x5gtn Total loading time: 0 Render date: 2024-05-17T22:16:24.597Z Has data issue: false hasContentIssue false
  • English
  • Français

Harnack inequality for the nonlocal equations with general growth

Part of: Partial differential equations Representations of solutions Linear function spaces and their duals Integral, integro-differential, and pseudodifferential operators Miscellaneous topics - Partial differential equations

Published online by Cambridge University Press:  23 August 2022

Yuzhou Fang  and
Chao Zhang Open the ORCID record for Chao Zhang [Opens in a new window]
Yuzhou Fang
Affiliation:
School of Mathematics, Harbin Institute of Technology, Harbin 150001, China (18b912036@hit.edu.cn)
Chao Zhang
Affiliation:
School of Mathematics and Institute for Advanced Study in Mathematics, Harbin Institute of Technology, Harbin 150001, China (czhangmath@hit.edu.cn)
Get access Rights & Permissions [Opens in a new window]

Abstract

We consider a class of generalized nonlocal $p$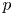-Laplacian equations. We find some proper structural conditions to establish a version of nonlocal Harnack inequalities of weak solutions to such nonlocal problems by using the expansion of positivity and energy estimates.

Keywords

Expansion of positivityfractional Orlicz–Sobolev spacegeneral growthHarnack inequalitynonlocal equations

MSC classification

Primary: 35R11: Fractional partial differential equations
Secondary: 47G20: Integro-differential operators 35B65: Smoothness and regularity of solutions 35D30: Weak solutions 46E30: Spaces of measurable functions ($L^p$-spaces, Orlicz spaces, Köthe function spaces, Lorentz spaces, rearrangement invariant spaces, ideal spaces, etc.)
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 153 , Issue 5 , October 2023 , pp. 1479 - 1502
Check for updates
Copyright
Copyright © The Author(s), 2022. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Brasco, L. and Lindgren, E.. Higher Sobolev regularity for the fractional $p$-Laplace equation in the superquadratic case. Adv. Math. 304 (2017), 300–354.10.1016/j.aim.2016.03.039CrossRefGoogle Scholar
Brasco, L., Lindgren, E. and Strömqvist, M.. Continuity of solutions to a nonlinear fractional diffusion equation. J. Evol. Equ. 21 (2021), 4319–4381.10.1007/s00028-021-00721-2CrossRefGoogle Scholar
Brasco, L. and Parini, E.. The second eigenvalue of the fractional $p$-Laplacian. Adv. Calc. Var. 9 (2016), 323–355.10.1515/acv-2015-0007CrossRefGoogle Scholar
Buryachenko, K. O. and Skrypnik, I. I.. Local continuity and Harnack's inequality for double-phase parabolic equations. Potential Anal. 56 (2022), 137–164.CrossRefGoogle Scholar
Byun, S. S., Kim, H. and Ok, J.. Local Hölder continuity for fractional nonlocal equations with general growth, eprint arXiv:2112.13958.Google Scholar
Byun, S. S., Ok, J. and Song, K.. Hölder regularity for weak solutions to nonlocal double phase problems, eprint arXiv:2108.09623.Google Scholar
Caffarelli, L., Chan, C. and Vasseur, A.. Regularity theory for parabolic nonlinear integral operators. J. Am. Math. Soc. 24 (2011), 849–869.10.1090/S0894-0347-2011-00698-XCrossRefGoogle Scholar
Chaker, J. and Kim, M.. Local regularity for nonlocal equations with variable exponents eprint, arXiv:2107.06043.Google Scholar
Chaker, J., Kim, M. and Weidner, M.. Regularity for nonlocal problems with non-standard growth, eprint arXiv:2111.09182.Google Scholar
De Filippis, C. and Mingione, G.. Nonuniformly elliptic Schauder theory, eprint arXiv:2201.07369.Google Scholar
De Filippis, C. and Palatucci, G.. Hölder regularity for nonlocal double phase equations. J. Differ. Equ. 267 (2019), 547–586.10.1016/j.jde.2019.01.017CrossRefGoogle Scholar
Di Castro, A., Kuusi, T. and Palatucci, G.. Nonlocal Harnack inequalities. J. Funct. Anal. 267 (2014), 1807–1836.10.1016/j.jfa.2014.05.023CrossRefGoogle Scholar
Di Castro, A., Kuusi, T. and Palatucci, G.. Local behavior of fractional $p$-minimizers. Ann. Inst. H. Poincaré Anal. Non Linéaire 33 (2016), 1279–1299.10.1016/j.anihpc.2015.04.003CrossRefGoogle Scholar
Diening, L., Stroffolini, B. and Verde, A.. Everywhere regularity of functionals with $\varphi$-growth. Manuscripta Math. 129 (2009), 449–481.10.1007/s00229-009-0277-0CrossRefGoogle Scholar
Ding, M., Zhang, C. and Zhou, S.. Local boundedness and Hölder continuity for the parabolic fractional $p$-Laplace equations. Calc. Var. Partial Differ. Equ. 60 (2021), 38.10.1007/s00526-020-01870-xCrossRefGoogle Scholar
Fang, Y. and Zhang, C.. On weak and viscosity solutions of nonlocal double phase equations. Int. Math. Res. Not. IMRN (2021). doi:10.1093/imrn/rnab351Google Scholar
Felsinger, M. and Kassmann, M.. Local regularity for parabolic nonlocal operators. Comm. Partial Differ. Equ. 38 (2013), 1539–1573.CrossRefGoogle Scholar
Fernández Bonder, J., Salort, A. and Vivas, H.. Interior and up to the boundary regularity for the fractional $g$-Laplacian: the convex case. Nonlinear Anal. 223 (2022), 113060.10.1016/j.na.2022.113060CrossRefGoogle Scholar
Fernández Bonder, J., Salort, A. and Vivas, H.. Global Hölder regularity for eigenfunctions of the fractional $g$-Laplacian, eprint arXiv:2112.00830.Google Scholar
Franzina, G. and Palatucci, G.. Fractional $p$-eigenvalues. Riv. Mat. Univ. Parma 5 (2014), 315–328.Google Scholar
Giacomoni, J., Kumar, D. and Sreenadh, K.. Interior and boundary regularity results for strongly nonhomogeneous $p,\, q$-fractional problems eprint arXiv:2102.06080.Google Scholar
Giacomoni, J., Kumar, D. and Sreenadh, K.. Global regularity results for nonhomogeneous growth fractional problems. J. Geom. Anal. 32 (2022), 36.10.1007/s12220-021-00837-4CrossRefGoogle Scholar
Giaquinta, M. and Giusti, E.. On the regularity of the minima of variational integrals. Acta Math. 148 (1982), 31–46.10.1007/BF02392725CrossRefGoogle Scholar
Giusti, E.. Direct Methods in the Calculus of Variations (River Edge: World Scientific Publishing Co Inc., 2003).CrossRefGoogle Scholar
Goel, D., Kumar, D. and Sreenadh, K.. Regularity and multiplicity results for fractional $(p,\, q)$-Laplacian equations. Commun. Contemp. Math. 22 (2020), 1950065.10.1142/S0219199719500652CrossRefGoogle Scholar
Harjulehto, P., Hästö, P. and Lee, M.. Hölder continuity of quasiminimizers and $\omega$-minimizers of functionals with generalized Orlicz growth. Ann. Sc. Norm. Super. Pisa Cl. Sci. 2 (2021), 549–582.Google Scholar
Iannizzotto, A., Mosconi, S. and Squassina, M.. Global Hölder regularity for the fractional $p$-Laplacian. Rev. Mat. Iberoam. 32 (2016), 1353–1392.10.4171/RMI/921CrossRefGoogle Scholar
Kassmann, M.. A new formulation of Harnack's inequality for nonlocal operators. C. R. Math. Acad. Sci. Paris 349 (2011), 637–640.CrossRefGoogle Scholar
Kinnunen, J. and Shanmugalingam, N.. Regularity of quasi-minimizers of metric spaces. Manuscripta Math. 105 (2001), 401–423.CrossRefGoogle Scholar
Korvenpää, J., Kuusi, T. and Lindgren, E.. Equivalence of solutions to fractional $p$-Laplace type equations. J. Math. Pures Appl. 132 (2019), 1–26.10.1016/j.matpur.2017.10.004CrossRefGoogle Scholar
Korvenpää, J., Kuusi, T. and Palatucci, G.. The obstacle problem for nonlinear integro-differential operators. Calc. Var. Partial Differ. Equ. 55 (2016), 63.CrossRefGoogle Scholar
Korvenpää, J., Kuusi, T. and Palatucci, G.. Fractional superharmonic functions and the Perron method for nonlinear integro-differential equations. Math. Ann. 369 (2017), 1443–1489.10.1007/s00208-016-1495-xCrossRefGoogle Scholar
Kuusi, T., Mingione, G. and Sire, Y.. Nonlocal self-improving properties. Anal. PDE 8 (2015), 57–114.CrossRefGoogle Scholar
Kuusi, T., Mingione, G. and Sire, Y.. Nonlocal equations with measure data. Commun. Math. Phys. 337 (2015), 1317–1368.CrossRefGoogle Scholar
Marcellini, P.. Regularity of minimizers of integrals of the calculus of variations with non standard growth conditions. Arch. Ration. Mech. Anal. 105 (1989), 267–284.CrossRefGoogle Scholar
Marcellini, P.. Regularity and existence of solutions of elliptic equations with $p,\, q$-growth conditions. J. Differ. Equ. 90 (1991), 1–30.10.1016/0022-0396(91)90158-6CrossRefGoogle Scholar
Mazón, J. M., Rossi, J. D. and Toledo, J.. Fractional $p$-Laplacian evolution equations. J. Math. Pures Appl. 105 (2016), 810–844.CrossRefGoogle Scholar
Mihăilescu, M. and Rădulescu, V.. Neumann problems associated to nonhomogeneous differential operators in Orlicz-Sobolev spaces. Ann. Inst. Fourier (Grenoble) 58 (2008), 2087–2111.CrossRefGoogle Scholar
Ok, J., Local Hölder regularity for nonlocal equations with variable powers, eprint arXiv:2107.06611.Google Scholar
Palatucci, G.. The Dirichlet problem for the fractional $p$-Laplace equation. Nonlinear Anal. 177 (2018), 699–732.10.1016/j.na.2018.05.004CrossRefGoogle Scholar
Salort, A.. Lower bounds for Orlicz eigenvalues. Discrete Contin. Dyn. Syst. 42 (2022), 1415–1434.CrossRefGoogle Scholar
Salort, A.. Eigenvalues and minimizers for a non-standard growth non-local operator. J. Differ. Equ. 268 (2020), 5413–5439.10.1016/j.jde.2019.11.027CrossRefGoogle Scholar
Salort, A. and Vivas, H.. Fractional eigenvalues in Orlicz spaces with no $\Delta _2$ condition. J. Differ. Equ. 327 (2022), 166–188.10.1016/j.jde.2022.04.029CrossRefGoogle Scholar
Skrypnik, I. I. and Voitovych, M. V.. On the continuity of solutions of quasilinear parabolic equations with generalized Orlicz growth under non-logarithmic conditions. Ann. Mat. Pura Appl. 201 (2022), 1381–1416.10.1007/s10231-021-01161-yCrossRefGoogle Scholar
Strömqvist, M.. Local boundedness of solutions to nonlocal parabolic equations modeled on the fractional $p$-Laplacian. J. Differ. Equ. 266 (2019), 7948–7979.10.1016/j.jde.2018.12.021CrossRefGoogle Scholar
Vázquez, J. L.. The Dirichlet problem for the fractional $p$-Laplacian evolution equation. J. Differ. Equ. 260 (2016), 6038–6056.10.1016/j.jde.2015.12.033CrossRefGoogle Scholar
Vázquez, J. L.. The fractional $p$-Laplacian evolution equation in $\mathbb {R}^{N}$ in the sublinear case. Calc. Var. Partial Differ. Eq. 60 (2021), 140.10.1007/s00526-021-02005-6CrossRefGoogle Scholar