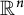1 Introduction
Global attractors hold a pivotal position in the realm of differential equations due to their prevalence in various significant equations, as emphasized in [Reference Robinson16]. They often play the role of stabilizing factors, demonstrating resilience even in the face of minor perturbations, as gauged through the Hausdorff distance [Reference Raugel14, Reference Robinson16]. Nevertheless, this stability is not an inherent trait across the board. A case in point is the ordinary differential equation (ODE) depicted in Chapter 3 of [Reference Raugel14], expressed as
Within this ODE, the interval
![]() $[0,1]$
stands as a global attractor. However, when subjected to perturbation, as illustrated by the modified equation:
$[0,1]$
stands as a global attractor. However, when subjected to perturbation, as illustrated by the modified equation:
the global attractor dwindles to the singleton set
![]() $\{1\}$
.
$\{1\}$
.
Another compelling illustration lies in the homeomorphism
![]() $g:\mathbb {R}\to \mathbb {R}$
, defined as
$g:\mathbb {R}\to \mathbb {R}$
, defined as
This homeomorphism features the global repelling fixed point at
![]() $0$
. Consequently, its inverse,
$0$
. Consequently, its inverse,
![]() $f=g^{-1}:\mathbb {R}\to \mathbb {R}$
, boasts
$f=g^{-1}:\mathbb {R}\to \mathbb {R}$
, boasts
![]() $\{0\}$
as a global attractor. However, a nuanced perspective emerges when considering
$\{0\}$
as a global attractor. However, a nuanced perspective emerges when considering
![]() $f(0) = 0$
and the limit as x tends to infinity:
$f(0) = 0$
and the limit as x tends to infinity:
![]() $\lim _{x\to \infty }(f(x)-x) = 0$
. This revelation opens the door for tailored perturbations, where by selecting a sufficiently large positive value, denoted as M, a new fixed point emerges at
$\lim _{x\to \infty }(f(x)-x) = 0$
. This revelation opens the door for tailored perturbations, where by selecting a sufficiently large positive value, denoted as M, a new fixed point emerges at
![]() $x=M$
, with the interval
$x=M$
, with the interval
![]() $[0, M]$
assuming the role of the global attractor.
$[0, M]$
assuming the role of the global attractor.
These intriguing phenomena are often characterized as “implosions” (as seen in the example (1.1) and its perturbation) and “explosions” (as witnessed in example (1.2) and its perturbation). Notably, explosions have been the subject of in-depth scrutiny with regard to both the nonwandering set and the chain recurrent set, as elucidated in [Reference Shub and Smale19] and [Reference Block and Franke4], respectively. Recent research endeavors have delved into explosions concerning the generalized recurrent set, bearing relevance to the field of Lyapunov functions. These investigations and their broader implications are well-documented in [Reference Bernardi, Florio and Wiseman3] and the referenced literature therein.
These examples motivate the search of necessary and sufficient conditions for the stability of the global attractor under small perturbations. The contemporary paradigm of stability in dynamical systems is anchored in the realm of topology, a concept that has evolved to address the fundamental aspects of system behavior. This innovative perspective on stability was first introduced by Walters, as documented in his seminal work [Reference Walters23], with the intent of assuming the role traditionally held by structural stability in topological dynamics [Reference Andromov and Pontrjagin1]. It is worth noting that a many dynamical systems, especially those inhabiting compact manifolds such as Morse-Smale and Anosov diffeomorphisms, or the broader class of Axiom A diffeomorphisms that adhere to the strong transversality condition, are inherently topologically stable [Reference Nitecki13]. Moreover, an interesting observation in the realm of expansive homeomorphisms within compact metric spaces is that they also exhibit topological stability, a phenomenon commonly referred to as the “Walters stability theorem” [Reference Walters22]. The pursuit of extending this remarkable theorem to the challenging noncompact domain has spurred endeavors in multiple directions. Researchers have embarked on ambitious endeavors to bridge the gap between compact and noncompact settings, with notable contributions documented in a body of literature that includes works such as [Reference Cousillas, Groisman and Xavier5, Reference Lee, Nguyen and Yang11, Reference Lee and Rojas12, Reference Sears18]. These exploratory undertakings strive to generalize the Walters stability theorem and unearth the underlying principles governing the stability of dynamical systems in an increasingly diverse and complex landscape.
Within the scope of this paper, we explore the intricate domain of global attractor stability in the presence of slight perturbations. To begin, we establish that when a homeomorphism possesses a global attractor in the Euclidean space
![]() $\mathbb {R}^n$
and exhibits topological stability, the global attractor remains steadfast in the face of these perturbations. This resilience extends even further, as we observe that the homeomorphism, in this context, is not only globally stable but also retains its topological stability specifically on its global attractor. However, it is crucial to note that the converse of this assertion does not hold, as we shall elucidate. In a distinct line of inquiry, when a homeomorphism boasts a global attractor within the realm of a locally compact metric space and also exhibits expansiveness coupled with the shadowing property, it invariably qualifies as topologically stable. Our research endeavors culminate in a precise statement of these results, underlining the intricate interplay between global attractors, topological stability, expansiveness, and shadowing properties.
$\mathbb {R}^n$
and exhibits topological stability, the global attractor remains steadfast in the face of these perturbations. This resilience extends even further, as we observe that the homeomorphism, in this context, is not only globally stable but also retains its topological stability specifically on its global attractor. However, it is crucial to note that the converse of this assertion does not hold, as we shall elucidate. In a distinct line of inquiry, when a homeomorphism boasts a global attractor within the realm of a locally compact metric space and also exhibits expansiveness coupled with the shadowing property, it invariably qualifies as topologically stable. Our research endeavors culminate in a precise statement of these results, underlining the intricate interplay between global attractors, topological stability, expansiveness, and shadowing properties.
Consider a metric space X. Denote by
![]() $\mathrm{id}_X$
the identity map of X. Define the
$\mathrm{id}_X$
the identity map of X. Define the
![]() $C^0$
-distance between maps
$C^0$
-distance between maps
![]() $l,r:X\to X$
by
$l,r:X\to X$
by
This distance satisfies all requirements of a metric except that it may take infinite value. It is then referred to as an
![]() $\infty $
-metric (p. 1014 in [Reference Dydak and Hoffland6]).
$\infty $
-metric (p. 1014 in [Reference Dydak and Hoffland6]).
The following is the classical definition of topologically stable homeomorphisms [Reference Walters22].
Definition 1.1 A homeomorphism of a metric space
![]() $f:X\to X$
is topologically stable if, for every
$f:X\to X$
is topologically stable if, for every
![]() $\varepsilon>0$
, there is
$\varepsilon>0$
, there is
![]() $\delta>0$
such that for any homeomorphism
$\delta>0$
such that for any homeomorphism
![]() $g:X\to X$
with
$g:X\to X$
with
![]() $d_{C^0}(f,g)\leq \delta $
, there is a continuous
$d_{C^0}(f,g)\leq \delta $
, there is a continuous
![]() $h:X\to X$
such that
$h:X\to X$
such that
![]() $d_{C^0}(h,\mathrm{id}_X)\leq \varepsilon $
and
$d_{C^0}(h,\mathrm{id}_X)\leq \varepsilon $
and
![]() ${f\circ h=h\circ g}$
.
${f\circ h=h\circ g}$
.
The second main definition is the following one. Following [Reference Robinson16], given subsets of a metric space
![]() $A,B\subset X$
we define
$A,B\subset X$
we define
Now, we remind the following the standard definition [Reference Robinson16].
Definition 1.2 A global attractor of a continuous map
![]() $f:X\to X$
is a nonempty compact subset
$f:X\to X$
is a nonempty compact subset
![]() $\mathbb {A}\subset X$
which is invariant (i.e.,
$\mathbb {A}\subset X$
which is invariant (i.e.,
![]() $f(\mathbb {A})=\mathbb {A}$
) and attracts bounded sets namely
$f(\mathbb {A})=\mathbb {A}$
) and attracts bounded sets namely
It is natural to compare this definition with the concept of Conley attractor: a compact invariant set
![]() $\mathcal {A}\subset X$
exhibiting a closed neighborhood U such that
$\mathcal {A}\subset X$
exhibiting a closed neighborhood U such that
It is imperative to establish a clear distinction between global attractors and Conley attractors, as they occupy distinct positions in the realm of dynamical systems. It is evident that every global attractor automatically qualifies as a Conley attractor, given their inherent relationship. However, the inverse does not hold, primarily due to the semilocal nature of Conley attractors. See, for instance, the homeomorphisms in Figure 1 where the points p and q are Conley attractors, and the segment from p to q passing through the saddle point s is the global attractor. Notice that this homeomorphism is topologically stable.

Figure 1 Comparing the global and Conley’s attractors.
In stark contrast, the global attractor, if it exists, is a unique entity that comprehensively encompasses all Conley attractors within its domain. This uniqueness is easily demonstrated through a straightforward argument: Should there be another candidate global attractor denoted as
![]() $\mathbb {A}'$
, the distance between
$\mathbb {A}'$
, the distance between
![]() $\mathbb {A}'$
and the original global attractor
$\mathbb {A}'$
and the original global attractor
![]() $\mathbb {A}$
is shown to approach zero, as expressed by
$\mathbb {A}$
is shown to approach zero, as expressed by
![]() $\mathrm{dist}(f^i(\mathbb {A}'),\mathbb {A})\to 0$
. Consequently, this leads to the conclusion that
$\mathrm{dist}(f^i(\mathbb {A}'),\mathbb {A})\to 0$
. Consequently, this leads to the conclusion that
![]() $\mathrm{dist}(\mathbb {A}',\mathbb {A})$
is also zero, implying that
$\mathrm{dist}(\mathbb {A}',\mathbb {A})$
is also zero, implying that
![]() $\mathbb {A}'$
is a subset of
$\mathbb {A}'$
is a subset of
![]() $\mathbb {A}$
. By virtue of symmetry, the roles of
$\mathbb {A}$
. By virtue of symmetry, the roles of
![]() $\mathbb {A}$
and
$\mathbb {A}$
and
![]() $\mathbb {A}'$
can be reversed, leading to the conclusion that
$\mathbb {A}'$
can be reversed, leading to the conclusion that
![]() $\mathbb {A}$
and
$\mathbb {A}$
and
![]() $\mathbb {A}'$
are, in fact, one and the same:
$\mathbb {A}'$
are, in fact, one and the same:
![]() $\mathbb {A} = \mathbb {A}'$
.
$\mathbb {A} = \mathbb {A}'$
.
In the context of homeomorphisms with global attractors, it is essential to note that they encompass all homeomorphisms of compact metric spaces, denoted as
![]() $f:X\to X$
, given that the entire space X inherently satisfies the prerequisites specified in the corresponding definition of a global attractor, namely, it is compact, exhibits invariance, and effectively attracts all bounded sets. However, it is crucial to underscore that many such homeomorphisms do not exhibit Conley attractors, with the identity transformation serving as a prime example.
$f:X\to X$
, given that the entire space X inherently satisfies the prerequisites specified in the corresponding definition of a global attractor, namely, it is compact, exhibits invariance, and effectively attracts all bounded sets. However, it is crucial to underscore that many such homeomorphisms do not exhibit Conley attractors, with the identity transformation serving as a prime example.
The realm of homeomorphisms with global attractors extends to a diverse array of scenarios, including contractions on complete metric spaces, the time-
![]() $1$
map of the equation (1.1), the inverse of the homeomorphism (1.2), the Lorenz equation, solenoid diffeomorphisms within
$1$
map of the equation (1.1), the inverse of the homeomorphism (1.2), the Lorenz equation, solenoid diffeomorphisms within
![]() $\mathbb {R}^3$
, the illustrative example presented in (1.3), and an array of other instances. In the case of contractions, the global attractor is given by the Banach contracting principle whereas the solenoid is the well-known example of a chaotic hyperbolic attractor suggested by Smale in his seminal paper [Reference Smale20]. For a more detailed exploration of the dynamics and characteristics of such homeomorphisms, one can refer to the extensive insights offered in [Reference Robinson16] or consult the comprehensive analysis in [Reference Robinson and Sánchez-Gabites17].
$\mathbb {R}^3$
, the illustrative example presented in (1.3), and an array of other instances. In the case of contractions, the global attractor is given by the Banach contracting principle whereas the solenoid is the well-known example of a chaotic hyperbolic attractor suggested by Smale in his seminal paper [Reference Smale20]. For a more detailed exploration of the dynamics and characteristics of such homeomorphisms, one can refer to the extensive insights offered in [Reference Robinson16] or consult the comprehensive analysis in [Reference Robinson and Sánchez-Gabites17].
Given a metric space X, we define the Hausdorff distance between subsets
![]() $A,B\subset X$
by
$A,B\subset X$
by
The following alternate notion of stability is related to the A-stability (p. 25 in [Reference Hale8]) or Definition 1.1 (p. 4 in [Reference Lee and Nguyen10]). We denote
![]() $i_A:A\to X$
the inclusion map.
$i_A:A\to X$
the inclusion map.
Definition 1.3 A homeomorphism with global attractor of a metric space
![]() $f:X\to X$
is topologically stable on its global attractor
$f:X\to X$
is topologically stable on its global attractor
![]() $\mathbb {A}$
if, for every
$\mathbb {A}$
if, for every
![]() $\epsilon>0,$
there is
$\epsilon>0,$
there is
![]() $\delta>0$
such that for any homeomorphism
$\delta>0$
such that for any homeomorphism
![]() $g:X\to X$
with
$g:X\to X$
with
![]() $d_{C^0}(f,g)\leq \delta $
, there are
$d_{C^0}(f,g)\leq \delta $
, there are
![]() $\mathbb {A}_g\subset X$
compact and a continuous map
$\mathbb {A}_g\subset X$
compact and a continuous map
![]() $h_g:\mathbb {A}_g\to \mathbb {A}$
(often called topological semiconjugacy) such that:
$h_g:\mathbb {A}_g\to \mathbb {A}$
(often called topological semiconjugacy) such that:
-
•
 $\mathbb {A}_g$
is a global attractor of g;
$\mathbb {A}_g$
is a global attractor of g; -
•
 $D(\mathbb {A},\mathbb {A}_g)<\epsilon $
;
$D(\mathbb {A},\mathbb {A}_g)<\epsilon $
; -
•
 $d_{C^0}(h_g,i_{\mathbb {A}_g})<\epsilon $
and
$d_{C^0}(h_g,i_{\mathbb {A}_g})<\epsilon $
and
 $f|_{\mathbb {A}}\circ h_g=h_g\circ g|_{\mathbb {A}_g}$
.
$f|_{\mathbb {A}}\circ h_g=h_g\circ g|_{\mathbb {A}_g}$
.
The Hausdorff distance
![]() $D(\mathbb {A},\mathbb {A}_g)$
in the above definition is used to quantify the extent of implosion or explosion experienced by the global attractor when subjected to minor perturbations (this is not possible with
$D(\mathbb {A},\mathbb {A}_g)$
in the above definition is used to quantify the extent of implosion or explosion experienced by the global attractor when subjected to minor perturbations (this is not possible with
![]() $\mathrm{dist}(\mathbb {A},\mathbb {A}_g)$
only). This measure provides a crucial gauge for assessing the dynamic response of the global attractor in the face of external influences, enabling us to discern the degree of stability or vulnerability exhibited in the system.
$\mathrm{dist}(\mathbb {A},\mathbb {A}_g)$
only). This measure provides a crucial gauge for assessing the dynamic response of the global attractor in the face of external influences, enabling us to discern the degree of stability or vulnerability exhibited in the system.
On the other hand, not every homeomorphism with a global attractor is topologically stable on its global attractor. A simple counterexample is the time one map of (1.1).
With these definitions, we can state the following result.
Theorem 1.4 Every topologically stable homeomorphism with global attractor of an Euclidean space
![]() $\mathbb {R}^n$
is topologically stable on its global attractor.
$\mathbb {R}^n$
is topologically stable on its global attractor.
We do not know whether, conversely, the topological stability on the global attractor implies the topological stability of the homeomorphism. At first glance, we could believe that a counterexample is the inverse
![]() $f:\mathbb {R}\to \mathbb {R}$
of the homeomorphism
$f:\mathbb {R}\to \mathbb {R}$
of the homeomorphism
![]() $g:\mathbb {R}\to \mathbb {R}$
described in (1.3). However, this f is not topologically stable on its global attractor
$g:\mathbb {R}\to \mathbb {R}$
described in (1.3). However, this f is not topologically stable on its global attractor
![]() $\{0\}$
, because, arbitrarily small perturbation of f may have
$\{0\}$
, because, arbitrarily small perturbation of f may have
![]() $[0,M]$
as a global attractor for some
$[0,M]$
as a global attractor for some
![]() $M>1$
and then
$M>1$
and then
![]() $D({0}, [0, M ]) = M> 1$
. We thank the anonymous referee who pointed this out to us.
$D({0}, [0, M ]) = M> 1$
. We thank the anonymous referee who pointed this out to us.
An anonymous referee raised an inquiry regarding the potential extension of the theorem presented above from
![]() $\mathbb {R}^n$
to noncompact manifolds. It appears that the affirmative holds, and we intend to explore this matter in our upcoming investigations.
$\mathbb {R}^n$
to noncompact manifolds. It appears that the affirmative holds, and we intend to explore this matter in our upcoming investigations.
Next, we give sufficient conditions for the topological stability of a given homeomorphism with global attractor. Recall that a homeomorphism
![]() $f:X\to X$
is expansive if there is
$f:X\to X$
is expansive if there is
![]() $e>0$
such that if
$e>0$
such that if
![]() $x,y\in X$
and
$x,y\in X$
and
![]() $d(f^n(x),f^n(y))\leq e$
for every
$d(f^n(x),f^n(y))\leq e$
for every
![]() $n\in \mathbb {Z}$
, then
$n\in \mathbb {Z}$
, then
![]() $x=y$
. This concept is due to Utz [Reference Utz21]. Given
$x=y$
. This concept is due to Utz [Reference Utz21]. Given
![]() $\delta>0$
, a bi-infinite sequence
$\delta>0$
, a bi-infinite sequence
![]() $(x_i)_{i\in \mathbb {Z}}$
is called
$(x_i)_{i\in \mathbb {Z}}$
is called
![]() $\delta $
-pseudo orbit if
$\delta $
-pseudo orbit if
![]() $d(f(x_i),x_{i+1})\leq \delta $
for all
$d(f(x_i),x_{i+1})\leq \delta $
for all
![]() $i\in \mathbb {Z}$
. We say that the sequence can be
$i\in \mathbb {Z}$
. We say that the sequence can be
![]() $\delta $
-shadowed if there is
$\delta $
-shadowed if there is
![]() $x\in X$
such that
$x\in X$
such that
![]() $d(f^i(x),x_i)\leq \delta $
for every
$d(f^i(x),x_i)\leq \delta $
for every
![]() $i\in \mathbb {Z}$
. A homeomorphism
$i\in \mathbb {Z}$
. A homeomorphism
![]() $f:X\to X$
has the shadowing property if, for every
$f:X\to X$
has the shadowing property if, for every
![]() $\epsilon>0$
, there is
$\epsilon>0$
, there is
![]() $\delta>0$
such that every
$\delta>0$
such that every
![]() $\delta $
-pseudo orbit can be
$\delta $
-pseudo orbit can be
![]() $\epsilon $
-shadowed.
$\epsilon $
-shadowed.
The previously mentioned Walters stability theorem (Theorem 4, p. 236 in [Reference Walters22]) asserts that every expansive homeomorphism with the shadowing property of a compact metric space is topologically stable. We would like to extend this theorem to homeomorphisms with global attractors on metric spaces. To do that we need an extra hypothesis on the space. Recall that a metric space is locally compact if every point has a compact neighborhood.
Theorem 1.5 Let
![]() $f:X\to X$
be a homeomorphism with global attractor of a locally compact metric space. If f is expansive and has the shadowing property, then f is topologically stable.
$f:X\to X$
be a homeomorphism with global attractor of a locally compact metric space. If f is expansive and has the shadowing property, then f is topologically stable.
This result can be applied to Examples 2.3 and 2.5. The paper is organized as follows: In Section 2, we give some preparatory lemmas. In Section 3, we use these lemmas to prove the theorems.
2 Preliminary lemmas
To prove Theorem 1.4, we will use the following lemma. It was extracted from Theorem 1.8 (p. 39 in [Reference Hirsch9]; and seemed well known). Denote by
![]() $B[x,r]$
, the closed r-ball centered at x.
$B[x,r]$
, the closed r-ball centered at x.
Lemma 2.1 For every
![]() $n\in \mathbb {N}$
and every compact
$n\in \mathbb {N}$
and every compact
![]() $\mathbb {A}\subset \mathbb {R}^n$
, there is
$\mathbb {A}\subset \mathbb {R}^n$
, there is
![]() $\rho>0$
such that if
$\rho>0$
such that if
![]() $h:\mathbb {R}^n\to \mathbb {R}^n$
is continuous and
$h:\mathbb {R}^n\to \mathbb {R}^n$
is continuous and
![]() $d_{C^0}(h,\mathrm{id}_{\mathbb {R}^n})<\rho $
, then
$d_{C^0}(h,\mathrm{id}_{\mathbb {R}^n})<\rho $
, then
![]() $\mathbb {A}\subset h(\mathbb {R}^n)$
.
$\mathbb {A}\subset h(\mathbb {R}^n)$
.
Proof We can assume that
![]() $\mathbb {A}= B[0,\gamma ]$
for some
$\mathbb {A}= B[0,\gamma ]$
for some
![]() $\gamma>0$
. Denote
$\gamma>0$
. Denote
![]() $B=B[0,2\gamma ]$
and
$B=B[0,2\gamma ]$
and
![]() $\partial B$
the boundary of B. Choose
$\partial B$
the boundary of B. Choose
![]() $\rho>0$
small enough such that for every
$\rho>0$
small enough such that for every
![]() $z\in B[0,\gamma ]$
, every
$z\in B[0,\gamma ]$
, every
![]() $x\in \partial B$
and
$x\in \partial B$
and
![]() $y\in \mathbb {R}^n$
with
$y\in \mathbb {R}^n$
with
![]() $\|x-y\|<\rho $
it is true that the line traced from z to y intersects
$\|x-y\|<\rho $
it is true that the line traced from z to y intersects
![]() $\partial B$
at some point u with
$\partial B$
at some point u with
![]() $\|u-x\|<4\gamma $
. Suppose by contradiction that there is
$\|u-x\|<4\gamma $
. Suppose by contradiction that there is
![]() $h:\mathbb {R}^n\to \mathbb {R}^n$
continuous such that
$h:\mathbb {R}^n\to \mathbb {R}^n$
continuous such that
Choose
![]() $z\in B[0,\gamma ]\setminus h(\mathbb {R}^n)$
. Define
$z\in B[0,\gamma ]\setminus h(\mathbb {R}^n)$
. Define
![]() $H:B\to \partial B$
by
$H:B\to \partial B$
by
![]() $H(x)=u$
, where u is as above with
$H(x)=u$
, where u is as above with
![]() $y=h(x)$
. Then, H is continuous and because
$y=h(x)$
. Then, H is continuous and because
![]() $\|h(x)-x\|<4\gamma $
(
$\|h(x)-x\|<4\gamma $
(
![]() $\forall x\in \partial B$
) we also have
$\forall x\in \partial B$
) we also have
![]() $H(x)\neq -x$
for every
$H(x)\neq -x$
for every
![]() $x\in \partial B$
. From this, we can construct a homotopy from
$x\in \partial B$
. From this, we can construct a homotopy from
![]() $H|_{\partial B}:\partial B\to \partial B$
to
$H|_{\partial B}:\partial B\to \partial B$
to
![]() $\mathrm{id}_{\partial B}$
through the minimal circle arc in
$\mathrm{id}_{\partial B}$
through the minimal circle arc in
![]() $\partial B$
from
$\partial B$
from
![]() $H(x)$
to x. This would imply that
$H(x)$
to x. This would imply that
![]() $\partial B$
is a retract of B which is impossible by the Brouwer fixed point theorem.
$\partial B$
is a retract of B which is impossible by the Brouwer fixed point theorem.
To prove Theorem 1.5, we need two lemmas. The first is a generalization of Walters’s stability theorem to certain metric spaces including the compact ones. To motivate the definition, we recall that a metric space X is proper when every closed ball of X is compact (see Gromov [Reference Gromov7]). Every compact metric space is proper but not conversely. Noncompact examples are the closed subset of the Euclidean space
![]() $\mathbb {R}^n$
, image of those sets under bi-Lipschitz maps, complete Riemannian manifolds, complete Finsler manifolds, complete sub-Riemannian or sub-Finsler manifolds or finite products of these spaces.
$\mathbb {R}^n$
, image of those sets under bi-Lipschitz maps, complete Riemannian manifolds, complete Finsler manifolds, complete sub-Riemannian or sub-Finsler manifolds or finite products of these spaces.
Actually, we will consider a little more general metric spaces defined as follows. A metric space X is uniformly locally compact if there is
![]() $0<\beta <\infty $
such that every closed
$0<\beta <\infty $
such that every closed
![]() $\beta $
-ball of X is compact. These spaces were first considered by A. Weil in his study of uniform spaces [Reference Weil24].
$\beta $
-ball of X is compact. These spaces were first considered by A. Weil in his study of uniform spaces [Reference Weil24].
Lemma 2.2 Every expansive homeomorphism with the shadowing property of a uniformly locally compact metric space is topologically stable.
Proof Let
![]() $f:X\to X$
be an expansive homeomorphism with the shadowing property of a uniformly locally compact metric space X. Let
$f:X\to X$
be an expansive homeomorphism with the shadowing property of a uniformly locally compact metric space X. Let
![]() $\beta $
be the positive number given by the uniform local compactness of X. Let e be an expansivity constant of f. Fix
$\beta $
be the positive number given by the uniform local compactness of X. Let e be an expansivity constant of f. Fix
![]() $\epsilon>0$
,
$\epsilon>0$
,
![]() $0<\epsilon '<\frac {1}{8}\min \{\epsilon ,e,\beta \}$
and take
$0<\epsilon '<\frac {1}{8}\min \{\epsilon ,e,\beta \}$
and take
![]() $\delta>0$
from the shadowing property of f for this
$\delta>0$
from the shadowing property of f for this
![]() $\epsilon '$
.
$\epsilon '$
.
Let
![]() $g:X\to X$
be a homeomorphism with
$g:X\to X$
be a homeomorphism with
![]() $d_{C^0}(f,g)<\delta $
. Given
$d_{C^0}(f,g)<\delta $
. Given
![]() $x\in X$
, we have that
$x\in X$
, we have that
Then,
![]() $\{g^i(x)\}_{i\in \mathbb {Z}}$
is a
$\{g^i(x)\}_{i\in \mathbb {Z}}$
is a
![]() $\delta $
-pseudo orbit and so, by the shadowing property, there is
$\delta $
-pseudo orbit and so, by the shadowing property, there is
![]() $y\in X$
such that
$y\in X$
such that
We have that there is only one y satisfying the above inequalities (for the given x). Indeed, if there were another
![]() $y'\in X$
satisfying
$y'\in X$
satisfying
we would have
Since e is an expansivity constant,
![]() $y=y'$
proving the assertion.
$y=y'$
proving the assertion.
Then, by making
![]() $y=h(x)$
, we obtain a map
$y=h(x)$
, we obtain a map
![]() $h:X\to X$
satisfying
$h:X\to X$
satisfying
By replacing
![]() $i=0$
above, we obtain
$i=0$
above, we obtain
![]() $d(h(x),x)\leq \epsilon '<\epsilon $
for all
$d(h(x),x)\leq \epsilon '<\epsilon $
for all
![]() $x\in X$
, hence
$x\in X$
, hence
Also, by replacing i by
![]() $i+1$
and x by
$i+1$
and x by
![]() $g(x)$
in (2.1), we obtain, respectively, that
$g(x)$
in (2.1), we obtain, respectively, that
![]() $\forall i\in \mathbb {Z}$
. Then, the triangle inequality implies
$\forall i\in \mathbb {Z}$
. Then, the triangle inequality implies
Since e is an expansivity constant, we conclude that
![]() $f(h(x))=h(g(x))$
for all
$f(h(x))=h(g(x))$
for all
![]() $x\in X$
proving
$x\in X$
proving
It remains to prove that h is continuous. The argument is similar to one used in [Reference Artigue2]. By contradiction, suppose that it is not. Then, there is a convergent sequence
![]() $x_n\to x$
such that
$x_n\to x$
such that
![]() $h(x_n)\not \to h(x)$
as
$h(x_n)\not \to h(x)$
as
![]() $n\to \infty $
. Then, up to passing to a subsequence if necessary, we can assume that there is
$n\to \infty $
. Then, up to passing to a subsequence if necessary, we can assume that there is
![]() $\Delta>0$
such that
$\Delta>0$
such that
Next, since
![]() $x_n\to x$
, up to discarding some finite set of this sequence, we can assume
$x_n\to x$
, up to discarding some finite set of this sequence, we can assume
Since
 $$ \begin{align*} d(h(x_n),h(x))& \leq d(h(x_n),x_n)+d(x_n,x)+d(h(x),x)\\ & \overset{(2.2)}{\leq} 2\epsilon'+\sup_{n\in \mathbb{N}}d(x_n,x)\\ & < \frac{\beta}{4}+\frac{\beta}4= \frac{\beta}2, \quad\quad\forall n\in\mathbb{N}, \end{align*} $$
$$ \begin{align*} d(h(x_n),h(x))& \leq d(h(x_n),x_n)+d(x_n,x)+d(h(x),x)\\ & \overset{(2.2)}{\leq} 2\epsilon'+\sup_{n\in \mathbb{N}}d(x_n,x)\\ & < \frac{\beta}{4}+\frac{\beta}4= \frac{\beta}2, \quad\quad\forall n\in\mathbb{N}, \end{align*} $$
we conclude that
![]() $h(x_n)$
is contained in the closed
$h(x_n)$
is contained in the closed
![]() $\beta $
-ball centered at
$\beta $
-ball centered at
![]() $h(x)$
for every
$h(x)$
for every
![]() ${n\in \mathbb {N}}.$
From this, we have that
${n\in \mathbb {N}}.$
From this, we have that
![]() $\{h(x_n)\}_{n\in \mathbb {N}}$
has a convergent subsequence. For simplicity, we assume that the sequence itself is convergent, namely
$\{h(x_n)\}_{n\in \mathbb {N}}$
has a convergent subsequence. For simplicity, we assume that the sequence itself is convergent, namely
![]() $h(x_n)\to z$
for some
$h(x_n)\to z$
for some
![]() $z\in X$
.
$z\in X$
.
Now, (2.1) implies
Then, since f and g are continuous, by fixing i and letting
![]() $n\to \infty $
above, we obtain
$n\to \infty $
above, we obtain
By (2.1) once more, we have
hence
Since e is an expansivity constant, we obtain
![]() $h(x)=z$
. However, by letting
$h(x)=z$
. However, by letting
![]() $n\to \infty $
in (2.3), we get
$n\to \infty $
in (2.3), we get
![]() $d(z,h(x))\geq \Delta>0$
that’s absurd. This contradiction proves that h is continuous and finishes the proof.
$d(z,h(x))\geq \Delta>0$
that’s absurd. This contradiction proves that h is continuous and finishes the proof.
This lemma can be applied to the following examples.
Example 2.3 Any expansive homeomorphism with the shadowing property of a compact metric space (more precisely, under the conditions of Walters stability theorem).
Example 2.4 Any hyperbolic linear homeomorphism of
![]() $\mathbb {R}^n$
(or any other finite dimensional Banach space).
$\mathbb {R}^n$
(or any other finite dimensional Banach space).
In particular, all such operators are topologically stable (an outline of the proof of this fact was done by Robbin [Reference Robbin15]).
Example 2.5 The family of homeomorphisms
![]() $f:\mathbb {R}\to \mathbb {R}$
defined by
$f:\mathbb {R}\to \mathbb {R}$
defined by
![]() $f(x)=x+x^{3n}$
,
$f(x)=x+x^{3n}$
,
![]() $\forall x\in \mathbb {R}$
and
$\forall x\in \mathbb {R}$
and
![]() $n\in \mathbb {N}$
.
$n\in \mathbb {N}$
.
The examples in this family are expansive with the shadowing property but not uniformly expansive. Then, we deduce their topological stability not from [Reference Walters22] (or [Reference Lee and Rojas12]) but from Lemma 2.2.
Now, recall that a continuous map of a metric space
![]() $f:X\to X$
is dissipative if it possesses a compact absorbing set C; that is, for any bounded
$f:X\to X$
is dissipative if it possesses a compact absorbing set C; that is, for any bounded
![]() $B\subset X$
there is
$B\subset X$
there is
![]() $n_0(B)\in \mathbb {N}\cup \{0\}$
such that
$n_0(B)\in \mathbb {N}\cup \{0\}$
such that
This is the discrete version of the corresponding definition for semigroups (Definition 10.2, p. 264 in [Reference Robinson16]).
Lemma 2.6 Let X be a metric space. If there is a dissipative homeomorphism
![]() ${f:X\to X}$
, then X is proper and so uniformly locally compact.
${f:X\to X}$
, then X is proper and so uniformly locally compact.
Proof Let C be the compact absorbing set. Since any closed ball B is bounded one has
![]() $f^n(B)\subset C$
for some n. Since f is a homeomorphism,
$f^n(B)\subset C$
for some n. Since f is a homeomorphism,
![]() $f^n(B)$
is closed and so compact since it is contained in C. Since f is a homeomorphism,
$f^n(B)$
is closed and so compact since it is contained in C. Since f is a homeomorphism,
![]() $B=f^{-n}(f^n(B))$
is also compact proving the result.
$B=f^{-n}(f^n(B))$
is also compact proving the result.
The corollary below follows from Lemmas 2.2 and 2.6.
Corollary 2.7 Every dissipative expansive homeomorphism with the shadowing property is topologically stable.
3 Proof of the theorems
First, we prove Theorem 1.4.
Proof Let
![]() $f:\mathbb {R}^n\to \mathbb {R}^n$
be a topologically stable homeomorphism with global attractor. Let
$f:\mathbb {R}^n\to \mathbb {R}^n$
be a topologically stable homeomorphism with global attractor. Let
![]() $\rho>0$
be given by Lemma 2.1 for
$\rho>0$
be given by Lemma 2.1 for
![]() $\mathbb {A}$
. Fix
$\mathbb {A}$
. Fix
![]() $0<\epsilon <\rho $
, and let
$0<\epsilon <\rho $
, and let
![]() $\delta $
be given by the topological stability of f for this
$\delta $
be given by the topological stability of f for this
![]() $\epsilon $
. Take a homeomorphism
$\epsilon $
. Take a homeomorphism
![]() $g:\mathbb {R}^n\to \mathbb {R}^n$
such that
$g:\mathbb {R}^n\to \mathbb {R}^n$
such that
![]() $d_{C^0}(f,g)<\delta $
. Then, the topological stability of f provides
$d_{C^0}(f,g)<\delta $
. Then, the topological stability of f provides
![]() $h:\mathbb {R}^n\to \mathbb {R}^n$
continuous such that
$h:\mathbb {R}^n\to \mathbb {R}^n$
continuous such that
![]() $d_{C^0}(h,\mathrm{id}_{\mathbb {R}^n})<\epsilon $
and
$d_{C^0}(h,\mathrm{id}_{\mathbb {R}^n})<\epsilon $
and
![]() $f\circ h=h\circ g$
. By Lemma 2.1, we have that
$f\circ h=h\circ g$
. By Lemma 2.1, we have that
![]() $\mathbb {A}\subset h(\mathbb {R}^n)$
. In particular, one has
$\mathbb {A}\subset h(\mathbb {R}^n)$
. In particular, one has
![]() $\mathbb {A}_g=h^{-1}(\mathbb {A})\neq \emptyset .$
Let us prove that this set satisfies the required properties.
$\mathbb {A}_g=h^{-1}(\mathbb {A})\neq \emptyset .$
Let us prove that this set satisfies the required properties.
Since h is continuous,
![]() $\mathbb {A}_g$
is closed. Also for all
$\mathbb {A}_g$
is closed. Also for all
![]() $y,y'\in \mathbb {A}_g$
, one has
$y,y'\in \mathbb {A}_g$
, one has
![]() $h(y)=x$
and
$h(y)=x$
and
![]() $h(y')=x'$
for some
$h(y')=x'$
for some
![]() $x,x'\in \mathbb {A}$
. So,
$x,x'\in \mathbb {A}$
. So,
where
![]() $\mathrm{diam}(C)=\sup \{d(c,c'):c,c'\in C\}$
is the diameter of C, and
$\mathrm{diam}(C)=\sup \{d(c,c'):c,c'\in C\}$
is the diameter of C, and
![]() $d(p,q)=\|p-q\|$
is the Euclidean distance. Since
$d(p,q)=\|p-q\|$
is the Euclidean distance. Since
![]() $\mathbb {A}$
is compact,
$\mathbb {A}$
is compact,
![]() $\mathrm{diam}(\mathbb {A})<\infty $
hence
$\mathrm{diam}(\mathbb {A})<\infty $
hence
![]() $\mathrm{diam}(\mathbb {A}_g)<\infty $
and so
$\mathrm{diam}(\mathbb {A}_g)<\infty $
and so
![]() $\mathbb {A}_g$
is bounded. Therefore,
$\mathbb {A}_g$
is bounded. Therefore,
![]() $\mathbb {A}_g$
is compact.
$\mathbb {A}_g$
is compact.
Also if
![]() $y\in \mathbb {A}_g$
(and then
$y\in \mathbb {A}_g$
(and then
![]() $h(y)=x\in \mathbb {A}$
for some
$h(y)=x\in \mathbb {A}$
for some
![]() $x\in \mathbb {A}$
), one has
$x\in \mathbb {A}$
), one has
![]() $ h(g(y))=f(h(y))=f(x)\in \mathbb {A} $
so
$ h(g(y))=f(h(y))=f(x)\in \mathbb {A} $
so
![]() $g(y)\in \mathbb {A}_g$
, thus
$g(y)\in \mathbb {A}_g$
, thus
![]() $g(\mathbb {A}_g)\subset \mathbb {A}_g.$
Likewise,
$g(\mathbb {A}_g)\subset \mathbb {A}_g.$
Likewise,
![]() $h(g^{-1}(y))=f^{-1}(h(y))=f^{-1}(x)\in \mathbb {A}$
, thus
$h(g^{-1}(y))=f^{-1}(h(y))=f^{-1}(x)\in \mathbb {A}$
, thus
![]() $y\in g(\mathbb {A}_g)$
proving
$y\in g(\mathbb {A}_g)$
proving
![]() $g(\mathbb {A}_g)=\mathbb {A}_g$
that is
$g(\mathbb {A}_g)=\mathbb {A}_g$
that is
![]() $\mathbb {A}_g$
is an invariant set of g.
$\mathbb {A}_g$
is an invariant set of g.
Finally, we prove that
![]() $\mathbb {A}_g$
attracts bounded sets of
$\mathbb {A}_g$
attracts bounded sets of
![]() $\mathbb {R}^n$
under g. It suffices to prove that it attracts compact sets
$\mathbb {R}^n$
under g. It suffices to prove that it attracts compact sets
![]() $B\subset \mathbb {R}^n$
. Suppose by contradiction that this is not true. Then, there is a compact
$B\subset \mathbb {R}^n$
. Suppose by contradiction that this is not true. Then, there is a compact
![]() $B\subset \mathbb {R}^n$
such that
$B\subset \mathbb {R}^n$
such that
![]() $\mathrm{dist}(g^i(B),h^{-1}(\mathbb {A}))\not \to 0$
as
$\mathrm{dist}(g^i(B),h^{-1}(\mathbb {A}))\not \to 0$
as
![]() $i\to \infty .$
Up to passing to a subsequence if necessary, we can assume that there are a sequence
$i\to \infty .$
Up to passing to a subsequence if necessary, we can assume that there are a sequence
![]() $b_i\in B$
and
$b_i\in B$
and
![]() $\Delta>0$
such that
$\Delta>0$
such that
However,
![]() $h(B)$
is compact (hence bounded) so
$h(B)$
is compact (hence bounded) so
Since
![]() $h(b_i)\in h(B)$
, the above limit implies
$h(b_i)\in h(B)$
, the above limit implies
![]() $\mathrm{dist}(f^i(h(b_i)),\mathbb {A})\to 0$
as
$\mathrm{dist}(f^i(h(b_i)),\mathbb {A})\to 0$
as
![]() $i\to \infty .$
In particular,
$i\to \infty .$
In particular,
![]() $f^i(h(b_i))$
is bounded and so we can assume that
$f^i(h(b_i))$
is bounded and so we can assume that
![]() $f^i(h(b_i))\to w$
is convergent. Clearly,
$f^i(h(b_i))\to w$
is convergent. Clearly,
![]() $w\in \mathbb {A}$
. Since
$w\in \mathbb {A}$
. Since
for all i we have that
![]() $g^i(b_i)$
is also bounded. Therefore, we can assume that
$g^i(b_i)$
is also bounded. Therefore, we can assume that
![]() ${g^i(b_i)\to z}$
is convergent too. But then
${g^i(b_i)\to z}$
is convergent too. But then
![]() $h(g^i(b_i))\to h(z)$
so
$h(g^i(b_i))\to h(z)$
so
![]() $h(z)=w\in \mathbb {A}$
, hence
$h(z)=w\in \mathbb {A}$
, hence
![]() $z\in h^{-1}(\mathbb {A}).$
Nevertheless, by letting
$z\in h^{-1}(\mathbb {A}).$
Nevertheless, by letting
![]() $i\to \infty $
in (3.1), we get
$i\to \infty $
in (3.1), we get
![]() $\mathrm{dist}(z,h^{-1}(\mathbb {A}))\geq \Delta $
that’s is absurd. Therefore,
$\mathrm{dist}(z,h^{-1}(\mathbb {A}))\geq \Delta $
that’s is absurd. Therefore,
![]() $\mathbb {A}_g$
attracts bounded subsets of
$\mathbb {A}_g$
attracts bounded subsets of
![]() $\mathbb {R}^n$
under g. Since
$\mathbb {R}^n$
under g. Since
![]() $d_{C^0}(h,\mathrm{id}_{\mathbb {R}^n})<\epsilon $
and
$d_{C^0}(h,\mathrm{id}_{\mathbb {R}^n})<\epsilon $
and
![]() $\mathbb {A}\subset h(\mathbb {R}^n)$
, we get
$\mathbb {A}\subset h(\mathbb {R}^n)$
, we get
![]() $D(\mathbb {A},\mathbb {A}_g)<\epsilon .$
Finally, by taking
$D(\mathbb {A},\mathbb {A}_g)<\epsilon .$
Finally, by taking
![]() $h_g=h|_{\mathbb {A}_g}$
, we get
$h_g=h|_{\mathbb {A}_g}$
, we get
![]() $h_g:\mathbb {A}_g\to \mathbb {A}$
such that
$h_g:\mathbb {A}_g\to \mathbb {A}$
such that
![]() $d_{C^0}(h_g,i_{\mathbb {A}_g})\leq d_{C^0}(h,\mathrm{id}_{\mathbb {R}^n})<\epsilon $
and
$d_{C^0}(h_g,i_{\mathbb {A}_g})\leq d_{C^0}(h,\mathrm{id}_{\mathbb {R}^n})<\epsilon $
and
![]() $f|_{\mathbb {A}}\circ h_g=h_g\circ g|_{\mathbb {A}_g}$
completing the proof.
$f|_{\mathbb {A}}\circ h_g=h_g\circ g|_{\mathbb {A}_g}$
completing the proof.
Finally, we prove Theorem 1.5.
Proof Let
![]() $f:X\to X$
be a homeomorphism with global attractor of a locally compact metric space. Suppose that f is expansive and has the shadowing property. Since X is locally compact and f has a global attractor, f is dissipative. Then, f is topologically stable by Corollary 2.7.
$f:X\to X$
be a homeomorphism with global attractor of a locally compact metric space. Suppose that f is expansive and has the shadowing property. Since X is locally compact and f has a global attractor, f is dissipative. Then, f is topologically stable by Corollary 2.7.
Data availability statement
No data were used for the research described in the article.
Competing interest
The authors have no competing interest to declare.