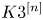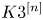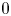1. Introduction
Let ![]() $S$ be a
$S$ be a ![]() $K3$ surface and
$K3$ surface and ![]() $H$ an ample divisor on
$H$ an ample divisor on ![]() $S$. By a theorem of Bogomolov and Mumford [Reference Mori and MukaiMM83], the linear system
$S$. By a theorem of Bogomolov and Mumford [Reference Mori and MukaiMM83], the linear system ![]() $|H|$ contains an element with irreducible components that are rational. A simple, yet striking application of the existence of ample rational curves on any projective
$|H|$ contains an element with irreducible components that are rational. A simple, yet striking application of the existence of ample rational curves on any projective ![]() $K3$ surface
$K3$ surface ![]() $S$ has been given by Beauville and Voisin in [Reference Beauville and VoisinBV04]. They remarked that any point on any rational curve on the
$S$ has been given by Beauville and Voisin in [Reference Beauville and VoisinBV04]. They remarked that any point on any rational curve on the ![]() $K3$ determines the same (canonical)
$K3$ determines the same (canonical) ![]() $0$-cycle
$0$-cycle ![]() $c_S$ of degree
$c_S$ of degree ![]() $1$, and proved that the image of the intersection product
$1$, and proved that the image of the intersection product ![]() $\textrm {Pic}(S)\otimes \textrm {Pic}(S)\to CH_0(S)$ is contained in
$\textrm {Pic}(S)\otimes \textrm {Pic}(S)\to CH_0(S)$ is contained in ![]() ${{\mathbb {Z}}} \cdot c_S$. Tensoring with
${{\mathbb {Z}}} \cdot c_S$. Tensoring with ![]() $\mathbb {Q}$ we may restate these two results as follows as an equality between the following three groups:
$\mathbb {Q}$ we may restate these two results as follows as an equality between the following three groups:
where ![]() $j_i:R_i\hookrightarrow S,\ i=1,2,$ are any two rational curves on the
$j_i:R_i\hookrightarrow S,\ i=1,2,$ are any two rational curves on the ![]() $K3$ surface
$K3$ surface ![]() $S$.
$S$.
The goal of this paper is to investigate the extent to which Bogomolov and Mumford's and Beauville and Voisin's results can be generalized to the higher-dimensional setting.
Let ![]() $X$ be a compact Kähler manifold. We say that
$X$ be a compact Kähler manifold. We say that ![]() $X$ is irreducible holomorphic symplectic (in the text, we often simply refer to such manifolds as holomorphic symplectic) if
$X$ is irreducible holomorphic symplectic (in the text, we often simply refer to such manifolds as holomorphic symplectic) if ![]() $X$ is simply connected and
$X$ is simply connected and ![]() $H^{0}(X, \Omega ^{2}_X)$ is spanned by an everywhere non-degenerate form of degree
$H^{0}(X, \Omega ^{2}_X)$ is spanned by an everywhere non-degenerate form of degree ![]() $2$. These objects were introduced by Beauville in [Reference BeauvilleBea83]. The holomorphic symplectic surfaces are the
$2$. These objects were introduced by Beauville in [Reference BeauvilleBea83]. The holomorphic symplectic surfaces are the ![]() $K3$ surfaces.
$K3$ surfaces.
The cohomology group ![]() $H^2(X, \mathbb{Z})$ is endowed with a natural quadratic form q, the Beauville–Bogomolov form. We denote it by q.
$H^2(X, \mathbb{Z})$ is endowed with a natural quadratic form q, the Beauville–Bogomolov form. We denote it by q.
It should be noted that holomorphic symplectic varieties with ![]() $b_2>3$ are not hyperbolic. This has been proved by Verbitsky, cf. [Reference VerbitskyVer15, Reference VerbitskyVer17], using among other things his global Torelli theorem [Reference VerbitskyVer13]. Much less seems to be known on the existence of rational curves on (projective) holomorphic symplectic varieties.
$b_2>3$ are not hyperbolic. This has been proved by Verbitsky, cf. [Reference VerbitskyVer15, Reference VerbitskyVer17], using among other things his global Torelli theorem [Reference VerbitskyVer13]. Much less seems to be known on the existence of rational curves on (projective) holomorphic symplectic varieties.
In order to investigate those, we make the following definition.
Definition 1.1 Let C be a stable curve of genus ![]() $0$, and let
$0$, and let ![]() $f : C\rightarrow X$ be a morphism that is unramified at all the generic points of C. We say that the curve
$f : C\rightarrow X$ be a morphism that is unramified at all the generic points of C. We say that the curve ![]() $f(C)$ in X is ruling if there exists a family
$f(C)$ in X is ruling if there exists a family
of stable curves over an irreducible, quasi-projective base S, a point ![]() $0\in S$, and a morphism
$0\in S$, and a morphism
such that ![]() $C=p^{-1}(0)$,
$C=p^{-1}(0)$, ![]() $\phi_{|C}=f$, and
$\phi_{|C}=f$, and ![]() $\phi(\mathscr{C})$ has codimension 1 in X.
$\phi(\mathscr{C})$ has codimension 1 in X.
We say that ![]() $\phi (\mathscr{C})$ is uniruled of codimension 1, that it is ruled by the stable curve
$\phi (\mathscr{C})$ is uniruled of codimension 1, that it is ruled by the stable curve ![]() $f:C\rightarrow X$ – or, for short, ruled by
$f:C\rightarrow X$ – or, for short, ruled by ![]() $f(C)$.
$f(C)$.
A stable genus ![]() $0$ curve in X is by definition a morphism
$0$ curve in X is by definition a morphism ![]() $f : C\rightarrow X$ as above.
$f : C\rightarrow X$ as above.
Given a curve R in X, we may consider the class ![]() $[R]$ of R in
$[R]$ of R in ![]() $H_2(X, \mathbb{Q})$. Let
$H_2(X, \mathbb{Q})$. Let ![]() $[R]^\vee\in H^2(X,\mathbb{Q})$ be the Poincaré dual of
$[R]^\vee\in H^2(X,\mathbb{Q})$ be the Poincaré dual of ![]() $[R]$. Then
$[R]$. Then ![]() $[R]^\vee$ is the class of a divisor in X. We say that R is positive if
$[R]^\vee$ is the class of a divisor in X. We say that R is positive if ![]() $q([R]^\vee)>0$, and that R is ample if
$q([R]^\vee)>0$, and that R is ample if ![]() $[R]^\vee$ is an ample class.
$[R]^\vee$ is an ample class.
Recall that a holomorphic symplectic manifold is said to be of ![]() $K3^{[n]}$-type if it is a deformation of the Hilbert scheme that parametrizes zero-dimensional subschemes of length
$K3^{[n]}$-type if it is a deformation of the Hilbert scheme that parametrizes zero-dimensional subschemes of length ![]() $n$ on some
$n$ on some ![]() $K3$ surface. Our main result is the following: we prove it in a slightly more precise and technical form in Theorem 4.5 and Proposition 4.6.
$K3$ surface. Our main result is the following: we prove it in a slightly more precise and technical form in Theorem 4.5 and Proposition 4.6.
Theorem 1.2 Let ![]() $n\geq 1$ be an integer. Let
$n\geq 1$ be an integer. Let ![]() $\mathfrak M=\bigcup _{d>0}\mathfrak M_{2d}$ be the union of the moduli spaces
$\mathfrak M=\bigcup _{d>0}\mathfrak M_{2d}$ be the union of the moduli spaces ![]() $\mathfrak M_{2d}$ of projective irreducible holomorphic symplectic varieties of
$\mathfrak M_{2d}$ of projective irreducible holomorphic symplectic varieties of ![]() $K3^{[n]}$-type polarized by a line bundle of degree
$K3^{[n]}$-type polarized by a line bundle of degree ![]() $2d$. For all
$2d$. For all ![]() $(X,H)\in \mathfrak M$, outside at most a finite number of connected components, the following holds:
$(X,H)\in \mathfrak M$, outside at most a finite number of connected components, the following holds:
(1) there exists a ruling genus
 $0$ stable curve in X with cohomology class proportional to the Poincaré-dual of the class of H;
$0$ stable curve in X with cohomology class proportional to the Poincaré-dual of the class of H;(2) there exists a positive integer m such that the linear system
 $|mH|$ contains a uniruled divisor.
$|mH|$ contains a uniruled divisor.
Remark 1.3 The ruling curve in (1) may be chosen to have primitive cohomology class, see the comments below. However, we are not able to control the integer m in (2).
Some comments are in order. The Beauville–Bogomolov form induces an embedding ![]() $H^2(X,\mathbb{Z})\hookrightarrow H_2(X,\mathbb{Z}), H\mapsto H^\vee$. By abuse of notation, we again denote by
$H^2(X,\mathbb{Z})\hookrightarrow H_2(X,\mathbb{Z}), H\mapsto H^\vee$. By abuse of notation, we again denote by ![]() $q$ the quadratic form on
$q$ the quadratic form on ![]() $H_2(X,\mathbb {Z})$. We can make explicit the components of
$H_2(X,\mathbb {Z})$. We can make explicit the components of ![]() $\mathfrak M$ for which the existence is obtained.
$\mathfrak M$ for which the existence is obtained.
Remark 1.4 The previous statement may be split into two parts. On the one hand, Theorem 4.5 ensures the existence of uniruled divisors covered by primitive rational curves if there exist integers ![]() $p,g$ and
$p,g$ and ![]() $\epsilon$ such that
$\epsilon$ such that ![]() $p\geq g$ and
$p\geq g$ and ![]() $\epsilon =0$ or
$\epsilon =0$ or ![]() $1$ such that the following two conditions hold:
$1$ such that the following two conditions hold:
(i) the class
 $\alpha :={H^{\vee }}/{ {\rm div}(H)}\in H_2(X,\mathbb {Z})$ can be written as
$\alpha :={H^{\vee }}/{ {\rm div}(H)}\in H_2(X,\mathbb {Z})$ can be written as  $\gamma +(2g-\epsilon )\eta$, with
$\gamma +(2g-\epsilon )\eta$, with  $\eta$ in the monodromy orbit of the class of the exceptional curve on a
$\eta$ in the monodromy orbit of the class of the exceptional curve on a  $K3^{[n]}$ and
$K3^{[n]}$ and  $\gamma \in \eta ^{\perp }$;
$\gamma \in \eta ^{\perp }$;(ii)
 $q(\gamma )=2p-2$ (hence,
$q(\gamma )=2p-2$ (hence,  $q(\alpha )=2p-2-{(2g-\epsilon )^{2}}/({2n-2})$).
$q(\alpha )=2p-2-{(2g-\epsilon )^{2}}/({2n-2})$).
On the other hand, thanks to Proposition 4.6, we can show that the two conditions above are satisfied outside at most a finite number of connected components. Observe furthermore that conditions (i) and (ii) determine the monodromy orbit of the polarization ![]() $H$ (cf. Corollary 2.8 for details).
$H$ (cf. Corollary 2.8 for details).
We list some relevant cases in which the conditions (i) and (ii) of Remark 1.4 are easily seen to be satisfied in the following.
Remark 1.5 (i) If ![]() $q(\alpha )\geq n-1$, then a multiple of
$q(\alpha )\geq n-1$, then a multiple of ![]() $H$ is uniruled by primitive rational curves of class
$H$ is uniruled by primitive rational curves of class ![]() $\alpha$ (see Proposition 4.6).
$\alpha$ (see Proposition 4.6).
(ii) If ![]() $\rho (X)\geq 2$, then
$\rho (X)\geq 2$, then ![]() $X$ always contains an ample uniruled divisor covered by primitive rational curves (cf. Corollary 4.7).
$X$ always contains an ample uniruled divisor covered by primitive rational curves (cf. Corollary 4.7).
(iii) If ![]() $n\leq 7$, then the conclusion of the theorem holds for all the connected components of
$n\leq 7$, then the conclusion of the theorem holds for all the connected components of ![]() $\mathfrak M$ (cf. Remark 4.8).
$\mathfrak M$ (cf. Remark 4.8).
(iv) If ![]() $n-1$ is a power of a prime number, then by [Reference MarkmanMar11, Lemma 9.2 and subsequent comment], the monodromy group is maximal. Therefore, it suffices to check that the square
$n-1$ is a power of a prime number, then by [Reference MarkmanMar11, Lemma 9.2 and subsequent comment], the monodromy group is maximal. Therefore, it suffices to check that the square ![]() $q(\alpha )$ is of the form
$q(\alpha )$ is of the form ![]() $2p-2-{(2g-\epsilon )^{2}}/({2n-2})$, with
$2p-2-{(2g-\epsilon )^{2}}/({2n-2})$, with ![]() $p\geq g$.
$p\geq g$.
The existence of uniruled divisors ruled by primitive rational curves on any projective holomorphic symplectic variety of ![]() $K3^{[n]}$-type was wrongly claimed in [Reference Charles and PacienzaCP14]. Counterexamples were recently provided by Oberdieck, Shen and Yin in [Reference Oberdieck, Shen and YinOSY19, Corollary A.3]. The proof presented in [Reference Charles and PacienzaCP14] was based upon the following three ingredients: (a) the existence of a ‘controlled’ polarized deformation of a polarized holomorphic symplectic variety
$K3^{[n]}$-type was wrongly claimed in [Reference Charles and PacienzaCP14]. Counterexamples were recently provided by Oberdieck, Shen and Yin in [Reference Oberdieck, Shen and YinOSY19, Corollary A.3]. The proof presented in [Reference Charles and PacienzaCP14] was based upon the following three ingredients: (a) the existence of a ‘controlled’ polarized deformation of a polarized holomorphic symplectic variety ![]() $(X,H)$ to a
$(X,H)$ to a ![]() $((K3)^{[n]}, H')$, as a consequence of Verbitsky's global Torelli theorem and Markman's study of the monodromy group (see § 2); (b) a geometric criterion to deform a rational curve on a holomorphic symplectic variety
$((K3)^{[n]}, H')$, as a consequence of Verbitsky's global Torelli theorem and Markman's study of the monodromy group (see § 2); (b) a geometric criterion to deform a rational curve on a holomorphic symplectic variety ![]() $X$ along its Hodge locus inside the moduli space of
$X$ along its Hodge locus inside the moduli space of ![]() $X$, which we derive from Mumford's theorem on
$X$, which we derive from Mumford's theorem on ![]() $0$-cycles and deformation theoretic arguments (cf. § 3); (c) the existence of uniruled divisors on a
$0$-cycles and deformation theoretic arguments (cf. § 3); (c) the existence of uniruled divisors on a ![]() $(K3)^{[n]}$, via points on nodal curves in the hyperplane linear system (see § 4). These three parts of the proof are correct and are presented here essentially as in [Reference Charles and PacienzaCP14]. The main difference is that, after the appearance of [Reference Oberdieck, Shen and YinOSY19], we realized that the examples we provided in ingredient (c) did not (and actually could not, because of [Reference Oberdieck, Shen and YinOSY19, Corollary A.3]) cover all the connected components of
$(K3)^{[n]}$, via points on nodal curves in the hyperplane linear system (see § 4). These three parts of the proof are correct and are presented here essentially as in [Reference Charles and PacienzaCP14]. The main difference is that, after the appearance of [Reference Oberdieck, Shen and YinOSY19], we realized that the examples we provided in ingredient (c) did not (and actually could not, because of [Reference Oberdieck, Shen and YinOSY19, Corollary A.3]) cover all the connected components of ![]() $\mathfrak M$. The same type of considerations and results hold in the generalized Kummer case, treated in [Reference Mongardi and PacienzaMP18] and amended in [Reference Mongardi and PacienzaMP21].
$\mathfrak M$. The same type of considerations and results hold in the generalized Kummer case, treated in [Reference Mongardi and PacienzaMP18] and amended in [Reference Mongardi and PacienzaMP21].
In the present paper we also show that conditions (i) and (ii) in Remark 1.4 are precisely satisfied in all cases where the obstruction discovered by [Reference Oberdieck, Shen and YinOSY19] does not prevent uniruled divisors covered by primitive rational curves to exist. In other words, we show that our result is sharp (see § 5.1 for details). In all cases where the theorem fails, using [Reference Knutsen, Lelli-Chiesa and MongardiKLM19], we can check the existence of a family of the expected dimension of rational curves covering a coisotropic subvariety of codimension ![]() $c\geq 2$ (cf. Proposition 5.13. Moreover, in some of these cases we can actually prove that
$c\geq 2$ (cf. Proposition 5.13. Moreover, in some of these cases we can actually prove that ![]() $c=2$ (see Theorem 5.11 in § 5.3).
$c=2$ (see Theorem 5.11 in § 5.3).
In § 5.2 we discuss the extent to which it might be possible to find uniruled divisors covered by possibly non-primite rational curves. We find an explicit condition (cf. Proposition 5.6) for the existence of such divisors, which can be easily checked on examples. For instance, this allows us to check the existence of ample uniruled divisors covered by non-primitive rational curves for all the connected components of the moduli space ![]() $\mathfrak M$ up to dimension
$\mathfrak M$ up to dimension ![]() $26$.
$26$.
It should be noted that applications of the existence of uniruled divisors to the study of Chow groups of ![]() $0$-cycles does not make use of the primitivity of the relevant rational curves. These applications are presented in § 6. To state our results recall that if
$0$-cycles does not make use of the primitivity of the relevant rational curves. These applications are presented in § 6. To state our results recall that if ![]() $Y$ is a variety,
$Y$ is a variety, ![]() $CH_0(Y)_{{\rm hom}}$ is the subgroup of
$CH_0(Y)_{{\rm hom}}$ is the subgroup of ![]() $CH_0(Y)$ consisting of
$CH_0(Y)$ consisting of ![]() $0$-cycles of degree
$0$-cycles of degree ![]() $0$.
$0$.
Definition 1.6 Let ![]() $D$ be an irreducible divisor on
$D$ be an irreducible divisor on ![]() $X$. We denote by
$X$. We denote by ![]() $S_1 CH_0(X)_{D,{\rm hom}}$ the subgroup
$S_1 CH_0(X)_{D,{\rm hom}}$ the subgroup
of ![]() $CH_0(X)$. We denote by
$CH_0(X)$. We denote by ![]() $S_1 CH_0(X)_{D}$ the subgroup
$S_1 CH_0(X)_{D}$ the subgroup
of ![]() $CH_0(X)$.
$CH_0(X)$.
We prove the following.
Theorem 1.7 Let ![]() $X$ be a projective holomorphic symplectic variety. Suppose that
$X$ be a projective holomorphic symplectic variety. Suppose that ![]() $X$ possesses an ample ruling curve. Then the subgroups
$X$ possesses an ample ruling curve. Then the subgroups ![]() $S_1 CH_0(X)_{D,{\rm hom}}$ and
$S_1 CH_0(X)_{D,{\rm hom}}$ and ![]() $S_1 CH_0(X)_{D}$ are independent of the irreducible uniruled divisor D.
$S_1 CH_0(X)_{D}$ are independent of the irreducible uniruled divisor D.
In light of the previous result, we set
where ![]() $j:D\hookrightarrow X$ is any irreducible uniruled divisor. It is natural to ask whether such a subgroup of
$j:D\hookrightarrow X$ is any irreducible uniruled divisor. It is natural to ask whether such a subgroup of ![]() $CH_0(X)_{{\mathbb {Q}}}$ has an intersection-theoretic interpretation, as for
$CH_0(X)_{{\mathbb {Q}}}$ has an intersection-theoretic interpretation, as for ![]() $K3$ surfaces. This is indeed the case.
$K3$ surfaces. This is indeed the case.
Theorem 1.8 Let ![]() $X$ be a projective holomorphic symplectic variety. Suppose that X possesses an ample ruling curve and that the group of cohomology classes of curves on X is generated over
$X$ be a projective holomorphic symplectic variety. Suppose that X possesses an ample ruling curve and that the group of cohomology classes of curves on X is generated over ![]() $\mathbb{Q}$ by classes of ruling curves. Then, for any non-trivial
$\mathbb{Q}$ by classes of ruling curves. Then, for any non-trivial ![]() $L\in \textrm {Pic}(X)$, we have
$L\in \textrm {Pic}(X)$, we have
In particular, the conclusions of the results given previously hold for all projective holomorphic symplectic variety of ![]() $K3^{[n]}$-type, for
$K3^{[n]}$-type, for ![]() $n\leq 13$, and, for higher
$n\leq 13$, and, for higher ![]() $n$, for all but finitely many components of
$n$, for all but finitely many components of ![]() $\mathfrak M$, as specified in Remark 1.4. The hypothesis on the Picard group is verified in applications by showing the existence of uniruled divisors linearly equivalent to certain multiple of each ample divisor. There is no evidence this could not hold in general.
$\mathfrak M$, as specified in Remark 1.4. The hypothesis on the Picard group is verified in applications by showing the existence of uniruled divisors linearly equivalent to certain multiple of each ample divisor. There is no evidence this could not hold in general.
The theorems given previously may be regarded as a higher-dimensional analogue of the Beauville–Voisin result. It is important to note that, for holomorphic symplectic varieties of higher dimension, Beauville has stated in [Reference BeauvilleBea07] a far-reaching conjectural generalization of their result, called the ‘weak splitting property’, which can be deduced by a (conjectural) splitting of the (conjectural) Bloch–Beilinson filtration on the Chow group. The conjecture was further refined by Voisin in [Reference VoisinVoi08]. These conjectures have been studied intensively in recent years. After the appearance of a first version of this paper, Voisin [Reference VoisinVoi16] has unveiled a surprising conjectural connection between the weak splitting property conjecture and the existence of subvarieties whose zero cycles are supported in lower dimension. A first instance of such an existence result is provided by Theorem 1.2. For a (non-exhaustive) list of works in this research direction, see [Reference VoisinVoi08, Reference FerrettiFer12, Reference FuFu13, Reference FuFu15, Reference HuybrechtsHuy14, Reference RiessRie16, Reference VoisinVoi15, Reference LaterveerLat18, Reference LinLin20, Reference LinLin16, Reference Lehn and PacienzaLP19, Reference YinYin15, Reference Shen and VialSV16, Reference Mongardi and PacienzaMP18, Reference VialVia17, Reference Shen, Yin and ZhaoSYZ20, Reference Shen and YinSY20, Reference Fu, Laterveer, Vial and ShenFLVS19, Reference Oberdieck, Shen and YinOSY19, Reference VoisinVoi22].
We end the paper with some speculations on possible generalizations of our results.
After the appearance of [Reference Charles and PacienzaCP14], other researchers studied the existence of rational curves on projective holomorphic symplectic varieties. We already mentioned [Reference Mongardi and PacienzaMP18, Reference Mongardi and PacienzaMP21] where the analogous existence results of ample uniruled divisors are established for deformations of generalized Kummer varieties. The existence of primitive rational curves moving in a family of the expected dimension on projective holomorphic symplectic varieties deformations of punctual Hilbert schemes on a ![]() $K3$ surface or of generalized Kummer varieties is shown in [Reference Mongardi and OttemMO20, Theorem 3.2] and [Reference Mongardi and PacienzaMP18, Theorem 5.1]. While finishing the first version of the paper [Reference Charles and PacienzaCP14], independent work of Amerik and Verbitsky [Reference Amerik and VerbitskyAV15] has appeared and § 4 of [Reference Amerik and VerbitskyAV15] has some overlap with the results and arguments presented here in § 3 concerning the deformations of rational curves on holomorphic symplectic varieties. Amerik and Verbitsky are more concerned with negative rational curves, whereas we focus on positive ones (i.e. dual to an ample class). As the goals and the results of the two papers are quite different, for the sake of completeness we did not try to eliminate similar discussions. We refer the reader to § 3 here for precise references to the similar results appearing in [Reference Amerik and VerbitskyAV15].
$K3$ surface or of generalized Kummer varieties is shown in [Reference Mongardi and OttemMO20, Theorem 3.2] and [Reference Mongardi and PacienzaMP18, Theorem 5.1]. While finishing the first version of the paper [Reference Charles and PacienzaCP14], independent work of Amerik and Verbitsky [Reference Amerik and VerbitskyAV15] has appeared and § 4 of [Reference Amerik and VerbitskyAV15] has some overlap with the results and arguments presented here in § 3 concerning the deformations of rational curves on holomorphic symplectic varieties. Amerik and Verbitsky are more concerned with negative rational curves, whereas we focus on positive ones (i.e. dual to an ample class). As the goals and the results of the two papers are quite different, for the sake of completeness we did not try to eliminate similar discussions. We refer the reader to § 3 here for precise references to the similar results appearing in [Reference Amerik and VerbitskyAV15].
We always work over the field ![]() ${{\mathbb {C}}}$ of complex numbers.
${{\mathbb {C}}}$ of complex numbers.
2. Varieties of  $K3^{[n]}$-type and their polarizations
$K3^{[n]}$-type and their polarizations
In this section we collect a number of known results on Hilbert schemes of points on a ![]() $K3$ surface and then move on to study the polarized deformations of irreducible holomorphic symplectic varieties of
$K3$ surface and then move on to study the polarized deformations of irreducible holomorphic symplectic varieties of ![]() $K3^{[n]}$-type.
$K3^{[n]}$-type.
2.1 Lattices
The general theory of irreducible holomorphic symplectic varieties as in [Reference BeauvilleBea83] shows that the group ![]() $H^{2}(X, {{\mathbb {Z}}})$ is endowed with a natural symmetric bilinear form
$H^{2}(X, {{\mathbb {Z}}})$ is endowed with a natural symmetric bilinear form ![]() $q_X$ of signature
$q_X$ of signature ![]() $(3, 20)$, the Beauville–Bogomolov form. When no confusion is possible, we denote the square
$(3, 20)$, the Beauville–Bogomolov form. When no confusion is possible, we denote the square ![]() $q_X(h)$ of an element
$q_X(h)$ of an element ![]() $h\in H^{2}(X, {{\mathbb {Z}}})$ simply by
$h\in H^{2}(X, {{\mathbb {Z}}})$ simply by ![]() $q(h)$ or by
$q(h)$ or by ![]() $h^{2}$. Therefore,
$h^{2}$. Therefore, ![]() $(H^{2}(X, {{\mathbb {Z}}}), q_X)$ is naturally a lattice. We try to use the notation
$(H^{2}(X, {{\mathbb {Z}}}), q_X)$ is naturally a lattice. We try to use the notation ![]() $h$ for elements of the cohomology lattice and the capital letter
$h$ for elements of the cohomology lattice and the capital letter ![]() $H$ for divisors/line bundles (but no confusion should hopefully arise if we do otherwise somewhere).
$H$ for divisors/line bundles (but no confusion should hopefully arise if we do otherwise somewhere).
Definition 2.1 Let ![]() $\Lambda$ be a free
$\Lambda$ be a free ![]() ${{\mathbb {Z}}}$-module of finite rank endowed with a symmetric bilinear form. If
${{\mathbb {Z}}}$-module of finite rank endowed with a symmetric bilinear form. If ![]() $h$ is an element of
$h$ is an element of ![]() $\Lambda$, the divisibility of
$\Lambda$, the divisibility of ![]() $h$ is the non-negative integer
$h$ is the non-negative integer ![]() $t$ such that
$t$ such that
It will be denoted by ![]() ${\rm div}(h)$. If
${\rm div}(h)$. If ![]() $(X, h)$ is a polarized irreducible holomorphic symplectic variety, then the divisibility of
$(X, h)$ is a polarized irreducible holomorphic symplectic variety, then the divisibility of ![]() $h$ is its divisibility as an element of the lattice
$h$ is its divisibility as an element of the lattice ![]() $H^{2}(X, {{\mathbb {Z}}})$ endowed with the Beauville–Bogomolov form.
$H^{2}(X, {{\mathbb {Z}}})$ endowed with the Beauville–Bogomolov form.
We use the following maps relating a lattice ![]() $(\Lambda ,q)$ and its dual
$(\Lambda ,q)$ and its dual ![]() $\Lambda ^{\vee }$.
$\Lambda ^{\vee }$.
There is a natural map
Given a class ![]() $c \in \Lambda ^{\vee }$ there exists a unique class
$c \in \Lambda ^{\vee }$ there exists a unique class ![]() $\lambda _c \in \Lambda \otimes {{\mathbb {Q}}}$ such that for all
$\lambda _c \in \Lambda \otimes {{\mathbb {Q}}}$ such that for all ![]() $\lambda '\in \Lambda$ we have
$\lambda '\in \Lambda$ we have
This induces a natural map
Using the previous results we can endow ![]() $\Lambda ^{\vee }$ with a quadratic form taking rational values. By abuse of notation, we still denote it by
$\Lambda ^{\vee }$ with a quadratic form taking rational values. By abuse of notation, we still denote it by ![]() $q$.
$q$.
We often make use of the following set-theoretic map:
One may easily check that the image is contained in the image of ![]() $\Lambda ^{\vee }$ under the map (2.2) and gives all primitive elements in it.
$\Lambda ^{\vee }$ under the map (2.2) and gives all primitive elements in it.
Finally, the map given previously will be composed with the projection onto the discriminant group ![]() $\Lambda ^{\vee }/\Lambda$:
$\Lambda ^{\vee }/\Lambda$:
2.2 Hilbert schemes of points on a  $K3$ surface
$K3$ surface
Let ![]() $S$ be a compact complex projective surface. If
$S$ be a compact complex projective surface. If ![]() $n$ is a positive integer, denote by
$n$ is a positive integer, denote by ![]() $S^{[n]}$ the Hilbert scheme (or the Douady space in the non-projective case) of length
$S^{[n]}$ the Hilbert scheme (or the Douady space in the non-projective case) of length ![]() $n$ subschemes of
$n$ subschemes of ![]() $S$. By [Reference FogartyFog68],
$S$. By [Reference FogartyFog68], ![]() $S^{[n]}$ is a smooth complex variety. The general theory of the Hilbert scheme shows that
$S^{[n]}$ is a smooth complex variety. The general theory of the Hilbert scheme shows that ![]() $S^{[n]}$ is projective if
$S^{[n]}$ is projective if ![]() $S$ is.
$S$ is.
Assume that ![]() $S$ is a
$S$ is a ![]() $K3$ surface. By [Reference BeauvilleBea83],
$K3$ surface. By [Reference BeauvilleBea83], ![]() $S^{[n]}$ is an irreducible holomorphic symplectic variety: it is simply connected, and the space
$S^{[n]}$ is an irreducible holomorphic symplectic variety: it is simply connected, and the space ![]() $H^{0}(S^{[n]}, \Omega ^{2}_{S^{[n]}})$ is one-dimensional, generated by a holomorphic symplectic form. Let
$H^{0}(S^{[n]}, \Omega ^{2}_{S^{[n]}})$ is one-dimensional, generated by a holomorphic symplectic form. Let ![]() $X$ be an irreducible holomorphic symplectic variety. We say that
$X$ be an irreducible holomorphic symplectic variety. We say that ![]() $X$ is of
$X$ is of ![]() $K3^{[n]}$-type if
$K3^{[n]}$-type if ![]() $X$ is deformation equivalent, as a complex variety, to
$X$ is deformation equivalent, as a complex variety, to ![]() $S^{[n]}$, where
$S^{[n]}$, where ![]() $S$ is a complex
$S$ is a complex ![]() $K3$ surface.
$K3$ surface.
Let ![]() $S$ be a
$S$ be a ![]() $K3$ surface, and let
$K3$ surface, and let ![]() $n>1$ be an integer. We briefly recall the description of the group
$n>1$ be an integer. We briefly recall the description of the group ![]() $H^{2}(S^{[n]}, {{\mathbb {Z}}})$ as in [Reference BeauvilleBea83, Proposition 6].
$H^{2}(S^{[n]}, {{\mathbb {Z}}})$ as in [Reference BeauvilleBea83, Proposition 6].
Let ![]() $S^{(n)}$ be the
$S^{(n)}$ be the ![]() $n$th symmetric product of
$n$th symmetric product of ![]() $S$, and let
$S$, and let ![]() $\epsilon : S^{[n]}\rightarrow S^{(n)}$ be the Hilbert–Chow morphism. The map
$\epsilon : S^{[n]}\rightarrow S^{(n)}$ be the Hilbert–Chow morphism. The map
is injective. Furthermore, let ![]() $\pi : S^{n}\rightarrow S^{(n)}$ be the canonical quotient map, and let
$\pi : S^{n}\rightarrow S^{(n)}$ be the canonical quotient map, and let ![]() $p_1, \ldots , p_n$ be the projections from
$p_1, \ldots , p_n$ be the projections from ![]() $S^{n}$ to
$S^{n}$ to ![]() $S$. There exists a unique map
$S$. There exists a unique map
such that for any ![]() $\alpha \in H^{2}(S, {{\mathbb {Z}}})$,
$\alpha \in H^{2}(S, {{\mathbb {Z}}})$, ![]() $i(\alpha )=\epsilon ^{*}(\beta )$, where
$i(\alpha )=\epsilon ^{*}(\beta )$, where ![]() $\pi ^{*}(\beta )=p_1^{*}(\alpha )+\cdots +p_n^{*}(\alpha )$. The map
$\pi ^{*}(\beta )=p_1^{*}(\alpha )+\cdots +p_n^{*}(\alpha )$. The map ![]() $i$ is an injection.
$i$ is an injection.
Let ![]() $E_n$ be the exceptional divisor in
$E_n$ be the exceptional divisor in ![]() $S^{[n]}$, that is, the divisor that parametrizes non-reduced subschemes (we will drop the index
$S^{[n]}$, that is, the divisor that parametrizes non-reduced subschemes (we will drop the index ![]() $n$ and simply write
$n$ and simply write ![]() $E=E_n$ when no confusion is possible). The cohomology class of
$E=E_n$ when no confusion is possible). The cohomology class of ![]() $E$ in
$E$ in ![]() $H^{2}(S^{[n]}, {{\mathbb {Z}}})$ is uniquely divisible by two; see [Reference BeauvilleBea83, Remarque after Proposition 6]. Let
$H^{2}(S^{[n]}, {{\mathbb {Z}}})$ is uniquely divisible by two; see [Reference BeauvilleBea83, Remarque after Proposition 6]. Let ![]() $\delta :=\delta _n$ be the element of
$\delta :=\delta _n$ be the element of ![]() $H^{2}(S^{[n]}, {{\mathbb {Z}}})$ such that
$H^{2}(S^{[n]}, {{\mathbb {Z}}})$ such that ![]() $2\delta _n=[E]$. Then we have
$2\delta _n=[E]$. Then we have
where the embedding of ![]() $H^{2}(S, {{\mathbb {Z}}})$ into
$H^{2}(S, {{\mathbb {Z}}})$ into ![]() $H^{2}(S^{[n]}, {{\mathbb {Z}}})$ is that given by the map
$H^{2}(S^{[n]}, {{\mathbb {Z}}})$ is that given by the map ![]() $i$.
$i$.
The decomposition (2.5) is orthogonal with respect to the Beauville–Bogomolov form ![]() $q$, and the restriction of
$q$, and the restriction of ![]() $q$ to
$q$ to ![]() $H^{2}(S, {{\mathbb {Z}}})$ is the canonical quadratic form on the second cohomology group of a surface induced by cup product. We have
$H^{2}(S, {{\mathbb {Z}}})$ is the canonical quadratic form on the second cohomology group of a surface induced by cup product. We have
By Poincaré duality, ![]() $H_2(S^{[n]}, {{\mathbb {Z}}})$ may be identified to the dual lattice of
$H_2(S^{[n]}, {{\mathbb {Z}}})$ may be identified to the dual lattice of ![]() $H^{2}(S^{[n]}, {{\mathbb {Z}}})$. Therefore, using (2.2), to a class
$H^{2}(S^{[n]}, {{\mathbb {Z}}})$. Therefore, using (2.2), to a class ![]() $Z\in H_2(S^{[n]}, {{\mathbb {Z}}})$ we can associate a unique class
$Z\in H_2(S^{[n]}, {{\mathbb {Z}}})$ we can associate a unique class ![]() $D_Z\in H^{2}(S^{[n]}, {{\mathbb {Q}}})$ such that for all
$D_Z\in H^{2}(S^{[n]}, {{\mathbb {Q}}})$ such that for all ![]() $D'\in H^{2}(S^{[n]}, {{\mathbb {Z}}})$ we have
$D'\in H^{2}(S^{[n]}, {{\mathbb {Z}}})$ we have
The class ![]() $D_Z$ is called the dual of
$D_Z$ is called the dual of ![]() $Z$ with respect to the Beauville–Bogomolov quadratic form. In this way, we obtain a quadratic form on the homology group
$Z$ with respect to the Beauville–Bogomolov quadratic form. In this way, we obtain a quadratic form on the homology group ![]() $H_2(S^{[n]}, {{\mathbb {Z}}})$ taking rational values and such that
$H_2(S^{[n]}, {{\mathbb {Z}}})$ taking rational values and such that
where ![]() $r_n$ is the homology class orthogonal to
$r_n$ is the homology class orthogonal to ![]() $H_2(S, {{\mathbb {Z}}})$ and such that
$H_2(S, {{\mathbb {Z}}})$ and such that
In particular, we have
which implies
Geometrically, ![]() $r_n$ is the class of an exceptional rational curve that is the general fiber of the Hilbert–Chow morphism (see, e.g., [Reference Hassett and TschinkelHT10]).
$r_n$ is the class of an exceptional rational curve that is the general fiber of the Hilbert–Chow morphism (see, e.g., [Reference Hassett and TschinkelHT10]).
By abuse of notation, if ![]() $h\in H^{2}(S,{{\mathbb {Z}}})$, we again denote by
$h\in H^{2}(S,{{\mathbb {Z}}})$, we again denote by ![]() $h$ the induced class in
$h$ the induced class in ![]() $H^{2}(S^{[n]}, {{\mathbb {Z}}})$ as well as that in
$H^{2}(S^{[n]}, {{\mathbb {Z}}})$ as well as that in ![]() $H_2(S^{[n]}, {{\mathbb {Z}}})$, using the embedding (2.1).
$H_2(S^{[n]}, {{\mathbb {Z}}})$, using the embedding (2.1).
2.3 Polarized deformations of varieties of  $K3^{[n]}$-type
$K3^{[n]}$-type
In this paper, we are interested in the possible deformation types for primitively polarized varieties ![]() $(X, h)$, where
$(X, h)$, where ![]() $X$ is a variety of
$X$ is a variety of ![]() $K3^{[n]}$-type and
$K3^{[n]}$-type and ![]() $h$ is a primitive polarization of
$h$ is a primitive polarization of ![]() $X$, that is, the numerical equivalence class of a primitive and ample line bundle on
$X$, that is, the numerical equivalence class of a primitive and ample line bundle on ![]() $X$.
$X$.
Definition 2.2 Let ![]() $X$ and
$X$ and ![]() $X'$ be two compact complex manifolds, and let
$X'$ be two compact complex manifolds, and let ![]() $h, h'$ be numerical equivalence classes of line bundles on
$h, h'$ be numerical equivalence classes of line bundles on ![]() $X$ and
$X$ and ![]() $X'$, respectively. We say that the pairs
$X'$, respectively. We say that the pairs ![]() $(X, h)$ and
$(X, h)$ and ![]() $(X', h')$ are deformation equivalent if there exists a connected complex variety
$(X', h')$ are deformation equivalent if there exists a connected complex variety ![]() $S$, a smooth, proper morphism
$S$, a smooth, proper morphism ![]() $\pi : \mathscr{X}\rightarrow S$, a line bundle
$\pi : \mathscr{X}\rightarrow S$, a line bundle ![]() $\mathscr{L}$ on
$\mathscr{L}$ on ![]() $\mathscr{X}$ and two points
$\mathscr{X}$ and two points ![]() $s, s'$ of
$s, s'$ of ![]() $S$ such
$S$ such ![]() $(\mathscr{X}_s, c_1(\mathscr{L}_s))$ is isomorphic to
$(\mathscr{X}_s, c_1(\mathscr{L}_s))$ is isomorphic to ![]() $(X, h)$ and
$(X, h)$ and ![]() $(\mathscr{X}_{s'}, c_1(\mathscr{L}_{s'}))$ is isomorphic to
$(\mathscr{X}_{s'}, c_1(\mathscr{L}_{s'}))$ is isomorphic to ![]() $(X', h')$.
$(X', h')$.
Remark 2.3 Let ![]() $X$ be a variety of
$X$ be a variety of ![]() $K3^{[n]}$-type, and let
$K3^{[n]}$-type, and let ![]() $h$ be a polarization on
$h$ be a polarization on ![]() $X$. Then Markman shows in [Reference MarkmanMar11, Proposition 7.1] that there exists a
$X$. Then Markman shows in [Reference MarkmanMar11, Proposition 7.1] that there exists a ![]() $K3$ surface
$K3$ surface ![]() $S$ and a polarization
$S$ and a polarization ![]() $h'$ on
$h'$ on ![]() $S^{[n]}$ such that
$S^{[n]}$ such that ![]() $(X, h)$ is deformation equivalent to
$(X, h)$ is deformation equivalent to ![]() $(S^{[n]}, h')$.
$(S^{[n]}, h')$.
In the surface case, that is, when ![]() $n=1$, the global Torelli theorem implies that two primitively polarized
$n=1$, the global Torelli theorem implies that two primitively polarized ![]() $K3$ surfaces
$K3$ surfaces ![]() $(X, h)$ and
$(X, h)$ and ![]() $(X', h')$ are deformation equivalent if and only
$(X', h')$ are deformation equivalent if and only ![]() $h^{2}=h'^{2}$. The situation is different in higher dimension.
$h^{2}=h'^{2}$. The situation is different in higher dimension.
Let ![]() $X$ be a variety of
$X$ be a variety of ![]() $K3^{[n]}$-type. If
$K3^{[n]}$-type. If ![]() $n=1$, the lattice
$n=1$, the lattice ![]() $H^{2}(X, {{\mathbb {Z}}})$ is unimodular, so that the divisibility of any non-zero primitive element of
$H^{2}(X, {{\mathbb {Z}}})$ is unimodular, so that the divisibility of any non-zero primitive element of ![]() $H^{2}(X, {{\mathbb {Z}}})$ is
$H^{2}(X, {{\mathbb {Z}}})$ is ![]() $1$. This is no longer the case as soon as
$1$. This is no longer the case as soon as ![]() $n>1$.
$n>1$.
By (2.3) we have the following map of sets
Let ![]() $(X, h)$ be a primitively polarized irreducible holomorphic symplectic variety. Both
$(X, h)$ be a primitively polarized irreducible holomorphic symplectic variety. Both ![]() $h^{2}$ and the divisibility of
$h^{2}$ and the divisibility of ![]() $h$ are constant along deformations of
$h$ are constant along deformations of ![]() $(X, h)$. However, as shown in [Reference ApostolovApo14], it is not true that these two invariants determine the deformation type of
$(X, h)$. However, as shown in [Reference ApostolovApo14], it is not true that these two invariants determine the deformation type of ![]() $(X, h)$. In this section, we give explicit representatives for all the deformation-equivalence classes of primitively polarized varieties of
$(X, h)$. In this section, we give explicit representatives for all the deformation-equivalence classes of primitively polarized varieties of ![]() $K3^{[n]}$-type.
$K3^{[n]}$-type.
We start by describing results due to Markman on deformation-equivalence of polarized varieties of ![]() $K3^{[n]}$-type. These results rely both on the global Torelli theorem [Reference VerbitskyVer13] and the computation of the monodromy group of varieties of
$K3^{[n]}$-type. These results rely both on the global Torelli theorem [Reference VerbitskyVer13] and the computation of the monodromy group of varieties of ![]() $K3^{[n]}$-type in [Reference MarkmanMar10]. Recently, Kreck and Su [Reference Kreck and SuKS21] provided a counterexample to some of the statements contained in Verbitsky's global Torelli theorem. However, this does not affect the results we are using, as they rely on Markman's formulation of the global Torelli theorem using marked moduli spaces instead of the Teichmuller space used by Verbitsky. We refer the reader to [Reference LooijengaLoo21, Theorem 3.1 and Remark 3.3] for the correct statement and a comment about the difference between the Teichmuller space and marked moduli spaces with respect to the global Torelli theorem (see also [Reference VerbitskyVer20]).
$K3^{[n]}$-type in [Reference MarkmanMar10]. Recently, Kreck and Su [Reference Kreck and SuKS21] provided a counterexample to some of the statements contained in Verbitsky's global Torelli theorem. However, this does not affect the results we are using, as they rely on Markman's formulation of the global Torelli theorem using marked moduli spaces instead of the Teichmuller space used by Verbitsky. We refer the reader to [Reference LooijengaLoo21, Theorem 3.1 and Remark 3.3] for the correct statement and a comment about the difference between the Teichmuller space and marked moduli spaces with respect to the global Torelli theorem (see also [Reference VerbitskyVer20]).
Let ![]() $S$ be a
$S$ be a ![]() $K3$ surface, and
$K3$ surface, and ![]() $n>1$ an integer. Let
$n>1$ an integer. Let ![]() $\tilde \Lambda$ be the Mukai lattice of
$\tilde \Lambda$ be the Mukai lattice of ![]() $S$
$S$
endowed with the quadratic form defined by
Let ![]() $v_n=(1, 0, 1-n)$. We identify
$v_n=(1, 0, 1-n)$. We identify ![]() $H^{2}(S^{[n]}, {{\mathbb {Z}}})$ endowed with the Beauville–Bogomolov quadratic form with the orthogonal of
$H^{2}(S^{[n]}, {{\mathbb {Z}}})$ endowed with the Beauville–Bogomolov quadratic form with the orthogonal of ![]() $v_n$ in
$v_n$ in ![]() $\tilde \Lambda$. The inclusion
$\tilde \Lambda$. The inclusion
is compatible with the decomposition (2.5).
If ![]() $h$ is any class in
$h$ is any class in ![]() $H^{2}(S^{[n]}, {{\mathbb {Z}}})\subset \tilde \Lambda$, let
$H^{2}(S^{[n]}, {{\mathbb {Z}}})\subset \tilde \Lambda$, let ![]() $T_S(h)$ be the saturation in
$T_S(h)$ be the saturation in ![]() $\tilde \Lambda$ of the lattice spanned by
$\tilde \Lambda$ of the lattice spanned by ![]() $h$ and
$h$ and ![]() $v_n$.
$v_n$.
Proposition 2.4 Let ![]() $S$ and
$S$ and ![]() $S'$ be two
$S'$ be two ![]() $K3$ surfaces, and let
$K3$ surfaces, and let ![]() $n>1$ be an integer. Let
$n>1$ be an integer. Let ![]() $h$ (respectively,
$h$ (respectively, ![]() $h'$) be the numerical equivalence class of a big line bundle on
$h'$) be the numerical equivalence class of a big line bundle on ![]() $S^{[n]}$ (respectively,
$S^{[n]}$ (respectively, ![]() $S'^{[n]}$). The pairs
$S'^{[n]}$). The pairs ![]() $(S^{[n]}, h)$ and
$(S^{[n]}, h)$ and ![]() $(S'^{[n]}, h')$ are deformation equivalent if and only if there exists an isometry
$(S'^{[n]}, h')$ are deformation equivalent if and only if there exists an isometry
mapping ![]() $h$ to
$h$ to ![]() $h'$.
$h'$.
Proof. In case ![]() $h$ and
$h$ and ![]() $h'$ are ample, this is the result of Markman written up in [Reference ApostolovApo14, Proposition 1.6].
$h'$ are ample, this is the result of Markman written up in [Reference ApostolovApo14, Proposition 1.6].
In the general case, choose small deformations ![]() $(X, h)$ and
$(X, h)$ and ![]() $(X', h')$ of
$(X', h')$ of ![]() $(S^{[n]}, h)$ and
$(S^{[n]}, h)$ and ![]() $(S'^{[n]}, h')$, respectively, such that both
$(S'^{[n]}, h')$, respectively, such that both ![]() $X$ and
$X$ and ![]() $X'$ have Picard number
$X'$ have Picard number ![]() $1$. By a theorem of Huybrechts [Reference HuybrechtsHuy99],
$1$. By a theorem of Huybrechts [Reference HuybrechtsHuy99], ![]() $h$ and
$h$ and ![]() $h'$ are ample classes on
$h'$ are ample classes on ![]() $X$ and
$X$ and ![]() $X'$, respectively.
$X'$, respectively.
The construction of the rank ![]() $2$ lattices
$2$ lattices ![]() $T_S(h)$ and
$T_S(h)$ and ![]() $T_{S'}(h')$ generalizes to
$T_{S'}(h')$ generalizes to ![]() $X$ and
$X$ and ![]() $X'$ to provide rank
$X'$ to provide rank ![]() $2$ lattices
$2$ lattices ![]() $T_X(h)$ and
$T_X(h)$ and ![]() $T_{X'}(h')$. This follows from the work of Markman as in [Reference MarkmanMar11], Corollary 9.5. We refer to [Reference MarkmanMar11] and the discussion in [Reference ApostolovApo14], § 1 for the precise construction.
$T_{X'}(h')$. This follows from the work of Markman as in [Reference MarkmanMar11], Corollary 9.5. We refer to [Reference MarkmanMar11] and the discussion in [Reference ApostolovApo14], § 1 for the precise construction.
The formation of ![]() $T_X(h)$ is compatible with parallel transport. As a consequence, the isomorphism
$T_X(h)$ is compatible with parallel transport. As a consequence, the isomorphism ![]() $T_S(h)\rightarrow T_{S'}(h')$ mapping
$T_S(h)\rightarrow T_{S'}(h')$ mapping ![]() $h$ to
$h$ to ![]() $h'$ induces an isomorphism
$h'$ induces an isomorphism ![]() $T_X(h)\rightarrow T_{X'}(h')$ mapping
$T_X(h)\rightarrow T_{X'}(h')$ mapping ![]() $h$ to
$h$ to ![]() $h'$. It follows once again from [Reference ApostolovApo14, Proposition 1.6], that
$h'$. It follows once again from [Reference ApostolovApo14, Proposition 1.6], that ![]() $(X, h)$ and
$(X, h)$ and ![]() $(X', h')$ are deformation equivalent.
$(X', h')$ are deformation equivalent.
We now state the main result of this section.
Theorem 2.5 Let ![]() $n>1$ be an integer and let
$n>1$ be an integer and let ![]() $(X, h)$ be a primitively polarized irreducible holomorphic symplectic variety of
$(X, h)$ be a primitively polarized irreducible holomorphic symplectic variety of ![]() $K3^{[n]}$-type. Let
$K3^{[n]}$-type. Let ![]() $t$ be the divisibility of
$t$ be the divisibility of ![]() $h$, and let
$h$, and let ![]() $I\subset {{\mathbb {Z}}}$ be a system of representatives of
$I\subset {{\mathbb {Z}}}$ be a system of representatives of ![]() ${{\mathbb {Z}}}/t{{\mathbb {Z}}}$, up to the action of
${{\mathbb {Z}}}/t{{\mathbb {Z}}}$, up to the action of ![]() $-1$ on
$-1$ on ![]() ${{\mathbb {Z}}}/t{{\mathbb {Z}}}$. Then
${{\mathbb {Z}}}/t{{\mathbb {Z}}}$. Then ![]() $t$ divides
$t$ divides ![]() $2n-2$ and there exists a
$2n-2$ and there exists a ![]() $K3$ surface
$K3$ surface ![]() $S$, a primitive polarization
$S$, a primitive polarization ![]() $h_S$ on
$h_S$ on ![]() $S$ and an integer
$S$ and an integer ![]() $\mu \in I$ such that the pair
$\mu \in I$ such that the pair ![]() $(X, h)$ is deformation equivalent to
$(X, h)$ is deformation equivalent to ![]() $(S^{[n]}, th_S-\mu \delta _n)$.
$(S^{[n]}, th_S-\mu \delta _n)$.
Remark 2.6 The class ![]() $th_S-\mu \delta _n$ is not ample in general. However, the argument of the proof of Proposition 2.4 shows that it is big.
$th_S-\mu \delta _n$ is not ample in general. However, the argument of the proof of Proposition 2.4 shows that it is big.
Proof. Remark 2.3 allows us to assume that ![]() $X=S^{[n]}$ for some
$X=S^{[n]}$ for some ![]() $K3$ surface
$K3$ surface ![]() $S$. Let
$S$. Let ![]() $\tilde \Lambda =H^{0}(S, {{\mathbb {Z}}})\oplus H^{2}(S, {{\mathbb {Z}}})\oplus H^{4}(S, {{\mathbb {Z}}})$ be the Mukai lattice of
$\tilde \Lambda =H^{0}(S, {{\mathbb {Z}}})\oplus H^{2}(S, {{\mathbb {Z}}})\oplus H^{4}(S, {{\mathbb {Z}}})$ be the Mukai lattice of ![]() $S$ and let
$S$ and let ![]() $v_n=(1, 0, 1-n).$
$v_n=(1, 0, 1-n).$
Write ![]() $2d$ and
$2d$ and ![]() $t$ for the Beauville–Bogomolov square and the divisibility of
$t$ for the Beauville–Bogomolov square and the divisibility of ![]() $h$, respectively. We start by describing the structure of the lattice
$h$, respectively. We start by describing the structure of the lattice ![]() $T_{S}(h)$.
$T_{S}(h)$.
Write
where ![]() $\lambda$ and
$\lambda$ and ![]() $\mu$ are two integers and
$\mu$ are two integers and ![]() $h_S$ is primitive. As
$h_S$ is primitive. As ![]() $h$ is primitive,
$h$ is primitive, ![]() $\lambda$ and
$\lambda$ and ![]() $\mu$ are relatively prime. It is readily checked that the divisibility of
$\mu$ are relatively prime. It is readily checked that the divisibility of ![]() $h$ is
$h$ is
and that ![]() $\mu$ and
$\mu$ and ![]() $t$ are relatively prime.
$t$ are relatively prime.
The element
belongs to ![]() $T_{S}(h)$, and the computation of [Reference ApostolovApo14, Proposition 2.2] show that
$T_{S}(h)$, and the computation of [Reference ApostolovApo14, Proposition 2.2] show that ![]() $w$ generates the group
$w$ generates the group ![]() $T_{S}(h)/({{\mathbb {Z}}}h\oplus {{\mathbb {Z}}}v_n)\simeq {{\mathbb {Z}}}/t{{\mathbb {Z}}}.$
$T_{S}(h)/({{\mathbb {Z}}}h\oplus {{\mathbb {Z}}}v_n)\simeq {{\mathbb {Z}}}/t{{\mathbb {Z}}}.$
The lattice ![]() $N$ spanned by
$N$ spanned by ![]() $h$ and
$h$ and ![]() $v_n$ in
$v_n$ in ![]() $\tilde \Lambda$ is isomorphic to
$\tilde \Lambda$ is isomorphic to ![]() $\langle 2d\rangle \oplus \langle 2n-2\rangle$. Its discriminant group
$\langle 2d\rangle \oplus \langle 2n-2\rangle$. Its discriminant group ![]() $N^{\vee }/N$ is
$N^{\vee }/N$ is ![]() ${{\mathbb {Z}}}/2d{{\mathbb {Z}}}\oplus {{\mathbb {Z}}}/(2n-2){{\mathbb {Z}}}$.
${{\mathbb {Z}}}/2d{{\mathbb {Z}}}\oplus {{\mathbb {Z}}}/(2n-2){{\mathbb {Z}}}$.
As in paragraph 4 of [Reference NikulinNik79] and under the identifications given previously, the inclusion
induces an injective morphism
By (2.8), ![]() $\varphi$ sends
$\varphi$ sends ![]() $1$ to
$1$ to ![]() $(2d/t, \mu (2n-2)/t)$.
$(2d/t, \mu (2n-2)/t)$.
Let ![]() $S'$ be a
$S'$ be a ![]() $K3$ surface with a primitive polarization
$K3$ surface with a primitive polarization ![]() $h_{S'}$. Let
$h_{S'}$. Let ![]() $\mu '$ be an arbitrary integer, and define
$\mu '$ be an arbitrary integer, and define
By [Reference NikulinNik79, Proposition 1.4.2] and the discussion given previously, a sufficient condition ensuring that there exists an isomorphism of lattices ![]() $T_{S}(h)\rightarrow T_{S'}(h')$ sending
$T_{S}(h)\rightarrow T_{S'}(h')$ sending ![]() $h$ to
$h$ to ![]() $h'$ is that
$h'$ is that
and
that is,
Now let ![]() $\mu '$ be an integer in
$\mu '$ be an integer in ![]() $I$ such that
$I$ such that
Then ![]() $\mu '$ is prime to
$\mu '$ is prime to ![]() $t$ because
$t$ because ![]() $\mu$ is. Furthermore,
$\mu$ is. Furthermore, ![]() $\lambda$ is divisible by
$\lambda$ is divisible by ![]() $t$ and
$t$ and ![]() $h_S^{2}$ is even, so that
$h_S^{2}$ is even, so that ![]() $\lambda ^{2}h_S^{2}$ is divisible by
$\lambda ^{2}h_S^{2}$ is divisible by ![]() $2t^{2}$. In addition,
$2t^{2}$. In addition, ![]() $(\mu ^{2}-\mu '^{2})(2n-2)$ is divisible by
$(\mu ^{2}-\mu '^{2})(2n-2)$ is divisible by ![]() $2t^{2}$: both
$2t^{2}$: both ![]() $(\mu ^{2}-\mu '^{2})$ and
$(\mu ^{2}-\mu '^{2})$ and ![]() $2n-2$ are divisible by
$2n-2$ are divisible by ![]() $t$, and at least one of these terms is divisible by
$t$, and at least one of these terms is divisible by ![]() $2t$, depending on the parity of
$2t$, depending on the parity of ![]() $t$. As a consequence, the integer
$t$. As a consequence, the integer
is divisible by ![]() $2t^{2}$. Write
$2t^{2}$. Write
and let ![]() $S'$ be a
$S'$ be a ![]() $K3$ surface with a primitive polarization
$K3$ surface with a primitive polarization ![]() $h_{S'}$ of degree
$h_{S'}$ of degree ![]() $2d'$.
$2d'$.
By construction, there exists an isomorphism of lattices ![]() $T_{S}(h)\rightarrow T_{S'}(h')$ sending
$T_{S}(h)\rightarrow T_{S'}(h')$ sending ![]() $h$ to
$h$ to ![]() $h'$. Proposition 2.4 shows that
$h'$. Proposition 2.4 shows that ![]() $(S^{[n]}, h)$ and
$(S^{[n]}, h)$ and ![]() $(S'^{[n]}, th_{S'}-\mu '\delta _n)$ are deformation equivalent.
$(S'^{[n]}, th_{S'}-\mu '\delta _n)$ are deformation equivalent.
The following is an immediate consequence of the theorem.
Corollary 2.7 Let ![]() $n>1$ be an integer and let
$n>1$ be an integer and let ![]() $(X, h)$ be a primitively polarized irreducible holomorphic symplectic variety of
$(X, h)$ be a primitively polarized irreducible holomorphic symplectic variety of ![]() $K3^{[n]}$-type. Let
$K3^{[n]}$-type. Let ![]() $I\subset {{\mathbb {Z}}}$ be a system of representatives of
$I\subset {{\mathbb {Z}}}$ be a system of representatives of ![]() ${{\mathbb {Z}}}/(2n-2){{\mathbb {Z}}}$, up to the action of
${{\mathbb {Z}}}/(2n-2){{\mathbb {Z}}}$, up to the action of ![]() $-1$ on
$-1$ on ![]() ${{\mathbb {Z}}}/(2n-2){{\mathbb {Z}}}$. Then there exists a positive integer
${{\mathbb {Z}}}/(2n-2){{\mathbb {Z}}}$. Then there exists a positive integer ![]() $m$, a
$m$, a ![]() $K3$ surface
$K3$ surface ![]() $S$, a primitive polarization
$S$, a primitive polarization ![]() $h_S$ on
$h_S$ on ![]() $S$ and an integer
$S$ and an integer ![]() $\mu \in I$ such that the pair
$\mu \in I$ such that the pair ![]() $(X, mh)$ is deformation equivalent to
$(X, mh)$ is deformation equivalent to ![]() $(S^{[n]}, (2n-2)h_S-\mu \delta _n)$.
$(S^{[n]}, (2n-2)h_S-\mu \delta _n)$.
We use the dual statement on curve classes.
Corollary 2.8 Let ![]() $n>1$ be an integer and let
$n>1$ be an integer and let ![]() $X$ be a primitively polarized irreducible holomorphic symplectic variety of
$X$ be a primitively polarized irreducible holomorphic symplectic variety of ![]() $K3^{[n]}$-type. Let
$K3^{[n]}$-type. Let ![]() $C\in H_2(X,{{\mathbb {Z}}})$ be a primitive curve class with positive square.
$C\in H_2(X,{{\mathbb {Z}}})$ be a primitive curve class with positive square.
Then there exists a ![]() $K3$ surface
$K3$ surface ![]() $S$, a primitive polarization
$S$, a primitive polarization ![]() $h_S$ on
$h_S$ on ![]() $S$ and an integer
$S$ and an integer ![]() $\mu \in [0, n-1)$ such that there exists a polarized deformation from
$\mu \in [0, n-1)$ such that there exists a polarized deformation from ![]() $X$ to
$X$ to ![]() $S^{[n]}$, carrying
$S^{[n]}$, carrying ![]() $C$ to
$C$ to ![]() $h_S-\mu r_n$.
$h_S-\mu r_n$.
Proof. Following (2.2) and (2.3) we write ![]() $C=h/{\rm div}(h)$, for some primitive and positive
$C=h/{\rm div}(h)$, for some primitive and positive ![]() $h\in H^{2}(X,{{\mathbb {Z}}})$. As in the proof of Proposition 2.4 we may assume that
$h\in H^{2}(X,{{\mathbb {Z}}})$. As in the proof of Proposition 2.4 we may assume that ![]() $h$ is ample. By Corollary 2.7 there exists a polarized
$h$ is ample. By Corollary 2.7 there exists a polarized ![]() $K3$ surface
$K3$ surface ![]() $(S,h_S)$ and an integer
$(S,h_S)$ and an integer ![]() $\mu \in [0, n-1)$ such that the pair
$\mu \in [0, n-1)$ such that the pair ![]() $(X, mh)$ is deformation equivalent to
$(X, mh)$ is deformation equivalent to ![]() $(S^{[n]}, (2n-2)h_S-\mu \delta _n)$, for some integer
$(S^{[n]}, (2n-2)h_S-\mu \delta _n)$, for some integer ![]() $m>0$. The divisibility of
$m>0$. The divisibility of ![]() $(2n-2)h_S-\mu \delta _n$ equals
$(2n-2)h_S-\mu \delta _n$ equals ![]() $2n-2$. Therefore, via the map (2.7), we obtain
$2n-2$. Therefore, via the map (2.7), we obtain
This class yields the desired parallel transport of ![]() $C$.
$C$.
Remark 2.9 The proofs of the previous two corollaries work for any choice of a system of representatives of ![]() ${{\mathbb {Z}}}/(2n-2){{\mathbb {Z}}}$, up to the action of
${{\mathbb {Z}}}/(2n-2){{\mathbb {Z}}}$, up to the action of ![]() $-1$ on
$-1$ on ![]() ${{\mathbb {Z}}}/(2n-2){{\mathbb {Z}}}$.
${{\mathbb {Z}}}/(2n-2){{\mathbb {Z}}}$.
3. Deforming rational curves
Let ![]() $\pi : \mathscr{X}\rightarrow B$ be a smooth projective morphism of complex quasi-projective varieties of relative dimension
$\pi : \mathscr{X}\rightarrow B$ be a smooth projective morphism of complex quasi-projective varieties of relative dimension ![]() $d$, and let
$d$, and let ![]() $\alpha$ be a global section of Hodge type
$\alpha$ be a global section of Hodge type ![]() $(d-1,d-1)$ of the local system
$(d-1,d-1)$ of the local system ![]() $R^{2d-2}\pi _*{{\mathbb {Z}}}$. Fixing such a section
$R^{2d-2}\pi _*{{\mathbb {Z}}}$. Fixing such a section ![]() $\alpha$, we can consider the relative Kontsevich moduli stack of genus zero stable curves
$\alpha$, we can consider the relative Kontsevich moduli stack of genus zero stable curves ![]() $\overline {{\mathscr{M}_0}}(\mathscr{X}/B, \alpha )$. We refer the reader to [Reference Behrend and ManinBM96, Reference Fulton and PandharipandeFP97, Reference Abramovich and VistoliAV02] for details and constructions.
$\overline {{\mathscr{M}_0}}(\mathscr{X}/B, \alpha )$. We refer the reader to [Reference Behrend and ManinBM96, Reference Fulton and PandharipandeFP97, Reference Abramovich and VistoliAV02] for details and constructions.
The space ![]() $\overline {{\mathscr{M}_0}}(\mathscr{X}/B, \alpha )$ parametrizes maps
$\overline {{\mathscr{M}_0}}(\mathscr{X}/B, \alpha )$ parametrizes maps ![]() $f : C\rightarrow X$ from genus zero stable curves to fibers
$f : C\rightarrow X$ from genus zero stable curves to fibers ![]() $X=\mathscr{X}_b$ of
$X=\mathscr{X}_b$ of ![]() $\pi$ such that
$\pi$ such that ![]() $f_*[C]=\alpha _b$. The map
$f_*[C]=\alpha _b$. The map ![]() $\overline {{\mathscr{M}_0}}(\mathscr{X}/B, \alpha )\rightarrow B$ is proper. If
$\overline {{\mathscr{M}_0}}(\mathscr{X}/B, \alpha )\rightarrow B$ is proper. If ![]() $f$ is a stable map, we denote by
$f$ is a stable map, we denote by ![]() $[f]$ the corresponding point of the Kontsevich moduli stack.
$[f]$ the corresponding point of the Kontsevich moduli stack.
For the remainder of this section, let ![]() $X$ be a smooth projective irreducible holomorphic symplectic variety of dimension
$X$ be a smooth projective irreducible holomorphic symplectic variety of dimension ![]() $2n$ and let
$2n$ and let ![]() $f : C\rightarrow X$ be a map from a stable curve
$f : C\rightarrow X$ be a map from a stable curve ![]() $C$ of genus zero to
$C$ of genus zero to ![]() $X$. We assume furthermore
$X$. We assume furthermore ![]() $f$ is unramified at the generic point of each irreducible component of
$f$ is unramified at the generic point of each irreducible component of ![]() $C$. Let
$C$. Let ![]() $\mathscr{X}\rightarrow B$ be a smooth projective morphism of smooth connected quasi-projective varieties and let
$\mathscr{X}\rightarrow B$ be a smooth projective morphism of smooth connected quasi-projective varieties and let ![]() $0$ be a point of
$0$ be a point of ![]() $B$ such that
$B$ such that ![]() $\mathscr{X}_0=X$. Let
$\mathscr{X}_0=X$. Let ![]() $\mathcal \alpha$ be a global section of Hodge type
$\mathcal \alpha$ be a global section of Hodge type ![]() $(2n-1,2n-1)$ of
$(2n-1,2n-1)$ of ![]() $R^{4n-2}\pi _*{{\mathbb {Z}}}$ such that
$R^{4n-2}\pi _*{{\mathbb {Z}}}$ such that ![]() $\alpha _0=f_*[C]$ in
$\alpha _0=f_*[C]$ in ![]() $H^{4n-2}(X, {{\mathbb {Z}}})$.
$H^{4n-2}(X, {{\mathbb {Z}}})$.
Proposition 3.1 Let ![]() $M$ be an irreducible component of
$M$ be an irreducible component of ![]() $\overline {M_0}(X, f_*[C])$ containing
$\overline {M_0}(X, f_*[C])$ containing ![]() $[f]$. Then the following hold:
$[f]$. Then the following hold:
(1) the stack
 $M$ has dimension at least
$M$ has dimension at least  $2n-2$;
$2n-2$;(2) if
 $M$ has dimension
$M$ has dimension  $2n-2$, then any irreducible component of the Kontsevich moduli stack
$2n-2$, then any irreducible component of the Kontsevich moduli stack  $\overline {{\mathscr{M}_0}}(\mathscr{X}/B, \alpha )$ that contains
$\overline {{\mathscr{M}_0}}(\mathscr{X}/B, \alpha )$ that contains  $M$ dominates
$M$ dominates  $B$.
$B$.
In other words, when assumption (2) holds, the stable map ![]() $f : C\rightarrow X$ deforms over a finite cover of
$f : C\rightarrow X$ deforms over a finite cover of ![]() $B$. Related results have been obtained by Ran, see, for instance, Example 5.2 of [Reference RanRan95]. See also [Reference Amerik and VerbitskyAV15, Theorem 4.1] for an alternative proof.
$B$. Related results have been obtained by Ran, see, for instance, Example 5.2 of [Reference RanRan95]. See also [Reference Amerik and VerbitskyAV15, Theorem 4.1] for an alternative proof.
Proof. Let ![]() $\tilde {\mathscr{X}}\rightarrow S$ be a local universal family of deformations of
$\tilde {\mathscr{X}}\rightarrow S$ be a local universal family of deformations of ![]() $X$ such that
$X$ such that ![]() $B$ is the Noether–Lefschetz locus associated to
$B$ is the Noether–Lefschetz locus associated to ![]() $f_*[C]$ in
$f_*[C]$ in ![]() $S$. In particular,
$S$. In particular, ![]() $B$ is a smooth divisor in
$B$ is a smooth divisor in ![]() $S$.
$S$.
Lemma 11 of [Reference Bogomolov, Hassett and TschinkelBHT11] and our hypothesis on ![]() $f$ show that we have an isomorphism
$f$ show that we have an isomorphism
in the derived category of coherent sheaves on ![]() $C$, for some coherent sheaf
$C$, for some coherent sheaf ![]() $N_f$. As a consequence, standard deformation theory shows that any component of the deformation space of the stable map
$N_f$. As a consequence, standard deformation theory shows that any component of the deformation space of the stable map ![]() $f$ over
$f$ over ![]() $S$ has dimension at least
$S$ has dimension at least
the latter equality following from the Riemann–Roch theorem on ![]() $C$ and the triviality of the canonical bundle of
$C$ and the triviality of the canonical bundle of ![]() $X$.
$X$.
As the image in ![]() $S$ of any component of the deformation space of the stable map
$S$ of any component of the deformation space of the stable map ![]() $f$ is contained in
$f$ is contained in ![]() $B$, the fibers of such a component all have dimension at least
$B$, the fibers of such a component all have dimension at least ![]() $2n-2$. If any fiber has dimension
$2n-2$. If any fiber has dimension ![]() $2n-2$, it also follows that the corresponding component has to dominate
$2n-2$, it also follows that the corresponding component has to dominate ![]() $B$, which shows the result.
$B$, which shows the result.
This result holds for any smooth projective variety ![]() $X$ with trivial canonical bundle. In order to use it, we need to study the locus spanned by a family of rational curves. The following gives a strong restriction on this locus, and makes crucial use of the symplectic form on
$X$ with trivial canonical bundle. In order to use it, we need to study the locus spanned by a family of rational curves. The following gives a strong restriction on this locus, and makes crucial use of the symplectic form on ![]() $X$.
$X$.
Proposition 3.2 Let ![]() $X$ be a projective manifold of dimension
$X$ be a projective manifold of dimension ![]() $2n$ endowed with a symplectic form, and let
$2n$ endowed with a symplectic form, and let ![]() $Y$ be a closed subvariety of codimension
$Y$ be a closed subvariety of codimension ![]() $k$ of
$k$ of ![]() $X$. Let
$X$. Let ![]() $W\subset X$ be a subvariety such that any point of
$W\subset X$ be a subvariety such that any point of ![]() $Y$ is rationally equivalent to a point of
$Y$ is rationally equivalent to a point of ![]() $W$. Then the codimension of
$W$. Then the codimension of ![]() $W$ is at most
$W$ is at most ![]() $2k$.
$2k$.
For a similar result, see [Reference Amerik and VerbitskyAV15, Theorem 4.4].
Before proving the proposition, we first record the following fact from linear algebra.
Lemma 3.3 Let ![]() $(F,\omega )$ be a symplectic vector space of dimension
$(F,\omega )$ be a symplectic vector space of dimension ![]() $2n$ and
$2n$ and ![]() $V$ a subspace of codimension
$V$ a subspace of codimension ![]() $k$ of
$k$ of ![]() $F$. Then
$F$. Then ![]() $V$ contains a subspace
$V$ contains a subspace ![]() $V'$ of codimension at most
$V'$ of codimension at most ![]() $2k$ in
$2k$ in ![]() $F$ such that the restriction
$F$ such that the restriction ![]() $\omega _{|V'}$ of the
$\omega _{|V'}$ of the ![]() $2$-form is symplectic on
$2$-form is symplectic on ![]() $V'$. In particular,
$V'$. In particular, ![]() $\omega ^{2n-2k}_{|V}\neq 0$.
$\omega ^{2n-2k}_{|V}\neq 0$.
Proof of Lemma 3.3 Let ![]() $V^{\perp }$ be the orthogonal to
$V^{\perp }$ be the orthogonal to ![]() $V$ with respect to the symplectic form
$V$ with respect to the symplectic form ![]() $\omega$. As
$\omega$. As ![]() $\omega$ is non-degenerate, we have
$\omega$ is non-degenerate, we have
which implies that ![]() $\dim (V\cap V^{\perp })\leq k$.
$\dim (V\cap V^{\perp })\leq k$.
Let ![]() $V'$ be a subspace of
$V'$ be a subspace of ![]() $V$ such that
$V$ such that ![]() $V'$ and
$V'$ and ![]() $V\cap V^{\perp }$ are in direct sum in
$V\cap V^{\perp }$ are in direct sum in ![]() $V$. Then
$V$. Then ![]() $\dim (V')\geq 2n-2k$. Furthermore, any
$\dim (V')\geq 2n-2k$. Furthermore, any ![]() $v\in V$ is orthogonal to
$v\in V$ is orthogonal to ![]() $V\cap V^{\perp }$, so that
$V\cap V^{\perp }$, so that ![]() $(V')^{\perp }=V^{\perp }$. As a consequence,
$(V')^{\perp }=V^{\perp }$. As a consequence, ![]() $V'\cap (V')^{\perp }=V'\cap V^{\perp }=0$, and the restriction of
$V'\cap (V')^{\perp }=V'\cap V^{\perp }=0$, and the restriction of ![]() $\omega$ to
$\omega$ to ![]() $V'$ is non-degenerate.
$V'$ is non-degenerate.
Let us recall the following result, proved by Voisin [Reference VoisinVoi16, Lemma 1.1] as an application of Mumford's theorem on ![]() $0$-cycles.
$0$-cycles.
Lemma 3.4 Let ![]() $f : Z\rightarrow X$ be a morphism between smooth projective varieties. Assume that there exists a surjective morphism
$f : Z\rightarrow X$ be a morphism between smooth projective varieties. Assume that there exists a surjective morphism ![]() $p : Z\rightarrow B$ to a smooth projective variety with the property that any two points of
$p : Z\rightarrow B$ to a smooth projective variety with the property that any two points of ![]() $Z$ with the same image under
$Z$ with the same image under ![]() $p$ are mapped by
$p$ are mapped by ![]() $f$ to rationally equivalent points of
$f$ to rationally equivalent points of ![]() $X$.
$X$.
Then for any holomorphic form ![]() $\eta$ on
$\eta$ on ![]() $X$ there exists a holomorphic form
$X$ there exists a holomorphic form ![]() $\eta _B$ on
$\eta _B$ on ![]() $B$ such that
$B$ such that
Proof of Proposition 3.2 Assume by contradiction that ![]() $W$ has dimension at most
$W$ has dimension at most ![]() $2n-2k-1$. We argue as in [Reference VoisinVoi16, Proof of Theorem 1.3]. If
$2n-2k-1$. We argue as in [Reference VoisinVoi16, Proof of Theorem 1.3]. If ![]() $w$ is any point of
$w$ is any point of ![]() $W$, define
$W$, define
where ![]() $\equiv _\textrm {rat}$ denotes rational equivalence. Then
$\equiv _\textrm {rat}$ denotes rational equivalence. Then ![]() $O_w$ is a countable union of subvarieties of
$O_w$ is a countable union of subvarieties of ![]() $X$, and a dimension count shows that for any
$X$, and a dimension count shows that for any ![]() $w$,
$w$, ![]() $O_w$ contains a component of dimension at least
$O_w$ contains a component of dimension at least ![]() $k+1$.
$k+1$.
By the countability of Hilbert schemes, there exists a generically finite cover ![]() $\varphi : B\to W$, a family
$\varphi : B\to W$, a family ![]() $f:Z\to B$ of varieties of dimension
$f:Z\to B$ of varieties of dimension ![]() $k+1$ and a morphism
$k+1$ and a morphism ![]() $f:Z\to X$ mapping every fiber of
$f:Z\to X$ mapping every fiber of ![]() $p$ generically finitely onto points that are all rationally equivalent in
$p$ generically finitely onto points that are all rationally equivalent in ![]() $X$. We can assume that
$X$. We can assume that ![]() $B$ and
$B$ and ![]() $Z$ are smooth and projective. Lemma 3.4 shows that for any holomorphic form
$Z$ are smooth and projective. Lemma 3.4 shows that for any holomorphic form ![]() $\eta$ on
$\eta$ on ![]() $X$ there exists a holomorphic form
$X$ there exists a holomorphic form ![]() $\eta _B$ on
$\eta _B$ on ![]() $B$ such that
$B$ such that
On the other hand, if ![]() $\omega$ is the symplectic form on
$\omega$ is the symplectic form on ![]() $X$, then, by Lemma 3.3, the
$X$, then, by Lemma 3.3, the ![]() $(2n-2k)$-holomorphic form
$(2n-2k)$-holomorphic form ![]() $\eta =\omega ^{n-k}$ verifies
$\eta =\omega ^{n-k}$ verifies
As ![]() $\dim (B)=\dim (W)<2n-2k$, the latter contradicts (3.1).
$\dim (B)=\dim (W)<2n-2k$, the latter contradicts (3.1).
These results allow us to give a simple criterion for the existence of uniruled divisors on polarized deformations of a given holomorphic symplectic variety ![]() $X$. For similar results, see [Reference Amerik and VerbitskyAV15, Corollaries 4.5 and 4.8].
$X$. For similar results, see [Reference Amerik and VerbitskyAV15, Corollaries 4.5 and 4.8].
Corollary 3.5 Let ![]() $f: C\to X$ be a genus zero stable curve in X, and let
$f: C\to X$ be a genus zero stable curve in X, and let ![]() $\alpha=f_*[C]$. Assume that f is ruling. Then the following holds.
$\alpha=f_*[C]$. Assume that f is ruling. Then the following holds.
(1) There exists an irreducible component of
 $\overline{\mathscr{M}_0}(\mathscr{X}/B, \alpha)$ containing
$\overline{\mathscr{M}_0}(\mathscr{X}/B, \alpha)$ containing  $[f]$ that dominates B. In particular, the stable map
$[f]$ that dominates B. In particular, the stable map  $[f]$ deforms over a finite cover of
$[f]$ deforms over a finite cover of  $B$.
$B$.(2) For any point b of B, the fiber
 $\mathscr{X}_b$ contains a uniruled codimension 1 subscheme D whose codimension 1 component of its cohomology class is a positive multiple of the Poincaré dual of
$\mathscr{X}_b$ contains a uniruled codimension 1 subscheme D whose codimension 1 component of its cohomology class is a positive multiple of the Poincaré dual of  $\alpha$, and such that D is ruled by a curve of class
$\alpha$, and such that D is ruled by a curve of class  $\alpha$.
$\alpha$.
Proof. Let M be an irreducible component of ![]() $\overline{M_0}(X,f_*[\mathbb{P}^1])$ containing
$\overline{M_0}(X,f_*[\mathbb{P}^1])$ containing ![]() $[f]$ such that, denoting by Y the subscheme of X covered by the deformations of f parametrized by M, Y is a divisor in X.
$[f]$ such that, denoting by Y the subscheme of X covered by the deformations of f parametrized by M, Y is a divisor in X.
Let ![]() $C \to M$ be the universal curve. By Proposition 3.1, the dimension of
$C \to M$ be the universal curve. By Proposition 3.1, the dimension of ![]() $M$ is at least
$M$ is at least ![]() $2n-2$. We claim that equality holds, which implies statement (1), again by Proposition 3.1. Assume by contradiction that
$2n-2$. We claim that equality holds, which implies statement (1), again by Proposition 3.1. Assume by contradiction that ![]() $\dim ( M)>2n-2$. As
$\dim ( M)>2n-2$. As ![]() $\dim (Y)=2n-1$, this implies that any fiber of the evaluation map
$\dim (Y)=2n-1$, this implies that any fiber of the evaluation map ![]() $C\to Y\subset X$ is at least one-dimensional, which, in turn, shows that there exists a subvariety
$C\to Y\subset X$ is at least one-dimensional, which, in turn, shows that there exists a subvariety ![]() $W\subset X$ of dimension at most
$W\subset X$ of dimension at most ![]() $\dim {Y}-2=2n-3$ such that any point of
$\dim {Y}-2=2n-3$ such that any point of ![]() $Y$ is rationally equivalent to a point in
$Y$ is rationally equivalent to a point in ![]() $W$. Proposition 3.2 provides the contradiction.
$W$. Proposition 3.2 provides the contradiction.
To show statement (2), it suffices to consider the case where B has dimension ![]() $1$ and passes through a very general point of the Noether–Lefschetz locus associated to
$1$ and passes through a very general point of the Noether–Lefschetz locus associated to ![]() $\alpha$. Let
$\alpha$. Let ![]() $\mathscr{M}$ be an irreducible component of
$\mathscr{M}$ be an irreducible component of ![]() $\overline{{\mathscr{M}_0}}(\mathscr{X}/B, \alpha)$ containing M. Then
$\overline{{\mathscr{M}_0}}(\mathscr{X}/B, \alpha)$ containing M. Then ![]() $\mathscr{M}$ dominates B. Let
$\mathscr{M}$ dominates B. Let ![]() $\mathscr{Y}\subset \mathscr{X}\rightarrow B$ be the locus in
$\mathscr{Y}\subset \mathscr{X}\rightarrow B$ be the locus in ![]() $\mathscr{X}$ covered by the deformations of f parametrized by
$\mathscr{X}$ covered by the deformations of f parametrized by ![]() $\mathscr{M}$. Since
$\mathscr{M}$. Since ![]() $\mathscr{M}$ dominates B, any irreducible component of
$\mathscr{M}$ dominates B, any irreducible component of ![]() $\mathscr{Y}$ dominates B. Since the fiber of
$\mathscr{Y}$ dominates B. Since the fiber of ![]() $\mathscr{Y}\rightarrow B$ over
$\mathscr{Y}\rightarrow B$ over ![]() $0$ is a divisor in
$0$ is a divisor in ![]() $\mathscr{X}_0=X$, the fiber of
$\mathscr{X}_0=X$, the fiber of ![]() $\mathscr{Y}\rightarrow B$ at any point b has codimension
$\mathscr{Y}\rightarrow B$ at any point b has codimension ![]() $1$.
$1$.
By construction, the irreducible codimension ![]() $1$ part of
$1$ part of ![]() $Y_b$ is ruled by a curve of class
$Y_b$ is ruled by a curve of class ![]() $\alpha$. Note that we cannot deduce that the class of the divisor
$\alpha$. Note that we cannot deduce that the class of the divisor ![]() $Y_b$ inside
$Y_b$ inside ![]() $\mathscr{X}_b$ equals that of Y because
$\mathscr{X}_b$ equals that of Y because ![]() $\mathscr{Y}_0$ contains Y and the inclusion could be proper.
$\mathscr{Y}_0$ contains Y and the inclusion could be proper.
Let b be very general in the Noether–Lefschetz locus. The Néron–Severi group of ![]() $\mathscr{X}_b$ has rank at most
$\mathscr{X}_b$ has rank at most ![]() $2$, and it is generated over
$2$, and it is generated over ![]() $\mathbb{Q}$ by the Poincaré dual
$\mathbb{Q}$ by the Poincaré dual ![]() $\alpha^\vee$ of
$\alpha^\vee$ of ![]() $\alpha$ and the class of the polarization. In particular, the codimension one component of the cohomology class of
$\alpha$ and the class of the polarization. In particular, the codimension one component of the cohomology class of ![]() $Y_b$ is a linear combination of
$Y_b$ is a linear combination of ![]() $\alpha^\vee$ and the class of the polarization. As a consequence, the same holds for
$\alpha^\vee$ and the class of the polarization. As a consequence, the same holds for ![]() $Y_0$. This holds for any choice of a polarization on X.
$Y_0$. This holds for any choice of a polarization on X.
First assume that ![]() $b_2(X)\geq 5$, so that
$b_2(X)\geq 5$, so that ![]() $h^{1,1}(X)\geq 3$. Assume that
$h^{1,1}(X)\geq 3$. Assume that ![]() $\alpha^\vee$ is not proportional to the codimension one component of the cohomology class of
$\alpha^\vee$ is not proportional to the codimension one component of the cohomology class of ![]() $Y_0$. Then the Picard number of
$Y_0$. Then the Picard number of ![]() $X_b$ is
$X_b$ is ![]() $2$ for general b, and we may choose B in such a way that there exists
$2$ for general b, and we may choose B in such a way that there exists ![]() $b'$ in B such that the Néron–Severi group of
$b'$ in B such that the Néron–Severi group of ![]() $NS(X_{b'})$ has rank at least
$NS(X_{b'})$ has rank at least ![]() $3$. We may replace X with
$3$. We may replace X with ![]() $X_{b'}$ and assume that NS(X) has rank at least
$X_{b'}$ and assume that NS(X) has rank at least ![]() $3$.
$3$.
Let h and ![]() $h'$ be two ample classes on X such that
$h'$ be two ample classes on X such that ![]() $\alpha^\vee, h$ and
$\alpha^\vee, h$ and ![]() $h'$ span a subspace of rank
$h'$ span a subspace of rank ![]() $3$ of NS(X). Then the codimension one component of the cohomology class of
$3$ of NS(X). Then the codimension one component of the cohomology class of ![]() $Y_0$ is both a linear combination of
$Y_0$ is both a linear combination of ![]() $\alpha^\vee$ and h and of
$\alpha^\vee$ and h and of ![]() $\alpha^\vee$ and
$\alpha^\vee$ and ![]() $h'$. As a consequence, it is proportional to
$h'$. As a consequence, it is proportional to ![]() $\alpha^\vee$.
$\alpha^\vee$.
To give a general argument that covers the case ![]() $b_2=4$, we may replace the moduli space of stable genus 0 curves – for which classical references require projectivity assumptions – with the (relative) Douady space parametrizing rational curves, and work with non projective manifolds as well. In this framework, we may deform X with the class
$b_2=4$, we may replace the moduli space of stable genus 0 curves – for which classical references require projectivity assumptions – with the (relative) Douady space parametrizing rational curves, and work with non projective manifolds as well. In this framework, we may deform X with the class ![]() $\alpha$ and the uniruled codimension one subscheme under consideration to an irreducible holomorphic symplectic variety with Néron–Severi group generated by
$\alpha$ and the uniruled codimension one subscheme under consideration to an irreducible holomorphic symplectic variety with Néron–Severi group generated by ![]() $\alpha^\vee$, which proves the proportionality result.
$\alpha^\vee$, which proves the proportionality result.
Knowing that the cohomology class of the codimension 1 component ![]() $Y_b$ is proportional to the Poincaré dual of
$Y_b$ is proportional to the Poincaré dual of ![]() $\alpha$, let
$\alpha$, let ![]() $\lambda$ be the rational number such that
$\lambda$ be the rational number such that
Then ![]() $\lambda$ is independent of b. To prove that
$\lambda$ is independent of b. To prove that ![]() $\lambda$ is positive, we may assume that
$\lambda$ is positive, we may assume that ![]() $X_b$ is projective by choosing a suitable deformation of the pair
$X_b$ is projective by choosing a suitable deformation of the pair ![]() $(X, \alpha)$. Let H be an ample class on
$(X, \alpha)$. Let H be an ample class on ![]() $X_b$. Then
$X_b$. Then ![]() $q(H, Y_b)>0$ and
$q(H, Y_b)>0$ and ![]() $H\alpha>0$, so that the coefficient of proportionality is positive.
$H\alpha>0$, so that the coefficient of proportionality is positive.
4. Examples, proof of Theorem 1.2 and further remarks
In this section, we use the notation and the basic facts recalled in § 2.1. We start by constructing examples of uniruled divisors using the classical Brill–Noether theory applied to the desingularizations of curves on a ![]() $K3$ surface. Then we present the proof of Theorem 1.2. We also compare conditions (i) and (ii) in Remark 1.4 with the condition appearing in [Reference Oberdieck, Shen and YinOSY19, Corollary A.3]. Finally, we show the existence of codimension 2 coisotropic subvarieties on projective holomorphic symplectic manifold of
$K3$ surface. Then we present the proof of Theorem 1.2. We also compare conditions (i) and (ii) in Remark 1.4 with the condition appearing in [Reference Oberdieck, Shen and YinOSY19, Corollary A.3]. Finally, we show the existence of codimension 2 coisotropic subvarieties on projective holomorphic symplectic manifold of ![]() $K3^{[n]}$-type in some of the cases where Theorem 1.2 does not provide uniruled divisors.
$K3^{[n]}$-type in some of the cases where Theorem 1.2 does not provide uniruled divisors.
4.1 Examples
Let ![]() $n$ be a positive integer, and let
$n$ be a positive integer, and let ![]() $k$ be an integer between
$k$ be an integer between ![]() $1$ and
$1$ and ![]() $n$. Write
$n$. Write ![]() $g=k-1$. Let
$g=k-1$. Let ![]() $(S,H)$ be a general polarized
$(S,H)$ be a general polarized ![]() $K3$ surface,
$K3$ surface, ![]() $m>0$ an integer and
$m>0$ an integer and ![]() $L:=mH$ with
$L:=mH$ with ![]() $p_a(L):=p\geq g$. Consider the Severi variety parametrizing nodal genus
$p_a(L):=p\geq g$. Consider the Severi variety parametrizing nodal genus ![]() $g$ curves inside
$g$ curves inside ![]() $|L|$. It is a locally closed subvariety of
$|L|$. It is a locally closed subvariety of ![]() $|L|$ (see, e.g., [Reference SernesiSer06] for the basic facts on Severi varieties).
$|L|$ (see, e.g., [Reference SernesiSer06] for the basic facts on Severi varieties).
Recall that the Severi variety on regular surfaces has the expected codimension (equal to the number of nodes) whenever non-empty (see, e.g., [Reference Chiantini and SernesiCS97, Example 1.3]). In the case of multiples of the hyperplane section on a general ![]() $K3$ surface non-emptiness has been shown by [Reference ChenChe02] (see also [Reference Galati and KnutsenGK14] for another proof and a generalization). The case
$K3$ surface non-emptiness has been shown by [Reference ChenChe02] (see also [Reference Galati and KnutsenGK14] for another proof and a generalization). The case ![]() $m=1$ corresponds to the ‘classical’ Bogomolov–Mumford theorem (see, e.g., [Reference Barth, Hulek, Peters and Van de VenBHPV04, Ch. VIII, § 23]).
$m=1$ corresponds to the ‘classical’ Bogomolov–Mumford theorem (see, e.g., [Reference Barth, Hulek, Peters and Van de VenBHPV04, Ch. VIII, § 23]).
Consider a ![]() $g$-dimensional family
$g$-dimensional family ![]() $\mathscr {C}_T\to T,\ T\subset |L|$, of curves of geometric genus
$\mathscr {C}_T\to T,\ T\subset |L|$, of curves of geometric genus ![]() $g$ whose general member is a nodal curve. Hence,
$g$ whose general member is a nodal curve. Hence, ![]() $T$ is given by an irreducible component of the Severi variety parametrizing curves with
$T$ is given by an irreducible component of the Severi variety parametrizing curves with ![]() $(p-g)$-nodes inside
$(p-g)$-nodes inside ![]() $|L|$.
$|L|$.
It is well known that the relative symmetric product ![]() ${\mathscr {C}}_T^{(g+1)}$ is uniruled. Indeed, for each
${\mathscr {C}}_T^{(g+1)}$ is uniruled. Indeed, for each ![]() $t\in T$ the symmetric product
$t\in T$ the symmetric product ![]() $C_t^{(g+1)}$ is uniruled as the Abel–Jacobi morphism
$C_t^{(g+1)}$ is uniruled as the Abel–Jacobi morphism ![]() $AJ_{g+1}: \tilde C_t^{(g+1)}\to \textrm {Pic}^{g+1} (\tilde C_t)$ onto the
$AJ_{g+1}: \tilde C_t^{(g+1)}\to \textrm {Pic}^{g+1} (\tilde C_t)$ onto the ![]() $g$-dimensional Picard variety of the desingularization
$g$-dimensional Picard variety of the desingularization ![]() $\tilde C_t\to C_t$ has fibers isomorphic to
$\tilde C_t\to C_t$ has fibers isomorphic to ![]() $\mathbb {P} H(\tilde C_t,L)$, for all
$\mathbb {P} H(\tilde C_t,L)$, for all ![]() $L\in \textrm {Pic}^{g+1} (\tilde C_t)$.
$L\in \textrm {Pic}^{g+1} (\tilde C_t)$.
Its dimension is ![]() $2g+1$. We have the following key result.
$2g+1$. We have the following key result.
Proposition 4.1 Let ![]() $S$ and
$S$ and ![]() $L$ be as given previously. Then
$L$ be as given previously. Then ![]() $S^{[g+1]}$ contains a uniruled divisor that is the image of
$S^{[g+1]}$ contains a uniruled divisor that is the image of ![]() ${\mathscr {C}}_T^{(g+1)}$ under the natural rational map
${\mathscr {C}}_T^{(g+1)}$ under the natural rational map
for a certain irreducible component ![]() $T$ of the Severi variety parametrizing curves with
$T$ of the Severi variety parametrizing curves with ![]() $(p-g)$-nodes inside
$(p-g)$-nodes inside ![]() $|L|$.
$|L|$.
Proof. We may assume that ![]() $\textrm {Pic}(S)=\mathbb {Z} H$. We prove this statement by induction on
$\textrm {Pic}(S)=\mathbb {Z} H$. We prove this statement by induction on ![]() $g$. For
$g$. For ![]() $g=0$, the statement is simply the existence of nodal rational curves proved by [Reference ChenChe02].
$g=0$, the statement is simply the existence of nodal rational curves proved by [Reference ChenChe02].
It is sufficient to show the claim on the symmetric product ![]() $S^{(g+1)}$ of
$S^{(g+1)}$ of ![]() $S$. More precisely, we prove the following statement: there exists an irreducible component
$S$. More precisely, we prove the following statement: there exists an irreducible component ![]() $V$ of the Zariski closure of the Severi variety parametrizing nodal genus
$V$ of the Zariski closure of the Severi variety parametrizing nodal genus ![]() $g$ curves inside
$g$ curves inside ![]() $|L|$ such that, if
$|L|$ such that, if ![]() $\mathscr {C}_V\to V$ denotes the universal curve and
$\mathscr {C}_V\to V$ denotes the universal curve and ![]() $\mathscr {C}^{(g+1)}_V\to V$ the relative symmetric product, the natural morphism
$\mathscr {C}^{(g+1)}_V\to V$ the relative symmetric product, the natural morphism
is generically finite onto its image. Note that this is equivalent to saying that ![]() $(g+1)$-generic points on a generic curve of the family lie only on a finite number of curves of the family.
$(g+1)$-generic points on a generic curve of the family lie only on a finite number of curves of the family.
Indeed as
it follows that the image is a divisor inside ![]() $S^{(g+1)}$, and such divisor is uniruled as observed previously.
$S^{(g+1)}$, and such divisor is uniruled as observed previously.
Note also that the positive dimensional fibers of the morphism ![]() $\mathscr {C}^{(g+1)}_V\to S^{(g+1)}$ cannot lie in a fiber of
$\mathscr {C}^{(g+1)}_V\to S^{(g+1)}$ cannot lie in a fiber of ![]() $\mathscr {C}_V^{(g+1)}\to V$, as
$\mathscr {C}_V^{(g+1)}\to V$, as ![]() $C_t^{(g+1)}$ injects into
$C_t^{(g+1)}$ injects into ![]() $S^{(g+1)}$ for every
$S^{(g+1)}$ for every ![]() $t\in V$.
$t\in V$.
By inductive hypothesis, there exists an irreducible component ![]() $W$ of the (Zariski closure of the) Severi variety parametrizing nodal genus
$W$ of the (Zariski closure of the) Severi variety parametrizing nodal genus ![]() $g-1$ curves inside
$g-1$ curves inside ![]() $|L|$ such that, if
$|L|$ such that, if ![]() $\mathscr {C}_W\to W$ denotes the universal curve and
$\mathscr {C}_W\to W$ denotes the universal curve and ![]() $\mathscr {C}^{(g)}_W\to W$ the relative symmetric product, the natural morphism
$\mathscr {C}^{(g)}_W\to W$ the relative symmetric product, the natural morphism
is generically finite onto its image.
Now let ![]() $V$ be the Zariski closure of an irreducible component of the Severi variety of nodal genus
$V$ be the Zariski closure of an irreducible component of the Severi variety of nodal genus ![]() $g$ curves in
$g$ curves in ![]() $|H|$ obtained by smoothing one node of the curves in
$|H|$ obtained by smoothing one node of the curves in ![]() $W$ (which can be done again by the regularity of the Severi variety, [Reference Chiantini and SernesiCS97, Example 1.3]). By construction,
$W$ (which can be done again by the regularity of the Severi variety, [Reference Chiantini and SernesiCS97, Example 1.3]). By construction, ![]() $W\subset V$. Let
$W\subset V$. Let ![]() $\mathscr {C}_V\to V$ be the universal curve. Its restriction over
$\mathscr {C}_V\to V$ be the universal curve. Its restriction over ![]() $W$ yields a map
$W$ yields a map ![]() $\mathscr {C}_W\to W$. Let
$\mathscr {C}_W\to W$. Let ![]() $D$ be the image of the morphism
$D$ be the image of the morphism
Observe that ![]() $D$ contains the image
$D$ contains the image ![]() $D_{W}$ of
$D_{W}$ of
We claim that by the inductive hypothesis ![]() $D_{W}$ has codimension 2 or, equivalently, that the morphism
$D_{W}$ has codimension 2 or, equivalently, that the morphism ![]() $\mathscr {C}^{(g+1)}_W\to S^{(g+1)}$ is generically finite onto its image. Indeed if
$\mathscr {C}^{(g+1)}_W\to S^{(g+1)}$ is generically finite onto its image. Indeed if ![]() $\xi =x_1+\cdots +x_{g+1}$ is a generic point of the image, then, say,
$\xi =x_1+\cdots +x_{g+1}$ is a generic point of the image, then, say, ![]() $x_1+\cdots +x_{g}$ is a generic point of the image of the morphism
$x_1+\cdots +x_{g}$ is a generic point of the image of the morphism ![]() $\mathscr {C}^{(g)}_W\to S^{(g)}$. By the inductive hypothesis the points
$\mathscr {C}^{(g)}_W\to S^{(g)}$. By the inductive hypothesis the points ![]() $x_1,\ldots , x_{g}$ lie on finitely many curves of the family
$x_1,\ldots , x_{g}$ lie on finitely many curves of the family ![]() $W$, a fortiori that will be true for
$W$, a fortiori that will be true for ![]() $x_1,\ldots , x_{g}, x_{g+1}$ and the claim follows.
$x_1,\ldots , x_{g}, x_{g+1}$ and the claim follows.
We want to prove that ![]() $D$ contains
$D$ contains ![]() $D_{W}$ strictly. If this were not the case, by irreducibility, we would have
$D_{W}$ strictly. If this were not the case, by irreducibility, we would have ![]() $D=D_{W}$. Let
$D=D_{W}$. Let ![]() $U\subset D$ be an open subset over which the morphisms
$U\subset D$ be an open subset over which the morphisms ![]() $\mathscr {C}^{(g+1)}_W\to S^{(g+1)}$ and
$\mathscr {C}^{(g+1)}_W\to S^{(g+1)}$ and ![]() $\mathscr {C}_V^{(g+1)}\to S^{(g+1)}$ are smooth and let
$\mathscr {C}_V^{(g+1)}\to S^{(g+1)}$ are smooth and let ![]() $p_1+p_2+\cdots +p_{g+1}$ be a point in
$p_1+p_2+\cdots +p_{g+1}$ be a point in ![]() $U$. Let
$U$. Let ![]() $C$ be a nodal genus
$C$ be a nodal genus ![]() $g$ curve in
$g$ curve in ![]() $V$ containing these points. Let us fix the first
$V$ containing these points. Let us fix the first ![]() $g$ points
$g$ points ![]() $p_1,\ldots ,p_g$. By induction, these points are contained inside a finite number of curves of genus
$p_1,\ldots ,p_g$. By induction, these points are contained inside a finite number of curves of genus ![]() $g-1$ belonging to
$g-1$ belonging to ![]() $W$. Let
$W$. Let ![]() $B_1,\ldots , B_m$ be all such curves. Let
$B_1,\ldots , B_m$ be all such curves. Let ![]() $U_C\subset C$ be an open subset such that for all
$U_C\subset C$ be an open subset such that for all ![]() $q\in U_C$ we have
$q\in U_C$ we have ![]() $p_1+\cdots +p_g+q\in U$. As we have shown previously,
$p_1+\cdots +p_g+q\in U$. As we have shown previously, ![]() $p_1,\ldots ,p_g,q$ lie on finitely many curves of genus
$p_1,\ldots ,p_g,q$ lie on finitely many curves of genus ![]() $g-1$ belonging to
$g-1$ belonging to ![]() $W$, and these curves must be
$W$, and these curves must be ![]() $B_1,\ldots , B_m$. Therefore, as
$B_1,\ldots , B_m$. Therefore, as ![]() $q$ varies in
$q$ varies in ![]() $U_C$, we deduce that
$U_C$, we deduce that ![]() $U_C$ is a subset of a finite union of genus
$U_C$ is a subset of a finite union of genus ![]() $(g-1)$ curves. As
$(g-1)$ curves. As ![]() $C$ is irreducible, there is an
$C$ is irreducible, there is an ![]() $i$ such that
$i$ such that ![]() $C= B_i$, which is clearly a contradiction. Therefore,
$C= B_i$, which is clearly a contradiction. Therefore, ![]() $D$ must strictly contain
$D$ must strictly contain ![]() $D_{W}$ and be a divisor, which is necessarily uniruled.
$D_{W}$ and be a divisor, which is necessarily uniruled.
Remark 4.2 Let ![]() $n\geq 2$ be an integer. Let
$n\geq 2$ be an integer. Let ![]() $S$ be a general projective
$S$ be a general projective ![]() $K3$ surface,
$K3$ surface, ![]() $H$ an ample divisor on it and
$H$ an ample divisor on it and ![]() $L=mH,\ m\geq 1$, a line bundle with
$L=mH,\ m\geq 1$, a line bundle with ![]() $p_a(L)\geq n-1$. For all
$p_a(L)\geq n-1$. For all ![]() $k=1,\ldots , n$, consider a
$k=1,\ldots , n$, consider a ![]() $(k-1)$-dimensional family
$(k-1)$-dimensional family ![]() $T\subset |L|$ of nodal curves of geometric genus
$T\subset |L|$ of nodal curves of geometric genus ![]() $k-1$. Then consider the closure of the image of the rational map
$k-1$. Then consider the closure of the image of the rational map
By Proposition 4.1 we obtain ![]() $n$ distinct uniruled divisors
$n$ distinct uniruled divisors ![]() $D_1,\ldots , D_n$ inside
$D_1,\ldots , D_n$ inside ![]() $S^{[n]}$.
$S^{[n]}$.
Let us now compute the class of the curve in the ruling of the divisors given previously. Let us denote by ![]() $\mathbb {P}^{1}_{\mathfrak g^{1}_k}\subset S^{[k]}$ the image of a rational curve associated to one of the
$\mathbb {P}^{1}_{\mathfrak g^{1}_k}\subset S^{[k]}$ the image of a rational curve associated to one of the ![]() $\mathfrak g^{1}_k$ given, as in the proof of Proposition 4.1, by any
$\mathfrak g^{1}_k$ given, as in the proof of Proposition 4.1, by any ![]() $k$-points on the curve. We remark that the hypotheses imply that for a general choice, these linear series are simple and the nodes of the curve are non-neutral (a node
$k$-points on the curve. We remark that the hypotheses imply that for a general choice, these linear series are simple and the nodes of the curve are non-neutral (a node ![]() $p\in C$ is said to be non-neutral with respect to a linear series
$p\in C$ is said to be non-neutral with respect to a linear series ![]() ${\mathfrak g^{1}_k}$ on the desingularization
${\mathfrak g^{1}_k}$ on the desingularization ![]() $\nu :\tilde C\to C$ if
$\nu :\tilde C\to C$ if ![]() ${\mathfrak g^{1}_k}(-\nu ^{-1}(p))=\emptyset$, i.e. if the two points above the node do not belong to the same fiber of the morphism associated to the linear series). Then, the Riemann–Hurwitz formula (see, e.g., [Reference Ciliberto and KnutsenCK14, § 2]) yields
${\mathfrak g^{1}_k}(-\nu ^{-1}(p))=\emptyset$, i.e. if the two points above the node do not belong to the same fiber of the morphism associated to the linear series). Then, the Riemann–Hurwitz formula (see, e.g., [Reference Ciliberto and KnutsenCK14, § 2]) yields
where ![]() $mh$ is the class of
$mh$ is the class of ![]() $L$ in the Néron–Severi group of
$L$ in the Néron–Severi group of ![]() $S$.
$S$.
If we add ![]() $(n-k)$-distinct generic points
$(n-k)$-distinct generic points ![]() $\eta =q_1+\cdots +q_{n-k}$ to
$\eta =q_1+\cdots +q_{n-k}$ to ![]() $\mathbb {P}^{1}_{\mathfrak g^{1}_k}\subset S^{[k]}$ we obtain a rational curve inside
$\mathbb {P}^{1}_{\mathfrak g^{1}_k}\subset S^{[k]}$ we obtain a rational curve inside ![]() $S^{[n]}$, which we denote by
$S^{[n]}$, which we denote by ![]() $R_k$.
$R_k$.
Proposition 4.3 Let ![]() $k$ be an integer between
$k$ be an integer between ![]() $1$ and
$1$ and ![]() $n$. Then:
$n$. Then:
(1) the class of
 $R_k\subset S^{[n]}$ in
$R_k\subset S^{[n]}$ in  $H_2(S^{[n]},{{\mathbb {Z}}})$ is
$H_2(S^{[n]},{{\mathbb {Z}}})$ is  $mh-(2k-2)r_n$;
$mh-(2k-2)r_n$;(2) the class of
 $D_k\subset S^{[n]}$ in
$D_k\subset S^{[n]}$ in  $H^{2}(S^{[n]},{{\mathbb {Z}}})$ is proportional to
$H^{2}(S^{[n]},{{\mathbb {Z}}})$ is proportional to  $(2n-2)mh-(2k-2)\delta _n$.
$(2n-2)mh-(2k-2)\delta _n$.
Proof. (1) Write ![]() $R_k = ah-b r_n$. As
$R_k = ah-b r_n$. As ![]() $R_k=\mathbb{P}^1_{\mathfrak{g}^1_k}+\eta$, the intersection product
$R_k=\mathbb{P}^1_{\mathfrak{g}^1_k}+\eta$, the intersection product ![]() $R_k\cdot h$ equals
$R_k\cdot h$ equals ![]() $2p-2$, from which, using the facts recalled in § 2.1 we deduce
$2p-2$, from which, using the facts recalled in § 2.1 we deduce ![]() $a=m$. Again for the choice of
$a=m$. Again for the choice of ![]() $\eta$ we have that
$\eta$ we have that ![]() $R_k\cdot \delta_n=\mathbb{P}^1_{\mathfrak{g}^1_k}\cdot \delta_k$. From this, from (4.1) and from § 2.1 we deduce that
$R_k\cdot \delta_n=\mathbb{P}^1_{\mathfrak{g}^1_k}\cdot \delta_k$. From this, from (4.1) and from § 2.1 we deduce that
![]() $b=2(k-1)$. This proves the first statement.
$b=2(k-1)$. This proves the first statement.
(2) For the second statement we argue similarly as follows. Write ![]() $D_k = ah-b \delta_n$. Let
$D_k = ah-b \delta_n$. Let ![]() $x_1, \ldots, x_{n-1}\in S$ be general points and let
$x_1, \ldots, x_{n-1}\in S$ be general points and let ![]() $C\in |L|$ be a general curve. Set
$C\in |L|$ be a general curve. Set ![]() $\xi :=x_1+ \cdots+ x_{n-1}$ and consider the curve in
$\xi :=x_1+ \cdots+ x_{n-1}$ and consider the curve in ![]() $S^{[n]}$ given by
$S^{[n]}$ given by ![]() $\xi+C$. Such a curve has class
$\xi+C$. Such a curve has class ![]() $h\in H_2(S,\mathbb{Z})$. We first describe all the points in the intersection between
$h\in H_2(S,\mathbb{Z})$. We first describe all the points in the intersection between ![]() $D_k$ and
$D_k$ and ![]() $\xi+C$. Let I be a subset of
$\xi+C$. Let I be a subset of ![]() $k-1$ indices among
$k-1$ indices among ![]() $\{1,\ldots, n-1 \}$ and let
$\{1,\ldots, n-1 \}$ and let ![]() $\xi_I$ be the corresponding zero-dimensional subscheme of length
$\xi_I$ be the corresponding zero-dimensional subscheme of length ![]() $k-1$. Notice that, as shown in Proposition 4.1, there exists a finite subfamily
$k-1$. Notice that, as shown in Proposition 4.1, there exists a finite subfamily ![]() $T_\xi$ of cardinality M given by curves in T passing through
$T_\xi$ of cardinality M given by curves in T passing through ![]() $\xi_I$. For each curve
$\xi_I$. For each curve ![]() $C'\in T_\xi$ and each point q of the
$C'\in T_\xi$ and each point q of the ![]() $2p-2$ intersection points between
$2p-2$ intersection points between ![]() $C'$ and C we get an intersection between
$C'$ and C we get an intersection between ![]() $D_k$ and
$D_k$ and ![]() $\xi+C$ given by
$\xi+C$ given by ![]() $q+\xi$. All in all we obtain that
$q+\xi$. All in all we obtain that
from which we deduce that ![]() $a=m M {{n-1}\choose{k-1}}$.
$a=m M {{n-1}\choose{k-1}}$.
Consider ![]() $p, x_1,\ldots, x_{n-2}\in S$ general points. Set
$p, x_1,\ldots, x_{n-2}\in S$ general points. Set ![]() $\xi=x_1+\cdots +x_{n-2}$ and consider the curve
$\xi=x_1+\cdots +x_{n-2}$ and consider the curve ![]() $\mathbb{P} T_p(S)+\xi$, which has class
$\mathbb{P} T_p(S)+\xi$, which has class ![]() $r_n$. We now describe all the points in the intersection between
$r_n$. We now describe all the points in the intersection between ![]() $D_k$ and
$D_k$ and ![]() $\mathbb{P} T_p(S)+\xi$. Let I be a subset of
$\mathbb{P} T_p(S)+\xi$. Let I be a subset of ![]() $k-2$ indices among
$k-2$ indices among ![]() $\{1,\ldots, n-2 \}$ and let
$\{1,\ldots, n-2 \}$ and let ![]() $\xi_I$ be the corresponding zero-dimensional subscheme of length
$\xi_I$ be the corresponding zero-dimensional subscheme of length ![]() $k-2$. As above, there exists a finite subfamily
$k-2$. As above, there exists a finite subfamily ![]() $T_{p+\xi}$ of cardinality M given by curves in T passing through
$T_{p+\xi}$ of cardinality M given by curves in T passing through ![]() $p+\xi_I$. For each curve
$p+\xi_I$. For each curve ![]() $C'\in T_{p+\xi}$ we get an intersection between
$C'\in T_{p+\xi}$ we get an intersection between ![]() $D_k$ and
$D_k$ and ![]() $r_n$ given by
$r_n$ given by ![]() $p_{C'}+\xi$, where
$p_{C'}+\xi$, where ![]() $p_{C'}$ is the length two zero-dimensional subschemes supported on p and determined by the tangent direction of
$p_{C'}$ is the length two zero-dimensional subschemes supported on p and determined by the tangent direction of ![]() $C'$ at p. We therefore have
$C'$ at p. We therefore have
from which we deduce that ![]() $b=M {{n-2}\choose{k-2}}$. Therefore
$b=M {{n-2}\choose{k-2}}$. Therefore
and we are done.
To account for other polarization types, we proceed as follows. For ![]() $k=2,\ldots , n$ let
$k=2,\ldots , n$ let ![]() $\xi ' \in S^{[k]}$ be a zero-dimensional non-reduced subscheme corresponding to a ramification point of a
$\xi ' \in S^{[k]}$ be a zero-dimensional non-reduced subscheme corresponding to a ramification point of a ![]() $\mathfrak g^{1}_k$ on a general curve
$\mathfrak g^{1}_k$ on a general curve ![]() $\tilde C_t$. Let
$\tilde C_t$. Let ![]() $\xi \in S^{[n]}$ be a zero-dimensional subscheme obtained by adding
$\xi \in S^{[n]}$ be a zero-dimensional subscheme obtained by adding ![]() $(n-k)$ distinct generic points to
$(n-k)$ distinct generic points to ![]() $\xi '$. Let
$\xi '$. Let ![]() $\mathbb {P}^{1}_\xi$ the exceptional rational curve passing through
$\mathbb {P}^{1}_\xi$ the exceptional rational curve passing through ![]() $\xi$. Consider the curve
$\xi$. Consider the curve
obtained by glueing along ![]() $\xi$ the curve
$\xi$ the curve ![]() $R_k$ corresponding to the
$R_k$ corresponding to the ![]() $\mathfrak g^{1}_k$ and the exceptional rational curve
$\mathfrak g^{1}_k$ and the exceptional rational curve ![]() $\mathbb {P}^{1}_\xi$. Working with families of rational curves, we obtain as before uniruled divisors
$\mathbb {P}^{1}_\xi$. Working with families of rational curves, we obtain as before uniruled divisors ![]() $D'_2, \ldots , D'_n$. The divisor
$D'_2, \ldots , D'_n$. The divisor ![]() $D'_k$ is the union of
$D'_k$ is the union of ![]() $D_k$ with the exceptional divisor of
$D_k$ with the exceptional divisor of ![]() $S^{[n]}$. As a direct consequence of Proposition 4.3, we can compute the relevant cohomology classes.
$S^{[n]}$. As a direct consequence of Proposition 4.3, we can compute the relevant cohomology classes.
Proposition 4.4 Let ![]() $k$ be an integer between
$k$ be an integer between ![]() $2$ and
$2$ and ![]() $n$. Then:
$n$. Then:
(1) the class of
 $R'_k\subset S^{[n]}$ in
$R'_k\subset S^{[n]}$ in  $H_2(S^{[n]},{{\mathbb {Z}}})$ is
$H_2(S^{[n]},{{\mathbb {Z}}})$ is  $h-(2k-1)r_n$;
$h-(2k-1)r_n$;(2) the class of
 $D'_k\subset S^{[n]}$ in
$D'_k\subset S^{[n]}$ in  $H^{2}(S^{[n]},{{\mathbb {Z}}})$ is proportional to
$H^{2}(S^{[n]},{{\mathbb {Z}}})$ is proportional to  $(2n-2)h-(2k-1)\delta _n$.
$(2n-2)h-(2k-1)\delta _n$.
We now move on to the result we alluded to in Remark 1.4 the introduction.
Theorem 4.5 Let ![]() $(X,H)$ be a polarized holomorphic symplectic variety of
$(X,H)$ be a polarized holomorphic symplectic variety of ![]() $K3^{[n]}$-type. Suppose there exist integers
$K3^{[n]}$-type. Suppose there exist integers ![]() $p,g$ and
$p,g$ and ![]() $\epsilon$ such that
$\epsilon$ such that ![]() $p\geq g$ and
$p\geq g$ and ![]() $\epsilon =0$ or
$\epsilon =0$ or ![]() $1$ such that the following two conditions hold:
$1$ such that the following two conditions hold:
(i) the class
 $\alpha :={H^{\vee }}/{ {\rm div}(H)}\in H_2(X,\mathbb {Z})$ can be written as
$\alpha :={H^{\vee }}/{ {\rm div}(H)}\in H_2(X,\mathbb {Z})$ can be written as  $\gamma +(2g-\epsilon )\eta$, with
$\gamma +(2g-\epsilon )\eta$, with  $\eta$ in the monodromy orbit of the class of the exceptional curve on a
$\eta$ in the monodromy orbit of the class of the exceptional curve on a  $K3^{[n]}$ and
$K3^{[n]}$ and  $\gamma \in \eta ^{\perp }$;
$\gamma \in \eta ^{\perp }$;(ii)
 $q(\gamma )=2p-2$ (hence,
$q(\gamma )=2p-2$ (hence,  $q(\alpha )=2p-2-{(2g-\epsilon )^{2}}/({2n-2})$).
$q(\alpha )=2p-2-{(2g-\epsilon )^{2}}/({2n-2})$).
Then there exists an integer ![]() $m>0$ such that the linear system
$m>0$ such that the linear system ![]() $|mH|$ contains a uniruled divisor covered by rational curves of primitive class equal to
$|mH|$ contains a uniruled divisor covered by rational curves of primitive class equal to ![]() $\alpha$.
$\alpha$.
Proof. Let ![]() $\alpha \in H_2(X, {{\mathbb {Z}}})$ as in the statement of the theorem. By Corollary 2.8, there exists a
$\alpha \in H_2(X, {{\mathbb {Z}}})$ as in the statement of the theorem. By Corollary 2.8, there exists a ![]() $K3$ surface
$K3$ surface ![]() $S$ as given previously such that the pair
$S$ as given previously such that the pair ![]() $(X, \alpha )$ is deformation equivalent to
$(X, \alpha )$ is deformation equivalent to ![]() $(S^{[n]}, [R_k])$ for some
$(S^{[n]}, [R_k])$ for some ![]() $k$ between
$k$ between ![]() $1$ and
$1$ and ![]() $n$, or to
$n$, or to ![]() $(S^{[n]}, [R'_k])$ for some
$(S^{[n]}, [R'_k])$ for some ![]() $k$ between
$k$ between ![]() $2$ and
$2$ and ![]() $n$ (with
$n$ (with ![]() $R_k$ and
$R_k$ and ![]() $R'_k$ as in Propositions 4.3 and 4.4). Note that being deformation equivalent means that
$R'_k$ as in Propositions 4.3 and 4.4). Note that being deformation equivalent means that ![]() $(X,\alpha )$ and
$(X,\alpha )$ and ![]() $(S^{[n]}, R_k)$ (or
$(S^{[n]}, R_k)$ (or ![]() $(S^{[n]}, [R'_k])$) are connected by a family
$(S^{[n]}, [R'_k])$) are connected by a family ![]() $f: \mathscr{X}\to B$, where
$f: \mathscr{X}\to B$, where ![]() $B$ is an irreducible curve, so that the parallel transport brings
$B$ is an irreducible curve, so that the parallel transport brings ![]() $\alpha$ to
$\alpha$ to ![]() $[R_k]$ (respectively,
$[R_k]$ (respectively, ![]() $[R'_k]$).
$[R'_k]$).
Corollary 3.5 shows indeed that X contains a uniruled divisor with class a multiple of h and the theorem now follows.
4.2 Finiteness of the exceptions and proof of the main theorem
In this section we prove that, for every dimension, there is at most a finite number of components of the moduli space of polarized irreducible holomorphic symplectic manifolds ![]() $(X,H)$ of
$(X,H)$ of ![]() $K3^{[n]}$-type where the strategy of the previous sections does not work. Together with Theorem 4.5 this will conclude the proof of Theorem 1.2.
$K3^{[n]}$-type where the strategy of the previous sections does not work. Together with Theorem 4.5 this will conclude the proof of Theorem 1.2.
The uniruled divisors we constructed in the previous paragraph have class ![]() $h_S-(2g)r_n$ (or
$h_S-(2g)r_n$ (or ![]() $h_S-(2g-1)r_n$), with
$h_S-(2g-1)r_n$), with ![]() $2p-2=h_S^{2}$ and
$2p-2=h_S^{2}$ and ![]() $h_S$ the primitive polarization on the
$h_S$ the primitive polarization on the ![]() $K3$ surface. We have the following result.
$K3$ surface. We have the following result.
Proposition 4.6 Let ![]() $C$ be a primitive class of a curve on a manifold of K3
$C$ be a primitive class of a curve on a manifold of K3![]() $^{[n]}$-type such that its square
$^{[n]}$-type such that its square ![]() $C^{2}$ with respect to the Beauville–Bogomolov form is
$C^{2}$ with respect to the Beauville–Bogomolov form is ![]() $>0$. If
$>0$. If ![]() $q(C)\geq n-1$, then
$q(C)\geq n-1$, then ![]() $C$ is deformation equivalent to the class of one of the curves constructed in the previous section.
$C$ is deformation equivalent to the class of one of the curves constructed in the previous section.
Proof. We know by Corollary 2.8 that ![]() $C$ is deformation equivalent to either
$C$ is deformation equivalent to either ![]() $h_S-2gr_n$ or
$h_S-2gr_n$ or ![]() $h_S-(2g-1)r_n$, with
$h_S-(2g-1)r_n$, with ![]() $2g\leq n-1$ (respectively,
$2g\leq n-1$ (respectively, ![]() $2g\leq n$). If
$2g\leq n$). If ![]() $p$ were
$p$ were ![]() $<g$, then, in both cases, we would have
$<g$, then, in both cases, we would have
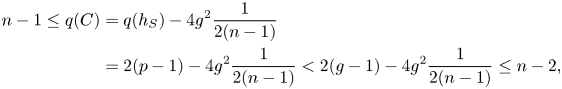 \begin{align*} n-1 \leq q(C)&= q(h_S) -4g^{2} \frac{1}{2(n-1)}\\ &= 2(p-1) -4g^{2} \frac{1}{2(n-1)}< 2(g-1)-4g^{2} \frac{1}{2(n-1)}\leq n -2, \end{align*}
\begin{align*} n-1 \leq q(C)&= q(h_S) -4g^{2} \frac{1}{2(n-1)}\\ &= 2(p-1) -4g^{2} \frac{1}{2(n-1)}< 2(g-1)-4g^{2} \frac{1}{2(n-1)}\leq n -2, \end{align*}
which is a contradiction.
Proof of Theorem 1.2 The components of ![]() $\mathfrak {M}$ are in bijective correspondence with the monodromy orbits of a given class of positive square in
$\mathfrak {M}$ are in bijective correspondence with the monodromy orbits of a given class of positive square in ![]() $H^{2}(X,\mathbb {Z})$, see [Reference ApostolovApo14, Corollary 2.4]. For a fixed square of
$H^{2}(X,\mathbb {Z})$, see [Reference ApostolovApo14, Corollary 2.4]. For a fixed square of ![]() $h$, there is a finite number of orbits (cf. [Reference Gritsenko, Hulek and SankaranGHS10, Proposition 1.2]), so it follows that if
$h$, there is a finite number of orbits (cf. [Reference Gritsenko, Hulek and SankaranGHS10, Proposition 1.2]), so it follows that if ![]() $X$ has a uniruled divisor when
$X$ has a uniruled divisor when ![]() $q(h)$ is big enough, our claim will hold. Let
$q(h)$ is big enough, our claim will hold. Let ![]() $C$ be a curve class in
$C$ be a curve class in ![]() $H_2(X,\mathbb {Z})$ such that
$H_2(X,\mathbb {Z})$ such that ![]() $C=h/{\rm div}(h)$ under the map (2.7). The divisibility of
$C=h/{\rm div}(h)$ under the map (2.7). The divisibility of ![]() $h$ is at most
$h$ is at most ![]() $2n-2$, therefore if
$2n-2$, therefore if ![]() $q(h)\geq (2n-2)^{2}(n-1)$ the curve class
$q(h)\geq (2n-2)^{2}(n-1)$ the curve class ![]() $C$ has square at least
$C$ has square at least ![]() $n-1$, so that Proposition 4.6 applies and both items of the theorem follow from Theorem 4.5.
$n-1$, so that Proposition 4.6 applies and both items of the theorem follow from Theorem 4.5.
Corollary 4.7 Let ![]() $X$ be a projective irreducible holomorphic symplectic variety of K3
$X$ be a projective irreducible holomorphic symplectic variety of K3![]() $^{[n]}$-type with Picard rank at least two. Then
$^{[n]}$-type with Picard rank at least two. Then ![]() $X$ has an ample divisor ruled by primitive rational curves.
$X$ has an ample divisor ruled by primitive rational curves.
Proof. As ![]() $X$ is projective and has Picard rank at least two, its Picard lattice is indefinite and contains primitive elements of positive arbitrary Beauville–Bogomolov square. The same holds for ample classes. Let
$X$ is projective and has Picard rank at least two, its Picard lattice is indefinite and contains primitive elements of positive arbitrary Beauville–Bogomolov square. The same holds for ample classes. Let ![]() $h$ be an ample divisor such that
$h$ be an ample divisor such that ![]() $q(h)\geq (2n-2)^{2}(n-1)$. Let
$q(h)\geq (2n-2)^{2}(n-1)$. Let ![]() $C$ be a curve class in
$C$ be a curve class in ![]() $H_2(X,\mathbb {Z})$ such that
$H_2(X,\mathbb {Z})$ such that ![]() $C=h/{\rm div}(h)$ under the map (2.7). As in the proof of Theorem 1.2 it follows that
$C=h/{\rm div}(h)$ under the map (2.7). As in the proof of Theorem 1.2 it follows that ![]() $q(C)\geq n-1$ and Proposition 4.6 yields our claim.
$q(C)\geq n-1$ and Proposition 4.6 yields our claim.
Remark 4.8 The estimate of Proposition 4.6 is definitely not sharp, indeed all primitive curves of positive square on irreducible holomorphic symplectic manifolds of ![]() $K3^{[n]}$-type with
$K3^{[n]}$-type with ![]() $n\leq 7$ are deformation equivalent to the curves we construct. Indeed, by Corollary 2.8 we can suppose that our pair is
$n\leq 7$ are deformation equivalent to the curves we construct. Indeed, by Corollary 2.8 we can suppose that our pair is ![]() $(S^{[n]},h_S-\mu r_n)$ with
$(S^{[n]},h_S-\mu r_n)$ with ![]() $0\leq \mu \leq n-1$ and
$0\leq \mu \leq n-1$ and ![]() $S$ is a K3 of genus
$S$ is a K3 of genus ![]() $p$. This curve is constructed from a curve of class
$p$. This curve is constructed from a curve of class ![]() $h_S-2g r_n$ with the eventual addition of a tail of class
$h_S-2g r_n$ with the eventual addition of a tail of class ![]() $r_n$, so that
$r_n$, so that ![]() $2g\leq n$. Let us suppose that
$2g\leq n$. Let us suppose that ![]() $g>p$ and
$g>p$ and ![]() $n\leq 7$. We have
$n\leq 7$. We have
However, the last value is never positive, hence ![]() $q(h_S-2g r_n)$ can only be negative. Analogously, for
$q(h_S-2g r_n)$ can only be negative. Analogously, for ![]() $C=h_S-(2g-1)r_n$, we have
$C=h_S-(2g-1)r_n$, we have ![]() $q(C)\leq ({20p-25-4p^{2}})/{12}$ with
$q(C)\leq ({20p-25-4p^{2}})/{12}$ with ![]() $g\geq p+1$ and
$g\geq p+1$ and ![]() $2g\leq n$, which is again not positive.
$2g\leq n$, which is again not positive.
5. Comparison with the work of Oberdieck, Shen and Yin and further results
In this section we compare our results with those of Oberdieck, Shen and Yin [Reference Oberdieck, Shen and YinOSY19, Corollary A.3]. We show that conditions (i) and (ii) in Theorem 4.5 are precisely satisfied in all cases where the obstruction discovered by [Reference Oberdieck, Shen and YinOSY19] does not prevent uniruled divisors covered by primitive rational curves to exist. In other words, we show that our result is sharp. We discuss how the counterexamples to the existence of uniruled divisors covered by rational curves of primitive class propagate in higher dimensions. Moreover, up to a relatively high value of the dimension (![]() $2n=26$), we show that the existence of uniruled divisors can nevertheless be obtained via non-primitive rational curves. Finally in some of the ‘exceptional cases’ (those where existence of uniruled divisors covered by rational curves of primitive class is excluded) we show that the codimension of the locus covered by the primitive rational curves is two.
$2n=26$), we show that the existence of uniruled divisors can nevertheless be obtained via non-primitive rational curves. Finally in some of the ‘exceptional cases’ (those where existence of uniruled divisors covered by rational curves of primitive class is excluded) we show that the codimension of the locus covered by the primitive rational curves is two.
5.1 Comparison with the work of Oberdieck, Shen and Yin
To conclude this section let us recall the condition in [Reference Oberdieck, Shen and YinOSY19, Corollary A.3].
Proposition 5.1 [Reference Oberdieck, Shen and YinOSY19]
Let ![]() $\beta$ be a curve class on a manifold
$\beta$ be a curve class on a manifold ![]() $X$ of K3
$X$ of K3![]() $^{[n]}$-type. Then there is a uniruled divisor swept out by
$^{[n]}$-type. Then there is a uniruled divisor swept out by ![]() $\beta$ if
$\beta$ if
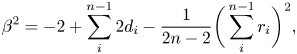 \begin{gather} \beta^{2} = -2+\sum^{n-1}_i 2d_i-\frac{1}{2n-2}\bigg(\sum^{n-1}_i r_i\bigg)^{2}, \end{gather}
\begin{gather} \beta^{2} = -2+\sum^{n-1}_i 2d_i-\frac{1}{2n-2}\bigg(\sum^{n-1}_i r_i\bigg)^{2}, \end{gather}
Here ![]() $[\beta ]$ denotes the class of
$[\beta ]$ denotes the class of ![]() $\beta$ seen as an element of the discriminant group
$\beta$ seen as an element of the discriminant group ![]() $H^{2}(X,\mathbb {Z})^{\vee }/H^{2}(X,\mathbb {Z})$ (with a generator of square
$H^{2}(X,\mathbb {Z})^{\vee }/H^{2}(X,\mathbb {Z})$ (with a generator of square ![]() $-1/(2n-2)$, which is in the same monodromy orbit of the class
$-1/(2n-2)$, which is in the same monodromy orbit of the class ![]() $r_n$ of exceptional lines on
$r_n$ of exceptional lines on ![]() $S^{[n]}$ for any
$S^{[n]}$ for any ![]() $K3$ surface
$K3$ surface ![]() $S$). The converse holds if
$S$). The converse holds if ![]() $\beta$ is irreducible.
$\beta$ is irreducible.
Proposition 5.2 Let ![]() $n,g>0$ be integers such that
$n,g>0$ be integers such that ![]() $2g\leq n$. Let
$2g\leq n$. Let ![]() $p$ be an integer number. The condition
$p$ be an integer number. The condition ![]() $p\geq g$ for a curve of class
$p\geq g$ for a curve of class ![]() $h_S-2g r_n$ with
$h_S-2g r_n$ with ![]() $h_S^{2}=2p-2$, on an Hilbert scheme
$h_S^{2}=2p-2$, on an Hilbert scheme ![]() $S^{[n]}$ is equivalent to the conditions in Proposition 5.1.
$S^{[n]}$ is equivalent to the conditions in Proposition 5.1.
Proof. Let us call ![]() $\beta =h_S-2g r_n$. We have
$\beta =h_S-2g r_n$. We have ![]() $\beta ^{2}=-2+2p-({1}/({2n-2}))4g^{2}$ and
$\beta ^{2}=-2+2p-({1}/({2n-2}))4g^{2}$ and ![]() $[\beta ]=[2g r_n]$ in the discriminant group. Therefore, we must have
$[\beta ]=[2g r_n]$ in the discriminant group. Therefore, we must have
If ![]() $p\geq g$, we can set
$p\geq g$, we can set ![]() $r_i= 2$ for
$r_i= 2$ for ![]() $g$ indices
$g$ indices ![]() $i$ such that
$i$ such that ![]() $d_i\neq 0$ and set
$d_i\neq 0$ and set ![]() $r_i=0$ for all the others, so that the conditions in Proposition 5.1 are satisfied. On the other hand, if
$r_i=0$ for all the others, so that the conditions in Proposition 5.1 are satisfied. On the other hand, if ![]() $g>p$, there is at least one
$g>p$, there is at least one ![]() $r_i>2d_i$, so that
$r_i>2d_i$, so that ![]() $4d_i-r_i^{2}<0$, contradicting the third item in Proposition 5.1.
$4d_i-r_i^{2}<0$, contradicting the third item in Proposition 5.1.
Remark 5.3 Observe that if ![]() $p,g$ and
$p,g$ and ![]() $n$ are as in Proposition 5.2, then, for all
$n$ are as in Proposition 5.2, then, for all ![]() $n'\geq n$, the integers
$n'\geq n$, the integers ![]() $p,g$ and
$p,g$ and ![]() $n'$ again provide examples of primitive classes
$n'$ again provide examples of primitive classes ![]() $h_S-2g r_{n'}\in H_2(S^{[n']},{{\mathbb {Z}}})$ which cannot rule a divisor.
$h_S-2g r_{n'}\in H_2(S^{[n']},{{\mathbb {Z}}})$ which cannot rule a divisor.
Remark 5.4 Condition (ii) in Remark 1.4 is not sufficient to ensure the existence of a uniruled divisor covered by primitive rational curves. Indeed, let ![]() $n=11$ and consider two general polarized
$n=11$ and consider two general polarized ![]() $K3$ surfaces
$K3$ surfaces ![]() $(S_1,h_1)$ and
$(S_1,h_1)$ and ![]() $(S_2,h_2)$ of genus
$(S_2,h_2)$ of genus ![]() $2$ and
$2$ and ![]() $4$, respectively. One checks that the classes
$4$, respectively. One checks that the classes ![]() $C_1:=h_{S_1}-r_{11}$ on
$C_1:=h_{S_1}-r_{11}$ on ![]() $S_1^{[11]}$ and
$S_1^{[11]}$ and ![]() $C_2:=h_{S_2}-9r_{11}$ on
$C_2:=h_{S_2}-9r_{11}$ on ![]() $S_2^{[11]}$ have the same square
$S_2^{[11]}$ have the same square ![]() $=2- 1/{20}$. The divisors
$=2- 1/{20}$. The divisors ![]() $h_i,\ i=1,2$, such that
$h_i,\ i=1,2$, such that ![]() $C_i=h_i/ {\rm div}(h_i)$ are
$C_i=h_i/ {\rm div}(h_i)$ are ![]() $h_1= 20 h_{S_1}-\delta _{11}$ and
$h_1= 20 h_{S_1}-\delta _{11}$ and ![]() $h_2= 20 h_{S_2}-9 \delta _{11}$. Nevertheless, they are not in the same orbit under the monodromy action. This can be seen as follows: by Markman, two classes are monodromy equivalent if and only if they have the same square and their images in the discriminant group are equal up to sign. Now the image of the first class is
$h_2= 20 h_{S_2}-9 \delta _{11}$. Nevertheless, they are not in the same orbit under the monodromy action. This can be seen as follows: by Markman, two classes are monodromy equivalent if and only if they have the same square and their images in the discriminant group are equal up to sign. Now the image of the first class is ![]() $[r_{11}]$ whereas that of the second is
$[r_{11}]$ whereas that of the second is ![]() $[9r_{11}]$. Moreover, by Proposition 5.1, only the first can be the class of rational curves covering a divisor.
$[9r_{11}]$. Moreover, by Proposition 5.1, only the first can be the class of rational curves covering a divisor.
5.2 Uniruledness via non-primitive rational curves
Let ![]() $\epsilon$ be equal to
$\epsilon$ be equal to ![]() $0$ or
$0$ or ![]() $1$. Suppose
$1$. Suppose ![]() $n,p$ and
$n,p$ and ![]() $g$ are positive integers such that
$g$ are positive integers such that
Let ![]() $(S,h_S)$ be a general polarized
$(S,h_S)$ be a general polarized ![]() $K3$ surface of genus
$K3$ surface of genus ![]() $p=p_a(h_S)$. Let
$p=p_a(h_S)$. Let ![]() $C\in H_2(S^{[n]},{{\mathbb {Z}}})$ be a primitive curve class of the form
$C\in H_2(S^{[n]},{{\mathbb {Z}}})$ be a primitive curve class of the form
Note that condition (5.7) is equivalent to ![]() $q(C)>0$. By condition (5.6) we cannot apply our main result to
$q(C)>0$. By condition (5.6) we cannot apply our main result to ![]() $C$ (and by [Reference Oberdieck, Shen and YinOSY19, Corollary A.3] there is no way to obtain a uniruled divisor covered by rational curves of class
$C$ (and by [Reference Oberdieck, Shen and YinOSY19, Corollary A.3] there is no way to obtain a uniruled divisor covered by rational curves of class ![]() $C$). Nevertheless, it makes sense to ask the following.
$C$). Nevertheless, it makes sense to ask the following.
Question 5.5 Does there exist an integer ![]() $m>0$ such that
$m>0$ such that ![]() $mC$ is represented by rational curves covering a divisor?
$mC$ is represented by rational curves covering a divisor?
In particular, as the Severi varieties of ![]() $|mh_S|$ are known to be non-empty by [Reference ChenChe02], it is natural to try and extend our approach to the multiple hyperplane linear system. Precisely, we can look for an integral nodal curve
$|mh_S|$ are known to be non-empty by [Reference ChenChe02], it is natural to try and extend our approach to the multiple hyperplane linear system. Precisely, we can look for an integral nodal curve ![]() $C'\in |mh_S|$ of genus
$C'\in |mh_S|$ of genus ![]() $g'=\lceil ({2mg-m\epsilon })/{2}\rceil$ and take
$g'=\lceil ({2mg-m\epsilon })/{2}\rceil$ and take ![]() $g'+1$ points on
$g'+1$ points on ![]() $C'$ to obtain a rational curve in
$C'$ to obtain a rational curve in ![]() $S^{[n]}$ of class
$S^{[n]}$ of class ![]() $mC$ (possibly after the union of an exceptional tail, depending on the parity of
$mC$ (possibly after the union of an exceptional tail, depending on the parity of ![]() $m$).
$m$).
The obvious necessary numerical conditions to be satisfied are
If such an integer ![]() $m$ exists, by applying Proposition 4.1 and the same strategy of Theorem 1.2 we would obtain the existence of uniruled divisors in the components of
$m$ exists, by applying Proposition 4.1 and the same strategy of Theorem 1.2 we would obtain the existence of uniruled divisors in the components of ![]() $\mathfrak M$ (which is the union
$\mathfrak M$ (which is the union ![]() $\cup _{d>0}\mathfrak M_{2d}$ of the moduli spaces
$\cup _{d>0}\mathfrak M_{2d}$ of the moduli spaces ![]() $\mathfrak M_{2d}$ of projective irreducible holomorphic symplectic varieties of
$\mathfrak M_{2d}$ of projective irreducible holomorphic symplectic varieties of ![]() $K3^{[n]}$-type polarized by a line bundle of degree
$K3^{[n]}$-type polarized by a line bundle of degree ![]() $2d$) left out from Theorem 1.2.
$2d$) left out from Theorem 1.2.
Let us define the following quantities (coming from conditions (5.8) and (5.9) by distinguishing according to the parity of ![]() $m$):
$m$):
From the discussion given previously, we deduce the following result.
Proposition 5.6 If there exists an integer ![]() $m>0$ such that
$m>0$ such that
then Question 5.5 has a positive answer.
To apply Proposition 5.6, one must first check that ![]() $m_\textrm {min}^{\bullet } \leq m_\textrm {max}^{\bullet }$. This easily follows from (5.7) when
$m_\textrm {min}^{\bullet } \leq m_\textrm {max}^{\bullet }$. This easily follows from (5.7) when ![]() $m$ is even or
$m$ is even or ![]() $\epsilon =0$.
$\epsilon =0$.
We can now show that the apparent persistence of the pathologies, observed in Remark 5.3, can be avoided by taking non-primitive curves.
Proposition 5.7 Let ![]() $n,p$ and
$n,p$ and ![]() $g$ as given previously. Then for all
$g$ as given previously. Then for all ![]() $n'\geq g+1+n$ there exists an integer
$n'\geq g+1+n$ there exists an integer ![]() $m>0$ satisfying (5.14).
$m>0$ satisfying (5.14).
Proof. If ![]() $n'\geq n+1$, we always have
$n'\geq n+1$, we always have ![]() $m_\textrm {min}^{\bullet } \leq m_\textrm {max}^{\bullet }$. Moreover, for
$m_\textrm {min}^{\bullet } \leq m_\textrm {max}^{\bullet }$. Moreover, for ![]() $n'\geq n+1+g$ the value of
$n'\geq n+1+g$ the value of ![]() $m_\textrm {max}^{\bullet }$ increases by at least one.
$m_\textrm {max}^{\bullet }$ increases by at least one.
We have shown in Remark 4.8 that we do have existence of uniruled divisors covered by primitive rational curves in sufficiently ample linear systems on irreducible holomorphic symplectic manifolds of ![]() $K3^{[n]}$-type with
$K3^{[n]}$-type with ![]() $n\leq 7$.
$n\leq 7$.
There are cases in which condition (5.14) is easily checked to hold: for instance, for all ![]() $p, g$ and
$p, g$ and ![]() $n$ verifying (5.6) and (5.7) and such that
$n$ verifying (5.6) and (5.7) and such that ![]() $(2p-2)| (2g-\epsilon )$. In particular, this yields the following.
$(2p-2)| (2g-\epsilon )$. In particular, this yields the following.
Theorem 5.8 Let ![]() $8\leq n\leq 13$ be an integer. Let
$8\leq n\leq 13$ be an integer. Let ![]() $\mathfrak M=\cup _{d>0}\mathfrak M_{2d}$ be the union of the moduli spaces
$\mathfrak M=\cup _{d>0}\mathfrak M_{2d}$ be the union of the moduli spaces ![]() $\mathfrak M_{2d}$ of projective irreducible holomorphic symplectic varieties of
$\mathfrak M_{2d}$ of projective irreducible holomorphic symplectic varieties of ![]() $K3^{[n]}$-type polarized by a line bundle of degree
$K3^{[n]}$-type polarized by a line bundle of degree ![]() $2d$. For all
$2d$. For all ![]() $(X,H)\in \mathfrak M$, there exists a positive integer
$(X,H)\in \mathfrak M$, there exists a positive integer ![]() $a$ such that the linear system
$a$ such that the linear system ![]() $|aH|$ contains a uniruled divisor.
$|aH|$ contains a uniruled divisor.
Proof. We follow the strategy of taking a curve in the multiple hyperplane system ![]() $|mH_S|$ outlined previously. In Table 1, we list the curve classes arising as exceptions to Theorem 1.2, together with the genus
$|mH_S|$ outlined previously. In Table 1, we list the curve classes arising as exceptions to Theorem 1.2, together with the genus ![]() $p$ of the
$p$ of the ![]() $K3$ surface
$K3$ surface ![]() $S$, the values of
$S$, the values of ![]() $g$ and
$g$ and ![]() $\epsilon$, the minimal
$\epsilon$, the minimal ![]() $m$ which satisfies (5.14) and the smallest
$m$ which satisfies (5.14) and the smallest ![]() $n$ such that
$n$ such that ![]() $h_S-(2g-\epsilon )r_n$ does not satisfy Theorem 1.2.
$h_S-(2g-\epsilon )r_n$ does not satisfy Theorem 1.2.
Table 1. Primitive curve classes not ruling a divisor.
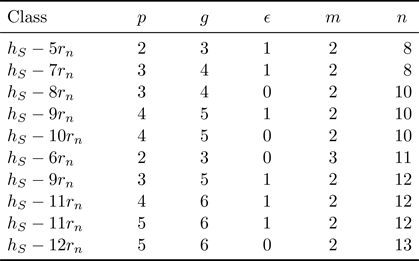
The conclusion immediately follows from Table 1.
The first case when this strategy does not work appears for ![]() $n=14$, by taking
$n=14$, by taking
where ![]() $h_S$ is a polarization of genus
$h_S$ is a polarization of genus ![]() $p_a(h_S)=3$ and one checks that
$p_a(h_S)=3$ and one checks that ![]() $2<m_\textrm {min}<m_\textrm {max}<3$.
$2<m_\textrm {min}<m_\textrm {max}<3$.
The bad news is that, even asymptotically in ![]() $n$, there is no hope that condition (5.14) can hold as shown by the following.
$n$, there is no hope that condition (5.14) can hold as shown by the following.
Example 5.9 Take ![]() $g=\lceil ({n-1})/{3}\rceil$,
$g=\lceil ({n-1})/{3}\rceil$, ![]() $p-1=\lceil ({n-1})/{9}\rceil +1$ and
$p-1=\lceil ({n-1})/{9}\rceil +1$ and ![]() $n$ large enough. Conditions (5.6) and (5.7) are satisfied. However, both
$n$ large enough. Conditions (5.6) and (5.7) are satisfied. However, both ![]() $m_\textrm {min}^{\bullet }$ and
$m_\textrm {min}^{\bullet }$ and ![]() $m_\textrm {max}^{\bullet }$ are
$m_\textrm {max}^{\bullet }$ are ![]() $<3$, but
$<3$, but ![]() $m_\textrm {min}^{\bullet } \to _{n\to +\infty } 3$.
$m_\textrm {min}^{\bullet } \to _{n\to +\infty } 3$.
Remark 5.10 One may wonder whether a minor modification of this strategy might still lead to the existence of uniruled divisors in all cases. One possibility is to construct different rational curves coming from the Brill–Noether theory of nodal curves in the multiple hyperplane linear system of a general ![]() $K3$. This approach presents two difficulties. One has first to control the Brill–Noether theory of such nodal curves (which does not seem to be an easy task, knowing that already smooth curves in multiples of the hyperplane section are not Brill–Noether general). Second, even if one disposed of a family of
$K3$. This approach presents two difficulties. One has first to control the Brill–Noether theory of such nodal curves (which does not seem to be an easy task, knowing that already smooth curves in multiples of the hyperplane section are not Brill–Noether general). Second, even if one disposed of a family of ![]() ${\mathfrak g}^{1}_n$ on nodal curves in
${\mathfrak g}^{1}_n$ on nodal curves in ![]() $|mh_S|$ of the right dimension, the analogue of Proposition 4.1 should still hold for such a family.
$|mh_S|$ of the right dimension, the analogue of Proposition 4.1 should still hold for such a family.
5.3 Some codimension 2 coisotropic subvarieties
In this section, we look at the cases where, by [Reference Oberdieck, Shen and YinOSY19], there are no uniruled divisors ruled by primitive rational curves, and try to study the codimension of the ruled locus in this case. In particular, we have the following.
Theorem 5.11 Let ![]() $X$ be a polarized irreducible holomorphic symplectic manifold of K3
$X$ be a polarized irreducible holomorphic symplectic manifold of K3![]() $^{[n]}$-type. Let
$^{[n]}$-type. Let ![]() $C$ be a curve class such that the pair
$C$ be a curve class such that the pair ![]() $(X,C)$ is deformation equivalent to
$(X,C)$ is deformation equivalent to ![]() $(S^{[n]},h_S-(2g-1)r_n)$, where
$(S^{[n]},h_S-(2g-1)r_n)$, where ![]() $q(h_S)=2g-4$, for a certain integer
$q(h_S)=2g-4$, for a certain integer ![]() $n/2\geq g> 2$. Then
$n/2\geq g> 2$. Then ![]() $X$ has a codimension 2 locus covered by rational surfaces ruled by a primitive curve class.
$X$ has a codimension 2 locus covered by rational surfaces ruled by a primitive curve class.
This will follow from the following result, proven in [Reference Knutsen, Lelli-Chiesa and MongardiKLM19, Theorem 6.1]. We refer the reader to [Reference Knutsen, Lelli-Chiesa and MongardiKLM19] for the notation.
Theorem 5.12 [Reference Knutsen, Lelli-Chiesa and MongardiKLM19, Theorem 6.1]
Let ![]() $(S,h_S)$ be a very general primitively polarized
$(S,h_S)$ be a very general primitively polarized ![]() $K3$ surface of genus
$K3$ surface of genus ![]() $p:=p_a(h_S) \geq 2$. Let
$p:=p_a(h_S) \geq 2$. Let ![]() $0\leq g \leq p$ and
$0\leq g \leq p$ and ![]() $n \geq 2$ be integers satisfying
$n \geq 2$ be integers satisfying
Then on ![]() $S^{[n]}$ there exists a
$S^{[n]}$ there exists a ![]() $(2n-2)$-dimensional family of rational curves of class
$(2n-2)$-dimensional family of rational curves of class
which covers a subvariety birational to a ![]() $\mathbb {P}^{\chi -2(p-g)-1}$-bundle on a holomorphic symplectic manifold of dimension
$\mathbb {P}^{\chi -2(p-g)-1}$-bundle on a holomorphic symplectic manifold of dimension ![]() $2(n+1+2(p-g)-\chi )$.
$2(n+1+2(p-g)-\chi )$.
This theorem applies only to finitely many deformation types of ![]() $(X,h)$ for any dimension, but it turns out that it can be used to produce coisotropic subvarieties of codimension at least
$(X,h)$ for any dimension, but it turns out that it can be used to produce coisotropic subvarieties of codimension at least ![]() $2$ in the exceptions to Theorem 1.2.
$2$ in the exceptions to Theorem 1.2.
Proposition 5.13 Let ![]() $(X,h)$ be a polarized irreducible holomorphic symplectic manifold of K3
$(X,h)$ be a polarized irreducible holomorphic symplectic manifold of K3![]() $^{[n]}$-type and let
$^{[n]}$-type and let ![]() $C$ be the primitive curve class equal to
$C$ be the primitive curve class equal to ![]() $h/{\rm div}(h)$. Suppose that the conditions in Proposition 5.1 are not satisfied. Then there exist a polarized
$h/{\rm div}(h)$. Suppose that the conditions in Proposition 5.1 are not satisfied. Then there exist a polarized ![]() $K3$ surface
$K3$ surface ![]() $(S,h_S)$ of genus
$(S,h_S)$ of genus ![]() $p=p_a(h_S)$ and an integer
$p=p_a(h_S)$ and an integer ![]() $g\leq p$ such that:
$g\leq p$ such that:
(i)
 $p$ and
$p$ and  $g$ satisfy conditions (5.15);
$g$ satisfy conditions (5.15);(ii)
 $X$ is deformation equivalent to
$X$ is deformation equivalent to  ${S^{[n]}}$ and the class
${S^{[n]}}$ and the class  $C$ is sent to
$C$ is sent to  $h_S-(g+n-1)r_n$ by the parallel transport.
$h_S-(g+n-1)r_n$ by the parallel transport.
Proof. By Corollary 2.8 the pair ![]() $(X,C)$ is deformation equivalent to
$(X,C)$ is deformation equivalent to ![]() $(\Sigma ^{[n]}, h_\Sigma -(2\gamma -\epsilon )r_n )$, where
$(\Sigma ^{[n]}, h_\Sigma -(2\gamma -\epsilon )r_n )$, where ![]() $\Sigma$ is a
$\Sigma$ is a ![]() $K3$ surface of genus
$K3$ surface of genus ![]() $\pi :=p_a(h_\Sigma )$,
$\pi :=p_a(h_\Sigma )$, ![]() $\gamma$ a positive integer and
$\gamma$ a positive integer and ![]() $\epsilon = 0, 1$. As the conditions in Proposition 5.1 are not satisfied, by Proposition 5.2 we have
$\epsilon = 0, 1$. As the conditions in Proposition 5.1 are not satisfied, by Proposition 5.2 we have ![]() $p_a(h_\Sigma )< \gamma$. By Remark 2.9 we can choose as system of representatives of
$p_a(h_\Sigma )< \gamma$. By Remark 2.9 we can choose as system of representatives of ![]() ${{\mathbb {Z}}}/(2n-2){{\mathbb {Z}}}$, up to the action of
${{\mathbb {Z}}}/(2n-2){{\mathbb {Z}}}$, up to the action of ![]() $-1$, the set
$-1$, the set ![]() $[2n-2, 3n-3]\cap {{\mathbb {N}}}$. Hence, we can deform to a different punctual Hilbert scheme on a polarized
$[2n-2, 3n-3]\cap {{\mathbb {N}}}$. Hence, we can deform to a different punctual Hilbert scheme on a polarized ![]() $K3$ surface
$K3$ surface ![]() $(S,h_S)$ such that our curve has class
$(S,h_S)$ such that our curve has class ![]() $h_S-(2n-2+2\gamma -\epsilon )r_n$ and the genus
$h_S-(2n-2+2\gamma -\epsilon )r_n$ and the genus ![]() $p$ of
$p$ of ![]() $S$ is
$S$ is ![]() $p=\pi +n-1+2\gamma -\epsilon$. Set
$p=\pi +n-1+2\gamma -\epsilon$. Set ![]() $g:=n-1+2\gamma -\epsilon$.
$g:=n-1+2\gamma -\epsilon$.
It follows that ![]() $\chi =2\gamma +2-\epsilon$. The conditions we must check are
$\chi =2\gamma +2-\epsilon$. The conditions we must check are
As ![]() $p-g=\pi <\gamma$ and
$p-g=\pi <\gamma$ and ![]() $2\gamma -\epsilon \leq n-1$, they are always satisfied and Theorem 5.12 applies, giving the desired locus of codimension
$2\gamma -\epsilon \leq n-1$, they are always satisfied and Theorem 5.12 applies, giving the desired locus of codimension ![]() ${\chi -2(p-g)-1}\geq 2$.
${\chi -2(p-g)-1}\geq 2$.
Proof of Theorem 5.11 Let ![]() $C$ be as in the statement. The conditions of Proposition 5.1 are not satisfied, as
$C$ be as in the statement. The conditions of Proposition 5.1 are not satisfied, as ![]() $p_a(h_S)=g-1$. Hence, we can apply Proposition 5.13 and obtain a new pair
$p_a(h_S)=g-1$. Hence, we can apply Proposition 5.13 and obtain a new pair ![]() $(S_1^{[n]}, h_{S_1}-((2g-1+n-1)+n-1)r_n)$ satisfying conditions (5.15). Therefore, we can apply Theorem 5.12 to
$(S_1^{[n]}, h_{S_1}-((2g-1+n-1)+n-1)r_n)$ satisfying conditions (5.15). Therefore, we can apply Theorem 5.12 to ![]() $(S_1^{[n]}, h_{S_1}-((2g-1+n-1)+n-1)r_n)$ to deduce the existence of a
$(S_1^{[n]}, h_{S_1}-((2g-1+n-1)+n-1)r_n)$ to deduce the existence of a ![]() $(2n-2)$-dimensional family of rational curves covering a coisotropic subvariety. By Proposition 3.1 these rational curves deform to the initial variety
$(2n-2)$-dimensional family of rational curves covering a coisotropic subvariety. By Proposition 3.1 these rational curves deform to the initial variety ![]() $X$ and by construction they have class equal to
$X$ and by construction they have class equal to ![]() $C$. By [Reference Oberdieck, Shen and YinOSY19], the codimension of the locus covered by their deformations on
$C$. By [Reference Oberdieck, Shen and YinOSY19], the codimension of the locus covered by their deformations on ![]() $X$ cannot be less than
$X$ cannot be less than ![]() $2$. Thus, if it is
$2$. Thus, if it is ![]() $2$ on
$2$ on ![]() $S_1^{[n]}$ that must be the case also on a general point
$S_1^{[n]}$ that must be the case also on a general point ![]() $X'$ in the component of the moduli space
$X'$ in the component of the moduli space ![]() $\mathfrak M$ containing
$\mathfrak M$ containing ![]() $(X,C)$. Observe that, in this case, we have
$(X,C)$. Observe that, in this case, we have ![]() $\chi =2g+1$ and the locus covered by these rational curves has therefore codimension
$\chi =2g+1$ and the locus covered by these rational curves has therefore codimension ![]() $2g+1-2g+1=2$. Hence, we have a coisotropic subvariety
$2g+1-2g+1=2$. Hence, we have a coisotropic subvariety ![]() $Z'\subset X'$ covered by rationally chain connected surfaces
$Z'\subset X'$ covered by rationally chain connected surfaces ![]() $F'$. The flat limit
$F'$. The flat limit ![]() $Z\subset X$ of
$Z\subset X$ of ![]() $Z'$ is covered by the flat limits
$Z'$ is covered by the flat limits ![]() $F$ of the RCC surfaces
$F$ of the RCC surfaces ![]() $F'$ and of course
$F'$ and of course ![]() $F$ is RCC. The theorem follows.
$F$ is RCC. The theorem follows.
Remark 5.14 For ![]() $n=8,9$, where the first exceptions discovered by [Reference Oberdieck, Shen and YinOSY19] appear, Theorem 5.11 applies. This is not the case for the exceptions of classes
$n=8,9$, where the first exceptions discovered by [Reference Oberdieck, Shen and YinOSY19] appear, Theorem 5.11 applies. This is not the case for the exceptions of classes ![]() $h_S-8r_{10}$ and
$h_S-8r_{10}$ and ![]() $h_S-10r_{10}$ in dimension
$h_S-10r_{10}$ in dimension ![]() $20$ (cf. Theorem 5.8).
$20$ (cf. Theorem 5.8).
6. Application to  $0$-cycles
$0$-cycles
In what follows, we always consider Chow groups with rational coefficients. Throughout this section, let ![]() $X$ be an irreducible holomorphic symplectic variety. If
$X$ be an irreducible holomorphic symplectic variety. If ![]() $Y$ is a variety, let
$Y$ is a variety, let ![]() $CH_0(Y)_{{\rm hom}}$ be the subgroup of
$CH_0(Y)_{{\rm hom}}$ be the subgroup of ![]() $CH_0(Y)$ consisting of
$CH_0(Y)$ consisting of ![]() $0$-cycles of degree
$0$-cycles of degree ![]() $0$.
$0$.
Definition 6.1 Let ![]() $D$ be an irreducible divisor on
$D$ be an irreducible divisor on ![]() $X$. We denote by
$X$. We denote by ![]() $S_1 CH_0(X)_{D,{\rm hom}}$ the subgroup
$S_1 CH_0(X)_{D,{\rm hom}}$ the subgroup
of ![]() $CH_0(X)$. We denote by
$CH_0(X)$. We denote by ![]() $S_1 CH_0(X)_{D}$ the subgroup
$S_1 CH_0(X)_{D}$ the subgroup
of ![]() $CH_0(X)$.
$CH_0(X)$.
Lemma 6.2 Let D and ![]() $D'$ be two irreducible uniruled divisors on X and let R and
$D'$ be two irreducible uniruled divisors on X and let R and ![]() $R'$ be the general curves in the respective rulings. If
$R'$ be the general curves in the respective rulings. If ![]() $DR'\neq 0$ and
$DR'\neq 0$ and ![]() $D'R\neq 0$, then
$D'R\neq 0$, then ![]() $S_1CH_0(X)_D=S_1CH_0(X)_{D'}$ and
$S_1CH_0(X)_D=S_1CH_0(X)_{D'}$ and ![]() $S_1CH_0(X)_{D, {\rm hom}}=S_1CH_0(X)_{D', {\rm hom}}$.
$S_1CH_0(X)_{D, {\rm hom}}=S_1CH_0(X)_{D', {\rm hom}}$.
Proof. Let ![]() $\pi : \widetilde{D}\rightarrow T$ and
$\pi : \widetilde{D}\rightarrow T$ and ![]() $\pi' : \widetilde{D}'\rightarrow T'$ be rulings of varieties
$\pi' : \widetilde{D}'\rightarrow T'$ be rulings of varieties ![]() $\widetilde{D}$ and
$\widetilde{D}$ and ![]() $\widetilde{D}'$ mapping finitely to D and
$\widetilde{D}'$ mapping finitely to D and ![]() $D'$, respectively. The curves R and
$D'$, respectively. The curves R and ![]() $R'$ are the images of the general fibers of
$R'$ are the images of the general fibers of ![]() $\pi$ and
$\pi$ and ![]() $\pi'$ respectively. As a consequence of the hypothesis, both projections in the diagram
$\pi'$ respectively. As a consequence of the hypothesis, both projections in the diagram

are surjective, which implies that
and
Proof of Theorem 1.7 We give the proof for ![]() $S_1 CH_0(X)_{D}$. The proof for
$S_1 CH_0(X)_{D}$. The proof for ![]() $S_1 CH_0(X)_{D,{\rm hom}}$ is exactly the same.
$S_1 CH_0(X)_{D,{\rm hom}}$ is exactly the same.
Applying Corollary 3.5, we may find an ample divisor H, ruled by a rational curve of class ![]() $\alpha$, Poincaré dual to that of H. First note that, since H is ample, for any index i we have
$\alpha$, Poincaré dual to that of H. First note that, since H is ample, for any index i we have
Then, by Lemma 6.2 we conclude that the groups ![]() $S_1CH_0(X)_{H_i}$ are independent of i.
$S_1CH_0(X)_{H_i}$ are independent of i.
Let D be an irreducible uniruled divisor on X and let R be a general curve of its ruling. Since H is ample, we may find an integer i such that ![]() $H_iR\neq 0$. Furthermore, as above, we have that
$H_iR\neq 0$. Furthermore, as above, we have that ![]() $R_iD\neq 0$. By Lemma 6.2, we obtain the equality
$R_iD\neq 0$. By Lemma 6.2, we obtain the equality
which concludes the proof.
Thanks to Theorem 1.7 we can drop the dependence on ![]() $D$ from the notation and, in what follows, under the same hypotheses, we will simply write
$D$ from the notation and, in what follows, under the same hypotheses, we will simply write ![]() $S_1 CH_0(X)$ and
$S_1 CH_0(X)$ and ![]() $S_1 CH_0(X)_{{\rm hom}}$ for the groups
$S_1 CH_0(X)_{{\rm hom}}$ for the groups ![]() $S_1CH_0(X)_D$ and
$S_1CH_0(X)_D$ and ![]() $S_1CH_0(X)_{D, {\rm hom}}$.
$S_1CH_0(X)_{D, {\rm hom}}$.
Proposition 6.3 Let X be a projective holomorphic symplectic variety, and let D be an irreducible uniruled divisor on X. Suppose that X possesses an ample ruling curve R. Then
Proof. We give the proof for ![]() $S_1 CH_0(X)_{{\rm hom}}$. Basic intersection theory [Reference FultonFul98, chapter 6] guarantees the inclusion
$S_1 CH_0(X)_{{\rm hom}}$. Basic intersection theory [Reference FultonFul98, chapter 6] guarantees the inclusion
To prove the other inclusion consider any irreducible uniruled component ![]() $H_{i}$ of the ample divisor H ruled by R. By Theorem 1.7 we have
$H_{i}$ of the ample divisor H ruled by R. By Theorem 1.7 we have ![]() $S_1 CH_0(X)_{D, {\rm hom}}=S_1 CH_0(X)_{H_{i_0}, {\rm hom}}$. Notice, moreover, that by the hypothesis,
$S_1 CH_0(X)_{D, {\rm hom}}=S_1 CH_0(X)_{H_{i_0}, {\rm hom}}$. Notice, moreover, that by the hypothesis,
Consider
where the ![]() $x_k$ lie in
$x_k$ lie in ![]() $H_{i_0}$. For each
$H_{i_0}$. For each ![]() $x_k$, let
$x_k$, let ![]() $R_{x_k}$ be a curve in the ruling of
$R_{x_k}$ be a curve in the ruling of ![]() $H_{i}$ containing it. Then, by (6.2), there exists a rational number
$H_{i}$ containing it. Then, by (6.2), there exists a rational number ![]() $\mu>0$, independent of k, such that
$\mu>0$, independent of k, such that
Hence
holds in ![]() $CH_0(D)$, from which we see that
$CH_0(D)$, from which we see that
Proof of Theorem 1.8 Write ![]() $L=\sum m_i D_i$, where
$L=\sum m_i D_i$, where ![]() $D_i$ is irreducible and uniruled for all
$D_i$ is irreducible and uniruled for all ![]() $i$. We have
$i$. We have
where the last equality holds thanks to Proposition 6.3 (note that we do not automatically have the equality, because some of the ![]() $m_i$ may be negative).
$m_i$ may be negative).
To prove the other inclusion we argue as in Proposition 6.3. We take an irreducible uniruled divisor D such that ![]() $L\cdot R_D \not =0$, where
$L\cdot R_D \not =0$, where ![]() $R_D$ is a curve in the ruling of D. Such a divisor exists by the hypothesis, as we can take any irreducible component of the divisor H ruled by the ample curve R. Let
$R_D$ is a curve in the ruling of D. Such a divisor exists by the hypothesis, as we can take any irreducible component of the divisor H ruled by the ample curve R. Let ![]() $Z:=\sum n_i x_i\in \mathop{\rm Im}\nolimits (CH_0(D)_{{\rm hom}}\to CH_0(X))=S_1CH_0(X)$. Then the equality
$Z:=\sum n_i x_i\in \mathop{\rm Im}\nolimits (CH_0(D)_{{\rm hom}}\to CH_0(X))=S_1CH_0(X)$. Then the equality
holds in ![]() $CH_0(X)$ for some rational number
$CH_0(X)$ for some rational number ![]() $\lambda$, hence
$\lambda$, hence
7. Some open questions
We briefly discuss some questions raised by our results. Theorem 1.2 suggests a natural extension to general projective holomorphic symplectic varieties in the following way.
Question 7.1 Let ![]() $X$ be a projective holomorphic symplectic variety of dimension
$X$ be a projective holomorphic symplectic variety of dimension ![]() $2n$, and let
$2n$, and let ![]() $k$ be an integer between
$k$ be an integer between ![]() $0$ and
$0$ and ![]() $n$. Does there exist a subscheme
$n$. Does there exist a subscheme ![]() $Y_k$ of
$Y_k$ of ![]() $X$ of pure dimension
$X$ of pure dimension ![]() $2n-k$ such that its
$2n-k$ such that its ![]() $0$-cycles are supported in dimension
$0$-cycles are supported in dimension ![]() $2n-2k$?
$2n-2k$?
This question has been put into a larger perspective by Voisin, in [Reference VoisinVoi16], as a key step towards the construction of a multiplicative splitting in the Chow group. In view of our constructions, it seems natural to hope for a positive answer for Question 7.1. Note that this is the case if ![]() $X$ is of the form
$X$ is of the form ![]() $S^{[n]}$ for some
$S^{[n]}$ for some ![]() $K3$ surface
$K3$ surface ![]() $S$ as follows by taking
$S$ as follows by taking ![]() $Y$ to be the closure in
$Y$ to be the closure in ![]() $S^{[n]}$ of the locus of points
$S^{[n]}$ of the locus of points ![]() $s_1+\cdots +s_n$, where the
$s_1+\cdots +s_n$, where the ![]() $s_i$ are distinct points of
$s_i$ are distinct points of ![]() $S$,
$S$, ![]() $k$ of which lie on a given rational curve of
$k$ of which lie on a given rational curve of ![]() $S$.
$S$.
It would be interesting to refine Question 7.1 to specify the expected cohomology classes of the subschemes ![]() $Y_k$.
$Y_k$.
The particular case of middle-dimensional subschemes seems of special interest in view of the study of rational equivalence on holomorphic symplectic varieties.
Question 7.2 Let ![]() $X$ be a projective holomorphic symplectic variety of dimension
$X$ be a projective holomorphic symplectic variety of dimension ![]() $2n$. Does there exist a rationally connected subvariety
$2n$. Does there exist a rationally connected subvariety ![]() $Y$ of
$Y$ of ![]() $X$ such that
$X$ such that ![]() $Y$ has dimension
$Y$ has dimension ![]() $n$ and non-zero self-intersection ?
$n$ and non-zero self-intersection ?
A positive answer to Question 7.2 would lead to the existence of a canonical ![]() $0$-cycle of degree
$0$-cycle of degree ![]() $1$ on
$1$ on ![]() $X$, as in the case of
$X$, as in the case of ![]() $K3$ surfaces. This raises the following question.
$K3$ surfaces. This raises the following question.
Question 7.3 Assume that Question 7.2 has a positive answer for ![]() $X$ and let
$X$ and let ![]() $y$ be any point of
$y$ be any point of ![]() $Y$. Let
$Y$. Let ![]() $H_1, \ldots , H_r$ be divisors on
$H_1, \ldots , H_r$ be divisors on ![]() $X$, and let
$X$, and let ![]() $k_1, \ldots , k_{n}$ be non-negative integers such that
$k_1, \ldots , k_{n}$ be non-negative integers such that ![]() $r+\sum _i 2ik_i =2n$.
$r+\sum _i 2ik_i =2n$.
Do we have
inside ![]() $CH_0(X)$ ?
$CH_0(X)$ ?
The existence of a degree ![]() $1$
$1$ ![]() $0$-cycle
$0$-cycle ![]() $c_X$ verifying the equality (7.1) is a consequence of the Beauville conjecture. We wonder whether such a
$c_X$ verifying the equality (7.1) is a consequence of the Beauville conjecture. We wonder whether such a ![]() $0$-cycle can be realized in a geometrically meaningful way as a point on a rationally connected half-dimensional subvariety.
$0$-cycle can be realized in a geometrically meaningful way as a point on a rationally connected half-dimensional subvariety.
Even in the case of a general polarized fourfold of ![]() $K3^{[2]}$-type, we do not know the answer to the preceding questions.
$K3^{[2]}$-type, we do not know the answer to the preceding questions.
Finally, Question 7.1 raises a counting problem as in the case of the Yau–Zaslow conjecture for rational curves on ![]() $K3$ surfaces [Reference Yau and ZaslowYZ96], which was solved in [Reference Klemm, Maulik, Pandharipande and ScheideggerKMPS10]. We do not know of a precise formulation for this question.
$K3$ surfaces [Reference Yau and ZaslowYZ96], which was solved in [Reference Klemm, Maulik, Pandharipande and ScheideggerKMPS10]. We do not know of a precise formulation for this question.
Acknowledgments
We thank D. Huybrechts and C. Voisin for interesting discussions on the subject of this paper. In particular, C. Voisin kindly suggested the applications to Chow groups presented here. We are grateful to E. Markman for enlightening discussions and comments, and for sharing with us some unpublished notes of his. We are grateful to A. Apostolov for his help with Theorem 2.5. We thank Q. Yin for valuable discussions on [Reference Oberdieck, Shen and YinOSY19]. Thanks also go to G. Ancona for a useful suggestion. We thank G. Saccà for pointing out to us that the proof of former Corollary 3.6 was incomplete, which led us to modify the manuscript accordingly.
F.C. is supported by the European Research Council (ERC) under the European Union's Horizon 2020 research and innovation programme (grant agreement No. 715747). G.M. is supported by ‘Progetto di ricerca INdAM per giovani ricercatori: Pursuit of IHS’ and would like to thank the Université de Lorraine for hosting him while this work was finished. G.P. was partially supported by the project ANR-16-CE40-0008 ‘Foliage’, the ANR project ‘CLASS’ no. ANR-10-JCJC-0111 and the University of Strasbourg Institute for Advanced Study (USIAS) as part of a USIAS Fellowship.
Conflicts of Interest
None.