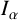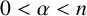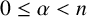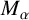1. Introduction
The purpose of this paper is to study the boundedness properties of the fractional integral operator
![]() $I_{\alpha }$
,
$I_{\alpha }$
,
![]() $0<\alpha <n$
, and the fractional maximal operator
$0<\alpha <n$
, and the fractional maximal operator
![]() $M_{\alpha }$
,
$M_{\alpha }$
,
![]() $0\le \alpha <n$
, in the framework of Choquet integrals with respect to Hausdorff content.
$0\le \alpha <n$
, in the framework of Choquet integrals with respect to Hausdorff content.
Let
![]() $n\in {\mathbb N}$
and
$n\in {\mathbb N}$
and
![]() $0<d\le n$
. The symbol
$0<d\le n$
. The symbol
![]() ${\mathcal Q}({\mathbb R}^n)$
denotes the family of all cubes with sides parallel to the coordinate axes in
${\mathcal Q}({\mathbb R}^n)$
denotes the family of all cubes with sides parallel to the coordinate axes in
![]() ${\mathbb R}^n$
. The d-dimensional Hausdorff content of
${\mathbb R}^n$
. The d-dimensional Hausdorff content of
![]() $E\subset {\mathbb R}^n$
is defined by
$E\subset {\mathbb R}^n$
is defined by
 $$ \begin{align*} H^d(E) = \inf\bigg\{ \sum_{j=1}^{{\infty}}\ell(Q_j)^d:\, E\subset\bigcup_{j=1}^{{\infty}}Q_j, Q_j\in{\mathcal Q}({\mathbb R}^n) \bigg\}, \end{align*} $$
$$ \begin{align*} H^d(E) = \inf\bigg\{ \sum_{j=1}^{{\infty}}\ell(Q_j)^d:\, E\subset\bigcup_{j=1}^{{\infty}}Q_j, Q_j\in{\mathcal Q}({\mathbb R}^n) \bigg\}, \end{align*} $$
where the infimum is taken over all coverings of the set E by countable families of cubes
![]() $Q_j$
and
$Q_j$
and
![]() $\ell (Q)$
stands for the side length of the cube Q. It is easily seen that
$\ell (Q)$
stands for the side length of the cube Q. It is easily seen that
![]() $H^n(E)$
is just the Lebesgue measure of E, which we will denote by
$H^n(E)$
is just the Lebesgue measure of E, which we will denote by
![]() $|E|$
. For any cube Q, one has
$|E|$
. For any cube Q, one has
![]() $H^d(Q)=\ell (Q)^d$
.
$H^d(Q)=\ell (Q)^d$
.
For a nonnegative function f, the integral of f with the respect to
![]() $H^d$
is taken in the Choquet sense:
$H^d$
is taken in the Choquet sense:
 $$ \begin{align*} \int_{{\mathbb R}^n}f\,{ d}H^d = \int_0^{{\infty}} H^d(\{ x\in {\mathbb R}^n:\,f(x)>t \})\,{d}t. \end{align*} $$
$$ \begin{align*} \int_{{\mathbb R}^n}f\,{ d}H^d = \int_0^{{\infty}} H^d(\{ x\in {\mathbb R}^n:\,f(x)>t \})\,{d}t. \end{align*} $$
For
![]() $0<p<{\infty }$
, the Choquet space
$0<p<{\infty }$
, the Choquet space
![]() $L^p(H^d)$
and the weak Choquet space
$L^p(H^d)$
and the weak Choquet space
![]() $\mathrm {w}\hskip -0.6pt{L}^p(H^d)$
consist of all functions with the properties
$\mathrm {w}\hskip -0.6pt{L}^p(H^d)$
consist of all functions with the properties
 $$ \begin{align*} \|f\|_{L^p(H^d)} &:= \bigg( \int_{{\mathbb R}^n}|f|^p\,{ d}H^d \bigg)^{1/p}\!<{\infty} \end{align*} $$
$$ \begin{align*} \|f\|_{L^p(H^d)} &:= \bigg( \int_{{\mathbb R}^n}|f|^p\,{ d}H^d \bigg)^{1/p}\!<{\infty} \end{align*} $$
and
respectively. For
![]() $0<q\le p<{\infty }$
, the Choquet–Morrey space
$0<q\le p<{\infty }$
, the Choquet–Morrey space
![]() ${\mathcal M}^p_q(H^d)$
consists of all functions with the property
${\mathcal M}^p_q(H^d)$
consists of all functions with the property
 $$ \begin{align*} \|f\|_{{\mathcal M}^p_q(H^d)} := \sup_{Q\in{\mathcal Q}} \ell(Q)^{d/p- d/q} \bigg( \int_{Q}|f|^q\,{ d}H^d \bigg)^{1/q}\!<{\infty}. \end{align*} $$
$$ \begin{align*} \|f\|_{{\mathcal M}^p_q(H^d)} := \sup_{Q\in{\mathcal Q}} \ell(Q)^{d/p- d/q} \bigg( \int_{Q}|f|^q\,{ d}H^d \bigg)^{1/q}\!<{\infty}. \end{align*} $$
The fractional maximal operator of order
![]() $\alpha $
,
$\alpha $
,
![]() $0\le \alpha <n$
, is defined by
$0\le \alpha <n$
, is defined by
 $$ \begin{align*} M_{\alpha}f(x) = \sup_{Q\in{\mathcal Q}({\mathbb R}^n)} \chi_{Q}(x) \ell(Q)^{\alpha-n}\int_{Q}|f(y)|\,{ d}y, \quad x\in{\mathbb R}^n, \end{align*} $$
$$ \begin{align*} M_{\alpha}f(x) = \sup_{Q\in{\mathcal Q}({\mathbb R}^n)} \chi_{Q}(x) \ell(Q)^{\alpha-n}\int_{Q}|f(y)|\,{ d}y, \quad x\in{\mathbb R}^n, \end{align*} $$
where
![]() $\chi _{E}$
is the characteristic function of the set E. For
$\chi _{E}$
is the characteristic function of the set E. For
![]() $\alpha =0$
, the operator
$\alpha =0$
, the operator
![]() $M_0$
is the usual Hardy–Littlewood maximal operator which is denoted simply by M. The fractional integral operator of order
$M_0$
is the usual Hardy–Littlewood maximal operator which is denoted simply by M. The fractional integral operator of order
![]() $\alpha $
,
$\alpha $
,
![]() $0<\alpha <n$
, is defined by
$0<\alpha <n$
, is defined by
 $$ \begin{align*} I_{\alpha}f(x) = \int_{{\mathbb R}^n} \frac{f(y)}{|x-y|^{n-\alpha}} \,{ d}y,\quad x\in{\mathbb R}^n. \end{align*} $$
$$ \begin{align*} I_{\alpha}f(x) = \int_{{\mathbb R}^n} \frac{f(y)}{|x-y|^{n-\alpha}} \,{ d}y,\quad x\in{\mathbb R}^n. \end{align*} $$
Orobitg and Verdera [Reference Orobitg and Verdera6] proved that, for
![]() $0<d<n$
and
$0<d<n$
and
![]() $p>d/n$
,
$p>d/n$
,
The tools and ideas that we will use are essentially contained in this classical paper.
We note a disadvantage of the Hausdorff content
![]() $H^d$
. It is not true that there exists a constant
$H^d$
. It is not true that there exists a constant
![]() $C>0$
such that if
$C>0$
such that if
![]() $Q_1,\ldots ,Q_m$
are nonoverlapping dyadic cubes and
$Q_1,\ldots ,Q_m$
are nonoverlapping dyadic cubes and
![]() $f\ge 0$
, then
$f\ge 0$
, then
 $$ \begin{align*} \sum_{j=1}^m\int_{Q_j}f\,{ d}H^d \le C \int_{\bigcup_jQ_j}f\,{ d}H^d. \end{align*} $$
$$ \begin{align*} \sum_{j=1}^m\int_{Q_j}f\,{ d}H^d \le C \int_{\bigcup_jQ_j}f\,{ d}H^d. \end{align*} $$
This can be shown by subdividing the interval
![]() $[0, 1]$
into
$[0, 1]$
into
![]() $2^m$
(m large enough) equal intervals and taking
$2^m$
(m large enough) equal intervals and taking
![]() $f\equiv 1$
.
$f\equiv 1$
.
However, an advantage of the Hausdorff content
![]() $H^d$
is that the spaces
$H^d$
is that the spaces
![]() $L^p(H^d)$
,
$L^p(H^d)$
,
![]() $0<p\le 1$
, have a block decomposition. As a corollary, (see [Reference Saito, Tanaka and Watanabe8, Theorem 2.3]), if T is a subadditive operator and
$0<p\le 1$
, have a block decomposition. As a corollary, (see [Reference Saito, Tanaka and Watanabe8, Theorem 2.3]), if T is a subadditive operator and
![]() $0<d,\delta <n$
,
$0<d,\delta <n$
,
![]() $0<p\le 1$
and
$0<p\le 1$
and
![]() $q\ge p$
, then the following statements are equivalent:
$q\ge p$
, then the following statements are equivalent:
-
(a) the inequality
 $\displaystyle \|Tf\|_{L^q(H^{\delta })} \le C_1 \|f\|_{L^p(H^d)}$
holds;
$\displaystyle \|Tf\|_{L^q(H^{\delta })} \le C_1 \|f\|_{L^p(H^d)}$
holds; -
(b) the testing inequality
 $\displaystyle \|T\chi _{Q}\|_{L^q(H^{\delta })} \le C_2\ell (Q)^{1/p}$
holds for any
$\displaystyle \|T\chi _{Q}\|_{L^q(H^{\delta })} \le C_2\ell (Q)^{1/p}$
holds for any
 $Q\in {\mathcal Q}({\mathbb R}^n)$
.
$Q\in {\mathcal Q}({\mathbb R}^n)$
.
Moreover, the least possible constants
![]() $C_1$
and
$C_1$
and
![]() $C_2$
are equivalent. Because of this advantage, by using an easy testing inequality, one can verify the following results from [Reference Adams2]. For
$C_2$
are equivalent. Because of this advantage, by using an easy testing inequality, one can verify the following results from [Reference Adams2]. For
![]() $d/n<p<d/\alpha $
,
$d/n<p<d/\alpha $
,
For
![]() $0<d\le n$
,
$0<d\le n$
,
The reason why the advantage influences the cases
![]() $p>1$
is that, for the fractional maximal operator
$p>1$
is that, for the fractional maximal operator
![]() $M_{\alpha }$
, the pointwise estimate
$M_{\alpha }$
, the pointwise estimate
gives a reduction to the case
![]() $p=1$
. This no longer works for the fractional integral operator
$p=1$
. This no longer works for the fractional integral operator
![]() $I_{\alpha }$
. The difficulty is overcome by using Hedberg’s trick (see Lemma 2.7), which is due to the first author (Hatano).
$I_{\alpha }$
. The difficulty is overcome by using Hedberg’s trick (see Lemma 2.7), which is due to the first author (Hatano).
Theorem 1.1. Let
![]() $0<d\le n$
and
$0<d\le n$
and
![]() $0\le \alpha <n$
. Suppose that
$0\le \alpha <n$
. Suppose that
![]() $d/n\le r<p<d/\alpha $
and
$d/n\le r<p<d/\alpha $
and
 $$ \begin{align*} \frac{d-\alpha r}{q} = \frac{d-\alpha p}{p}. \end{align*} $$
$$ \begin{align*} \frac{d-\alpha r}{q} = \frac{d-\alpha p}{p}. \end{align*} $$
Then:
-
(i)
 $\|M_{\alpha }f\|_{\mathrm {w}\hskip -0.6pt{L}^q(H^{d-\alpha r})} \lesssim \|f\|_{\mathrm {w}\hskip -0.6pt{L}^p(H^d)}$
;
$\|M_{\alpha }f\|_{\mathrm {w}\hskip -0.6pt{L}^q(H^{d-\alpha r})} \lesssim \|f\|_{\mathrm {w}\hskip -0.6pt{L}^p(H^d)}$
; -
(ii)
 $\|I_{\alpha }f\|_{\mathrm {w}\hskip -0.6pt{L}^q(H^{d-\alpha r})} \lesssim \|f\|_{\mathrm {w}\hskip -0.6pt{L}^p(H^d)}$
for
$\|I_{\alpha }f\|_{\mathrm {w}\hskip -0.6pt{L}^q(H^{d-\alpha r})} \lesssim \|f\|_{\mathrm {w}\hskip -0.6pt{L}^p(H^d)}$
for
 $0<d<n$
,
$0<d<n$
,
 $0<\alpha <n$
and
$0<\alpha <n$
and
 $d/n<r$
.
$d/n<r$
. -
(iii)
 $\|I_{\alpha }f\|_{L^q(H^{d-\alpha r})} \lesssim \|f\|_{L^p(H^d)}$
for
$\|I_{\alpha }f\|_{L^q(H^{d-\alpha r})} \lesssim \|f\|_{L^p(H^d)}$
for
 $0<d<n$
,
$0<d<n$
,
 $0<\alpha <n$
and
$0<\alpha <n$
and
 $d/n<r$
.
$d/n<r$
.
Remark 1.2. Taking into account (1.1), one might expect that Theorem 1.1(i) holds for the case
![]() $q=r=p$
. However, this is not so because, as a special case, it would give the false inequality
$q=r=p$
. However, this is not so because, as a special case, it would give the false inequality
![]() $\|M_{\alpha }f\|_{\mathrm {w}\hskip -0.6pt{L}^1(H^{n-\alpha })} \lesssim \|f\|_{\mathrm {w}\hskip -0.6pt{L}^1(H^n)}$
.
$\|M_{\alpha }f\|_{\mathrm {w}\hskip -0.6pt{L}^1(H^{n-\alpha })} \lesssim \|f\|_{\mathrm {w}\hskip -0.6pt{L}^1(H^n)}$
.
The key ingredient in the proof of Theorem 1.1(i) is a Kolmogorov-type inequality: for any measurable set
![]() $E\subset {\mathbb R}^n$
,
$E\subset {\mathbb R}^n$
,
 $$ \begin{align*} H^d(E)^{1/p-1/q} \|f\chi_{E}\|_{L^q(H^d)} \le \bigg(\frac{q}{p-q}\bigg)^{1/p} \|f\chi_{E}\|_{\mathrm{w}\hskip-0.6pt{L}^p(H^d)}, \quad 0<q<p<{\infty}. \end{align*} $$
$$ \begin{align*} H^d(E)^{1/p-1/q} \|f\chi_{E}\|_{L^q(H^d)} \le \bigg(\frac{q}{p-q}\bigg)^{1/p} \|f\chi_{E}\|_{\mathrm{w}\hskip-0.6pt{L}^p(H^d)}, \quad 0<q<p<{\infty}. \end{align*} $$
This means that weak
![]() $L^p$
integrability implies local
$L^p$
integrability implies local
![]() $L^q$
integrability provided that
$L^q$
integrability provided that
![]() ${q<p}$
. In the above inequality, taking the supremum over all measurable sets, we can obtain the reverse inequality and, as a consequence, the weak Choquet norm can be estimated as follows (see Proposition 2.5). For
${q<p}$
. In the above inequality, taking the supremum over all measurable sets, we can obtain the reverse inequality and, as a consequence, the weak Choquet norm can be estimated as follows (see Proposition 2.5). For
![]() $0<q<p<{\infty }$
,
$0<q<p<{\infty }$
,
 $$ \begin{align*} \|f\|_{\mathrm{w}\hskip-0.6pt{L}^p(H^d)} \le \sup_{0<H^d(E)<{\infty}} H^d(E)^{1/p-1/q} \|f\chi_{E}\|_{L^q(H^d)} \le \bigg(\frac{q}{p-q}\bigg)^{1/p} \|f\|_{\mathrm{w}\hskip-0.6pt{L}^p(H^d)}. \end{align*} $$
$$ \begin{align*} \|f\|_{\mathrm{w}\hskip-0.6pt{L}^p(H^d)} \le \sup_{0<H^d(E)<{\infty}} H^d(E)^{1/p-1/q} \|f\chi_{E}\|_{L^q(H^d)} \le \bigg(\frac{q}{p-q}\bigg)^{1/p} \|f\|_{\mathrm{w}\hskip-0.6pt{L}^p(H^d)}. \end{align*} $$
We notice that the parameter q does not affect the set
![]() $\mathrm {w}\hskip -0.6pt{L}^p(H^d)$
. However, in the above supremum, if one restricts to the cube
$\mathrm {w}\hskip -0.6pt{L}^p(H^d)$
. However, in the above supremum, if one restricts to the cube
![]() $Q\in {\mathcal Q}({\mathbb R}^n)$
instead of the general set
$Q\in {\mathcal Q}({\mathbb R}^n)$
instead of the general set
![]() $E\subset {\mathbb R}^n$
, then one gets Morrey spaces and one can no longer ignore the influence of the second parameter q. We establish the following results. The first part is from [Reference Tang10, Theorem 2].
$E\subset {\mathbb R}^n$
, then one gets Morrey spaces and one can no longer ignore the influence of the second parameter q. We establish the following results. The first part is from [Reference Tang10, Theorem 2].
Theorem 1.3. Let
![]() $0<d\le n$
and
$0<d\le n$
and
![]() $0\le \alpha <n$
. Suppose that
$0\le \alpha <n$
. Suppose that
![]() $d/n<r\le p<d/\alpha $
and
$d/n<r\le p<d/\alpha $
and
 $$ \begin{align*} \frac{d-\alpha r}{q} = \frac{d-\alpha p}{p}. \end{align*} $$
$$ \begin{align*} \frac{d-\alpha r}{q} = \frac{d-\alpha p}{p}. \end{align*} $$
Then:
-
(i)
 $\|M_{\alpha }f\|_{{\mathcal M}^q_r(H^{d-\alpha r})} \lesssim \|f\|_{{\mathcal M}^p_r(H^d)}$
;
$\|M_{\alpha }f\|_{{\mathcal M}^q_r(H^{d-\alpha r})} \lesssim \|f\|_{{\mathcal M}^p_r(H^d)}$
; -
(ii)
 $\|I_{\alpha }f\|_{{\mathcal M}^q_r(H^{d-\alpha r})} \lesssim \|f\|_{{\mathcal M}^p_r(H^d)}$
for
$\|I_{\alpha }f\|_{{\mathcal M}^q_r(H^{d-\alpha r})} \lesssim \|f\|_{{\mathcal M}^p_r(H^d)}$
for
 $0<d<n$
and
$0<d<n$
and
 $0<\alpha <n$
.
$0<\alpha <n$
.
The paper is organised as follows. In Section 2, we give a proof of the Kolmogorov-type inequality and summarise some elementary properties. In Section 3, we prove the theorems. In Section 4, as an appendix, we gather some further results.
Throughout the paper, we use the following notation. If X and Y are normed spaces with
![]() $\|\cdot \|_{X} \lesssim \|\cdot \|_{Y}$
, then we write
$\|\cdot \|_{X} \lesssim \|\cdot \|_{Y}$
, then we write
![]() $X\hookleftarrow Y$
or
$X\hookleftarrow Y$
or
![]() $Y\hookrightarrow X$
(sometimes called the embedding). If
$Y\hookrightarrow X$
(sometimes called the embedding). If
![]() $X\hookleftarrow Y$
and
$X\hookleftarrow Y$
and
![]() $X\hookrightarrow Y$
, then we write
$X\hookrightarrow Y$
, then we write
![]() $X=Y$
.
$X=Y$
.
For quantities A and B, if
![]() $A\le C B$
, then we write
$A\le C B$
, then we write
![]() $A\lesssim B$
or
$A\lesssim B$
or
![]() $B\gtrsim A$
, and if
$B\gtrsim A$
, and if
![]() $A\lesssim B$
and
$A\lesssim B$
and
![]() $A\gtrsim B$
, then we write
$A\gtrsim B$
, then we write
![]() $A\sim B$
.
$A\sim B$
.
2. Preliminaries
We use the following fact about the Hausdorff content, due to Orobitg and Verdera [Reference Orobitg and Verdera6, Lemma 3].
Lemma 2.1. If
![]() $0<d\le n$
,
$0<d\le n$
,
![]() $0<p<{\infty }$
and
$0<p<{\infty }$
and
![]() $1\le \theta \le n/d$
, then
$1\le \theta \le n/d$
, then
Proof. By the substitution
![]() $t=s^{\theta }$
,
$t=s^{\theta }$
,
 $$ \begin{align*} \|f\|_{L^{\theta p}(H^{\theta d})}^{\theta p}=\int_0^{{\infty}}H^{\theta d}(\{|f|^{\theta p}>t\})\,{ d}t =\theta\int_0^{{\infty}}H^{\theta d}(\{|f|^p>s\})s^{\theta-1}\,{ d}s. \end{align*} $$
$$ \begin{align*} \|f\|_{L^{\theta p}(H^{\theta d})}^{\theta p}=\int_0^{{\infty}}H^{\theta d}(\{|f|^{\theta p}>t\})\,{ d}t =\theta\int_0^{{\infty}}H^{\theta d}(\{|f|^p>s\})s^{\theta-1}\,{ d}s. \end{align*} $$
Since
 $$ \begin{align} \bigg(\sum_j a_j^q\bigg)^{1/q} \le \bigg(\sum_j a_j^p\bigg)^{1/p} \quad \mbox{for } a_j\ge 0 \mbox{ and } 0<p<q<{\infty}, \end{align} $$
$$ \begin{align} \bigg(\sum_j a_j^q\bigg)^{1/q} \le \bigg(\sum_j a_j^p\bigg)^{1/p} \quad \mbox{for } a_j\ge 0 \mbox{ and } 0<p<q<{\infty}, \end{align} $$
we obtain
Thus,
 $$ \begin{align*} \|f\|_{L^{\theta p}(H^{\theta d})}^{\theta p} &\le \theta \int_0^{{\infty}} (H^d(\{|f|^p>s\}))^{\theta} s^{\theta-1} \,{ d}s \\ &= \theta \int_0^{{\infty}} (H^d(\{|f|^p>s\})s)^{\theta-1} H^d(\{|f|^p>s\}) \,{ d}s. \end{align*} $$
$$ \begin{align*} \|f\|_{L^{\theta p}(H^{\theta d})}^{\theta p} &\le \theta \int_0^{{\infty}} (H^d(\{|f|^p>s\}))^{\theta} s^{\theta-1} \,{ d}s \\ &= \theta \int_0^{{\infty}} (H^d(\{|f|^p>s\})s)^{\theta-1} H^d(\{|f|^p>s\}) \,{ d}s. \end{align*} $$
Since
 $$ \begin{align*} H^d(\{|f|^p>s\})s = \int_{\{|f|^p>s\}}s\,{ d}H^d \le \int_{\{|f|^p>s\}}|f|^p\,{ d}H^d \le \int_{{\mathbb R}^n}|f|^p\,{ d}H^d, \end{align*} $$
$$ \begin{align*} H^d(\{|f|^p>s\})s = \int_{\{|f|^p>s\}}s\,{ d}H^d \le \int_{\{|f|^p>s\}}|f|^p\,{ d}H^d \le \int_{{\mathbb R}^n}|f|^p\,{ d}H^d, \end{align*} $$
it follows that
We summarise some elementary properties of the weak Choquet spaces.
Proposition 2.2
-
(W1) (Chebyshev’s inequality) For
 $p>0$
,
$p>0$
,
 $L^p(H^d)\hookrightarrow \mathrm {w}\hskip -0.6pt{L}^p(H^d)$
.
$L^p(H^d)\hookrightarrow \mathrm {w}\hskip -0.6pt{L}^p(H^d)$
. -
(W2) If
 $0<d\le \theta d\le n$
and
$0<d\le \theta d\le n$
and
 $0<p<{\infty }$
, then
$0<p<{\infty }$
, then
 $\mathrm {w}\hskip -0.6pt{L}^p(H^d) \hookrightarrow \mathrm {w}\hskip -0.6pt{L}^{\theta p}(H^{\theta d})$
.
$\mathrm {w}\hskip -0.6pt{L}^p(H^d) \hookrightarrow \mathrm {w}\hskip -0.6pt{L}^{\theta p}(H^{\theta d})$
. -
(W3) If
 $d=n$
, then the weak Choquet spaces
$d=n$
, then the weak Choquet spaces
 $\mathrm {w}\hskip -0.6pt{L}^p(H^d)$
are the usual weak
$\mathrm {w}\hskip -0.6pt{L}^p(H^d)$
are the usual weak
 $L^p$
spaces
$L^p$
spaces
 $\mathrm {w}\hskip -0.6pt{L}^p({\mathbb R}^n)$
.
$\mathrm {w}\hskip -0.6pt{L}^p({\mathbb R}^n)$
.
Proof. The assertions (W1) and (W3) can be shown immediately from the definition. To prove assertion (W2), we appeal to (2.2) and observe that
 $$ \begin{align*} \|f\|_{\mathrm{w}\hskip-0.6pt{L}^{\theta p}(H^{\theta d})} &= \sup_{t>0} t\,H^{\theta d}(\{ x\in{\mathbb R}^n:\,|f(x)|>t \})^{1/(\theta p)} \\ &\le \sup_{t>0} t\,H^d(\{ x\in{\mathbb R}^n:\,|f(x)|>t \})^{1/p} = \|f\|_{\mathrm{w}\hskip-0.6pt{L}^p(H^d)}.\\[-40pt] \end{align*} $$
$$ \begin{align*} \|f\|_{\mathrm{w}\hskip-0.6pt{L}^{\theta p}(H^{\theta d})} &= \sup_{t>0} t\,H^{\theta d}(\{ x\in{\mathbb R}^n:\,|f(x)|>t \})^{1/(\theta p)} \\ &\le \sup_{t>0} t\,H^d(\{ x\in{\mathbb R}^n:\,|f(x)|>t \})^{1/p} = \|f\|_{\mathrm{w}\hskip-0.6pt{L}^p(H^d)}.\\[-40pt] \end{align*} $$
We next prove the Kolmogorov-type inequality. For
![]() $0<p,r\le {\infty }$
, we define the Lorentz quasinorm
$0<p,r\le {\infty }$
, we define the Lorentz quasinorm
 $$ \begin{align*} \|f\|_{L^{p,r}(H^d)} = \bigg( \int_0^{{\infty}} (t^pH^d(\{|f|>t\}))^{ r/p} \,\frac{ dt}{t} \bigg)^{1/r}. \end{align*} $$
$$ \begin{align*} \|f\|_{L^{p,r}(H^d)} = \bigg( \int_0^{{\infty}} (t^pH^d(\{|f|>t\}))^{ r/p} \,\frac{ dt}{t} \bigg)^{1/r}. \end{align*} $$
The Lorentz space
![]() $L^{p,r}(H^d)$
is the set of all functions for which this quasinorm is finite.
$L^{p,r}(H^d)$
is the set of all functions for which this quasinorm is finite.
Proposition 2.3. Let
![]() $0<d\le n$
,
$0<d\le n$
,
![]() $0<q<p<{\infty }$
and E be any measurable set.
$0<q<p<{\infty }$
and E be any measurable set.
-
(a) If
 $q\le r\le p$
, then
$q\le r\le p$
, then  $$ \begin{align*} H^d(E)^{1/p-1/q} \bigg(\int_{E}|f|^q\,{ d}H^d\bigg)^{1/q} \lesssim q^{1/r} \|f\chi_{E}\|_{L^{p,q}(H^d)}. \end{align*} $$
$$ \begin{align*} H^d(E)^{1/p-1/q} \bigg(\int_{E}|f|^q\,{ d}H^d\bigg)^{1/q} \lesssim q^{1/r} \|f\chi_{E}\|_{L^{p,q}(H^d)}. \end{align*} $$
-
(b) If
 $p<r$
, then (2.3)
$p<r$
, then (2.3) $$ \begin{align} H^d(E)^{1/p-1/q} \bigg(\int_{E}|f|^q\,{ d}H^d\bigg)^{1/q} \lesssim \bigg(q\bigg(\frac{r-p}{r(p-q)}\bigg)^{{(r-p)}/{r}}\bigg)^{1/p} \|f\chi_{E}\|_{L^{p,r}(H^d)}. \end{align} $$
$$ \begin{align} H^d(E)^{1/p-1/q} \bigg(\int_{E}|f|^q\,{ d}H^d\bigg)^{1/q} \lesssim \bigg(q\bigg(\frac{r-p}{r(p-q)}\bigg)^{{(r-p)}/{r}}\bigg)^{1/p} \|f\chi_{E}\|_{L^{p,r}(H^d)}. \end{align} $$
Proof. (a) Let E be an arbitrary measurable set satisfying
![]() $0<H^d(E)<{\infty }$
. For any
$0<H^d(E)<{\infty }$
. For any
![]() $A>0$
,
$A>0$
,
 $$ \begin{align*} &\int_{E}|f|^q\,{ d}H^d = \int_0^{{\infty}} H^d(E\cap\{|f|^q>t\})\,{ d}t = q\int_0^{{\infty}} t^{q-1} H^d(E\cap\{|f|>t\})\,{ d}t\\ &\quad \le q\int_0^{A} t^{q-1}H^d(E)\,{ d}t + q\int_{A}^{{\infty}} t^{q-r} t^rH^d(E\cap\{|f|>t\})^{ r/p} H^d(E\cap\{|f|>t\})^{1- r/p} \,\frac{{ d}t}{t}\\ &\quad \le H^d(E)^{1- r/p} ( A^qH^d(E)^{ rp} + qA^{q-r} \|f\chi_{E}\|_{L^{p,r}(H^d)}^r). \end{align*} $$
$$ \begin{align*} &\int_{E}|f|^q\,{ d}H^d = \int_0^{{\infty}} H^d(E\cap\{|f|^q>t\})\,{ d}t = q\int_0^{{\infty}} t^{q-1} H^d(E\cap\{|f|>t\})\,{ d}t\\ &\quad \le q\int_0^{A} t^{q-1}H^d(E)\,{ d}t + q\int_{A}^{{\infty}} t^{q-r} t^rH^d(E\cap\{|f|>t\})^{ r/p} H^d(E\cap\{|f|>t\})^{1- r/p} \,\frac{{ d}t}{t}\\ &\quad \le H^d(E)^{1- r/p} ( A^qH^d(E)^{ rp} + qA^{q-r} \|f\chi_{E}\|_{L^{p,r}(H^d)}^r). \end{align*} $$
If we take
![]() $A^r = q\|f\chi _{E}\|_{L^{p,r}(H^d)}^r H^d(E)^{-r/p}$
, then the two terms on the right-hand side of the above inequality balance. Thus,
$A^r = q\|f\chi _{E}\|_{L^{p,r}(H^d)}^r H^d(E)^{-r/p}$
, then the two terms on the right-hand side of the above inequality balance. Thus,
 $$ \begin{align*} \int_{E}|f|^q\,{ d}H^d & \le 2 H^d(E)^{1- r/p} (q\|f\chi_{E}\|_{L^{p,r}(H^d)}^r)^{ q/r} H^d(E)^{ r/p- q/p} \\ & \le 2q^{ q/r} H^d(E)^{1- q/p} \|f\chi_{E}\|_{L^{p,r}(H^d)}^q, \end{align*} $$
$$ \begin{align*} \int_{E}|f|^q\,{ d}H^d & \le 2 H^d(E)^{1- r/p} (q\|f\chi_{E}\|_{L^{p,r}(H^d)}^r)^{ q/r} H^d(E)^{ r/p- q/p} \\ & \le 2q^{ q/r} H^d(E)^{1- q/p} \|f\chi_{E}\|_{L^{p,r}(H^d)}^q, \end{align*} $$
and this implies
 $$ \begin{align*} H^d(E)^{1/p-1/q} \bigg(\int_{E}|f|^q\,{ d}H^d\bigg)^{1/q} \lesssim q^{1/r} \|f\chi_{E}\|_{L^{p,q}(H^d)}. \end{align*} $$
$$ \begin{align*} H^d(E)^{1/p-1/q} \bigg(\int_{E}|f|^q\,{ d}H^d\bigg)^{1/q} \lesssim q^{1/r} \|f\chi_{E}\|_{L^{p,q}(H^d)}. \end{align*} $$
(b) For any
![]() $A>0$
,
$A>0$
,
 $$ \begin{align*} \int_{A}^{{\infty}} t^{q-1} & H^d(E\cap\{|f|>t\})\,{ d}t \\ &\le \int_{A}^{{\infty}} t^qH^d(\{|f|>t\}) \,\frac{{ d}t}{t} = \int_{A}^{{\infty}} t^{q-p} \cdot t^pH^d(\{|f|>t\}) \,\frac{{ d}t}{t} \\ &\le \bigg( \int_{A}^{{\infty}} t^{(q-p){r}/{(r-p)}} \,\frac{{ d}t}{t} \bigg)^{{(r-p)}/{r}} \bigg( \int_{A}^{{\infty}} (t^pH^d(\{|f|>t\}))^{ r/p} \,\frac{{ d}t}{t} \bigg)^{ p/r} \\ &\le \bigg(\frac{r-p}{r(p-q)}\bigg)^{{(r-p)}/{r}} A^{q-p} \|f\chi_{E}\|_{L^{p,r}(H^d)}^p. \end{align*} $$
$$ \begin{align*} \int_{A}^{{\infty}} t^{q-1} & H^d(E\cap\{|f|>t\})\,{ d}t \\ &\le \int_{A}^{{\infty}} t^qH^d(\{|f|>t\}) \,\frac{{ d}t}{t} = \int_{A}^{{\infty}} t^{q-p} \cdot t^pH^d(\{|f|>t\}) \,\frac{{ d}t}{t} \\ &\le \bigg( \int_{A}^{{\infty}} t^{(q-p){r}/{(r-p)}} \,\frac{{ d}t}{t} \bigg)^{{(r-p)}/{r}} \bigg( \int_{A}^{{\infty}} (t^pH^d(\{|f|>t\}))^{ r/p} \,\frac{{ d}t}{t} \bigg)^{ p/r} \\ &\le \bigg(\frac{r-p}{r(p-q)}\bigg)^{{(r-p)}/{r}} A^{q-p} \|f\chi_{E}\|_{L^{p,r}(H^d)}^p. \end{align*} $$
This implies
 $$ \begin{align*} \int_{E}|f|^q\,{ d}H^d \lesssim A^qH^d(E) + q\bigg(\frac{r-p}{r(p-q)}\bigg)^{{(r-p)}/{r}} A^{q-p} \|f\chi_{E}\|_{L^{p,r}(H^d)}^p \end{align*} $$
$$ \begin{align*} \int_{E}|f|^q\,{ d}H^d \lesssim A^qH^d(E) + q\bigg(\frac{r-p}{r(p-q)}\bigg)^{{(r-p)}/{r}} A^{q-p} \|f\chi_{E}\|_{L^{p,r}(H^d)}^p \end{align*} $$
and, proceeding as before,
 $$ \begin{align*} \int_{E}|f|^q\,{ d}H^d \lesssim \bigg(q\bigg(\frac{r-p}{r(p-q)}\bigg)^{{(r-p)}/{r}}\bigg)^{ q/p} H^d(E)^{1- q/p} \|f\chi_{E}\|_{L^{p,r}(H^d)}^q, \end{align*} $$
$$ \begin{align*} \int_{E}|f|^q\,{ d}H^d \lesssim \bigg(q\bigg(\frac{r-p}{r(p-q)}\bigg)^{{(r-p)}/{r}}\bigg)^{ q/p} H^d(E)^{1- q/p} \|f\chi_{E}\|_{L^{p,r}(H^d)}^q, \end{align*} $$
which yields (2.3).
Remark 2.4. Taking
![]() $E=Q\in {\mathcal Q}({\mathbb R}^n)$
and
$E=Q\in {\mathcal Q}({\mathbb R}^n)$
and
![]() $r={\infty }$
in (2.3) yields
$r={\infty }$
in (2.3) yields
For an arbitrary r with
![]() $p\le r<{\infty }$
, multiplying by
$p\le r<{\infty }$
, multiplying by
![]() $\displaystyle \ell (Q)^{ d/r- d/p}$
on both sides gives
$\displaystyle \ell (Q)^{ d/r- d/p}$
on both sides gives
This means that, for
![]() $0<q<p\le r<{\infty }$
,
$0<q<p\le r<{\infty }$
,
The estimate (2.4) can be found in [Reference Gunawan, Hakim, Limanta and Masta4] and the book [Reference Sawano, Di Fazio and Hakim9]. It is interesting because one always has
In the case
![]() $r={\infty }$
in Proposition 2.3, we can show the reverse inequality.
$r={\infty }$
in Proposition 2.3, we can show the reverse inequality.
Proposition 2.5. Let
![]() $0<d\le n$
and
$0<d\le n$
and
![]() $0<q<p<{\infty }$
. Then
$0<q<p<{\infty }$
. Then
 $$ \begin{align*} \|f\|_{\mathrm{w}\hskip-0.6pt{L}^p(H^d)} \le \sup_{0<H^d(E)<{\infty}} H^d(E)^{1/p-1/q} \bigg( \int_{E}|f|^q\,{ d}H^d \bigg)^{ 1/q} \le \bigg(\frac{q}{p-q}\bigg)^{1/p} \|f\|_{\mathrm{w}\hskip-0.6pt{L}^p(H^d)}. \end{align*} $$
$$ \begin{align*} \|f\|_{\mathrm{w}\hskip-0.6pt{L}^p(H^d)} \le \sup_{0<H^d(E)<{\infty}} H^d(E)^{1/p-1/q} \bigg( \int_{E}|f|^q\,{ d}H^d \bigg)^{ 1/q} \le \bigg(\frac{q}{p-q}\bigg)^{1/p} \|f\|_{\mathrm{w}\hskip-0.6pt{L}^p(H^d)}. \end{align*} $$
Proof. The second inequality follows by taking
![]() $r={\infty }$
in (2.3). We prove the reverse inequality. For all
$r={\infty }$
in (2.3). We prove the reverse inequality. For all
![]() $t>0$
,
$t>0$
,
 $$ \begin{align*} tH^d(\{ & x\in{\mathbb R}^n: \,|f(x)|>t \})^{1/p} \\ &= H^d(\{ x\in{\mathbb R}^n:\,|f(x)|>t \})^{1/p-1/q} \cdot t\,H^d(\{ x\in{\mathbb R}^n:\,|f(x)|>t \})^{1/q} \\ &\le H^d(\{ x\in{\mathbb R}^n:\,|f(x)|>t \})^{1/p-1/q} \bigg( \int_{\{|f|>t\}}|f|^q\,{ d}H^d \bigg)^{1/q}. \end{align*} $$
$$ \begin{align*} tH^d(\{ & x\in{\mathbb R}^n: \,|f(x)|>t \})^{1/p} \\ &= H^d(\{ x\in{\mathbb R}^n:\,|f(x)|>t \})^{1/p-1/q} \cdot t\,H^d(\{ x\in{\mathbb R}^n:\,|f(x)|>t \})^{1/q} \\ &\le H^d(\{ x\in{\mathbb R}^n:\,|f(x)|>t \})^{1/p-1/q} \bigg( \int_{\{|f|>t\}}|f|^q\,{ d}H^d \bigg)^{1/q}. \end{align*} $$
Hence,
 $$ \begin{align*} tH^d(\{ x\in{\mathbb R}^n:\,\,|f(x)|>t \})^{1/p} \le \sup_{0<H^d(E)<{\infty}} H^d(E)^{1/p-1/q} \bigg( \int_{E}|f|^q\,{ d}H^d \bigg)^{1/q}, \end{align*} $$
$$ \begin{align*} tH^d(\{ x\in{\mathbb R}^n:\,\,|f(x)|>t \})^{1/p} \le \sup_{0<H^d(E)<{\infty}} H^d(E)^{1/p-1/q} \bigg( \int_{E}|f|^q\,{ d}H^d \bigg)^{1/q}, \end{align*} $$
as desired.
We summarise some elementary properties of the Choquet–Morrey spaces.
Proposition 2.6
-
(M1) If
 $0<p=q<{\infty }$
, then
$0<p=q<{\infty }$
, then
 ${\mathcal M}_p^p(H^d)=L^p(H^d)$
.
${\mathcal M}_p^p(H^d)=L^p(H^d)$
. -
(M2) If
 $0<q<p<{\infty }$
, then
$0<q<p<{\infty }$
, then
 $\mathrm {w}\hskip -0.6pt{L}^p(H^d) \hookrightarrow {\mathcal M}_q^p(H^d)$
.
$\mathrm {w}\hskip -0.6pt{L}^p(H^d) \hookrightarrow {\mathcal M}_q^p(H^d)$
. -
(M3) If
 $d=n$
, then the Choquet–Morrey spaces
$d=n$
, then the Choquet–Morrey spaces
 ${\mathcal M}^p_q(H^d)$
are the usual Morrey spaces
${\mathcal M}^p_q(H^d)$
are the usual Morrey spaces
 ${\mathcal M}^p_q({\mathbb R}^n)$
.
${\mathcal M}^p_q({\mathbb R}^n)$
. -
(M4) If
 $0<q\le r\le p<{\infty }$
, then
$0<q\le r\le p<{\infty }$
, then
 ${\mathcal M}^p_r(H^d) \hookrightarrow {\mathcal M}^p_q(H^d)$
.
${\mathcal M}^p_r(H^d) \hookrightarrow {\mathcal M}^p_q(H^d)$
. -
(M5) If
 $0<d\le \theta d\le n$
and
$0<d\le \theta d\le n$
and
 $0<q\le p<{\infty }$
, then
$0<q\le p<{\infty }$
, then
 ${\mathcal M}^p_q(H^d) \hookrightarrow {\mathcal M}^{\theta p}_{\theta q}(H^{\theta d})$
.
${\mathcal M}^p_q(H^d) \hookrightarrow {\mathcal M}^{\theta p}_{\theta q}(H^{\theta d})$
.
Proof. The assertions (M1)–(M4) are obvious. For assertion (M5), we use (2.1) and set
![]() $\tilde {d}=\theta d$
,
$\tilde {d}=\theta d$
,
![]() $\tilde {p}=\theta p$
and
$\tilde {p}=\theta p$
and
![]() $\tilde {q}=\theta q$
, to give
$\tilde {q}=\theta q$
, to give
It is well known, from Hedberg [Reference Hedberg5], that for
![]() $1\le p<{\infty }$
, the pointwise estimate
$1\le p<{\infty }$
, the pointwise estimate
holds. To prove the boundedness of the fractional integral operators on weak Choquet and Choquet–Morrey spaces with Hausdorff content, we extend Hedberg’s inequality.
Lemma 2.7. Let
![]() $0\le \beta <\alpha <n$
and
$0\le \beta <\alpha <n$
and
![]() $d/n\le p<q<{\infty }$
with
$d/n\le p<q<{\infty }$
with
 $$ \begin{align*} \frac{d-\beta p}{q} = \frac{d-\alpha p}{p}. \end{align*} $$
$$ \begin{align*} \frac{d-\beta p}{q} = \frac{d-\alpha p}{p}. \end{align*} $$
Then
 $$ \begin{align*} |I_{\alpha}f(x)| \lesssim \bigg(\frac dp-\alpha\bigg)^{ (p/q)-1} (\alpha-\beta)^{- p/q} \|f\|_{{\mathcal M}^p_{d/n}(H^d)}^{1- p/q} M_{\beta}f(x)^{ p/q} \quad \mbox{for } x\in{\mathbb R}^n. \end{align*} $$
$$ \begin{align*} |I_{\alpha}f(x)| \lesssim \bigg(\frac dp-\alpha\bigg)^{ (p/q)-1} (\alpha-\beta)^{- p/q} \|f\|_{{\mathcal M}^p_{d/n}(H^d)}^{1- p/q} M_{\beta}f(x)^{ p/q} \quad \mbox{for } x\in{\mathbb R}^n. \end{align*} $$
Proof. Fix
![]() $r>0$
. We decompose
$r>0$
. We decompose
 $$ \begin{align*} |I_{\alpha}f(x)| &\le \sum_{j=-{\infty}}^{{\infty}} \int_{2^jr \le |x-y| < 2^{j+1}r} \frac{|f(y)|}{|x-y|^{n-\alpha}} \,{ d}y \\ &\le \sum_{j=-{\infty}}^{{\infty}} \frac{1}{(2^jr)^{n-\alpha}} \int_{|x-y|<2^{j+1}r} |f(y)|\,{ d}y \\ &= \sum_{j=-{\infty}}^{-1} \frac{1}{(2^jr)^{n-\alpha}} \int_{|x-y|<2^{j+1}r} |f(y)|\,{ d}y + \sum_{j=0}^{{\infty}} \frac{1}{(2^jr)^{n-\alpha}} \int_{ |x-y|<2^{j+1}r} |f(y)|\,{ d}y \\ &=: J_1+J_2. \end{align*} $$
$$ \begin{align*} |I_{\alpha}f(x)| &\le \sum_{j=-{\infty}}^{{\infty}} \int_{2^jr \le |x-y| < 2^{j+1}r} \frac{|f(y)|}{|x-y|^{n-\alpha}} \,{ d}y \\ &\le \sum_{j=-{\infty}}^{{\infty}} \frac{1}{(2^jr)^{n-\alpha}} \int_{|x-y|<2^{j+1}r} |f(y)|\,{ d}y \\ &= \sum_{j=-{\infty}}^{-1} \frac{1}{(2^jr)^{n-\alpha}} \int_{|x-y|<2^{j+1}r} |f(y)|\,{ d}y + \sum_{j=0}^{{\infty}} \frac{1}{(2^jr)^{n-\alpha}} \int_{ |x-y|<2^{j+1}r} |f(y)|\,{ d}y \\ &=: J_1+J_2. \end{align*} $$
We estimate
 $$ \begin{align*} J_1 \lesssim \sum_{j=-{\infty}}^{-1} (2^jr)^{\alpha-\beta} M_{\beta}f(x) \lesssim \frac{r^{\alpha-\beta}}{\alpha-\beta} M_{\beta}f(x), \end{align*} $$
$$ \begin{align*} J_1 \lesssim \sum_{j=-{\infty}}^{-1} (2^jr)^{\alpha-\beta} M_{\beta}f(x) \lesssim \frac{r^{\alpha-\beta}}{\alpha-\beta} M_{\beta}f(x), \end{align*} $$
where we have used
![]() $2^{\alpha -\beta }-1 \gtrsim \alpha -\beta $
. By (2.1),
$2^{\alpha -\beta }-1 \gtrsim \alpha -\beta $
. By (2.1),
 $$ \begin{align*} J_2 \lesssim \sum_{j=1}^{{\infty}} (2^jr)^{\alpha- d/p} \|f\|_{{\mathcal M}^p_{d/n}(H^d)} \lesssim \frac{r^{\alpha- d/p}}{ (d/p)-\alpha} \|f\|_{{\mathcal M}^p_{d/n}(H^d)}, \end{align*} $$
$$ \begin{align*} J_2 \lesssim \sum_{j=1}^{{\infty}} (2^jr)^{\alpha- d/p} \|f\|_{{\mathcal M}^p_{d/n}(H^d)} \lesssim \frac{r^{\alpha- d/p}}{ (d/p)-\alpha} \|f\|_{{\mathcal M}^p_{d/n}(H^d)}, \end{align*} $$
where we have used
![]() $2^{ (d/p)-\alpha }-1 \gtrsim (d/p)-\alpha $
. Then, taking the optimal quantity
$2^{ (d/p)-\alpha }-1 \gtrsim (d/p)-\alpha $
. Then, taking the optimal quantity
![]() $r>0$
in the previous inequalities and noticing that
$r>0$
in the previous inequalities and noticing that
 $$ \begin{align*} \frac pq = \frac{d-\alpha p}{d-\beta p}, \end{align*} $$
$$ \begin{align*} \frac pq = \frac{d-\alpha p}{d-\beta p}, \end{align*} $$
we obtain
 $$ \begin{align*} |I_{\alpha}f(x)| \lesssim \bigg(\frac dp-\alpha\bigg)^{ p/q-1} (\alpha-\beta)^{- p/q} \|f\|_{{\mathcal M}^p_{d/n}(H^d)}^{1- p/q} M_{\beta}f(x)^{ p/q}, \end{align*} $$
$$ \begin{align*} |I_{\alpha}f(x)| \lesssim \bigg(\frac dp-\alpha\bigg)^{ p/q-1} (\alpha-\beta)^{- p/q} \|f\|_{{\mathcal M}^p_{d/n}(H^d)}^{1- p/q} M_{\beta}f(x)^{ p/q}, \end{align*} $$
as desired.
3. Proof of the theorems
Proof of Theorem 1.1(i)
Set
![]() $\delta =d-\alpha r$
. For
$\delta =d-\alpha r$
. For
![]() $t>0$
, we see that
$t>0$
, we see that
 $$ \begin{align*} \Omega(M_{\alpha}f;t) &:= \{x\in{\mathbb R}^n:\,M_{\alpha}f(x)>t\} \\ &\ = \{x\in{\mathbb R}^n:\, M_{\alpha}[f\chi_{\Omega(M_{\alpha}f;t)}](x)>t\}. \end{align*} $$
$$ \begin{align*} \Omega(M_{\alpha}f;t) &:= \{x\in{\mathbb R}^n:\,M_{\alpha}f(x)>t\} \\ &\ = \{x\in{\mathbb R}^n:\, M_{\alpha}[f\chi_{\Omega(M_{\alpha}f;t)}](x)>t\}. \end{align*} $$
Thus,
 $$ \begin{align*} tH^{\delta}(\Omega(M_{\alpha}f;t))^{1/q} &= H^{\delta}(\Omega(M_{\alpha}f;t))^{1/q-1/r} \cdot t\,H^{\delta}(\Omega(M_{\alpha}f;t))^{1/r} \\ &\lesssim H^{\delta}(\Omega(M_{\alpha}f;t))^{1/q-1/r} \cdot \|f\chi_{\Omega(M_{\alpha}f;t)}\|_{L^r(H^d)}, \end{align*} $$
$$ \begin{align*} tH^{\delta}(\Omega(M_{\alpha}f;t))^{1/q} &= H^{\delta}(\Omega(M_{\alpha}f;t))^{1/q-1/r} \cdot t\,H^{\delta}(\Omega(M_{\alpha}f;t))^{1/r} \\ &\lesssim H^{\delta}(\Omega(M_{\alpha}f;t))^{1/q-1/r} \cdot \|f\chi_{\Omega(M_{\alpha}f;t)}\|_{L^r(H^d)}, \end{align*} $$
where we have used (1.1), or (1.2) when
![]() $r=d/n$
. By the Kolmogorov-type inequality (2.3), we need only verify that, for a compact set
$r=d/n$
. By the Kolmogorov-type inequality (2.3), we need only verify that, for a compact set
![]() $E\subset {\mathbb R}^n$
,
$E\subset {\mathbb R}^n$
,
![]() $H^{\delta }(E)^{1/r-1/q} \ge H^d(E)^{1/r-1/p}$
. By (2.2) and since
$H^{\delta }(E)^{1/r-1/q} \ge H^d(E)^{1/r-1/p}$
. By (2.2) and since
![]() $\delta <d$
,
$\delta <d$
,
and
 $$ \begin{align*} \frac{\delta}{d}\bigg(\frac1r-\frac1q\bigg) = \frac1r-\frac\alpha d - \frac{d-\alpha p}{dp} = \frac1r-\frac1p. \end{align*} $$
$$ \begin{align*} \frac{\delta}{d}\bigg(\frac1r-\frac1q\bigg) = \frac1r-\frac\alpha d - \frac{d-\alpha p}{dp} = \frac1r-\frac1p. \end{align*} $$
This completes the proof.
Proof of Theorem 1.1(ii)
Thanks to the continuity, one can choose
![]() $\theta $
and
$\theta $
and
![]() $\beta $
so that
$\beta $
so that
![]() $1<\theta <n/d$
,
$1<\theta <n/d$
,
![]() $ r \alpha /p<\beta <\alpha $
and
$ r \alpha /p<\beta <\alpha $
and
Setting
![]() $\delta =\theta d$
and
$\delta =\theta d$
and
![]() $u=\theta p$
gives
$u=\theta p$
gives
![]() $\delta -\beta u=d-\alpha r$
. This equation and
$\delta -\beta u=d-\alpha r$
. This equation and
 $$ \begin{align*} \frac{d-\alpha r }{q} = \frac{d-\alpha p}{p} \end{align*} $$
$$ \begin{align*} \frac{d-\alpha r }{q} = \frac{d-\alpha p}{p} \end{align*} $$
immediately imply
 $$ \begin{align*} \frac{\delta-\beta u}{q} = \frac{d-\alpha r}{q} = \frac{d-\alpha p}{p} = \frac dp-\alpha = \frac\delta u-\alpha = \frac{\delta-\alpha u}{u}. \end{align*} $$
$$ \begin{align*} \frac{\delta-\beta u}{q} = \frac{d-\alpha r}{q} = \frac{d-\alpha p}{p} = \frac dp-\alpha = \frac\delta u-\alpha = \frac{\delta-\alpha u}{u}. \end{align*} $$
Since
![]() $\beta <\alpha $
, we see that
$\beta <\alpha $
, we see that
![]() $p<u<q$
. The equation
$p<u<q$
. The equation
and Lemma 2.7 yield
The equations
 $$ \begin{align*} \frac{d-\alpha r}u = \frac{\delta-\beta u}u = \frac{d-\alpha p}p \end{align*} $$
$$ \begin{align*} \frac{d-\alpha r}u = \frac{\delta-\beta u}u = \frac{d-\alpha p}p \end{align*} $$
and Theorem 1.1(i) yield
Since we always have
![]() $\|f\|_{{\mathcal M}^u_{\delta /n}(H^{\delta })} \lesssim \|f\|_{{\mathcal M}^p_{d/n}(H^d)} \lesssim \|f\|_{\mathrm {w}\hskip -0.6pt{L}^p(H^d)}$
, this completes the proof.
$\|f\|_{{\mathcal M}^u_{\delta /n}(H^{\delta })} \lesssim \|f\|_{{\mathcal M}^p_{d/n}(H^d)} \lesssim \|f\|_{\mathrm {w}\hskip -0.6pt{L}^p(H^d)}$
, this completes the proof.
Proof of Theorem 1.1(iii)
Setting
![]() $\beta = r \alpha /p$
, we have
$\beta = r \alpha /p$
, we have
 $$ \begin{align*} \frac{d-\beta p}{q} = \frac{d-\alpha r}{q} = \frac{d-\alpha p}{p}. \end{align*} $$
$$ \begin{align*} \frac{d-\beta p}{q} = \frac{d-\alpha r}{q} = \frac{d-\alpha p}{p}. \end{align*} $$
From Lemma 2.7,
By (1.1),
![]() $\|M_{\beta }f\|_{L^p(H^{d-\beta p})} \lesssim \|f\|_{L^p(H^d)}$
and, by the inclusion property,
$\|M_{\beta }f\|_{L^p(H^{d-\beta p})} \lesssim \|f\|_{L^p(H^d)}$
and, by the inclusion property,
![]() $\|f\|_{{\mathcal M}^p_{d/n}(H^d)} \lesssim \|f\|_{L^p(H^d)}$
. These estimates complete the proof.
$\|f\|_{{\mathcal M}^p_{d/n}(H^d)} \lesssim \|f\|_{L^p(H^d)}$
. These estimates complete the proof.
4. Appendix
In this section, we give some further consequences of our theorems and the embedding lemma (Lemma 2.1).
Proposition 4.1. Let
![]() $0<d,\delta <n$
and
$0<d,\delta <n$
and
![]() $0\le \alpha <n$
. If
$0\le \alpha <n$
. If
![]() $q>p$
,
$q>p$
,
![]() $d/n<p<d/\alpha $
and
$d/n<p<d/\alpha $
and
 $$ \begin{align*} \frac{\delta}{q}=\frac{d}{p}-\alpha, \end{align*} $$
$$ \begin{align*} \frac{\delta}{q}=\frac{d}{p}-\alpha, \end{align*} $$
then
Proof. Because
![]() $\delta>d-\alpha p$
, we can choose u, r and
$\delta>d-\alpha p$
, we can choose u, r and
![]() $\theta>1$
so that
$\theta>1$
so that
![]() $d/n<r<p$
and
$d/n<r<p$
and
Since
 $$ \begin{align*} \frac{d-\alpha r}{u} = \frac{d-\alpha p}{p}, \end{align*} $$
$$ \begin{align*} \frac{d-\alpha r}{u} = \frac{d-\alpha p}{p}, \end{align*} $$
Theorem 1.1(i) yields
![]() $\|M_{\alpha }f\|_{\mathrm {w}\hskip -0.6pt{L}^u(H^{d-\alpha r})} \lesssim \|f\|_{\mathrm {w}\hskip -0.6pt{L}^p(H^d)}$
, which gives, by the embedding in Proposition 2.2,
$\|M_{\alpha }f\|_{\mathrm {w}\hskip -0.6pt{L}^u(H^{d-\alpha r})} \lesssim \|f\|_{\mathrm {w}\hskip -0.6pt{L}^p(H^d)}$
, which gives, by the embedding in Proposition 2.2,
![]() $\|M_{\alpha }f\|_{\mathrm {w}\hskip -0.6pt{L}^q(H^{\delta })} \lesssim \|f\|_{\mathrm {w}\hskip -0.6pt{L}^p(H^d)}$
. This completes the proof.
$\|M_{\alpha }f\|_{\mathrm {w}\hskip -0.6pt{L}^q(H^{\delta })} \lesssim \|f\|_{\mathrm {w}\hskip -0.6pt{L}^p(H^d)}$
. This completes the proof.
Proposition 4.2. Let
![]() $0<d,\delta \le n$
and
$0<d,\delta \le n$
and
![]() $0\le \alpha <n$
. Suppose that
$0\le \alpha <n$
. Suppose that
![]() $d/n<r\le p<d/\alpha $
,
$d/n<r\le p<d/\alpha $
,
![]() $q\ge p$
,
$q\ge p$
,
![]() $\delta \ge d-\alpha r$
,
$\delta \ge d-\alpha r$
,
![]() $s\ge r$
and
$s\ge r$
and
 $$ \begin{align*} \frac{\delta}{q}=\frac{d}{p}-\alpha, \quad \frac{\delta}{s}=\frac{d}{r}-\alpha. \end{align*} $$
$$ \begin{align*} \frac{\delta}{q}=\frac{d}{p}-\alpha, \quad \frac{\delta}{s}=\frac{d}{r}-\alpha. \end{align*} $$
Then
Proof. We can choose u and
![]() $\theta \ge 1$
so that
$\theta \ge 1$
so that
![]() $q=\theta u$
and
$q=\theta u$
and
![]() $\delta =\theta (d-\alpha r)$
. Since
$\delta =\theta (d-\alpha r)$
. Since
 $$ \begin{align*} \frac{d-\alpha r}{u} = \frac{d-\alpha p}{p}, \end{align*} $$
$$ \begin{align*} \frac{d-\alpha r}{u} = \frac{d-\alpha p}{p}, \end{align*} $$
it follows from Theorem 1.3(i) that
![]() $\|M_{\alpha }f\|_{{\mathcal M}^u_r(H^{d-\alpha r})} \lesssim \|f\|_{{\mathcal M}^p_r(H^d)}$
. Since
$\|M_{\alpha }f\|_{{\mathcal M}^u_r(H^{d-\alpha r})} \lesssim \|f\|_{{\mathcal M}^p_r(H^d)}$
. Since
we must have
![]() $s=\theta r$
. Thus, by the inclusion property (Proposition 2.6),
$s=\theta r$
. Thus, by the inclusion property (Proposition 2.6),
as desired.
The case
![]() $d=\delta =n$
of Proposition 4.2 was first studied in unpublished work of Spanne (for the fractional integral operator) and Peetre published in [Reference Peetre7, Theorem 5.4]. After that, the condition used by Spanne was generalised to
$d=\delta =n$
of Proposition 4.2 was first studied in unpublished work of Spanne (for the fractional integral operator) and Peetre published in [Reference Peetre7, Theorem 5.4]. After that, the condition used by Spanne was generalised to
 $$ \begin{align*} \frac1q=\frac1p-\frac \alpha n, \quad \frac{s}{q}=\frac{r}{p} \end{align*} $$
$$ \begin{align*} \frac1q=\frac1p-\frac \alpha n, \quad \frac{s}{q}=\frac{r}{p} \end{align*} $$
by Adams [Reference Adams1], and Chiarenza and Frasca [Reference Chiarenza and Frasca3].
Proposition 4.3. Let
![]() $0<d\le n$
,
$0<d\le n$
,
![]() $0<\alpha <n$
,
$0<\alpha <n$
,
![]() $d/n<r\le p<{\infty }$
and
$d/n<r\le p<{\infty }$
and
![]() $0<s\le q<{\infty }$
. If
$0<s\le q<{\infty }$
. If
 $$ \begin{align*} \frac1q=\frac1p-\frac\alpha d, \quad \frac sq=\frac rp, \end{align*} $$
$$ \begin{align*} \frac1q=\frac1p-\frac\alpha d, \quad \frac sq=\frac rp, \end{align*} $$
then
Proof. Taking
![]() $\beta =0$
in Lemma 2.7 gives
$\beta =0$
in Lemma 2.7 gives
![]() $|I_{\alpha }f(x)| \lesssim \|f\|_{{\mathcal M}^p_{d/n}(H^d)}^{1- p/q} Mf(x)^{ p/q}$
. By the boundedness of M on the Morrey spaces,
$|I_{\alpha }f(x)| \lesssim \|f\|_{{\mathcal M}^p_{d/n}(H^d)}^{1- p/q} Mf(x)^{ p/q}$
. By the boundedness of M on the Morrey spaces,
 $$ \begin{align*} \|I_{\alpha}f\|_{{\mathcal M}^q_s(H^d)} &\lesssim \|f\|_{{\mathcal M}^p_{d/n}(H^d)}^{1- p/q} \|(Mf)^{p/q}\|_{{\mathcal M}^q_s(H^d)} \\ &= \|f\|_{{\mathcal M}^p_{d/n}(H^d)}^{1- p/q} \|Mf\|_{{\mathcal M}^p_r(H^d)}^{p/q} \\ &\lesssim \|f\|_{{\mathcal M}^p_{d/n}(H^d)}^{1- p/q} \|f\|_{{\mathcal M}^p_r(H^d)}^{ p/q} \lesssim \|f\|_{{\mathcal M}^p_r(H^d)}, \end{align*} $$
$$ \begin{align*} \|I_{\alpha}f\|_{{\mathcal M}^q_s(H^d)} &\lesssim \|f\|_{{\mathcal M}^p_{d/n}(H^d)}^{1- p/q} \|(Mf)^{p/q}\|_{{\mathcal M}^q_s(H^d)} \\ &= \|f\|_{{\mathcal M}^p_{d/n}(H^d)}^{1- p/q} \|Mf\|_{{\mathcal M}^p_r(H^d)}^{p/q} \\ &\lesssim \|f\|_{{\mathcal M}^p_{d/n}(H^d)}^{1- p/q} \|f\|_{{\mathcal M}^p_r(H^d)}^{ p/q} \lesssim \|f\|_{{\mathcal M}^p_r(H^d)}, \end{align*} $$
where, in the last inequality, we have used Proposition 2.6.