Completions of categories were studied by Lambek in [3], using the contravariant Horn functor to embed a small category C into the functor category (C*, S), where C* is the opposite category of C, and S is the category of sets. Three completions of C were considered; the completion (C*, S), the full subcategory (C*, C)inf⊆(C*, S) whose objects consist of all inf-preserving functors, and the full sub-category B⊆(C*, S)inf consisting of all subobjects of products of representable functors of the form HomC(—, C), C an object of C.

Since Cauchy's time the theory of analytic functions of a complex variable has depended on complex integration theory, and in particular on the fundamental integral theorem (1825) and integral formulas bearing his name. Cauchy defined an analytic function to be one which had a continuous first derivative in a region D, and showed that an analytic function had derivatives of all orders in D. It was not until 1900, with E. Goursat's famous proof of Cauchy's integral theorem, that the continuity of the first derivative could be inferred from its mere existence at all points of D.

The purpose of this note is to obtain an extension of the classical Sturm separation theorem for the second order, linear selfadjoint differential equation

1

to the case of a noncompact interval. The classical theorem (cf. [3, p. 209], [4, p. 224]) assumes that r and s are continuous with r positive on a compact interval I, and concludes that between each pair of zeros (on I) of one (nontrivial) solution of (1) there lies precisely one zero of any other linearly independent solution of (1). If I is not compact, a function y which is a solution of (1) on /may often be extended (continuously) to an endpoint, say a, of I.

Luxemburg and Zaanen [5] call an element φ of the topological dual of a normed or seminormed vector space V an integral if

We denote the space of integrals by VI, For the Lλ function spaces introduced by Ellis and Halperin [2] another Banach subspace of the dual emerges, namely the conjugate space Lλ* which is the Lλ space determined by the conjugate length function λ*-Lλ* is contained in (Lλ)I but need not coincide with it.

If S is an ordered set we write tp S to denote the order type of S and |5| for the cardinal of S. We also write [S]k for the set {X:X ⊂ S, |X|=k}. The partition symbol

(1)

Let (X, d1) and (Y, d2) be metric spaces. A mapping f:X→Y is said to be a Lipschitz mapping if there exists a real number λ such that

for each x,y∊X. We call λ a Lipschitz constant for f. If λ∊[0, 1), f is called a contraction mapping. Throughout this note CB(Y) denotes the set of closed and bounded subsets of Y equipped with the Hausdorff metric induced by d2.

A hypersurface of a globally framed f-manifold (briefly, a framed manifold), does not in general possess a framed structure as one may see by considering the 4-sphere S4 in R5 or S5. For, a hypersurface so endowed carries an almost complex structure, or else, it admits a nonsingular differentiable vector field. Since an almost complex manifold may be considered as being globally framed, with no complementary frames, this situation is in marked contrast with the well known fact that a hypersurface (real codimension 1) of an almost complex manifold admits a framed structure, more specifically, an almost contact structure.

It is shown that a 3rd-Engel group is an extension of a soluble group by a group of exponent 5.

King [5] devised two tests for determining when z = 1 is a singular point of the function f(z) defined by

1

having radius of convergence equal to one. The point z = 1 and radius of convergence one may be chosen without loss of generality.

We denote by ZG the integral group ring of the finite group G. We call ±g, for g in G, a trivial unit of ZG. For G abelian, Higman [4] (see also [3, p. 262 ff]) showed that every unit of finite order in ZG is trivial. For arbitrary finite G (indeed, for a torsion group G, not necessarily finite), Higman [4] showed that every unit in ZG is trivial if and only if G is

1. (i) abelian and the order of each element divides 4, or

2. (ii) abelian and the order of each element divides 6, or

3. (iii) the direct product of the quaternion group of order 8 and an abelian group of exponent 2.

Let C be a convex subset of a normed linear space E. The following properties of C were studied by Clark [2]:

1. (1) C is of finite width/ that is, C lies between two parallel closed hyperplanes;

2. (2) C is of finite width in some direction; that is, for some line L in E there is a finite upper bound for the lengths of C's intersections with lines parallel to L;

3. (3) C is partially bounded; that is, there is a finite upper bound for the radii of balls contained in C.

A group G is called a T3-group if it contains subgroups K and H, HΔK, with the property that if g and gb are members of G—K there is exactly one h∊H which satisfies the equation gh=gb. In these circumstances (G, K, H) is called a T3-triple.

It is shown in this paper that Rao's criterion of comparing two unbiased estimators on the basis of definiteness of the difference between their dispersion matrices is equivalent to Cramer's criterion based on their concentration ellipsoids. When the estimators have normal distributions it is shown that both the criteria have a desirable property in terms of the probabilities of the estimators lying in ellipsoids with the parameter point as the center.

Various generalizations of the Bernstein operator, defined on C[0, 1] by the relation

1.1

where

have been given. Note that bnk(x) is the well-known binomial distribution.

Let p and q be polynomial symbols of a type of algebras having operations ∨, ∧, and; (interpreted as the join, meet, and product of congruence relations). If 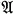is an algebra, L(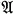), the local variety of 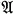, is the class of all algebras such that for each finite subset G of there is a finite subset F of 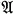such that every identity of F is also an identity of G.

THEOREM. There is an algorithm which, for each inequality

p≤q,

and pair of integers n, k≥2, determines a set Un, k of (Malcev) equations with the property:

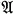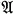For each algebra, p≤q is true in the congruence lattice offor each∊L() if and only if for each finite subset F of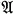and integer n≥2 there is a k=k(n, F) such that Un, kare identities of F.

This generalizes a corresponding result for varieties due to Wille (Kongruenzklassengeometrien, Lect. Notes in Math. Springer- Verlag, Berlin-Heidelberg, New York, 1970) and at the same time provides a more direct proof.

In [1] Higman and Neumann asked the questions whether the Frattini subgroup of a generalized free product can be larger than the amalgamated subgroup and whether such groups necessarily have maximal subgroups. In [4] Whittemore gave answers to the special cases of generalized free products of finitely many free groups with cyclic amalgamation and of generalized free products of finitely many finitely generated abelian groups. In this paper we shall study the Frattini subgroups of generalized free products of any groups with cyclic amalgamation.

It is shown that a topological space has a unique compatible quasi-uniformity if its topology is finite. Examples are given to show the converse is false for T1 and for normal second countable spaces. Two sufficient conditions are given for a topological space to have a compatible quasi-uniformity strictly finer than the associated Császár-Pervin quasi-uniformity. These conditions are used to show that a Hausdorff, semi-regular or first countable T1 space has a unique compatible quasi-uniformity if and only if its topology is finite. Császár and Pervin described, in quite different ways, quasi-uniformities which induce a given topology. It is shown that, for a given topological space, Császár and Pervin described the same quasi-uniformity.

Lehmer [3] and Reisel [7] have devised tests for determining the primality of integers of the form A2n—1. Tables of primes of these forms may be found in [7] and Williams and Zarnke [10]. Little work, however, seems to have been done on integers of the form N=2A3n—1. Lucas [6] gave conditions that were only sufficient for the primality of N. Recently Lehmer [4] has given a method for determining the primality of an integer N if the factorization of N+1 is known.

Let X be any completely regular Hausdorff topological space, and let βX denote its Stone-Čech compactification. This note is devoted to proving the following result:

5. THEOREM. Let X be realcompact and noncompact. Then βX—X is not connected im kleinen at any point.

Using a well-known theorem of Burnside on permutation groups of prime degree we offer new and simplified proofs of Theorems A, B, B' below for the case q=p a prime.