Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-12T22:00:21.420Z Has data issue: false hasContentIssue false
  • English
  • Français

Optimal Bicubic Finite Volume Methods on Quadrilateral Meshes

Published online by Cambridge University Press:  29 May 2015

Yanli Chen  and
Yonghai Li
Yanli Chen
Affiliation:
Institute of Mathematics, Jilin University, Changchun 130012, China
Yonghai Li*
Affiliation:
School of Mathematics, Jilin University, Changchun 130012, China
*
*Corresponding author. Email: chenyanli7532@sina.com (Y. L. Chen), yonghai@jlu.edu.cn (Y. H. Li)
Get access

Abstract

In this paper, an optimal bicubic finite volume method is established and analyzed for elliptic equations on quadrilateral meshes. Base on the so-called elementwise stiffness matrix analysis technique, we proceed the stability analysis. It is proved that the new scheme has optimal 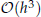convergence rate in H1 norm. Additionally, we apply this analysis technique to bilinear finite volume method. Finally, numerical examples are provided to confirm the theoretical analysis of bicubic finite volume method.

Keywords

65N3065N15Finite volume methodbicubic elementquadrilateral meshes
Type
Research Article
Information
Advances in Applied Mathematics and Mechanics , Volume 7 , Issue 4 , August 2015 , pp. 454 - 471
Copyright
Copyright © Global-Science Press 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1]Cai, Z., On the finite volume element method, Numer. Math., 58(7) (1991), pp. 713–735.Google Scholar
[2]Cai, Z., Mandel, J. and McCormick, S., The finite volume element method for diffusion equations on general triangulations, SIAM J. Numer. Anal., 28 (1991), pp. 392–402.CrossRefGoogle Scholar
[3]Huang, J. and Xi, S., On the finite volume element method for general self-adjoint elliptic problems, SIAM J. Numer. Anal., 35(5) (1998), pp. 1762–1774.Google Scholar
[4]Chou, S. H., Kwak, D. Y. and Li, Q., Lp error estimates and superconvergence for covolume or finite volume element methods, Numerical Methods Partial Differential Equations, 19(4) (2003), pp. 463–486.Google Scholar
[5]Wu, H. and Li, R., Eerror estimate for finite volume element methods for general second order elliptic problem, Numerical Methods for Partial Differential Equations, 19 (2003), pp. 693–708.CrossRefGoogle Scholar
[6]Chatzipantelidis, P., A finite volume method based on the Crouzeix-Raviart element for elliptic PDE’s in two dimensions, Numer. Math., 82 (1999), pp. 409–432.Google Scholar
[7]Süli, E., Convergence of finite volume schemes for Poissons equation on nonuniform meshes, SIAM J. Numer. Anal., 28 (1991), pp. 1419–1430.Google Scholar
[8]Chou, S. H. and Ye, X., Unified analysis of finite volume methods for second order elliptic problems, SIAM J. Numer. Anal., 45 (2007), pp. 1639–1653.Google Scholar
[9]Chou, S. H., Kwak, D. Y., and Kim, K. Y., A general framwork for constructing and analyzing mixed finite volume methods on quadrilateral grids: the overlapping covolume case, SIAM J. Numer. Anal., 39 (2001), pp. 1170–1196.Google Scholar
[10]Yang, M., A second-order finite volume element method on quadrilateral meshes for elliptic equations, M2An, 40 (2006), pp. 1053–1067.Google Scholar
[11]Wang, T. and Gu, Y., Superconvergent biquadratic finite volume element method for two-dimensional Poissons equations, J. Comput. Appl. Math., 234(2) (2010), pp. 447–460.Google Scholar
[12]Lv, J. and Li, Y., Optimal biquadratic finite volume element methods on quadrilateral meshes, SIAM J. Numer. Anal., 50(5) (2012), pp. 2379–2399.Google Scholar
[13]Chen, Z.Li, R., and Zhou, A., A note on the optimal L2-estimate of the finite volume element method, Adv. Comput. Math., 16 (2002), pp. 291–303.CrossRefGoogle Scholar
[14]Liebau, F., The Finite volume element method with quadratic basis functions, Computing, 57 (1996), pp. 281–299.Google Scholar
[15]Yang, M., Liu, J. and Chen, C., Error estimation of a quadratic finite volume method on right quadrangular prism grids, J. Comput. Appl. Math., 229 (2009), pp. 274–282.Google Scholar
[16]Cao, W., Zhang, Z. and Zou, Q., Superconvergence of any order finite volume schemes for 1D general elliptic equations, J. Sci. Comput., 56 (2013), pp. 1–25.Google Scholar
[17]Chen, Z., L2 estimates of linear element generalized difference schemes, Acta Sci. Natur. Univ. Sunyaseni, 4 (1994), pp. 22–28.Google Scholar
[18]Chen, Z., Superconvergence of generalized difference methods for elliptic boundary value problem, Numer. Math. J. Chinese Univ. (English Ser.), 3 (1994), pp. 163–171.Google Scholar
[19]Chen, Z., The error estimate of generalized difference methods of 3rd-order Hermite type for elliptic partial differential equations, Northeast. Math., J., 8 (1992), pp. 127–135.Google Scholar
[20]Li, R., Chen, Z. and Wu, W., Generalized Difference Methods for Differential Equations, Marcel Dekker, New York, 2000.Google Scholar
[21]Li, Y. and Li, R., Generalized difference methods on arbitrary quadrilateral networks, J. Comput. Math., 17(6) (1999), pp. 653–672.Google Scholar
[22]Wu, W. and Li, R., A generalized difference method for solving one-dimensional second-order elliptic and parabolic differential equations, Chinese Ann. Math. Ser. A, 5(3) (1984), pp. 303–312 (in Chinese).Google Scholar
[23]Zhu, P. and Li, R., Generalized difference methods for second order elliptic partial differential equations (II)-quadrilateral grids, Numer. Math. J. Chinese Univ., 4 (1982), pp. 360–375.Google Scholar
[24]Tian, M. and Chen, Z., Quadratic element generalized difference methods for elliptic equations, Numer. Math. J. Chinese Univ., 2 (1991), pp. 99–113.Google Scholar
[25]Bank, R. E. and Rose, D. J., Some error estimates for the box method, SIAM J. Numer. Anal., 24(4) (1987), pp. 777–787.Google Scholar
[26]Hackbusch, W., On first and second order box schemes, Computing, 41 (1989), pp. 277–296.CrossRefGoogle Scholar
[27]Schmidt, T., Box schemes on quadrilateral meshes, Compting, 51 (1993), pp. 271–292.Google Scholar
[28]Chou, S. H. and Li, Q., Error estimates in L2, H1 and L∞ in covolume methods for elliptic and parabolic problems: a unified approach, Math. Comput., 69(229) (2000), pp. 103–120.CrossRefGoogle Scholar
[29]Ewing, R. E., Liu, M., and Wang, J., Superconvergence of mixed finite element aproximations over quadrialterals, SIAM J. Numer. Anal., 36 (1999), pp. 772–787.Google Scholar
[30]Chen, Z., Wu, J. and Xu, Y., Higher-order finite volume methods for elliptic boundary value problems, Adv. Comput. Math., 37(2) (2012), pp. 191–253.Google Scholar
[31]Zhang, Z. and Zou, Q., A family of finite volume schemes of arbitrary order on rectangular meshes, J. Sci. Comput., 58 (2014), pp. 308–330.Google Scholar
[32]Shi, Z., A convergence condition for the quadrilateral wilson element, Numer. Math., 44 (1984), pp. 349–361.Google Scholar
[33]Ciarlet, P. G., The Finite Element Methods for Elliptic Problems, North-Holland, Amsterdam, 1978.Google Scholar
[34]Zhang, Z. and Zou, Q., Finite volume schemes of any order over quadrilateral meshes, Numer. Math., DOI 10.1007/s00211–014-0664–7, 2014.Google Scholar
[35]Xu, J. and Zou, Q., Analysis of linear and quadratic simplitical finite volume methods for elliptic equations, Numer. Math., 111 (2009), pp. 469–492.Google Scholar