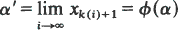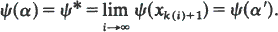Other
Conference on the Analysis of Spatial Data
-
- Published online by Cambridge University Press:
- 01 July 2016, p. 491
-
- Article
- Export citation
Seventh Conference on Stochastic Processes and their Applications: Twente University of Technology, Enschede, The Netherlands, 15–19 August 1977
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 255-259
-
- Article
- Export citation
Symposium on Mathematical Genetics
-
- Published online by Cambridge University Press:
- 01 July 2016, p. 1
-
- Article
- Export citation
Conference in Honour of Professor J. E. Moyal
-
- Published online by Cambridge University Press:
- 01 July 2016, p. 703
-
- Article
- Export citation
Research Article
A note on random walks at constant speed
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 704-707
-
- Article
- Export citation
I. Invited Review and Research Papers
Recent developments in reliability theory
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 261-262
-
- Article
- Export citation
Other
A numerical investigation of a model of Moran
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 1-3
-
- Article
- Export citation
Structural choices for spatial models
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 491-492
-
- Article
- Export citation
The effect of linkage disequilibrium on the genetic variance of a quantitative trait
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 4-6
-
- Article
- Export citation
I. Invited Review and Research Papers
Markov point processes
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 262-263
-
- Article
- Export citation
Research Article
Fluctuating interest rates
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 707-711
-
- Article
- Export citation
Other
Comparing surfaces
-
- Published online by Cambridge University Press:
- 01 July 2016, p. 492
-
- Article
- Export citation
I. Invited Review and Research Papers
A stochastic model of economic equilibrium
-
- Published online by Cambridge University Press:
- 01 July 2016, p. 263
-
- Article
- Export citation
Other
The generalised war of attrition
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 6-7
-
- Article
- Export citation
Research Article
The envelope of an oscillatory process and its upcrossings
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 711-716
-
- Article
- Export citation
Other
Short distances and flat triangles
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 492-493
-
- Article
- Export citation
I. Invited Review and Research Papers
Some very applied problems in applied probability
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 263-265
-
- Article
- Export citation
Other
Small distances, and Monte Carlo testing of spatial pattern
-
- Published online by Cambridge University Press:
- 01 July 2016, p. 493
-
- Article
- Export citation
Research Article
A relaxation view of a genetic problem
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 716-720
-
- Article
- Export citation
Other
A model to detect maternal and epistatic effects
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 7-10
-
- Article
- Export citation

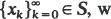 where
where  say). Let Φ (mapping
say). Let Φ (mapping