Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-26T19:45:02.786Z Has data issue: false hasContentIssue false
  • English
  • Français

SUMS OF FOUR SQUARES WITH A CERTAIN RESTRICTION

Part of: Forms and linear algebraic groups

Published online by Cambridge University Press:  14 January 2021

YUE-FENG SHE Open the ORCID record for YUE-FENG SHE [Opens in a new window]  and
HAI-LIANG WU Open the ORCID record for HAI-LIANG WU [Opens in a new window]
YUE-FENG SHE
Affiliation:
Department of Mathematics, Nanjing University, Nanjing210093, People’s Republic of China e-mail: she.math@smail.nju.edu.cn
HAI-LIANG WU*
Affiliation:
School of Science, Nanjing University of Posts and Telecommunications, Nanjing210023, People’s Republic of China
*
e-mail: whl.math@smail.nju.edu.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

Z.-W. Sun [‘Refining Lagrange’s four-square theorem’, J. Number Theory175 (2017), 169–190] conjectured that every positive integer n can be written as $ x^2+y^2+z^2+w^2\ (x,y,z,w\in \mathbb {N}=\{0,1,\ldots \})$ with 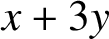$x+3y$ a square and also as 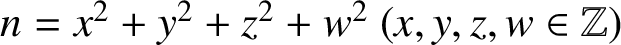$n=x^2+y^2+z^2+w^2\ (x,y,z,w \in \mathbb {Z})$ with 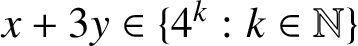$x+3y\in \{4^k:k\in \mathbb {N}\}$ . In this paper, we confirm these conjectures via the arithmetic theory of ternary quadratic forms.

Keywords

sums of four squaresternary quadratic forms

MSC classification

Primary: 11E25: Sums of squares and representations by other particular quadratic forms
Secondary: 11E12: Quadratic forms over global rings and fields 11E20: General ternary and quaternary quadratic forms; forms of more than two variables
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 104 , Issue 2 , October 2021 , pp. 218 - 227
Check for updates
Copyright
© 2021 Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

This research was supported by the National Natural Science Foundation of China (grant no. 11971222). The second author was supported by NUPTSF (grant no. NY220159).

References

Cassels, J. W. S., Rational Quadratic Forms (Academic Press, London, 1978).Google Scholar
Dickson, L. E., ‘Quaternary quadratic forms representing all integers’, Amer. J. Math. 49 (1927), 39–56.CrossRefGoogle Scholar
Dickson, L. E., Modern Elementary Theory of Numbers (University of Chicago Press, Chicago, IL, 1939).Google Scholar
Grosswald, E., Representation of Integers as Sums of Squares (Springer, New York, 1985).CrossRefGoogle Scholar
Jones, B. W., The Arithmetic Theory of Quadratic Forms, The Carus Mathematical Monographs, 10 (Mathematical Association of America, Buffalo, NY, 1950).CrossRefGoogle Scholar
Krachun, D. and Sun, Z.-W., ‘On sums of four pentagonal numbers with coefficients’, Electron. Res. Arch. 28 (2020), 559–566.CrossRefGoogle Scholar
O’Meara, O. T., Introduction to Quadratic Forms (Springer, New York, 1963).CrossRefGoogle Scholar
Ramanujan, S., ‘On the expression of a number in the form $a{x}^2+b{y}^2+c{z}^2+d{w}^2$ ’, Proc. Cambridge Philos. Soc. 19 (1917), 11–21.Google Scholar
Sun, Y.-C. and Sun, Z.-W., ‘Some variants of Lagrange’s four squares theorem’, Acta Arith. 183 (2018), 339–356.CrossRefGoogle Scholar
Sun, Z.-W., ‘On universal sums of polygonal numbers’, Sci. China Math. 58 (2015), 1367–1396.CrossRefGoogle Scholar
Sun, Z.-W., ‘Refining Lagrange’s four-square theorem’, J. Number Theory 175 (2017), 169–190.CrossRefGoogle Scholar
Sun, Z.-W., ‘Restricted sums of four squares’, Int. J. Number Theory 15 (2019), 1863–1893.CrossRefGoogle Scholar
Sun, Z.-W., ‘Sequence A300666’ (10 March 2018), in: The Online Encyclopedia of Integer Sequences, http://oeis.org Google Scholar
Wu, H.-L. and Sun, Z.-W., ‘On the 1-3-5 conjecture and related topics’, Acta Arith. 193 (2020), 253–268.CrossRefGoogle Scholar