Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-24T06:07:43.426Z Has data issue: false hasContentIssue false
  • English
  • Français

BORNOLOGIES AND LOCALLY LIPSCHITZ FUNCTIONS

Part of: Spaces with richer structures Real functions Topological linear spaces and related structures

Published online by Cambridge University Press:  15 May 2014

GERALD BEER  and
M. I. GARRIDO
GERALD BEER*
Affiliation:
Department of Mathematics, California State University Los Angeles, 5151 State University Drive, Los Angeles, CA 90032, USA email gbeer@cslanet.calstatela.edu
M. I. GARRIDO
Affiliation:
Instituto de Matemática Interdisciplinar (IMI), Departamento de Geometría y Topología, Universidad Complutense de Madrid, 28040 Madrid, Spain email maigarri@mat.ucm.es
*
✉gbeer@cslanet.calstatela.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

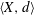Let $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\langle X,d \rangle $ be a metric space. We characterise the family of subsets of $X$ on which each locally Lipschitz function defined on 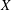$X$ is bounded, as well as the family of subsets on which each member of two different subfamilies consisting of uniformly locally Lipschitz functions is bounded. It suffices in each case to consider real-valued functions.

Keywords

Lipschitz functionlocally Lipschitz functionuniformly locally Lipschitz functionLipschitz in the small functionfunction preserving Cauchy sequencesbornologytotally bounded setBourbaki bounded set

MSC classification

Secondary: 26A16: Lipschitz (Hölder) classes 46A17: Bornologies and related structures; Mackey convergence, etc. 54E35: Metric spaces, metrizability
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 90 , Issue 2 , October 2014 , pp. 257 - 263
Copyright
Copyright © 2014 Australian Mathematical Publishing Association Inc. 

References

Atsuji, M., ‘Uniform continuity of continuous functions of metric spaces’, Pacific J. Math. 8 (1958), 11–16.CrossRefGoogle Scholar
Beer, G., Topologies on Closed and Closed Convex Sets (Kluwer Academic, Dordrecht, The Netherlands, 1993).Google Scholar
Beer, G., ‘Embeddings of bornological universes’, Set-Valued Anal. 16 (2008), 477–488.CrossRefGoogle Scholar
Beer, G. and Levi, S., ‘Total boundedness and bornologies’, Topology Appl. 156 (2009), 1271–1288.Google Scholar
Bourbaki, N., Elements of Mathematics. General Topology. Part 1 (Hermann, Paris, 1966).Google Scholar
Caserta, A., Di Maio, G. and Holá, L., ‘Arzelà’s theorem and strong uniform convergence on bornologies’, J. Math. Anal. Appl. 371 (2010), 384–392.Google Scholar
Engelking, R., General Topology (Polish Scientific Publishers, Warsaw, 1977).Google Scholar
Garrido, M. I. and Jaramillo, J., ‘Homomorphisms on function lattices’, Monatsh. Math. 141 (2004), 127–146.Google Scholar
Garrido, M. I. and Jaramillo, J., ‘Lipschitz-type functions on metric spaces’, J. Math. Anal. Appl. 340 (2008), 282–290.Google Scholar
Garrido, M. I. and Meroño, A. S., ‘New types of completeness in metric spaces’, Ann. Acad. Sci. Fenn. Math. to appear.Google Scholar
Goldberg, R., Methods of Real Analysis, 2nd edn (Wiley, New York, 1976).Google Scholar
Hejcman, J., ‘Boundedness in uniform spaces and topological groups’, Czech. Math. J. 9 (1959), 544–563.CrossRefGoogle Scholar
Hogbe-Nlend, H., Bornologies and Functional Analysis (North-Holland, Amsterdam, 1977).Google Scholar
Roberts, A. and Varberg, D., Convex Functions (Academic Press, New York, 1973).Google Scholar
Taylor, A. and Lay, D., Introduction to Functional Analysis, 2nd edn (Wiley, New York, 1980).Google Scholar
Vroegrijk, T., ‘Uniformizable and realcompact bornological universes’, Appl. Gen. Topol. 10 (2009), 277–287.CrossRefGoogle Scholar