Hostname: page-component-6bf8c574d5-9nwgx Total loading time: 0 Render date: 2025-02-21T00:12:02.574Z Has data issue: false hasContentIssue false
  • English
  • Français

ON THE NUMBER OF ALGEBRAIC POINTS ON THE GRAPH OF THE WEIERSTRASS SIGMA FUNCTIONS

Part of: Other special functions Diophantine approximation, transcendental number theory

Published online by Cambridge University Press:  13 January 2023

GOREKH PRASAD SENA Open the ORCID record for GOREKH PRASAD SENA [Opens in a new window]  and
K. SENTHIL KUMAR Open the ORCID record for K. SENTHIL KUMAR [Opens in a new window]
GOREKH PRASAD SENA*
Affiliation:
National Institute of Science Education and Research, Bhubaneswar, An OCC of Homi Bhabha National Institute, Khurda 752050, Odisha, India
K. SENTHIL KUMAR
Affiliation:
National Institute of Science Education and Research, Bhubaneswar, An OCC of Homi Bhabha National Institute, Khurda 752050, Odisha, India e-mail: senthil@niser.ac.in
*
e-mail: gorekh.sena@niser.ac.in
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 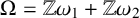$\Omega =\mathbb {Z}\omega _1+\mathbb {Z}\omega _2$ be a lattice in $\mathbb {C}$ with invariants $g_2,g_3$ and 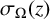$\sigma _{\Omega }(z)$ the associated Weierstrass 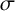$\sigma $-function. Let 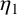$\eta _1$ and $\eta _2$ be the quasi-periods associated to 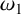$\omega _1$ and 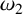$\omega _2$, respectively. Assuming 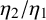$\eta _2/\eta _1$ is a nonzero real number, we give an upper bound for the number of algebraic points on the graph of 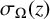$\sigma _{\Omega }(z)$ of bounded degrees and bounded absolute Weil heights in some unbounded region of $\mathbb {C}$ in the following three cases: (i) 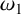$\omega _1$ and 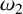$\omega _2$ algebraic; (ii) $g_2$ and 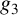$g_3$ algebraic; (iii) the algebraic points are far from the lattice points.

Keywords

Weierstrass σ-functionalgebraic pointsquasi-periodsWeil heightMahler measure

MSC classification

Primary: 11J89: Transcendence theory of elliptic and abelian functions
Secondary: 11J82: Measures of irrationality and of transcendence 33E05: Elliptic functions and integrals
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 108 , Issue 2 , October 2023 , pp. 205 - 216
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

This research is partially supported by the MATRICS grant MTR/2021/000476 and the second author is thankful to the SERB, India.

References

Besson, E., ‘Points algébriques de la fonction sigma de Weierstrass’, Preprint, 2015. Available online at https://rivoal.perso.math.cnrs.fr/sigma.pdf.Google Scholar
Boxall, G., Chalebgwa, T. and Jones, G., ‘On algebraic values of Weierstrass $\sigma$ -functions’, Atti Accad. Naz. Lincei Rend. Lincei Mat. Appl. 32(4) (2021), 819–833.10.4171/RLM/960CrossRefGoogle Scholar
David, S. and Hirata-Kohno, N., ‘Linear forms in elliptic logarithms’, J. reine angew. Math. 628 (2009), 37–89.Google Scholar
Heins, M., ‘On the pseudo-periods of the Weierstrass zeta functions. II’, Nagoya Math. J. 30 (1967), 113–119.10.1017/S002776300001240XCrossRefGoogle Scholar
Masser, D., Elliptic Functions and Transcendence, Lecture Notes in Mathematics, 437 (Springer, Berlin–Heidelberg, 1975).10.1007/BFb0069432CrossRefGoogle Scholar
Masser, D., ‘Rational values of the Riemann zeta function’, J. Number Theory 131(11) (2011), 2037–2046.10.1016/j.jnt.2011.03.013CrossRefGoogle Scholar
Masser, D., Auxiliary Polynomials in Number Theory, Cambridge Tracts in Mathematics, 207 (Cambridge University Press, Cambridge, 2016).10.1017/CBO9781107448018CrossRefGoogle Scholar