Online ordering is currently unavailable due to technical issues. We apologise for any delays responding to customers while we resolve this. For further updates please visit our website: https://www.cambridge.org/news-and-insights/technical-incident Due to planned maintenance there will be periods of time where the website may be unavailable. We apologise for any inconvenience.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Published online by Cambridge University Press: 03 July 2017
Let 
$G$ be a locally compact amenable group and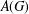 
$A(G)$ and 
$B(G)$ be the Fourier and the Fourier–Stieltjes algebras of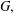 
$G,$ respectively. For a power bounded element 
$u$ of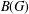 
$B(G)$, let 
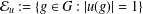${\mathcal{E}}_{u}:=\{g\in G:|u(g)|=1\}$. We prove some convergence theorems for iterates of multipliers in Fourier algebras.
(a) If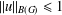 
$\Vert u\Vert _{B(G)}\leq 1$, then 
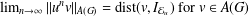$\lim _{n\rightarrow \infty }\Vert u^{n}v\Vert _{A(G)}=\text{dist}(v,I_{{\mathcal{E}}_{u}})\text{ for }v\in A(G)$, where 
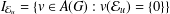$I_{{\mathcal{E}}_{u}}=\{v\in A(G):v({\mathcal{E}}_{u})=\{0\}\}$.
(b) The sequence 
$\{u^{n}v\}_{n\in \mathbb{N}}$ converges for every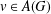 
$v\in A(G)$ if and only if 
${\mathcal{E}}_{u}$ is clopen and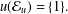 
$u({\mathcal{E}}_{u})=\{1\}.$
(c) If the sequence 
$\{u^{n}v\}_{n\in \mathbb{N}}$ converges weakly in 
$A(G)$ for some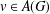 
$v\in A(G)$, then it converges strongly.
You have
Access
