Hostname: page-component-669899f699-2mbcq Total loading time: 0 Render date: 2025-04-27T12:11:31.233Z Has data issue: false hasContentIssue false
  • English
  • Français

Faltings’ main p-adic comparison theorems for non-smooth schemes

Part of: (Co)homology theory

Published online by Cambridge University Press:  12 January 2024

Tongmu He Open the ORCID record for Tongmu He [Opens in a new window]
Tongmu He*
Affiliation:
Institut des Hautes Études Scientifiques, 35 route de Chartres, 91440 Bures-sur-Yvette, France
*
e-mail: hetm15@ihes.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

To understand the p-adic étale cohomology of a proper smooth variety over a p-adic field, Faltings compared it to the cohomology of his ringed topos, by the so-called Faltings’ main p-adic comparison theorem, and then deduced various comparisons with p-adic cohomologies originating from differential forms. In this article, we generalize the former to any proper and finitely presented morphism of coherent schemes over an absolute integral closure of 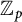$\mathbb {Z}_p$ (without any smoothness assumption) for torsion abelian étale sheaves (not necessarily finite locally constant). Our proof relies on our cohomological descent for Faltings’ ringed topos, using a variant of de Jong’s alteration theorem for morphisms of schemes due to Gabber–Illusie–Temkin to reduce to the relative case of proper log-smooth morphisms of log-smooth schemes over a complete discrete valuation ring proved by Abbes–Gros. A by-product of our cohomological descent is a new construction of Faltings’ comparison morphism, which does not use Achinger’s results on $K(\pi ,1)$-schemes.

Keywords

Cohomological descentFaltings toposv-topologyp-adic Hodge theorycomparison

MSC classification

Primary: 14F30: $p$-adic cohomology, crystalline cohomology
Type
Article
Information
Canadian Journal of Mathematics , Volume 77 , Issue 2 , April 2025 , pp. 426 - 458
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press on behalf of Canadian Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Abbes, A. and Gros, M., Les suites spectrales de Hodge–Tate. To appear in Astérisque, 2020. https://arxiv.org/abs/2003.04714.Google Scholar
Abbes, A. and Gros, M., Correspondance de Simpson p-adique II: fonctorialité par image directe propre et systèmes locaux de Hodge–Tate. Preprint, 2022. https://arxiv.org/abs/2210.10580.Google Scholar
Abbes, A., Gros, M., and Tsuji, T., The $p$ -adic Simpson correspondence, Annals of Mathematics Studies, 193, Princeton University Press, Princeton, NJ, 2016.Google Scholar
Achinger, P., Wild ramification and $K(\pi, 1)$ spaces . Invent. Math. 210(2017), no. 2, 453–499.CrossRefGoogle Scholar
Adiprasito, K., Liu, G., Pak, I., and Temkin, M., Log smoothness and polystability over valuation rings. Preprint, 2019. https://arxiv.org/abs/1806.09168v3.Google Scholar
Bourbaki, N., Algèbre commutative. Chapitres 5 à 7, Springer, Berlin, 2006. Edition originale publiée par Herman, Paris, 1975.CrossRefGoogle Scholar
de Jong, A. J., Smoothness, semi-stability and alterations . Inst. Hautes Études Sci. Publ. Math. 83 (1996), 51–93.CrossRefGoogle Scholar
Faltings, G., $p$ -adic Hodge theory . J. Amer. Math. Soc. 1(1988), no. 1, 255–299.Google Scholar
Faltings, G., Almost étale extensions . Astérisque 279(2002), 185–270.Google Scholar
Faltings, G., A $p$ -adic Simpson correspondence . Adv. Math. 198(2005), no. 2, 847–862.CrossRefGoogle Scholar
Gabber, O. and Ramero, L., Foundations for almost ring theory – release 7.5. Preprint, 2004. https://arxiv.org/abs/math/0409584v13.Google Scholar
Grothendieck, A., Éléments de géométrie algébrique. IV. Étude locale des schémas et des morphismes de schémas. II . Inst. Hautes Études Sci. Publ. Math. 24(1965), 231.Google Scholar
Grothendieck, A., Éléments de géométrie algébrique. IV. Étude locale des schémas et des morphismes de schémas. III . Inst. Hautes Études Sci. Publ. Math. 28(1966), 255.Google Scholar
Grothendieck, A., Éléments de géométrie algébrique. IV. Étude locale des schémas et des morphismes de schémas IV . Inst. Hautes Études Sci. Publ. Math. 32(1967), 361.Google Scholar
He, T., Cohomological descent for Faltings ringed topos . Preprint, 2023. https://sites.google.com/view/tongmu.CrossRefGoogle Scholar
Illusie, L., Laszlo, Y., and Orgogozo, F. (editors), Travaux de Gabber sur l’uniformisation locale et la cohomologie étale des schémas quasi-excellents, Société Mathématique de France, Paris, 2014. Séminaire à l’École Polytechnique 2006–2008. [Seminar of the Polytechnic School 2006–2008], With the collaboration of Frédéric Déglise, Alban Moreau, Vincent Pilloni, Michel Raynaud, Joël Riou, Benoît Stroh, Michael Temkin and Weizhe Zheng, Astérisque No. 363–364 (2014) (2014).Google Scholar
Kato, K., Logarithmic structures of Fontaine–Illusie . In: Algebraic analysis, geometry, and number theory (Baltimore, MD, 1988), Johns Hopkins University Press, Baltimore, MD, 1989, pp. 191–224.Google Scholar
Kato, K., Toric singularities . Amer. J. Math. 116(1994), no. 5, 1073–1099.CrossRefGoogle Scholar
Nizioł, W., Toric singularities: log-blow-ups and global resolutions . J. Algebraic Geom. 15(2006), no. 1, pp. 1–29.CrossRefGoogle Scholar
Ogus, A., Lectures on logarithmic algebraic geometry, Cambridge Studies in Advanced Mathematics, 178, Cambridge University Press, Cambridge, 2018.CrossRefGoogle Scholar
Scholze, P., $p$ -adic Hodge theory for rigid-analytic varieties . Forum Math. Pi 1(2013), e1.CrossRefGoogle Scholar
Scholze, P., Perfectoid spaces: A survey . In: Current developments in mathematics 2012, International Press, Somerville, MA, 2013, pp. 193–227.Google Scholar
Théorie des topos et cohomologie étale des schémas. Tome 1: Théorie des topos, Lecture Notes in Mathematics, 269, Springer, Berlin–New York, 1972. Séminaire de Géométrie Algébrique du Bois-Marie 1963–1964 (SGA 4), Dirigé par M. Artin, A. Grothendieck, et J. L. Verdier. Avec la collaboration de N. Bourbaki, P. Deligne et B. Saint-Donat.Google Scholar
Théorie des topos et cohomologie étale des schémas. Tome 2, Lecture Notes in Mathematics, 270, Springer, Berlin–New York, 1972. Séminaire de Géométrie Algébrique du Bois-Marie 1963–1964 (SGA 4), Dirigé par M. Artin, A. Grothendieck et J. L. Verdier. Avec la collaboration de N. Bourbaki, P. Deligne et B. Saint-Donat.Google Scholar
Théorie des topos et cohomologie étale des schémas. Tome 3, Lecture Notes in Mathematics, 305, Springer, Berlin–New York, 1973. Séminaire de Géométrie Algébrique du Bois-Marie 1963–1964 (SGA 4), Dirigé par M. Artin, A. Grothendieck et J. L. Verdier. Avec la collaboration de P. Deligne et B. Saint-Donat.Google Scholar
The Stacks Project Authors, The stacks project. 2023. https://stacks.math.columbia.edu.Google Scholar
Tsuji, T., Saturated morphisms of logarithmic schemes . Tunis. J. Math. 1(2019), no. 2, 185–220.CrossRefGoogle Scholar