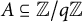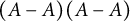1 Introduction
The landmark question about solvability of equations of the form
![]() $f(x_1,\dots ,x_n) = 0$
, where
$f(x_1,\dots ,x_n) = 0$
, where
![]() $f\in {\mathbb {Z}}[x_1,\dots ,x_n]$
and the variables
$f\in {\mathbb {Z}}[x_1,\dots ,x_n]$
and the variables
![]() $x_j \in X_j$
belong to some “large” but unspecified sets
$x_j \in X_j$
belong to some “large” but unspecified sets
![]() $X_j$
of the prime field
$X_j$
of the prime field
![]() $\mathbb {F}_q$
was firstly posed, probably, in [Reference Sárközy12]. Interesting in its own right, the problem has a clear connection with the sum–product phenomenon [Reference Tao and Vu21] due to the fact that as a rule the polynomial f includes both the addition and the multiplication. This theme becomes rather popular last years (see, e.g., [Reference Gyarmati and Sárközy7–Reference Pach10, Reference Sárközy12, Reference Shkredov16, Reference Shparlinski19] and many other papers).
$\mathbb {F}_q$
was firstly posed, probably, in [Reference Sárközy12]. Interesting in its own right, the problem has a clear connection with the sum–product phenomenon [Reference Tao and Vu21] due to the fact that as a rule the polynomial f includes both the addition and the multiplication. This theme becomes rather popular last years (see, e.g., [Reference Gyarmati and Sárközy7–Reference Pach10, Reference Sárközy12, Reference Shkredov16, Reference Shparlinski19] and many other papers).
The question about a partial resolution of some specific equations
![]() $f(x_1,\dots ,x_n)=0$
in large subrings of rings
$f(x_1,\dots ,x_n)=0$
in large subrings of rings
![]() ${\mathbb {Z}}_q := {\mathbb {Z}}/(q {\mathbb {Z}})$
for composite q was firstly considered by Fish in [Reference Fish5] (nevertheless, let us remark that a similar problem was formulated in [Reference Gyarmati and Sárközy8, Problem 5]). In particular, in [Reference Fish5, Corollary 1.2], Fish considered the polynomial
${\mathbb {Z}}_q := {\mathbb {Z}}/(q {\mathbb {Z}})$
for composite q was firstly considered by Fish in [Reference Fish5] (nevertheless, let us remark that a similar problem was formulated in [Reference Gyarmati and Sárközy8, Problem 5]). In particular, in [Reference Fish5, Corollary 1.2], Fish considered the polynomial
![]() $f(x_1,x_2,x_3,x_4) = (x_1-x_2)(x_3-x_4)$
and proved the following result.
$f(x_1,x_2,x_3,x_4) = (x_1-x_2)(x_3-x_4)$
and proved the following result.
Theorem 1.1 [Fish]
Let q be a positive integer, let
![]() $A, B\subset {\mathbb {Z}}_q$
be sets,
$A, B\subset {\mathbb {Z}}_q$
be sets,
![]() $|A| = \alpha q$
,
$|A| = \alpha q$
,
![]() $|B|=\beta q$
, and suppose that
$|B|=\beta q$
, and suppose that
![]() $\alpha \geqslant \beta $
. Then there is
$\alpha \geqslant \beta $
. Then there is
![]() $d|q$
with
$d|q$
with
where
![]() $C>0$
is an absolute constant and such that
$C>0$
is an absolute constant and such that
Here, we use the following standard notation [Reference Tao and Vu21], namely, given two sets
![]() $A,B\subset {\mathbb {Z}}_q$
, define the sumset of A and B as
$A,B\subset {\mathbb {Z}}_q$
, define the sumset of A and B as
In a similar way, we define the difference sets
![]() $A-A$
, the higher sumsets, e.g.,
$A-A$
, the higher sumsets, e.g.,
![]() $2A-A$
is
$2A-A$
is
![]() $A+A-A$
and, further, the products sets
$A+A-A$
and, further, the products sets
the higher product sets and so on. Finally, if
![]() $A \subseteq {\mathbb {Z}}_q$
and
$A \subseteq {\mathbb {Z}}_q$
and
![]() $\lambda \in {\mathbb {Z}}_q$
, then we write
$\lambda \in {\mathbb {Z}}_q$
, then we write
It is easy to see that in this generality one cannot have
![]() ${\mathbb {Z}}_q = (A-A)(B-B)$
in inclusion (1.2) for all sets
${\mathbb {Z}}_q = (A-A)(B-B)$
in inclusion (1.2) for all sets
![]() $A,B,$
and thus, we indeed need this additional (but small) divisor d. In contrary, for prime
$A,B,$
and thus, we indeed need this additional (but small) divisor d. In contrary, for prime
![]() $q,$
the divisor d can be omitted and the questions of this type were studied in [Reference Hart, Iosevich and Solymosi9] and [Reference Shparlinski19]. As we have seen the dependence in
$q,$
the divisor d can be omitted and the questions of this type were studied in [Reference Hart, Iosevich and Solymosi9] and [Reference Shparlinski19]. As we have seen the dependence in
![]() $F(\beta )$
was triple exponential on
$F(\beta )$
was triple exponential on
![]() $\beta ^{-1}$
. Using a series of other methods, we improve and generalize the last result in several directions. The signs
$\beta ^{-1}$
. Using a series of other methods, we improve and generalize the last result in several directions. The signs
![]() $\ll $
and
$\ll $
and
![]() $\gg $
below are the usual Vinogradov symbols.
$\gg $
below are the usual Vinogradov symbols.
Theorem 1.2 Let q be a positive integer, let
![]() $A, B\subset {\mathbb {Z}}_q$
be sets,
$A, B\subset {\mathbb {Z}}_q$
be sets,
![]() $|A| = \alpha q$
,
$|A| = \alpha q$
,
![]() $|B|=\beta q$
, and suppose that
$|B|=\beta q$
, and suppose that
![]() $\alpha \geqslant \beta $
. Then there is
$\alpha \geqslant \beta $
. Then there is
![]() $d|q$
with
$d|q$
with
where
![]() $C>0$
is an absolute constant and such that
$C>0$
is an absolute constant and such that
In [Reference Fish5], the author posed a series of questions in much more general form, as well as for other polynomials
![]() $f(x_1,\dots ,x_n)$
. Using different approaches, we partially resolve some of them (see Sections 3 and 4). In particular, we have deal with the equation
$f(x_1,\dots ,x_n)$
. Using different approaches, we partially resolve some of them (see Sections 3 and 4). In particular, we have deal with the equation
and
for rather general two-dimensional sets
![]() $\mathcal {A}, \mathcal {B} \subseteq {\mathbb {Z}}_q \times {\mathbb {Z}}_q$
and composite numbers q with some restrictions on its prime divisors (see Theorems 3.2, 3.5, and 4.1). As an example, we formulate a part of Theorem 3.2. Giving a positive integer
$\mathcal {A}, \mathcal {B} \subseteq {\mathbb {Z}}_q \times {\mathbb {Z}}_q$
and composite numbers q with some restrictions on its prime divisors (see Theorems 3.2, 3.5, and 4.1). As an example, we formulate a part of Theorem 3.2. Giving a positive integer
![]() $q,$
we denote by
$q,$
we denote by
![]() $\omega (q)$
the total number of prime divisors of q.
$\omega (q)$
the total number of prime divisors of q.
Theorem 1.3 Let q be a squarefree number, let
![]() $\mathcal {A}, \mathcal {B} \subseteq {\mathbb {Z}}_q^2$
be sets,
$\mathcal {A}, \mathcal {B} \subseteq {\mathbb {Z}}_q^2$
be sets,
![]() $|\mathcal {A}| = \alpha q^2$
,
$|\mathcal {A}| = \alpha q^2$
,
![]() $|\mathcal {B}|=\beta q^2$
, and suppose that
$|\mathcal {B}|=\beta q^2$
, and suppose that
![]() $\alpha \geqslant \beta $
. Then
$\alpha \geqslant \beta $
. Then
with
In particular, for
![]() $\mathcal {A} = \mathcal {B}$
, one has with the same d that
$\mathcal {A} = \mathcal {B}$
, one has with the same d that
Our another result may be interesting in itself (even in the case of prime q) due to it gives a new necessary condition for a set to be a difference set, but moreover, in addition, it yields another proof of Theorem 1.1 (see Theorems 5.4 and 5.9).
Theorem 1.4 Let q be a positive integer, and let
![]() $A \subseteq {\mathbb {Z}}_q$
be a set,
$A \subseteq {\mathbb {Z}}_q$
be a set,
![]() $|A| = \alpha q$
. Suppose that the least prime factor of q greater than
$|A| = \alpha q$
. Suppose that the least prime factor of q greater than
![]() $2 \alpha ^{-1}+3$
. Then, there is
$2 \alpha ^{-1}+3$
. Then, there is
![]() $X \subseteq {\mathbb {Z}}_q$
such that
$X \subseteq {\mathbb {Z}}_q$
such that
and
The equation
![]() $X(A-A) = {\mathbb {Z}}_q$
for a set
$X(A-A) = {\mathbb {Z}}_q$
for a set
![]() $X \subseteq {\mathbb {Z}}_q$
induces a coloring of
$X \subseteq {\mathbb {Z}}_q$
induces a coloring of
![]() ${\mathbb {Z}}_q$
via suitable subsets of our difference set
${\mathbb {Z}}_q$
via suitable subsets of our difference set
![]() $A-A$
. Hence, Theorem 1.4 gives us a new connection between coloring problems and difference sets. Finally, our result and the Ruzsa covering lemma [Reference Ruzsa11] (see inclusion (5.2)) show that for any set
$A-A$
. Hence, Theorem 1.4 gives us a new connection between coloring problems and difference sets. Finally, our result and the Ruzsa covering lemma [Reference Ruzsa11] (see inclusion (5.2)) show that for any set
![]() $A\subseteq {\mathbb {Z}}_q$
,
$A\subseteq {\mathbb {Z}}_q$
,
![]() $|A| \gg q$
, where q is a prime number, say, the set
$|A| \gg q$
, where q is a prime number, say, the set
![]() $A-A$
is a syndetic set (i.e., having bounded gaps between its consecutive elements, e.g., see [Reference Furstenberg6]) in both multiplicative and additive ways.
$A-A$
is a syndetic set (i.e., having bounded gaps between its consecutive elements, e.g., see [Reference Furstenberg6]) in both multiplicative and additive ways.
Let us say a few words about the notation. Having a positive integer q, we denote by
![]() $\omega (q)$
the total number of prime divisors of q and by
$\omega (q)$
the total number of prime divisors of q and by
![]() $\tau (q)$
the number of all divisors. Let
$\tau (q)$
the number of all divisors. Let
![]() $\varphi (q)$
be the Euler function. We use the same capital letter to denote a set
$\varphi (q)$
be the Euler function. We use the same capital letter to denote a set
![]() $A\subseteq {\mathbb {Z}}_q$
and its characteristic function
$A\subseteq {\mathbb {Z}}_q$
and its characteristic function
![]() $A: {\mathbb {Z}}_q \to \{0,1 \}$
. If
$A: {\mathbb {Z}}_q \to \{0,1 \}$
. If
![]() $\mathcal {R}$
is a ring, then we write
$\mathcal {R}$
is a ring, then we write
![]() $\mathcal {R}^*$
for the group of all inverse elements of
$\mathcal {R}^*$
for the group of all inverse elements of
![]() $\mathcal {R}$
. Let
$\mathcal {R}$
. Let
![]() $e_q(x) = e^{2\pi i x/q}$
, and let us denote by
$e_q(x) = e^{2\pi i x/q}$
, and let us denote by
![]() $[n]$
the set
$[n]$
the set
![]() $\{1,2,\dots , n\}$
. All logarithms are to base
$\{1,2,\dots , n\}$
. All logarithms are to base
![]() $2$
.
$2$
.
2 An effective version of Fish’s theorem
Having a positive integer n and a set
![]() $A \subseteq {\mathbb {Z}}_q \times \dots \times {\mathbb {Z}}_q = {\mathbb {Z}}^n_q$
(or just
$A \subseteq {\mathbb {Z}}_q \times \dots \times {\mathbb {Z}}_q = {\mathbb {Z}}^n_q$
(or just
![]() $A\subset {\mathbb {Z}}^n$
), as well as a divisor
$A\subset {\mathbb {Z}}^n$
), as well as a divisor
![]() $q_*|q$
, we write
$q_*|q$
, we write
We need a regularization result similar to [Reference Bourgain2, Lemma 2.1].
Lemma 2.1 Let
![]() $\delta ,\varepsilon \in (0,1)$
,
$\delta ,\varepsilon \in (0,1)$
,
![]() $M\geqslant 2$
be real numbers, let n be a positive integer, and let
$M\geqslant 2$
be real numbers, let n be a positive integer, and let
![]() $A\subset {\mathbb {Z}}^n_q$
be a set,
$A\subset {\mathbb {Z}}^n_q$
be a set,
![]() $|A|=\delta q^n$
. Then, there is
$|A|=\delta q^n$
. Then, there is
![]() $q_*|q$
, and a set
$q_*|q$
, and a set
![]() $A_* \subseteq A$
,
$A_* \subseteq A$
,
![]() $|\pi _{q/q_*} (A_*)| = 1$
such that
$|\pi _{q/q_*} (A_*)| = 1$
such that
![]() $q_* = \frac {q}{q_1 \dots q_s}$
,
$q_* = \frac {q}{q_1 \dots q_s}$
,
![]() $M\leqslant q_j \leqslant \delta ^{-\varepsilon ^{-1}}$
, s is the least number with
$M\leqslant q_j \leqslant \delta ^{-\varepsilon ^{-1}}$
, s is the least number with
![]() $\delta M^{\varepsilon s}> 1$
and for all
$\delta M^{\varepsilon s}> 1$
and for all
![]() $\tilde {q}|q_*$
,
$\tilde {q}|q_*$
,
![]() $\tilde {q} \geqslant M$
one has
$\tilde {q} \geqslant M$
one has
 $$ \begin{align} \max_{\xi \in {\mathbb{Z}}^n_{\tilde{q}}} |A_* \cap \pi^{-1}_{\tilde{q}} (\xi)| \leqslant \frac{|A_*|}{\tilde{q}^{1-\varepsilon}} . \end{align} $$
$$ \begin{align} \max_{\xi \in {\mathbb{Z}}^n_{\tilde{q}}} |A_* \cap \pi^{-1}_{\tilde{q}} (\xi)| \leqslant \frac{|A_*|}{\tilde{q}^{1-\varepsilon}} . \end{align} $$
Proof Suppose not. Then for a certain
![]() $\xi \in {\mathbb {Z}}^n_{q}$
and
$\xi \in {\mathbb {Z}}^n_{q}$
and
![]() $q_1|q$
,
$q_1|q$
,
![]() $q_1 \geqslant M$
, we find
$q_1 \geqslant M$
, we find
![]() ${A' := A \cap \pi ^{-1}_{q_1} (\xi )}$
with
${A' := A \cap \pi ^{-1}_{q_1} (\xi )}$
with
![]() $|A'|\geqslant \frac {|A|}{q^{1-\varepsilon }_1}$
. Clearly,
$|A'|\geqslant \frac {|A|}{q^{1-\varepsilon }_1}$
. Clearly,
![]() $|\pi _{q_1} (A')| = 1$
and the density of
$|\pi _{q_1} (A')| = 1$
and the density of
![]() $A'$
in the appropriate shift of
$A'$
in the appropriate shift of
![]() ${\mathbb {Z}}^n_{q/q_1}$
is at least
${\mathbb {Z}}^n_{q/q_1}$
is at least
![]() $\delta q^\varepsilon _1 \geqslant \delta M^{\varepsilon }$
. Hence, applying the same procedure to the set
$\delta q^\varepsilon _1 \geqslant \delta M^{\varepsilon }$
. Hence, applying the same procedure to the set
![]() $A'$
and to the new module
$A'$
and to the new module
![]() $q/q_1$
, we see that our algorithm must stop after at most s steps. Notice that condition (2.1) holds automatically if
$q/q_1$
, we see that our algorithm must stop after at most s steps. Notice that condition (2.1) holds automatically if
![]() $\tilde {q} \geqslant \delta ^{-\varepsilon ^{-1}}$
, and hence, at the final step of our procedure, we find a set
$\tilde {q} \geqslant \delta ^{-\varepsilon ^{-1}}$
, and hence, at the final step of our procedure, we find a set
![]() $A_* \subset A$
,
$A_* \subset A$
,
![]() $|\pi _{q/q_*} (A_*)| = 1$
, having all required properties. This completes the proof.
$|\pi _{q/q_*} (A_*)| = 1$
, having all required properties. This completes the proof.
Example 2.2 Let
![]() $n=1$
and
$n=1$
and
![]() $q=p_1\dots p_s$
, where
$q=p_1\dots p_s$
, where
![]() $p_j$
be some prime numbers. Given a set
$p_j$
be some prime numbers. Given a set
![]() $A\subset {\mathbb {Z}}_q$
, we are interested in distribution of A among arithmetic progressions of the form
$A\subset {\mathbb {Z}}_q$
, we are interested in distribution of A among arithmetic progressions of the form
![]() $\alpha \tilde {q}+\beta $
, where
$\alpha \tilde {q}+\beta $
, where
![]() $\tilde {q}\geqslant M$
is any divisor of q,
$\tilde {q}\geqslant M$
is any divisor of q,
![]() $\beta $
is a fixed number from the segment
$\beta $
is a fixed number from the segment
![]() $[\tilde {q}]$
and
$[\tilde {q}]$
and
![]() $\alpha $
runs over the segment
$\alpha $
runs over the segment
![]() $[q/\tilde {q}]$
. Of course, not all sets A are uniformly distributed among such progressions, e.g., take
$[q/\tilde {q}]$
. Of course, not all sets A are uniformly distributed among such progressions, e.g., take
![]() $A=A_0=\{0, \tilde {q}, 2 \tilde {q} \dots , L \tilde {q}\}$
,
$A=A_0=\{0, \tilde {q}, 2 \tilde {q} \dots , L \tilde {q}\}$
,
![]() ${L=q/\tilde {q} - 1}$
but nevertheless one can always find a subset
${L=q/\tilde {q} - 1}$
but nevertheless one can always find a subset
![]() $A_*$
of our set such that this new set
$A_*$
of our set such that this new set
![]() $A_*$
does not correlate with these arithmetic progressions in the sense of inequality (2.1). In our particular case, just take
$A_*$
does not correlate with these arithmetic progressions in the sense of inequality (2.1). In our particular case, just take
![]() $A_* = A_0$
and
$A_* = A_0$
and
![]() $q_* = q/\tilde {q}$
.
$q_* = q/\tilde {q}$
.
Now, we are ready to obtain the main result of this section, which implies Theorem 1.2 from the introduction. Our proof uses the Fourier analysis (its standard facts can be found in [Reference Tao and Vu21], say) and classical estimates for the Kloosterman sums. Having a group
![]() ${\mathbf G}$
, we define for any function
${\mathbf G}$
, we define for any function
![]() $f:{\mathbf G} \to \mathbb {C}$
and a representation
$f:{\mathbf G} \to \mathbb {C}$
and a representation
![]() $\rho \in \widehat {{\mathbf G}}$
the Fourier transform of f at
$\rho \in \widehat {{\mathbf G}}$
the Fourier transform of f at
![]() $\rho $
by the formula
$\rho $
by the formula
Theorem 2.3 Let q be a positive integer, let
![]() $A, B\subset {\mathbb {Z}}_q$
be sets,
$A, B\subset {\mathbb {Z}}_q$
be sets,
![]() $|A| = \alpha q$
,
$|A| = \alpha q$
,
![]() $|B|=\beta q$
, and suppose that
$|B|=\beta q$
, and suppose that
![]() $\alpha \geqslant \beta $
. Then, there is
$\alpha \geqslant \beta $
. Then, there is
![]() $d|q$
with
$d|q$
with
where
![]() $C>0$
is an absolute constant and such that
$C>0$
is an absolute constant and such that
In addition,
Proof Let
![]() $q=p_1^{\rho _1} \dots p_t^{\rho _t}$
, where
$q=p_1^{\rho _1} \dots p_t^{\rho _t}$
, where
![]() $p_j$
are different primes,
$p_j$
are different primes,
![]() $p_1<\dots <p_t$
. Also, let
$p_1<\dots <p_t$
. Also, let
![]() $M\geqslant 2$
,
$M\geqslant 2$
,
![]() $\varepsilon \in (0,1)$
be parameters, which we will choose later. First of all, we remove all divisors less than M from q. More precisely, for any
$\varepsilon \in (0,1)$
be parameters, which we will choose later. First of all, we remove all divisors less than M from q. More precisely, for any
![]() $p_j$
,
$p_j$
,
![]() $j\in [t]$
let
$j\in [t]$
let
![]() $\gamma _j \leqslant \rho _j$
be the maximal nonnegative integer such that
$\gamma _j \leqslant \rho _j$
be the maximal nonnegative integer such that
![]() $p^{\gamma _j}_j \leqslant M$
. Clearly,
$p^{\gamma _j}_j \leqslant M$
. Clearly,
![]() $\gamma _1 \geqslant \gamma _2 \geqslant \dots \gamma _t \geqslant 0$
and let
$\gamma _1 \geqslant \gamma _2 \geqslant \dots \gamma _t \geqslant 0$
and let
![]() $t_0 \leqslant t$
be the maximal j with
$t_0 \leqslant t$
be the maximal j with
![]() $\gamma _j \neq 0$
. Thus
$\gamma _j \neq 0$
. Thus
![]() $t_0 \leqslant \pi (M)$
. Now, we define
$t_0 \leqslant \pi (M)$
. Now, we define
 $$ \begin{align} Q_1 := \prod_{j=1}^{t_0} p_j^{\gamma_j} \leqslant M^{t_0} \leqslant \min\{ M^{\pi (M)}, M^{\omega(q)} \}, \end{align} $$
$$ \begin{align} Q_1 := \prod_{j=1}^{t_0} p_j^{\gamma_j} \leqslant M^{t_0} \leqslant \min\{ M^{\pi (M)}, M^{\omega(q)} \}, \end{align} $$
and take
![]() $A_1 \subseteq A$
such that a shift of
$A_1 \subseteq A$
such that a shift of
![]() $A_1$
belongs to
$A_1$
belongs to
![]() ${\mathbb {Z}}_{q/Q_1}$
and has density at least
${\mathbb {Z}}_{q/Q_1}$
and has density at least
![]() $\alpha $
. In particular,
$\alpha $
. In particular,
![]() $|\pi _{Q_1} (A_1)|=1$
and of course such a shift exists by the Dirichlet principle. Similarly, we can do the same with the set B so as not to lose the density. Secondly, we apply Lemma 2 with
$|\pi _{Q_1} (A_1)|=1$
and of course such a shift exists by the Dirichlet principle. Similarly, we can do the same with the set B so as not to lose the density. Secondly, we apply Lemma 2 with
![]() $n=1$
,
$n=1$
,
![]() $A=A_1$
to regularize the set
$A=A_1$
to regularize the set
![]() $A_1$
and find a set
$A_1$
and find a set
![]() $A_* \subseteq A_1$
and a module
$A_* \subseteq A_1$
and a module
![]() $q_*$
that satisfies (2.1) and all other restrictions. Again, using the Dirichlet principle, we take
$q_*$
that satisfies (2.1) and all other restrictions. Again, using the Dirichlet principle, we take
![]() $B_* \subseteq B$
such that the density of B does not decrease. Let
$B_* \subseteq B$
such that the density of B does not decrease. Let
![]() $\lambda \in {\mathbb {Z}}_{q_*}$
be an arbitrary number and we first suppose that
$\lambda \in {\mathbb {Z}}_{q_*}$
be an arbitrary number and we first suppose that
![]() $\lambda \in {\mathbb {Z}}^*_{q_*}$
. To prove
$\lambda \in {\mathbb {Z}}^*_{q_*}$
. To prove
![]() $\lambda \in (A-A)(B-B),$
it is enough to show that
$\lambda \in (A-A)(B-B),$
it is enough to show that
![]() $\lambda \in (A_*-A_*)(B_*-B_*)$
or, equivalently, in terms of the Fourier transform, it suffices obtain the inequality
$\lambda \in (A_*-A_*)(B_*-B_*)$
or, equivalently, in terms of the Fourier transform, it suffices obtain the inequality
 $$ \begin{align} \frac{|A_*|^2 |B_*|^2}{q_*}> \frac{1}{q_*} \sum_{r\neq 0} |\widehat{B}_* (r)|^2 \cdot \sum_{a_1,a_2\in A_*} e_{q_*} \left(\frac{\lambda r}{a_1-a_2} \right) := \sigma . \end{align} $$
$$ \begin{align} \frac{|A_*|^2 |B_*|^2}{q_*}> \frac{1}{q_*} \sum_{r\neq 0} |\widehat{B}_* (r)|^2 \cdot \sum_{a_1,a_2\in A_*} e_{q_*} \left(\frac{\lambda r}{a_1-a_2} \right) := \sigma . \end{align} $$
Now clearly,
 $$ \begin{align} \sigma \leqslant \frac{1}{q_*} \sum_{q_2|q_*,\, q_2>1}\, \sum_{z\in {\mathbb{Z}}^*_{q_2}} |\widehat{B}_* (zq_* q^{-1}_2)|^2 \left| \sum_{a_1,a_2\in A_*} e_{q_2} \left(\frac{\lambda z}{a_1-a_2} \right) \right| . \end{align} $$
$$ \begin{align} \sigma \leqslant \frac{1}{q_*} \sum_{q_2|q_*,\, q_2>1}\, \sum_{z\in {\mathbb{Z}}^*_{q_2}} |\widehat{B}_* (zq_* q^{-1}_2)|^2 \left| \sum_{a_1,a_2\in A_*} e_{q_2} \left(\frac{\lambda z}{a_1-a_2} \right) \right| . \end{align} $$
In terms of the Kloosterman sums
 $$\begin{align*}K_q (\lambda,r) := \sum_{x\in {\mathbb{Z}}^*_{q}} e_{q} \left( \frac{\lambda}{x} + rx\right) \end{align*}$$
$$\begin{align*}K_q (\lambda,r) := \sum_{x\in {\mathbb{Z}}^*_{q}} e_{q} \left( \frac{\lambda}{x} + rx\right) \end{align*}$$
and the density function
one has (recall that
![]() $\lambda \in {\mathbb {Z}}^*_{q_*}$
and
$\lambda \in {\mathbb {Z}}^*_{q_*}$
and
![]() $z \in {\mathbb {Z}}^*_{q_2}$
)
$z \in {\mathbb {Z}}^*_{q_2}$
)
 $$ \begin{align} &\sum_{a_1,a_2\in A_*} e_{q_2} \left(\frac{\lambda z}{a_1-a_2} \right) =\\&\quad \sum_{\xi_1,\xi_2 \in {\mathbb{Z}}_{q_2}} \eta_{q_2}(\xi_1) \eta_{q_2} (\xi_2) e_{q_2} \left(\frac{\lambda z}{\xi_1-\xi_2} \right) = q^{-1}_2 \sum_{\xi \in {\mathbb{Z}}_{q_2}} |\widehat{\eta}_{q_2} (\xi)|^2 K_{q_2} (\lambda z, \xi) \nonumber\end{align} $$
$$ \begin{align} &\sum_{a_1,a_2\in A_*} e_{q_2} \left(\frac{\lambda z}{a_1-a_2} \right) =\\&\quad \sum_{\xi_1,\xi_2 \in {\mathbb{Z}}_{q_2}} \eta_{q_2}(\xi_1) \eta_{q_2} (\xi_2) e_{q_2} \left(\frac{\lambda z}{\xi_1-\xi_2} \right) = q^{-1}_2 \sum_{\xi \in {\mathbb{Z}}_{q_2}} |\widehat{\eta}_{q_2} (\xi)|^2 K_{q_2} (\lambda z, \xi) \nonumber\end{align} $$
In the last line, we have applied the well-known bound for the Kloosterman sum and the Parseval identity. Now, to estimate
![]() $\| \eta _{q_2}\|_2^2$
, we use the regularity property of
$\| \eta _{q_2}\|_2^2$
, we use the regularity property of
![]() $A_*$
and derive
$A_*$
and derive
 $$ \begin{align} \| \eta_{q_2}\|_2^2 \leqslant \| \eta_{q_2}\|_\infty \| \eta_{q_2}\|_1 \leqslant \frac{|A_*|^2}{q^{1-\varepsilon}_2} . \end{align} $$
$$ \begin{align} \| \eta_{q_2}\|_2^2 \leqslant \| \eta_{q_2}\|_\infty \| \eta_{q_2}\|_1 \leqslant \frac{|A_*|^2}{q^{1-\varepsilon}_2} . \end{align} $$
Further, let us obtain a lower bound for divisors
![]() $q_2$
. Since
$q_2$
. Since
![]() $|\pi _{Q_1} (A_1)|=1$
, it follows that for all
$|\pi _{Q_1} (A_1)|=1$
, it follows that for all
![]() $q_1|Q_1$
, we have
$q_1|Q_1$
, we have
 $$\begin{align*}\frac{1}{q_1} \sum_{\xi \in {\mathbb{Z}}_{q_1}} |\widehat{\eta}_{q_1} (\xi)|^2 K_{q_1} (\lambda z, \xi) = \frac{|A_*|^2}{q_1} \sum_{\xi \in {\mathbb{Z}}_{q_1}} K_{q_1} (\lambda z, \xi) = 0 . \end{align*}$$
$$\begin{align*}\frac{1}{q_1} \sum_{\xi \in {\mathbb{Z}}_{q_1}} |\widehat{\eta}_{q_1} (\xi)|^2 K_{q_1} (\lambda z, \xi) = \frac{|A_*|^2}{q_1} \sum_{\xi \in {\mathbb{Z}}_{q_1}} K_{q_1} (\lambda z, \xi) = 0 . \end{align*}$$
Thus, one can see that summations in (2.8) is taken over
![]() $q_2 \geqslant M$
. Choosing
$q_2 \geqslant M$
. Choosing
![]() $\varepsilon =1/4$
, say, and using the last fact, we get in view of the Parseval identity that
$\varepsilon =1/4$
, say, and using the last fact, we get in view of the Parseval identity that
 $$\begin{align*}\sigma \ll M^{-1/4} \frac{|A_*|^2}{q_*} \sum_{q_2|q_*,\, q_2>1}\, \sum_{z\in {\mathbb{Z}}^*_{q_2}} |\widehat{B}_* (zq_* q^{-1}_2)|^2 \leqslant M^{-1/4} |A_*|^2 |B_*| . \end{align*}$$
$$\begin{align*}\sigma \ll M^{-1/4} \frac{|A_*|^2}{q_*} \sum_{q_2|q_*,\, q_2>1}\, \sum_{z\in {\mathbb{Z}}^*_{q_2}} |\widehat{B}_* (zq_* q^{-1}_2)|^2 \leqslant M^{-1/4} |A_*|^2 |B_*| . \end{align*}$$
Returning to (2.7), we obtain a contradiction provided
![]() $|B_*| \gg q_* M^{-1/4}$
. In other words, we have for a certain
$|B_*| \gg q_* M^{-1/4}$
. In other words, we have for a certain
![]() $s\geqslant 0$
,
$s\geqslant 0$
,
![]() $\alpha M^{s/4}> 1$
that
$\alpha M^{s/4}> 1$
that
and this implies
![]() $M \ll \beta ^{-4}$
. Thus, in view of our restriction to the divisors of
$M \ll \beta ^{-4}$
. Thus, in view of our restriction to the divisors of
![]() $q_*$
, the condition
$q_*$
, the condition
![]() $\alpha \geqslant \beta $
, the first bound for
$\alpha \geqslant \beta $
, the first bound for
![]() $Q_1$
from (2.6), and the bound for s, which follows from Lemma 2, we get
$Q_1$
from (2.6), and the bound for s, which follows from Lemma 2, we get
as required.
Now, let
![]() $\lambda \in {\mathbb {Z}}_{q_*}$
be an arbitrary element. Write
$\lambda \in {\mathbb {Z}}_{q_*}$
be an arbitrary element. Write
![]() $\lambda = q' \lambda '$
, where
$\lambda = q' \lambda '$
, where
![]() $q' |q$
and
$q' |q$
and
![]() $\lambda '\in {\mathbb {Z}}^*_{q_*/q'}$
. Using the Dirichlet principle, choose a subset of
$\lambda '\in {\mathbb {Z}}^*_{q_*/q'}$
. Using the Dirichlet principle, choose a subset of
![]() $B' \subseteq B_*$
of density at least
$B' \subseteq B_*$
of density at least
![]() $\beta $
such that all elements of a shift of
$\beta $
such that all elements of a shift of
![]() $B'$
are divisible by
$B'$
are divisible by
![]() $q'$
. Then our inclusion can be rewritten as
$q'$
. Then our inclusion can be rewritten as
![]() $\lambda ' \in (A_*-A_*)(B'-B')$
modulo
$\lambda ' \in (A_*-A_*)(B'-B')$
modulo
![]() $q_*/q'$
and we can apply the arguments above replacing module
$q_*/q'$
and we can apply the arguments above replacing module
![]() $q_*$
to
$q_*$
to
![]() $q_*/q'$
.
$q_*/q'$
.
To obtain (2.5), we use the second bound for
![]() $Q_1$
from (2.6) and derive as above
$Q_1$
from (2.6) and derive as above
This completes the proof.
As one can see from the proof of Theorem 2.3 that the constant four in (2.3) can be decreased to
![]() $2+o(1)$
but we leave such calculations to the interested reader.
$2+o(1)$
but we leave such calculations to the interested reader.
3 On the general case
In [Reference Fish5, Problem 2], Fish considered a more general two-dimensional case (actually, in his paper, he had to deal with even more general dynamical setting) and formulated the following problem.
Problem 3.1 [Fish]. Let q be a positive number and
![]() $\mathcal {A}, \mathcal {B} \subseteq {\mathbb {Z}}_q^2$
be sets,
$\mathcal {A}, \mathcal {B} \subseteq {\mathbb {Z}}_q^2$
be sets,
![]() $|\mathcal {A}| = \alpha q^2$
,
$|\mathcal {A}| = \alpha q^2$
,
![]() ${|\mathcal {B}|=\beta q^2}$
, and suppose that
${|\mathcal {B}|=\beta q^2}$
, and suppose that
![]() $\alpha \geqslant \beta $
. Prove that in the case
$\alpha \geqslant \beta $
. Prove that in the case
![]() $\mathcal {A} = \mathcal {B}$
for a certain function F there is
$\mathcal {A} = \mathcal {B}$
for a certain function F there is
![]() $d|q$
such that
$d|q$
such that
![]() $d\leqslant F(\beta )$
and
$d\leqslant F(\beta )$
and
provided
![]() $\beta $
is sufficiently large.
$\beta $
is sufficiently large.
In this section, we study the number
![]() $N_{\mathcal {A}, \mathcal {B}} (\lambda )$
of the solutions to the equation
$N_{\mathcal {A}, \mathcal {B}} (\lambda )$
of the solutions to the equation
and give a partial answer to the problem above. We consider the squarefree case for simplicity and emphasis one more time that our sets
![]() $\mathcal {A}$
,
$\mathcal {A}$
,
![]() $\mathcal {B}$
are arbitrary (in the case of Cartesian products and squarefree
$\mathcal {B}$
are arbitrary (in the case of Cartesian products and squarefree
![]() $q,$
one can apply other methods, see [Reference Pach10]). Also, in the case of prime
$q,$
one can apply other methods, see [Reference Pach10]). Also, in the case of prime
![]() $q,$
we obtain a result of Vinh-type [Reference Vinh22], see asymptotic formula (3.5).
$q,$
we obtain a result of Vinh-type [Reference Vinh22], see asymptotic formula (3.5).
Theorem 3.2 Let q be a squarefree number, let
![]() $\mathcal {A}, \mathcal {B} \subseteq {\mathbb {Z}}_q^2$
be sets,
$\mathcal {A}, \mathcal {B} \subseteq {\mathbb {Z}}_q^2$
be sets,
![]() $|\mathcal {A}| = \alpha q^2$
,
$|\mathcal {A}| = \alpha q^2$
,
![]() $|\mathcal {B}|=\beta q^2$
, and suppose that
$|\mathcal {B}|=\beta q^2$
, and suppose that
![]() $\alpha \geqslant \beta $
. Then
$\alpha \geqslant \beta $
. Then
with
In particular, for
![]() $\mathcal {A} = \mathcal {B},$
one has with the same d that
$\mathcal {A} = \mathcal {B},$
one has with the same d that
In the case when q is a prime number, we have
 $$ \begin{align} \left| N_{\mathcal{A}, \mathcal{B}} (\lambda) - \frac{|\mathcal{A}| |\mathcal{B}|}{q} \right| < 4 q^{7/8} \sqrt{|\mathcal{A}||\mathcal{B}|} . \end{align} $$
$$ \begin{align} \left| N_{\mathcal{A}, \mathcal{B}} (\lambda) - \frac{|\mathcal{A}| |\mathcal{B}|}{q} \right| < 4 q^{7/8} \sqrt{|\mathcal{A}||\mathcal{B}|} . \end{align} $$
In particular, equality (3.1) holds for
![]() $|\mathcal {A}| |\mathcal {B}| \geqslant 16 q^{15/4}$
and
$|\mathcal {A}| |\mathcal {B}| \geqslant 16 q^{15/4}$
and
![]() $d=1$
.
$d=1$
.
Proof We start with (3.3). The proof follows the arguments of the proof of Theorem 2.3, and thus, we use the notation from this result. In particular, writing
![]() $q=p_1^{\rho _1} \dots p_t^{\rho _t}$
,
$q=p_1^{\rho _1} \dots p_t^{\rho _t}$
,
![]() $t=\omega (q)$
with
$t=\omega (q)$
with
![]() $\rho _j=1$
,
$\rho _j=1$
,
![]() $j\in [t]$
we define
$j\in [t]$
we define
![]() $Q_1 = \prod _{j=1}^s p_j$
such that (2.6) holds and further we take
$Q_1 = \prod _{j=1}^s p_j$
such that (2.6) holds and further we take
![]() $\lambda \in {\mathbb {Z}}^*_q$
. The only difference is that one should use Lemma 2 with
$\lambda \in {\mathbb {Z}}^*_q$
. The only difference is that one should use Lemma 2 with
![]() $n=2$
to regularize the two-dimensional set
$n=2$
to regularize the two-dimensional set
![]() $\mathcal {A}$
and let
$\mathcal {A}$
and let
![]() $\varepsilon =1/4$
. For a moment, we assume that
$\varepsilon =1/4$
. For a moment, we assume that
![]() $M \geqslant 100 t^2$
, say, and we will choose the parameter M later. Finally, with some abuse of the notation, we do not use new letters
$M \geqslant 100 t^2$
, say, and we will choose the parameter M later. Finally, with some abuse of the notation, we do not use new letters
![]() $\mathcal {A}_*, \mathcal {B}_*$
,
$\mathcal {A}_*, \mathcal {B}_*$
,
![]() $q_*$
below but the old ones
$q_*$
below but the old ones
![]() $\mathcal {A}, \mathcal {B},$
and q (in other words, one can think that
$\mathcal {A}, \mathcal {B},$
and q (in other words, one can think that
![]() $\mathcal {A}$
is a regularized set already). Also, we utilize the fact that
$\mathcal {A}$
is a regularized set already). Also, we utilize the fact that
![]() ${\mathbb {Z}}_q = {\mathbb {Z}}_{p^{\rho _1}_1} \times \dots \times {\mathbb {Z}}_{p^{\rho _t}_t} = {\mathbb {Z}}_{p^{}_1} \times \dots \times {\mathbb {Z}}_{p^{}_t}$
thanks the Chinese remainder theorem.
${\mathbb {Z}}_q = {\mathbb {Z}}_{p^{\rho _1}_1} \times \dots \times {\mathbb {Z}}_{p^{\rho _t}_t} = {\mathbb {Z}}_{p^{}_1} \times \dots \times {\mathbb {Z}}_{p^{}_t}$
thanks the Chinese remainder theorem.
Now, for
![]() $a = (a_1,a_2)$
and
$a = (a_1,a_2)$
and
![]() $b=(b_1,b_2),$
let us write
$b=(b_1,b_2),$
let us write
![]() $I(a,b) = 1$
if the pair
$I(a,b) = 1$
if the pair
![]() $a,b$
satisfies (3.2) and
$a,b$
satisfies (3.2) and
![]() $I(a,b) = 0$
, otherwise. Then clearly,
$I(a,b) = 0$
, otherwise. Then clearly,
Without loosing of the generality, we assume that
![]() $\lambda =1$
. Obviously,
$\lambda =1$
. Obviously,
![]() $I(a,b) = I(b,a)$
and we can rewrite the matrix
$I(a,b) = I(b,a)$
and we can rewrite the matrix
![]() $I(a,b)$
as
$I(a,b)$
as
![]() $I(a,b) = \sum _{j=1}^{q^2} \mu _j u_j (a) \overline {u}_j (b)$
, where
$I(a,b) = \sum _{j=1}^{q^2} \mu _j u_j (a) \overline {u}_j (b)$
, where
![]() $\mu _j$
are eigenvalues and
$\mu _j$
are eigenvalues and
![]() $u_j (x)$
are correspondent normalized eigenfunctions of I. One can easily check that
$u_j (x)$
are correspondent normalized eigenfunctions of I. One can easily check that
![]() $u_1 (x) = q^{-1} (1,\dots , 1)$
,
$u_1 (x) = q^{-1} (1,\dots , 1)$
,
![]() $\|u_1\|_2 = 1$
and
$\|u_1\|_2 = 1$
and
![]() $\mu _1 = |{\mathbb {Z}}^*_q| = \varphi (q)$
. Writing
$\mu _1 = |{\mathbb {Z}}^*_q| = \varphi (q)$
. Writing
![]() $I'(a,b) = I(a,b) - \mu _1 u_1 (a) \overline {u}_1 (b)$
, we obtain
$I'(a,b) = I(a,b) - \mu _1 u_1 (a) \overline {u}_1 (b)$
, we obtain
 $$ \begin{align} N_{\mathcal{A}, \mathcal{B}} (\lambda) - \frac{|\mathcal{A}| |\mathcal{B}| \varphi (q)}{q^2} = \sum_{a\in \mathcal{A}, b\in \mathcal{B}} I'(a,b) := N^{\prime}_{\mathcal{A}, \mathcal{B}} (\lambda) . \end{align} $$
$$ \begin{align} N_{\mathcal{A}, \mathcal{B}} (\lambda) - \frac{|\mathcal{A}| |\mathcal{B}| \varphi (q)}{q^2} = \sum_{a\in \mathcal{A}, b\in \mathcal{B}} I'(a,b) := N^{\prime}_{\mathcal{A}, \mathcal{B}} (\lambda) . \end{align} $$
By the Cauchy–Schwarz inequality, we get the following.
Here,
![]() $(I')^2$
is the second power of the matrix
$(I')^2$
is the second power of the matrix
![]() $I'$
. Similarly,
$I'$
. Similarly,
![]() $I^2 (a,a') = \sum _{b} I(a,b) I(a',b)$
and the last quantity coincides with the number of the solutions to the equation
$I^2 (a,a') = \sum _{b} I(a,b) I(a',b)$
and the last quantity coincides with the number of the solutions to the equation
 $$ \begin{align} a_2 - a^{\prime}_2 = \frac{a^{\prime}_1-a_1}{(a_1+x)(a^{\prime}_1+x)} , \end{align} $$
$$ \begin{align} a_2 - a^{\prime}_2 = \frac{a^{\prime}_1-a_1}{(a_1+x)(a^{\prime}_1+x)} , \end{align} $$
where
![]() $b=(x,y)$
,
$b=(x,y)$
,
![]() $a=(a_1,a_2)$
and
$a=(a_1,a_2)$
and
![]() $a'=(a^{\prime }_1,a^{\prime }_2)$
. Assume that
$a'=(a^{\prime }_1,a^{\prime }_2)$
. Assume that
![]() $a\neq a'$
and rewrite our equation (3.8) as
$a\neq a'$
and rewrite our equation (3.8) as
 $$ \begin{align} x^2 + (a_1+a^{\prime}_1)x + a_1 a^{\prime}_1 + \frac{a_1-a^{\prime}_1}{a_2-a^{\prime}_2} = 0 , \end{align} $$
$$ \begin{align} x^2 + (a_1+a^{\prime}_1)x + a_1 a^{\prime}_1 + \frac{a_1-a^{\prime}_1}{a_2-a^{\prime}_2} = 0 , \end{align} $$
and its discriminant is
![]() $D'(a,a') := (a_1-a^{\prime }_1) (a_2-a^{\prime }_2)^{-1} [(a_1-a^{\prime }_1) (a_2-a^{\prime }_2) - 4]$
. Notice that if
$D'(a,a') := (a_1-a^{\prime }_1) (a_2-a^{\prime }_2)^{-1} [(a_1-a^{\prime }_1) (a_2-a^{\prime }_2) - 4]$
. Notice that if
![]() $a=a'$
, then we have
$a=a'$
, then we have
![]() $\varphi (q)$
solutions to equation (3.8). By
$\varphi (q)$
solutions to equation (3.8). By
![]() $\chi _p$
denote the Legendre symbol modulo a prime p and let
$\chi _p$
denote the Legendre symbol modulo a prime p and let
![]() $\chi _0$
be the main character (modulo p). We have the identity
$\chi _0$
be the main character (modulo p). We have the identity
![]() $\chi _p (x^{-1}) = \chi _p (x)$
,
$\chi _p (x^{-1}) = \chi _p (x)$
,
![]() $x\in {\mathbb {Z}}_p^*$
and hence
$x\in {\mathbb {Z}}_p^*$
and hence
![]() $\chi _p (D'(a,a')) = \chi _p ( (a_1-a^{\prime }_1) (a_2-a^{\prime }_2)^{} [(a_1-a^{\prime }_1) (a_2-a^{\prime }_2) - 4] := \chi _p (D(a,a'))$
. In view of the Chinese remainder theorem, and our choice of the regularized set
$\chi _p (D'(a,a')) = \chi _p ( (a_1-a^{\prime }_1) (a_2-a^{\prime }_2)^{} [(a_1-a^{\prime }_1) (a_2-a^{\prime }_2) - 4] := \chi _p (D(a,a'))$
. In view of the Chinese remainder theorem, and our choice of the regularized set
![]() $\mathcal {A},$
one has
$\mathcal {A},$
one has
 $$ \begin{align} I^2 (a,a') = \prod_{j=s+1}^t \left( \chi_{p_j} (D(a,a')) + \chi_0 (D(a,a')) + (p_j-1) \delta_{p_j} (a_1-a^{\prime}_1, a_2-a^{\prime}_2) \right) \end{align} $$
$$ \begin{align} I^2 (a,a') = \prod_{j=s+1}^t \left( \chi_{p_j} (D(a,a')) + \chi_0 (D(a,a')) + (p_j-1) \delta_{p_j} (a_1-a^{\prime}_1, a_2-a^{\prime}_2) \right) \end{align} $$
 $$ \begin{align} = \mathcal{E} (a,a') + \prod_{j=s+1}^t (\chi_0 (D(a,a'))+ (p_j-1) \delta_{p_j} (a_1-a^{\prime}_1, a_2-a^{\prime}_2)) = \mathcal{E} (a,a') + \mathcal{E}' (a,a') , \end{align} $$
$$ \begin{align} = \mathcal{E} (a,a') + \prod_{j=s+1}^t (\chi_0 (D(a,a'))+ (p_j-1) \delta_{p_j} (a_1-a^{\prime}_1, a_2-a^{\prime}_2)) = \mathcal{E} (a,a') + \mathcal{E}' (a,a') , \end{align} $$
where for a positive integer
![]() $m,$
we have put
$m,$
we have put
![]() $\delta _m (z,w) = 1$
if
$\delta _m (z,w) = 1$
if
![]() $z \equiv w \equiv 0 \pmod m$
, and 0 otherwise. Equivalently, writing T for the segment
$z \equiv w \equiv 0 \pmod m$
, and 0 otherwise. Equivalently, writing T for the segment
![]() $[s+1,t]$
, one has
$[s+1,t]$
, one has
Notice that
![]() $\mathcal {E} (a,a) = 0$
. From (3.10) and (3.11), it follows that
$\mathcal {E} (a,a) = 0$
. From (3.10) and (3.11), it follows that
![]() $\mathcal {E} u_1 = 0$
. Indeed, we know that
$\mathcal {E} u_1 = 0$
. Indeed, we know that
![]() $I^2 u_1 = \mu _1^2 u_1 = \varphi ^2 (q) u_1$
and
$I^2 u_1 = \mu _1^2 u_1 = \varphi ^2 (q) u_1$
and
 $$ \begin{align} \sum_a \prod_{j=s+1}^t (\chi_0 (D(a,a'))+ (p_j-1) \delta_{p_j} (a_1-a^{\prime}_1, a_2-a^{\prime}_2)) \end{align} $$
$$ \begin{align} \sum_a \prod_{j=s+1}^t (\chi_0 (D(a,a'))+ (p_j-1) \delta_{p_j} (a_1-a^{\prime}_1, a_2-a^{\prime}_2)) \end{align} $$
 $$ \begin{align} = \prod_{j=s+1}^t \left( \sum_{z,w\in {\mathbb{Z}}_{p_j}} \chi_0((zw)^2 - 4zw) + p_j-1 \right) \end{align} $$
$$ \begin{align} = \prod_{j=s+1}^t \left( \sum_{z,w\in {\mathbb{Z}}_{p_j}} \chi_0((zw)^2 - 4zw) + p_j-1 \right) \end{align} $$
 $$ \begin{align} \qquad= \prod_{j=s+1}^t \left( (p_j-1) \sum_{z\in {\mathbb{Z}}_{p_j}} \chi_0(z^2 - 4z) + p_j-1 \right) = \prod_{j=s+1}^t (p_j-1)^2 = \varphi^2 (q) . \end{align} $$
$$ \begin{align} \qquad= \prod_{j=s+1}^t \left( (p_j-1) \sum_{z\in {\mathbb{Z}}_{p_j}} \chi_0(z^2 - 4z) + p_j-1 \right) = \prod_{j=s+1}^t (p_j-1)^2 = \varphi^2 (q) . \end{align} $$
Hence, in very deed
![]() $\mathcal {E} u_1 = 0,$
and thus
$\mathcal {E} u_1 = 0,$
and thus
where
![]() $f_{\mathcal {A}} (a) = \mathcal {A} (a) - \langle \mathcal {A}, u_1 \rangle u_1 (a)$
,
$f_{\mathcal {A}} (a) = \mathcal {A} (a) - \langle \mathcal {A}, u_1 \rangle u_1 (a)$
,
![]() $\sum _a f_{\mathcal {A}} (a) = 0$
. Let us estimate the term
$\sum _a f_{\mathcal {A}} (a) = 0$
. Let us estimate the term
![]() ${r:=\langle \mathcal {E}' f_{\mathcal {A}}, f_{\mathcal {A}} \rangle }$
rather roughly. Since the function
${r:=\langle \mathcal {E}' f_{\mathcal {A}}, f_{\mathcal {A}} \rangle }$
rather roughly. Since the function
![]() $f_{\mathcal {A}}$
is orthogonal to
$f_{\mathcal {A}}$
is orthogonal to
![]() $u_1$
and
$u_1$
and
![]() $\| f_{\mathcal {A}}\|_\infty \leqslant 1$
, it follows that:
$\| f_{\mathcal {A}}\|_\infty \leqslant 1$
, it follows that:
 $$\begin{align*}|r|= \left| \sum_{a,a'} f_{\mathcal{A}} (a) f_{\mathcal{A}} (a') \prod_{j=s+1}^t \left(1- \delta_{p_j} (D(a,a')) +(p_j-1) \delta_{p_j} (a_1-a^{\prime}_1, a_2-a^{\prime}_2) \right) \right| \end{align*}$$
$$\begin{align*}|r|= \left| \sum_{a,a'} f_{\mathcal{A}} (a) f_{\mathcal{A}} (a') \prod_{j=s+1}^t \left(1- \delta_{p_j} (D(a,a')) +(p_j-1) \delta_{p_j} (a_1-a^{\prime}_1, a_2-a^{\prime}_2) \right) \right| \end{align*}$$
 $$\begin{align*}\leqslant \sum_{\emptyset \neq S\subseteq T} \left| \sum_{a,a'} f_{\mathcal{A}} (a) f_{\mathcal{A}} (a') \prod_{j\in S} (- \delta_{p_j} (D(a,a')) +(p_j-1) \delta_{p_j} (a_1-a^{\prime}_1, a_2-a^{\prime}_2) ) \right| \end{align*}$$
$$\begin{align*}\leqslant \sum_{\emptyset \neq S\subseteq T} \left| \sum_{a,a'} f_{\mathcal{A}} (a) f_{\mathcal{A}} (a') \prod_{j\in S} (- \delta_{p_j} (D(a,a')) +(p_j-1) \delta_{p_j} (a_1-a^{\prime}_1, a_2-a^{\prime}_2) ) \right| \end{align*}$$
 $$\begin{align*}\leqslant 2|\mathcal{A}| q^2 \sum_{n=1}^{t-s}\, \sum_{S\subseteq T,\, |S|=n}\, \prod_{j\in S} \left( \frac{3}{p_j} + \frac{p_j-1}{p^2_j} \right) \leqslant 2|\mathcal{A}| q^2 \sum_{n=1}^{t-s}\, \binom{t-s}{n} \left(\frac{4}{M} \right)^n \end{align*}$$
$$\begin{align*}\leqslant 2|\mathcal{A}| q^2 \sum_{n=1}^{t-s}\, \sum_{S\subseteq T,\, |S|=n}\, \prod_{j\in S} \left( \frac{3}{p_j} + \frac{p_j-1}{p^2_j} \right) \leqslant 2|\mathcal{A}| q^2 \sum_{n=1}^{t-s}\, \binom{t-s}{n} \left(\frac{4}{M} \right)^n \end{align*}$$
Now, returning to the definition of the operator
![]() $\mathcal {E} (a,a')$
, recalling estimate (3.8) and using the Cauchy–Schwarz inequality, we obtain
$\mathcal {E} (a,a')$
, recalling estimate (3.8) and using the Cauchy–Schwarz inequality, we obtain
The term with
![]() $a \equiv a' \pmod q$
gives us a contribution at most
$a \equiv a' \pmod q$
gives us a contribution at most
![]() $4^{t} |\mathcal {A}| q^2$
into the last sum (see (3.12)—(3.14) to estimate
$4^{t} |\mathcal {A}| q^2$
into the last sum (see (3.12)—(3.14) to estimate
![]() $\| w_{p_j}\|_1$
for
$\| w_{p_j}\|_1$
for
![]() $j\notin S$
and use the trivial fact that
$j\notin S$
and use the trivial fact that
![]() $\|\chi _p \|_{\infty } \leqslant 1$
to bound the rest). Now, let
$\|\chi _p \|_{\infty } \leqslant 1$
to bound the rest). Now, let
![]() $a \neq a' \pmod q$
but
$a \neq a' \pmod q$
but
![]() $a \equiv a' \pmod {q_*}$
with maximal
$a \equiv a' \pmod {q_*}$
with maximal
![]() $q_*|q$
. Thus
$q_*|q$
. Thus
![]() $q_* \neq q$
and
$q_* \neq q$
and
![]() $Q_1|q_*$
. We can write
$Q_1|q_*$
. We can write
![]() $q_* = q_* (W) = Q_1 \prod _{j\in W} p_j$
for a certain (possibly empty) set
$q_* = q_* (W) = Q_1 \prod _{j\in W} p_j$
for a certain (possibly empty) set
![]() $W \subseteq T$
. Let us say that all primes p such that
$W \subseteq T$
. Let us say that all primes p such that
![]() $p|(q/q_*)$
(that is,
$p|(q/q_*)$
(that is,
![]() $p|q$
and
$p|q$
and
![]() $p\notin W$
) are good. In particular, for all good primes
$p\notin W$
) are good. In particular, for all good primes
![]() $p,$
one has
$p,$
one has
![]() $p>M$
. Now for a good prime
$p>M$
. Now for a good prime
![]() $p,$
the sum above
$p,$
the sum above
![]() $\sum _{x,y \mod {\mathbb {Z}}_p}\chi _p (D(x,y), (a_1,a_2))$
(or, analogously, the sum
$\sum _{x,y \mod {\mathbb {Z}}_p}\chi _p (D(x,y), (a_1,a_2))$
(or, analogously, the sum
![]() $\sum _{x,y \mod {\mathbb {Z}}_p}\chi _p (D(x,y), (a^{\prime }_1,a^{\prime }_2))$
) is either at most
$\sum _{x,y \mod {\mathbb {Z}}_p}\chi _p (D(x,y), (a^{\prime }_1,a^{\prime }_2))$
) is either at most
![]() $3p^{3/2}$
by Weil, or the sum over y is p if
$3p^{3/2}$
by Weil, or the sum over y is p if
![]() $\frac {2}{x-a_1} + a_2 = \frac {2}{x-a^{\prime }_1} + a^{\prime }_2$
modulo p. The last equation is nontrivial one by our choice of
$\frac {2}{x-a_1} + a_2 = \frac {2}{x-a^{\prime }_1} + a^{\prime }_2$
modulo p. The last equation is nontrivial one by our choice of
![]() $p,$
hence it has at most two solutions, and thus, in any case, the sum over
$p,$
hence it has at most two solutions, and thus, in any case, the sum over
![]() $x,y \mod {\mathbb {Z}}_p$
is at most
$x,y \mod {\mathbb {Z}}_p$
is at most
![]() $3p^{3/2} < 3p^2/\sqrt {M}$
. Further, we split the sets
$3p^{3/2} < 3p^2/\sqrt {M}$
. Further, we split the sets
![]() $S_1,S_2$
as
$S_1,S_2$
as
![]() $S_1 = S^*_1 \bigsqcup G_1$
,
$S_1 = S^*_1 \bigsqcup G_1$
,
![]() $S_2 = S^*_2 \bigsqcup G_2$
, where (possibly empty) sets
$S_2 = S^*_2 \bigsqcup G_2$
, where (possibly empty) sets
![]() $G_1, G_2$
correspond to good primes and the sets
$G_1, G_2$
correspond to good primes and the sets
![]() $S^*_1 \subseteq W$
,
$S^*_1 \subseteq W$
,
![]() $S^*_2 \subseteq W$
correspond to the divisors of
$S^*_2 \subseteq W$
correspond to the divisors of
![]() $q_* (W)$
. Since
$q_* (W)$
. Since
![]() $S_1, S_2 \neq \emptyset $
, it follows that either
$S_1, S_2 \neq \emptyset $
, it follows that either
![]() $G_1 \bigcup G_2 \neq \emptyset $
or
$G_1 \bigcup G_2 \neq \emptyset $
or
![]() $S^*_1,S^*_2 \neq \emptyset $
. Using the notation as in (2.9), namely,
$S^*_1,S^*_2 \neq \emptyset $
. Using the notation as in (2.9), namely,
we see that the number of pairs
![]() $a\equiv a' \pmod {\tilde {q}}$
is exactly
$a\equiv a' \pmod {\tilde {q}}$
is exactly
![]() $\| \eta _{\tilde {q}} \|_2^2$
for any
$\| \eta _{\tilde {q}} \|_2^2$
for any
![]() $\tilde {q} |q$
and one can use bound (2.11) to estimate the last quantity. Now, recalling inequality (2.1) and splitting sum (3.17) according the case
$\tilde {q} |q$
and one can use bound (2.11) to estimate the last quantity. Now, recalling inequality (2.1) and splitting sum (3.17) according the case
![]() $W \neq \emptyset $
or not, we get
$W \neq \emptyset $
or not, we get
 $$ \begin{align} 4^{t} |\mathcal{A}| q^2 + q^2 \sum_{\emptyset \neq W \subseteq T}\, \sum_{a,a' \in \mathcal{A},\, a \equiv a' \pmod {q(W)}} 4^{|W|} + q^2 \sum_{a,a' \in \mathcal{A}} \sum_{n+m\geqslant 1} \binom{t-s}{n} \binom{t-s}{m} \left(\frac{3}{\sqrt{M}} \right)^{n+m} \end{align} $$
$$ \begin{align} 4^{t} |\mathcal{A}| q^2 + q^2 \sum_{\emptyset \neq W \subseteq T}\, \sum_{a,a' \in \mathcal{A},\, a \equiv a' \pmod {q(W)}} 4^{|W|} + q^2 \sum_{a,a' \in \mathcal{A}} \sum_{n+m\geqslant 1} \binom{t-s}{n} \binom{t-s}{m} \left(\frac{3}{\sqrt{M}} \right)^{n+m} \end{align} $$
Using (3.6), (3.7), (3.8), (3.16), and the Cauchy–Schwarz inequality, we get
 $$ \begin{align} N_{\mathcal{A}, \mathcal{B}} (\lambda) - \frac{|\mathcal{A}| |\mathcal{B}| \varphi (q)}{q^2} \ll (t^{} M^{-1/2})^{1/4} \cdot |\mathcal{A}|^{3/4} \sqrt{|\mathcal{B}| q} + (t M^{-1})^{1/2} \cdot \sqrt{|\mathcal{A}| |\mathcal{B}|} q . \end{align} $$
$$ \begin{align} N_{\mathcal{A}, \mathcal{B}} (\lambda) - \frac{|\mathcal{A}| |\mathcal{B}| \varphi (q)}{q^2} \ll (t^{} M^{-1/2})^{1/4} \cdot |\mathcal{A}|^{3/4} \sqrt{|\mathcal{B}| q} + (t M^{-1})^{1/2} \cdot \sqrt{|\mathcal{A}| |\mathcal{B}|} q . \end{align} $$
We have
![]() $\varphi (q) \gg q/\log t,$
and hence after some calculations, we see that
$\varphi (q) \gg q/\log t,$
and hence after some calculations, we see that
![]() $N_{\mathcal {A}, \mathcal {B}} (\lambda )> 0$
provided
$N_{\mathcal {A}, \mathcal {B}} (\lambda )> 0$
provided
![]() $M\gg t^{2} \beta ^{-6} \log ^8 t$
. As in Theorem 2.3, one has
$M\gg t^{2} \beta ^{-6} \log ^8 t$
. As in Theorem 2.3, one has
![]() $Q_1 \leqslant M^t$
, and thus
$Q_1 \leqslant M^t$
, and thus
In the case of prime
![]() $q,$
the argument is even simpler because one do not need the regularization, the second term in (3.19) plus the quantity r is negligible, see estimate (3.16). Finally, let
$q,$
the argument is even simpler because one do not need the regularization, the second term in (3.19) plus the quantity r is negligible, see estimate (3.16). Finally, let
![]() $\mathcal {A} = \mathcal {B}$
and if
$\mathcal {A} = \mathcal {B}$
and if
![]() $\lambda \notin {\mathbb {Z}}^*_q$
, then write
$\lambda \notin {\mathbb {Z}}^*_q$
, then write
![]() $\lambda = q' \lambda '$
, where
$\lambda = q' \lambda '$
, where
![]() $q' |q$
and
$q' |q$
and
![]() $\lambda '\in {\mathbb {Z}}^*_{q_*/q'}$
. Using the Dirichlet principle, choose a subset of
$\lambda '\in {\mathbb {Z}}^*_{q_*/q'}$
. Using the Dirichlet principle, choose a subset of
![]() $\mathcal {A}' \subseteq \mathcal {A}$
of density at least
$\mathcal {A}' \subseteq \mathcal {A}$
of density at least
![]() $\alpha $
such that
$\alpha $
such that
![]() $|\pi _{q'} (\mathcal {A}')|=1$
. Then the required inclusion (3.4) can be rewritten as
$|\pi _{q'} (\mathcal {A}')|=1$
. Then the required inclusion (3.4) can be rewritten as
and we can apply the arguments above replacing
![]() $q_*$
to
$q_*$
to
![]() $q_*/q'$
. This completes the proof.
$q_*/q'$
. This completes the proof.
Remark 3.3 Of course, inclusion (3.4) does not hold for
![]() $\mathcal {A} \neq \mathcal {B}$
, just take
$\mathcal {A} \neq \mathcal {B}$
, just take
![]() ${\mathcal {A} = (d \cdot {\mathbb {Z}}_q) \times (d \cdot {\mathbb {Z}}_q)}$
and
${\mathcal {A} = (d \cdot {\mathbb {Z}}_q) \times (d \cdot {\mathbb {Z}}_q)}$
and
![]() $\mathcal {B} = (d \cdot {\mathbb {Z}}_q + 1) \times (d \cdot {\mathbb {Z}}_q + 1)$
for an arbitrary
$\mathcal {B} = (d \cdot {\mathbb {Z}}_q + 1) \times (d \cdot {\mathbb {Z}}_q + 1)$
for an arbitrary
![]() $d|q$
,
$d|q$
,
![]() $1< d \ll 1$
. Also, the author thinks that the error term in (3.5) can be improved but this weaker bound is enough for us to resolve our equation for sets of positive densities.
$1< d \ll 1$
. Also, the author thinks that the error term in (3.5) can be improved but this weaker bound is enough for us to resolve our equation for sets of positive densities.
Remark 3.4 The attentive reader may be alerted that we have two different main terms in (2.7) and in (3.20). Nevertheless, they are asymptotically the same due to the fact that in (3.20), our parameter M depends on growing quantity
![]() $\omega (q)$
.
$\omega (q)$
.
Similarly, we obtain an affirmative answer to [Reference Fish5, Problem 1] in the case of squarefree q. By
![]() $M_{\mathcal {A}, \mathcal {B}} (\lambda ),$
denote the number of the solutions to the equation
$M_{\mathcal {A}, \mathcal {B}} (\lambda ),$
denote the number of the solutions to the equation
Theorem 3.5 Let q be a squarefree number, let
![]() $\mathcal {A}, \mathcal {B} \subseteq \mathbb {F}_q^2$
be sets,
$\mathcal {A}, \mathcal {B} \subseteq \mathbb {F}_q^2$
be sets,
![]() $|\mathcal {A}| = \alpha q^2$
,
$|\mathcal {A}| = \alpha q^2$
,
![]() $|\mathcal {B}|=\beta q^2$
, and suppose that
$|\mathcal {B}|=\beta q^2$
, and suppose that
![]() $\alpha \geqslant \beta $
. Then
$\alpha \geqslant \beta $
. Then
with
In particular, for
![]() $\mathcal {A} = \mathcal {B},$
one has with the same d that
$\mathcal {A} = \mathcal {B},$
one has with the same d that
In the case when q is a prime number, one has
 $$ \begin{align} M_{\mathcal{A}, \mathcal{B}} (\lambda) - \frac{|\mathcal{A}| |\mathcal{B}|}{q} < 4 q^{7/8} \sqrt{|\mathcal{A}||\mathcal{B}|} . \end{align} $$
$$ \begin{align} M_{\mathcal{A}, \mathcal{B}} (\lambda) - \frac{|\mathcal{A}| |\mathcal{B}|}{q} < 4 q^{7/8} \sqrt{|\mathcal{A}||\mathcal{B}|} . \end{align} $$
Proof The argument differs from the proof of Theorem 3.2 in some unimportant details only, so we use the notation from the former result. Indeed, for
![]() $a = (a_1,a_2)$
and
$a = (a_1,a_2)$
and
![]() $b=(b_1,b_2),$
we write
$b=(b_1,b_2),$
we write
![]() $\tilde {I}(a,b) = 1$
if the pair
$\tilde {I}(a,b) = 1$
if the pair
![]() $a,b$
satisfies (3.21) and
$a,b$
satisfies (3.21) and
![]() $\tilde {I}(a,b) = 0,$
otherwise. Calculating
$\tilde {I}(a,b) = 0,$
otherwise. Calculating
![]() $\tilde {I}^2 (a,a')$
, we arrive to the equation
$\tilde {I}^2 (a,a')$
, we arrive to the equation
and hence, we can find x via y or y via x, provided
![]() $a\neq a' \pmod q$
. Assuming that
$a\neq a' \pmod q$
. Assuming that
![]() $a^{\prime }_2 \neq a_2$
, say, we derive
$a^{\prime }_2 \neq a_2$
, say, we derive
 $$\begin{align*}y= \frac{(a^{\prime}_1)^2-a_1^2 +a_2^2 - (a^{\prime}_2)^2}{2(a_2 - a^{\prime}_2)} + \frac{a_1-a^{\prime}_1}{a_2 - a^{\prime}_2} \cdot x = s + t x , \end{align*}$$
$$\begin{align*}y= \frac{(a^{\prime}_1)^2-a_1^2 +a_2^2 - (a^{\prime}_2)^2}{2(a_2 - a^{\prime}_2)} + \frac{a_1-a^{\prime}_1}{a_2 - a^{\prime}_2} \cdot x = s + t x , \end{align*}$$
and hence substituting the last expression into (3.21) and computing the discriminant
![]() $\tilde {D} (a,a')$
(without loss of the generality, we put
$\tilde {D} (a,a')$
(without loss of the generality, we put
![]() $\lambda =1$
), one obtains
$\lambda =1$
), one obtains
As in the proof of Theorem 3.2, we consider
![]() $\mathcal {E} (a,a')$
, take good primes and so on. The first eigenvalue
$\mathcal {E} (a,a')$
, take good primes and so on. The first eigenvalue
![]() $\mu _1$
equals the number of the solutions to the equation
$\mu _1$
equals the number of the solutions to the equation
![]() $x^2-y^2 \equiv 1 \pmod q$
, that is,
$x^2-y^2 \equiv 1 \pmod q$
, that is,
![]() $\varphi (q)$
again. Also,
$\varphi (q)$
again. Also,
![]() $\tilde {I}^{2} (a,a) = \mu _1$
and for
$\tilde {I}^{2} (a,a) = \mu _1$
and for
![]() $a\neq a'$
the quantity
$a\neq a'$
the quantity
![]() $\tilde {I}^{2} (a,a')$
expressed exactly as in (3.10) (with another discriminant
$\tilde {I}^{2} (a,a')$
expressed exactly as in (3.10) (with another discriminant
![]() $\tilde {D}$
, of course), and thus, one can check that
$\tilde {D}$
, of course), and thus, one can check that
![]() $\mathcal {E} u_1$
vanishes making calculations as in (3.12)—(3.14). Further, as in Theorem 3.2, we apply the standard Weil bound to estimate the sum of characters. For any good prime
$\mathcal {E} u_1$
vanishes making calculations as in (3.12)—(3.14). Further, as in Theorem 3.2, we apply the standard Weil bound to estimate the sum of characters. For any good prime
![]() $p,$
it gives us a nontrivial bound of the form
$p,$
it gives us a nontrivial bound of the form
![]() $O(p^{3/2}) = O(p^2/\sqrt {M}),$
and hence, we obtain (3.22) and thus (3.23) by the same argument as at the end of Theorem 3.2 (one can check or see below that all obtained varieties are non–degenerated). Finally, to get (3.24), we need to estimate
$O(p^{3/2}) = O(p^2/\sqrt {M}),$
and hence, we obtain (3.22) and thus (3.23) by the same argument as at the end of Theorem 3.2 (one can check or see below that all obtained varieties are non–degenerated). Finally, to get (3.24), we need to estimate
and by the Weil estimate, it is at most
![]() $20 q^{3/2}$
, say, excluding the case
$20 q^{3/2}$
, say, excluding the case
![]() $\tilde {D}((x,y),(a_1,a_2))$
is proportional to
$\tilde {D}((x,y),(a_1,a_2))$
is proportional to
![]() $\tilde {D}((x,y),(a^{\prime }_1,a^{\prime }_2))$
. In particular, it means that the coefficients of these polynomials are proportional ones and using (3.26) and comparing the coefficients before the highest degrees in x, say, we get
$\tilde {D}((x,y),(a^{\prime }_1,a^{\prime }_2))$
. In particular, it means that the coefficients of these polynomials are proportional ones and using (3.26) and comparing the coefficients before the highest degrees in x, say, we get
![]() $\frac {a_2-2a_1-y}{(y-a_2)^4} = \frac {a^{\prime }_2-2a^{\prime }_1-y}{(y-a^{\prime }_2)^4}$
. Again, thanks to
$\frac {a_2-2a_1-y}{(y-a_2)^4} = \frac {a^{\prime }_2-2a^{\prime }_1-y}{(y-a^{\prime }_2)^4}$
. Again, thanks to
![]() $a \neq a',$
we see that this equation is nontrivial one, and hence, it has at most four solutions. It follows that our sum is at most
$a \neq a',$
we see that this equation is nontrivial one, and hence, it has at most four solutions. It follows that our sum is at most
![]() $4q$
in this case. Thus, as in (3.19) and (3.20), we have
$4q$
in this case. Thus, as in (3.19) and (3.20), we have
 $$\begin{align*}M_{\mathcal{A}, \mathcal{B}} (\lambda) - \frac{|\mathcal{A}| |\mathcal{B}| \varphi (q)}{q^2} \leqslant 3 (q^{3/2} |\mathcal{A}| )^ {1/4} \sqrt{|\mathcal{A}| |\mathcal{B}|} \leqslant 3 q^{7/8} \sqrt{|\mathcal{A}| |\mathcal{B}|} . \end{align*}$$
$$\begin{align*}M_{\mathcal{A}, \mathcal{B}} (\lambda) - \frac{|\mathcal{A}| |\mathcal{B}| \varphi (q)}{q^2} \leqslant 3 (q^{3/2} |\mathcal{A}| )^ {1/4} \sqrt{|\mathcal{A}| |\mathcal{B}|} \leqslant 3 q^{7/8} \sqrt{|\mathcal{A}| |\mathcal{B}|} . \end{align*}$$
This completes the proof.
Remark 3.6 We have used a direct way of the proof of Theorem 3.5, another approach is to notice that
![]() $\tilde {I}(a,b) = I(ga,gb)$
, where the linear transformation g is given by the formula
$\tilde {I}(a,b) = I(ga,gb)$
, where the linear transformation g is given by the formula
![]() $g(x,y) = (x+y,x-y)$
. After that one can apply Theorem 3.2 with the sets
$g(x,y) = (x+y,x-y)$
. After that one can apply Theorem 3.2 with the sets
![]() $g^{-1}(\mathcal {A})$
,
$g^{-1}(\mathcal {A})$
,
![]() $g^{-1}(\mathcal {B})$
.
$g^{-1}(\mathcal {B})$
.
Also, let us remark that one can consider the equation
![]() $(a_1-b_1)^2 + (a_2 - b_2)^2 = \lambda \,{\neq}\, 0,$
instead of (3.21), that is the question about the distance between points
$(a_1-b_1)^2 + (a_2 - b_2)^2 = \lambda \,{\neq}\, 0,$
instead of (3.21), that is the question about the distance between points
![]() $(a_1,a_2) \in \mathcal {A}$
and
$(a_1,a_2) \in \mathcal {A}$
and
![]() $ (b_1,b_2) \in \mathcal {B}$
. We leave it to the interested reader to check that all parts of the proof have remained almost the same (formula (3.25), the identity
$ (b_1,b_2) \in \mathcal {B}$
. We leave it to the interested reader to check that all parts of the proof have remained almost the same (formula (3.25), the identity
![]() $\mu _1 = \varphi (q)$
are exactly the same).
$\mu _1 = \varphi (q)$
are exactly the same).
4 On an application of group actions
In this section, we discuss another approach to results of Fish-type, namely, we consider an intermediate situation between Theorems 2.3 and 3.2, our set
![]() $\mathcal {A} \subseteq {\mathbb {Z}}^2_q$
is an arbitrary but the set
$\mathcal {A} \subseteq {\mathbb {Z}}^2_q$
is an arbitrary but the set
![]() $\mathcal {B} \subseteq {\mathbb {Z}}^2_q$
is a Cartesian product. In this case, one can deal with rather general q (and not just squarefree). For simplicity, we do not do any regularization as in the previous section immediately assuming that all prime factors of q are large.
$\mathcal {B} \subseteq {\mathbb {Z}}^2_q$
is a Cartesian product. In this case, one can deal with rather general q (and not just squarefree). For simplicity, we do not do any regularization as in the previous section immediately assuming that all prime factors of q are large.
In the proof, we follow the methods from [Reference Bourgain and Gamburd3] and [Reference Shkredov17].
Theorem 4.1 Let q be a positive odd integer, and let
![]() $\mathcal {A}, \mathcal {B} \subseteq {\mathbb {Z}}_q^2$
be sets,
$\mathcal {A}, \mathcal {B} \subseteq {\mathbb {Z}}_q^2$
be sets,
![]() $|\mathcal {A}| = \delta q^2$
,
$|\mathcal {A}| = \delta q^2$
,
![]() $\mathcal {B} = A\times B$
,
$\mathcal {B} = A\times B$
,
![]() $|A| = \alpha q$
,
$|A| = \alpha q$
,
![]() $|B| = \beta q$
. Suppose that all prime divisors of q are at least M, where
$|B| = \beta q$
. Suppose that all prime divisors of q are at least M, where
and
![]() $C_1, C_2>0$
are absolute constants. Then
$C_1, C_2>0$
are absolute constants. Then
Proof Let
![]() $q=p^{\rho _1}_1 \dots p^{\rho _t}_t$
, where
$q=p^{\rho _1}_1 \dots p^{\rho _t}_t$
, where
![]() $p_j$
are different odd primes and
$p_j$
are different odd primes and
![]() $\rho _j$
are positive integers. By our assumption
$\rho _j$
are positive integers. By our assumption
![]() $p_j \geqslant M$
for all
$p_j \geqslant M$
for all
![]() $j\in [t]$
. Without loosing of the generality, one can take
$j\in [t]$
. Without loosing of the generality, one can take
![]() $\lambda = -1$
in formula (3.2). Recall that
$\lambda = -1$
in formula (3.2). Recall that
![]() $\mathrm {SL}_2({\mathbb {Z}}_q)$
acts on
$\mathrm {SL}_2({\mathbb {Z}}_q)$
acts on
![]() ${\mathbb {Z}}_q$
via Möbius transformations:
${\mathbb {Z}}_q$
via Möbius transformations:
![]() $x\to gx = \frac {ax+b}{cx+d}$
, where
$x\to gx = \frac {ax+b}{cx+d}$
, where
 $g=\left (\begin {array}{cc} a & b \\ c & d \end {array}\right )$
(for composite
$g=\left (\begin {array}{cc} a & b \\ c & d \end {array}\right )$
(for composite
![]() $q,$
the equivalence is taken over
$q,$
the equivalence is taken over
![]() ${\mathbb {Z}}^*_q$
, of course). Since
${\mathbb {Z}}^*_q$
, of course). Since
![]() $\mathcal {B} = A\times B,$
we can rewrite our equation (3.2) as
$\mathcal {B} = A\times B,$
we can rewrite our equation (3.2) as
where
![]() $G \subset \mathrm {SL}_2 ({\mathbb {Z}}_q)$
is the set of matrices of the form
$G \subset \mathrm {SL}_2 ({\mathbb {Z}}_q)$
is the set of matrices of the form
 $$\begin{align*}g= \left( {\begin{array}{cc} -\alpha & \alpha \beta+1 \\ -1 & \beta \\ \end{array} } \right) \,, \quad \quad (\alpha,\beta) \in \mathcal{A} , \end{align*}$$
$$\begin{align*}g= \left( {\begin{array}{cc} -\alpha & \alpha \beta+1 \\ -1 & \beta \\ \end{array} } \right) \,, \quad \quad (\alpha,\beta) \in \mathcal{A} , \end{align*}$$
see [Reference Shkredov17, Section 5] or just make a direct calculation. Clearly,
![]() $|G| = |\mathcal {A}|$
. Further by [Reference Shkredov17, Lemma 15], the multiplicative energy
$|G| = |\mathcal {A}|$
. Further by [Reference Shkredov17, Lemma 15], the multiplicative energy
![]() $\mathsf {E}(G)$
of the set G, that is,
$\mathsf {E}(G)$
of the set G, that is,
coincides with the number of the solutions to the system
 $$\begin{align*}&\beta_1 - \beta_2 = \beta_3 - \beta_4 := s \,, \quad \quad s(\alpha_1 - \alpha_3) = s(\alpha_2 - \alpha_4) = 0 \,, \\& \alpha_1 - \alpha_2 - \alpha_1 \alpha_2 s = \alpha_3 - \alpha_4 - \alpha_3 \alpha_4 s , \end{align*}$$
$$\begin{align*}&\beta_1 - \beta_2 = \beta_3 - \beta_4 := s \,, \quad \quad s(\alpha_1 - \alpha_3) = s(\alpha_2 - \alpha_4) = 0 \,, \\& \alpha_1 - \alpha_2 - \alpha_1 \alpha_2 s = \alpha_3 - \alpha_4 - \alpha_3 \alpha_4 s , \end{align*}$$
where
![]() $(\alpha _i,\beta _i) \in \mathcal {A}$
,
$(\alpha _i,\beta _i) \in \mathcal {A}$
,
![]() $i\in [4]$
. Let
$i\in [4]$
. Let
![]() $s = d s'$
, where d is a divisor of q and
$s = d s'$
, where d is a divisor of q and
![]() $s'$
is coprime to q. Taking
$s'$
is coprime to q. Taking
![]() $(\alpha _1,\beta _1), (\alpha _4,\beta _4) \in \mathcal {A}$
, we find
$(\alpha _1,\beta _1), (\alpha _4,\beta _4) \in \mathcal {A}$
, we find
![]() $\beta _2$
,
$\beta _2$
,
![]() $\beta _3$
from the first equation and
$\beta _3$
from the first equation and
![]() $\alpha _2,\alpha _3$
modulo
$\alpha _2,\alpha _3$
modulo
![]() $q/d$
from the second one. Also, using
$q/d$
from the second one. Also, using
![]() $\alpha _3$
we can reconstruct
$\alpha _3$
we can reconstruct
![]() $\alpha _2$
from the third equation, provided
$\alpha _2$
from the third equation, provided
![]() $d>1$
. In other words, for fixed
$d>1$
. In other words, for fixed
![]() $d,$
there are
$d,$
there are
![]() $q/d$
possibilities for
$q/d$
possibilities for
![]() $s'$
and d possibilities for
$s'$
and d possibilities for
![]() $\alpha _3$
. Finally, if
$\alpha _3$
. Finally, if
![]() $d=1$
, then we have at most
$d=1$
, then we have at most
![]() $q|G|^2$
solutions. Thus, we obtain the bound
$q|G|^2$
solutions. Thus, we obtain the bound
 $$ \begin{align} \mathsf{E}(G) \leqslant |G|^2 \sum_{d|q} \frac{q}{d} \cdot d \leqslant \tau(q) q |G|^2 . \end{align} $$
$$ \begin{align} \mathsf{E}(G) \leqslant |G|^2 \sum_{d|q} \frac{q}{d} \cdot d \leqslant \tau(q) q |G|^2 . \end{align} $$
Now, let us say a few words about representations of the group
![]() $\mathrm {SL}_2 ({\mathbb {Z}}_q)$
(see [Reference Bourgain and Gamburd3, Sections 7 and 8]). First of all, for any irreducible representation
$\mathrm {SL}_2 ({\mathbb {Z}}_q)$
(see [Reference Bourgain and Gamburd3, Sections 7 and 8]). First of all, for any irreducible representation
![]() $\rho _q$
of
$\rho _q$
of
![]() $\mathrm {SL}_2 ({\mathbb {Z}}_q),$
we have
$\mathrm {SL}_2 ({\mathbb {Z}}_q),$
we have
![]() $\rho = \rho _q = \rho _{p^{\rho _1}_1} \otimes \dots \otimes \rho _{p^{\rho _t}_t}$
, and hence, it is sufficient to understand the representation theory for
$\rho = \rho _q = \rho _{p^{\rho _1}_1} \otimes \dots \otimes \rho _{p^{\rho _t}_t}$
, and hence, it is sufficient to understand the representation theory for
![]() $\mathrm {SL}_2 ({\mathbb {Z}}_{p^n})$
, where p is a prime number and n is a positive integer. Now, by [Reference Bourgain and Gamburd3, Lemma 7.1], we know that for any odd prime, the dimension of any faithful irreducible representation of
$\mathrm {SL}_2 ({\mathbb {Z}}_{p^n})$
, where p is a prime number and n is a positive integer. Now, by [Reference Bourgain and Gamburd3, Lemma 7.1], we know that for any odd prime, the dimension of any faithful irreducible representation of
![]() $\mathrm {SL}_2 ({\mathbb {Z}}_{p^n})$
is at least
$\mathrm {SL}_2 ({\mathbb {Z}}_{p^n})$
is at least
![]() $2^{-1} p^{n-2} (p-1)(p+1)$
. For an arbitrary
$2^{-1} p^{n-2} (p-1)(p+1)$
. For an arbitrary
![]() $r\leqslant n,$
we can consider the natural projection
$r\leqslant n,$
we can consider the natural projection
![]() $\pi _r : \mathrm {SL}_2 ({\mathbb {Z}}_{p^n}) \to \mathrm {SL}_2 ({\mathbb {Z}}_{p^r}),$
and let
$\pi _r : \mathrm {SL}_2 ({\mathbb {Z}}_{p^n}) \to \mathrm {SL}_2 ({\mathbb {Z}}_{p^r}),$
and let
![]() $H_r = \mathrm {Ker\,} \pi _r$
. One can show that the set
$H_r = \mathrm {Ker\,} \pi _r$
. One can show that the set
![]() $\{ H_r \}_{r\leqslant n}$
gives all normal subgroups of
$\{ H_r \}_{r\leqslant n}$
gives all normal subgroups of
![]() $\mathrm {SL}_2 ({\mathbb {Z}}_{p^n}),$
and hence, any nonfaithful irreducible representation arises as a faithful irreducible representation of
$\mathrm {SL}_2 ({\mathbb {Z}}_{p^n}),$
and hence, any nonfaithful irreducible representation arises as a faithful irreducible representation of
![]() $\mathrm {SL}_2 ({\mathbb {Z}}_{p^r})$
for a certain
$\mathrm {SL}_2 ({\mathbb {Z}}_{p^r})$
for a certain
![]() $r<n$
. Anyway, we see that the multiplicity (dimension)
$r<n$
. Anyway, we see that the multiplicity (dimension)
![]() $d_\rho $
of any nontrivial irreducible representation
$d_\rho $
of any nontrivial irreducible representation
![]() $\rho $
of
$\rho $
of
![]() $\mathrm {SL}_2 ({\mathbb {Z}}_{p^n})$
is at least
$\mathrm {SL}_2 ({\mathbb {Z}}_{p^n})$
is at least
![]() $p/3 \geqslant M/3$
.
$p/3 \geqslant M/3$
.
Applying estimate (4.3), using the formula for
![]() $\mathsf {E}(G)$
via the representations and taking into account, the obtained lower bound for the multiplicities of the representations, we get
$\mathsf {E}(G)$
via the representations and taking into account, the obtained lower bound for the multiplicities of the representations, we get
 $$\begin{align*}\frac{M\| \widehat{G}\|^4_{op}}{3|\mathrm{SL}_2 ({\mathbb{Z}}_q)|} \leqslant \frac{1}{|\mathrm{SL}_2 ({\mathbb{Z}}_q)|} \sum_{\rho} d_\rho \| \widehat{G} (\rho) \widehat{G}^* (\rho) \|_{}^{2} = \mathsf{E}(G) \leqslant \tau(q) q |G|^2 , \end{align*}$$
$$\begin{align*}\frac{M\| \widehat{G}\|^4_{op}}{3|\mathrm{SL}_2 ({\mathbb{Z}}_q)|} \leqslant \frac{1}{|\mathrm{SL}_2 ({\mathbb{Z}}_q)|} \sum_{\rho} d_\rho \| \widehat{G} (\rho) \widehat{G}^* (\rho) \|_{}^{2} = \mathsf{E}(G) \leqslant \tau(q) q |G|^2 , \end{align*}$$
and hence
 $$ \begin{align} \| \widehat{G}\|_{op} \leqslant |G| \cdot \left( \frac{3\tau(q)}{M\delta^2} \right)^{1/4} := \frac{|G|}{K} , \end{align} $$
$$ \begin{align} \| \widehat{G}\|_{op} \leqslant |G| \cdot \left( \frac{3\tau(q)}{M\delta^2} \right)^{1/4} := \frac{|G|}{K} , \end{align} $$
where by
![]() $\| \widehat {G}\|_{op}$
, we have denoted the maximum of the operator norm of matrices
$\| \widehat {G}\|_{op}$
, we have denoted the maximum of the operator norm of matrices
![]() $\widehat {G} (\rho )$
for all nontrivial representations
$\widehat {G} (\rho )$
for all nontrivial representations
![]() $\rho $
and
$\rho $
and
![]() $\| \cdot \|$
is the usual Hilbert–Schmidt norm. Thanks to our choice of
$\| \cdot \|$
is the usual Hilbert–Schmidt norm. Thanks to our choice of
![]() $M,$
one can see that bound (4.4) is nontrivial, that is,
$M,$
one can see that bound (4.4) is nontrivial, that is,
![]() $K>1$
. Returning to (4.2) and using the standard scheme (see, e.g., [Reference Shkredov17, Lemma 13 and Sections 5 and 6]), we obtain
$K>1$
. Returning to (4.2) and using the standard scheme (see, e.g., [Reference Shkredov17, Lemma 13 and Sections 5 and 6]), we obtain
 $$\begin{align*}N_{\mathcal{A}, \mathcal{B}} (\lambda) - \frac{|A||B||G| q}{J_2(q)} \leqslant \sqrt{|A||B|} |G| q^{-1/k} , \end{align*}$$
$$\begin{align*}N_{\mathcal{A}, \mathcal{B}} (\lambda) - \frac{|A||B||G| q}{J_2(q)} \leqslant \sqrt{|A||B|} |G| q^{-1/k} , \end{align*}$$
where
![]() $k \sim \log q/\log K$
and
$k \sim \log q/\log K$
and
![]() $|\mathrm {SL}_2 ({\mathbb {Z}}_q)| = q J_2 (q) = q^3 \prod _{p|q} (1-p^{-2})$
. Hence
$|\mathrm {SL}_2 ({\mathbb {Z}}_q)| = q J_2 (q) = q^3 \prod _{p|q} (1-p^{-2})$
. Hence
![]() $N_{\mathcal {A}, \mathcal {B}} (\lambda )> 0$
, provided
$N_{\mathcal {A}, \mathcal {B}} (\lambda )> 0$
, provided
![]() $K \gg (\sqrt {\alpha \beta })^{-O(1)}$
. The last condition is equivalent to
$K \gg (\sqrt {\alpha \beta })^{-O(1)}$
. The last condition is equivalent to
![]() $M\gg \tau (q) \delta ^{-2} (\sqrt {\alpha \beta })^{-O(1)}$
. This completes the proof.
$M\gg \tau (q) \delta ^{-2} (\sqrt {\alpha \beta })^{-O(1)}$
. This completes the proof.
5 On the covering numbers of difference sets
Let us recall the definition of the covering number of a set (see, e.g., [Reference Bollobás, Janson and Riordan1] or [Reference Ruzsa11]).
Definition 5.1 Let
![]() ${\mathbf G}$
be a finite abelian group with the group operation
${\mathbf G}$
be a finite abelian group with the group operation
![]() $+$
, and let
$+$
, and let
![]() $A\subseteq {\mathbf G}$
be a set. We write
$A\subseteq {\mathbf G}$
be a set. We write
and the quantity
![]() $\mathrm {cov}^{+} (A)$
is called the (additive) covering number of A.
$\mathrm {cov}^{+} (A)$
is called the (additive) covering number of A.
Having a finite ring
![]() $\mathcal {R}$
with two operations
$\mathcal {R}$
with two operations
![]() $+, \times $
, we underline which covering number we use, writing
$+, \times $
, we underline which covering number we use, writing
![]() $\mathrm {cov}^{+}$
or
$\mathrm {cov}^{+}$
or
![]() $\mathrm {cov}^\times $
. It is known [Reference Bollobás, Janson and Riordan1, Corollary 3.2] that for any set
$\mathrm {cov}^\times $
. It is known [Reference Bollobás, Janson and Riordan1, Corollary 3.2] that for any set
![]() $A \subseteq {\mathbf G}$
, one has
$A \subseteq {\mathbf G}$
, one has
![]() $\mathrm {cov}^{+} (A) = O\left (\frac {|{\mathbf G}|}{|A|} \log |A| \right )$
and the last bound is tight. In this section, we study difference sets
$\mathrm {cov}^{+} (A) = O\left (\frac {|{\mathbf G}|}{|A|} \log |A| \right )$
and the last bound is tight. In this section, we study difference sets
![]() $A-A$
,
$A-A$
,
![]() $A \subseteq {\mathbb {Z}}_q$
and show that
$A \subseteq {\mathbb {Z}}_q$
and show that
![]() $\mathrm {cov}^{\times } (A-A)$
is always small. First of all, let us make a remark about a connection between
$\mathrm {cov}^{\times } (A-A)$
is always small. First of all, let us make a remark about a connection between
![]() $\mathrm {cov}^{+}$
and
$\mathrm {cov}^{+}$
and
![]() $\mathrm {cov}^{\times }$
in a ring
$\mathrm {cov}^{\times }$
in a ring
![]() $\mathcal {R}$
.
$\mathcal {R}$
.
Proposition 5.2 Let
![]() $\mathcal {R}$
be a finite ring, and let
$\mathcal {R}$
be a finite ring, and let
![]() $S \subseteq \mathcal {R}$
be a set. Then
$S \subseteq \mathcal {R}$
be a set. Then
provided all numbers
![]() $1,\dots , \mathrm {cov}^{+} (S)$
belong to
$1,\dots , \mathrm {cov}^{+} (S)$
belong to
![]() $\mathcal {R}^*$
.
$\mathcal {R}^*$
.
Proof Let
![]() $S+X = {\mathbb {Z}}_q$
and
$S+X = {\mathbb {Z}}_q$
and
![]() $|X| = \mathrm {cov}^{+} (S) := k$
. For any
$|X| = \mathrm {cov}^{+} (S) := k$
. For any
![]() $g\in {\mathbb {Z}}_q$
, consider
$g\in {\mathbb {Z}}_q$
, consider
![]() $jg$
, where
$jg$
, where
![]() $j=0,1,\dots , k$
. By the pigeonhole principle, there are different
$j=0,1,\dots , k$
. By the pigeonhole principle, there are different
![]() $j_1 \neq j_2$
such that
$j_1 \neq j_2$
such that
![]() ${j_1 g \in S+x}$
and
${j_1 g \in S+x}$
and
![]() $j_2 g \in S+x$
with the same
$j_2 g \in S+x$
with the same
![]() $x\in X$
. It implies that
$x\in X$
. It implies that
![]() $(j_1-j_2)g \in S-S,$
and hence
$(j_1-j_2)g \in S-S,$
and hence
![]() $g\in (j_1-j_2)^{-1} (S-S)$
, provided
$g\in (j_1-j_2)^{-1} (S-S)$
, provided
![]() $(j_1-j_2)^{-1} \in \mathcal {R}^*$
. It remains to notice that
$(j_1-j_2)^{-1} \in \mathcal {R}^*$
. It remains to notice that
![]() $[-k,k]^{-1} \cdot (S-S) = [k]^{-1} \cdot (S-S)$
. This completes the proof.
$[-k,k]^{-1} \cdot (S-S) = [k]^{-1} \cdot (S-S)$
. This completes the proof.
By the well-known consequence of the Ruzsa covering lemma [Reference Tao and Vu21, Section 2.4], we have for any finite group
![]() ${\mathbf G}$
and a set
${\mathbf G}$
and a set
![]() $A\subseteq {\mathbf G}$
that for a certain set
$A\subseteq {\mathbf G}$
that for a certain set
![]() $Z\subseteq {\mathbf G}$
, one has
$Z\subseteq {\mathbf G}$
, one has
In particular, it means that
![]() $\mathrm {cov}^{+} (A-A) \leqslant |{\mathbf G}|/|A|$
. Thus, Proposition 5.2 gives us the following result.
$\mathrm {cov}^{+} (A-A) \leqslant |{\mathbf G}|/|A|$
. Thus, Proposition 5.2 gives us the following result.
Corollary 5.3 Let
![]() $\mathcal {R}$
be a finite ring, and let
$\mathcal {R}$
be a finite ring, and let
![]() $A \subseteq \mathcal {R}$
be a set,
$A \subseteq \mathcal {R}$
be a set,
![]() $|A| = \alpha |\mathcal {R}|$
. Then
$|A| = \alpha |\mathcal {R}|$
. Then
provided all numbers
![]() $1,\dots , [\alpha ^{-1}]$
belong to
$1,\dots , [\alpha ^{-1}]$
belong to
![]() $\mathcal {R}^*$
.
$\mathcal {R}^*$
.
Using the same method, one can estimate the multiplicative covering number of a Bohr set in
![]() ${\mathbb {Z}}_p$
(p is a prime number):
${\mathbb {Z}}_p$
(p is a prime number):
namely, we have
It is interesting to decrease the number of summands in Corollary 5.3. To this end, let us obtain the main result of this section.
Theorem 5.4 Let q be a positive integer, let
![]() $A \subseteq {\mathbb {Z}}_q$
be a set,
$A \subseteq {\mathbb {Z}}_q$
be a set,
![]() $|A| = \alpha q$
. Suppose that the least prime factor of q greater than
$|A| = \alpha q$
. Suppose that the least prime factor of q greater than
![]() $2 \alpha ^{-1}+3$
. Then
$2 \alpha ^{-1}+3$
. Then
More concretely,
![]() $[k_*]^{-1} \cdot (A-A) = {\mathbb {Z}}_q$
for a certain
$[k_*]^{-1} \cdot (A-A) = {\mathbb {Z}}_q$
for a certain
![]() $k_* \leqslant \alpha ^{-1}+1$
.
$k_* \leqslant \alpha ^{-1}+1$
.
Proof Let
![]() $p_1$
be the least prime factor of q. By our assumption, we know that
$p_1$
be the least prime factor of q. By our assumption, we know that
![]() ${p_1 \geqslant 2 \alpha ^{-1}+3}$
. Write
${p_1 \geqslant 2 \alpha ^{-1}+3}$
. Write
![]() $p_1=2k+1$
and take
$p_1=2k+1$
and take
![]() $\Lambda = \{0,1,\dots , k_*\}$
, where
$\Lambda = \{0,1,\dots , k_*\}$
, where
![]() $\lceil \alpha ^{-1} - 1 \rceil + 1 = k_* \leqslant k$
. Then one has
$\lceil \alpha ^{-1} - 1 \rceil + 1 = k_* \leqslant k$
. Then one has
![]() $Y:= (\Lambda - \Lambda )\setminus \{0\} \subseteq {\mathbb {Z}}^*_q$
. First of all, consider
$Y:= (\Lambda - \Lambda )\setminus \{0\} \subseteq {\mathbb {Z}}^*_q$
. First of all, consider
![]() $n\in {\mathbb {Z}}_q^*$
and form the set
$n\in {\mathbb {Z}}_q^*$
and form the set
![]() $n\cdot \Lambda + A$
. Since
$n\cdot \Lambda + A$
. Since
![]() $|\Lambda ||A| = (k_* +1) \alpha q> q$
, it follows that there are different
$|\Lambda ||A| = (k_* +1) \alpha q> q$
, it follows that there are different
![]() $\lambda _1,\lambda _2 \in \Lambda $
such that
$\lambda _1,\lambda _2 \in \Lambda $
such that
where
![]() $a_1, a_2 \in A$
and
$a_1, a_2 \in A$
and
![]() $a_1 \neq a_2$
. Hence
$a_1 \neq a_2$
. Hence
![]() $n\in Y^{-1} (A-A)$
and thus
$n\in Y^{-1} (A-A)$
and thus
![]() ${\mathbb {Z}}_q^* \subseteq Y^{-1} (A-A)$
. Also, notice that as in Proposition 5.2, one has
${\mathbb {Z}}_q^* \subseteq Y^{-1} (A-A)$
. Also, notice that as in Proposition 5.2, one has
![]() $Y^{-1} (A-A) = [k_*]^{-1} \cdot (A-A)$
.
$Y^{-1} (A-A) = [k_*]^{-1} \cdot (A-A)$
.
Now, let
![]() $n=n' q_1$
, where
$n=n' q_1$
, where
![]() $q_1|q$
and
$q_1|q$
and
![]() $n'$
is coprime to q. By the pigeonhole principle, there is
$n'$
is coprime to q. By the pigeonhole principle, there is
![]() $B \subseteq {\mathbb {Z}}_{q/q_1}$
and
$B \subseteq {\mathbb {Z}}_{q/q_1}$
and
![]() $s\in {\mathbb {Z}}_q$
such that
$s\in {\mathbb {Z}}_q$
such that
![]() $q_1 B+s \subseteq A$
and the density of B in
$q_1 B+s \subseteq A$
and the density of B in
![]() ${\mathbb {Z}}_{q/q_1}$
is at least
${\mathbb {Z}}_{q/q_1}$
is at least
![]() $\alpha $
. In particular, we have
$\alpha $
. In particular, we have
![]() $q_1 (B-B) \subseteq A-A$
. By the same argument as above, one has
$q_1 (B-B) \subseteq A-A$
. By the same argument as above, one has
![]() $n' \equiv y^{-1} (b_1-b_2) \pmod {q/q_1}$
, where
$n' \equiv y^{-1} (b_1-b_2) \pmod {q/q_1}$
, where
![]() $y\in Y$
and
$y\in Y$
and
![]() $b_1,b_2 \in B$
. It follows that
$b_1,b_2 \in B$
. It follows that
![]() ${n \equiv y^{-1} (a_1-a_2) \pmod q}$
as required. Thus, we have proved that
${n \equiv y^{-1} (a_1-a_2) \pmod q}$
as required. Thus, we have proved that
![]() $[k_*]^{-1} (A-A) = {\mathbb {Z}}_q,$
and hence
$[k_*]^{-1} (A-A) = {\mathbb {Z}}_q,$
and hence
![]() $\mathrm {cov}^\times (A-A) \leqslant k_* \leqslant \alpha ^{-1}+1$
. This completes the proof.
$\mathrm {cov}^\times (A-A) \leqslant k_* \leqslant \alpha ^{-1}+1$
. This completes the proof.
Remark 5.5 After the paper was written, the author was informed by Fish that Theorem 5.4 holds in greater generality, namely, for any measure preserving system the same is true for the set of return times of a set of positive measure.
Theorem 5.4 implies a consequence about the multiplicative covering numbers of the intersections of difference sets in the spirit of paper [Reference Stewart and Tijdeman20] (see [Reference Stewart and Tijdeman20, Theorems 1 and 3]).
Corollary 5.6 Let q be a positive integer, and let
![]() $A_1,\dots , A_k \subseteq {\mathbb {Z}}_q$
be sets,
$A_1,\dots , A_k \subseteq {\mathbb {Z}}_q$
be sets,
![]() $|A_i| = \alpha _i q$
,
$|A_i| = \alpha _i q$
,
![]() $i\in [k]$
. Suppose that the least prime factor of q greater than
$i\in [k]$
. Suppose that the least prime factor of q greater than
![]() $2 (\alpha _1, \dots , \alpha _k)^{-1}+3$
. Then
$2 (\alpha _1, \dots , \alpha _k)^{-1}+3$
. Then
 $$ \begin{align} \mathrm{cov}^{\times} \left(\bigcap_{i=1}^k (A_i - A_i) \right) \leqslant \frac{1}{\alpha_1, \dots, \alpha_k} + 1 . \end{align} $$
$$ \begin{align} \mathrm{cov}^{\times} \left(\bigcap_{i=1}^k (A_i - A_i) \right) \leqslant \frac{1}{\alpha_1, \dots, \alpha_k} + 1 . \end{align} $$
Proof Put
![]() $A_{\vec {s}} = A_1 \cap (A_2-s_1) \cap \dots (A_k-s_{k-1})$
, where
$A_{\vec {s}} = A_1 \cap (A_2-s_1) \cap \dots (A_k-s_{k-1})$
, where
![]() $\vec {s} = (s_1,\dots , s_{k-1}) \in {\mathbb {Z}}^{k-1}_q$
. We have
$\vec {s} = (s_1,\dots , s_{k-1}) \in {\mathbb {Z}}^{k-1}_q$
. We have
![]() $\sum _{\vec {s}} |A_{\vec {s}}| = |A_1| \dots |A_k|$
, and hence, there is
$\sum _{\vec {s}} |A_{\vec {s}}| = |A_1| \dots |A_k|$
, and hence, there is
![]() $\vec {s}_*$
such that
$\vec {s}_*$
such that
![]() $|A_{\vec {s}_*}| \geqslant \alpha _1, \dots , \alpha _k q$
. Clearly, for any
$|A_{\vec {s}_*}| \geqslant \alpha _1, \dots , \alpha _k q$
. Clearly, for any
![]() $\vec {s}$
, one has
$\vec {s}$
, one has
 $$\begin{align*}A_{\vec{s}} - A_{\vec{s}} \subseteq \bigcap_{i=1}^k (A_i - A_i) . \end{align*}$$
$$\begin{align*}A_{\vec{s}} - A_{\vec{s}} \subseteq \bigcap_{i=1}^k (A_i - A_i) . \end{align*}$$
Applying Theorem 5.4 with
![]() $A = A_{\vec {s}_*}$
, we obtain bound (5.4). This completes the proof.
$A = A_{\vec {s}_*}$
, we obtain bound (5.4). This completes the proof.
As we have seen before, Corollary 5.3 and Theorem 5.4 give us some bounds for the multiplicative covering numbers of difference sets. On the other hand, one can see that Theorem 5.4 does not hold for, say, nonzero shifts of Bohr sets, for the sumsets
![]() $A+A$
, for the higher sumsets
$A+A$
, for the higher sumsets
![]() $nA$
,
$nA$
,
![]() $n>2$
and so on. Indeed, consider the following.
$n>2$
and so on. Indeed, consider the following.
Example 5.7 Let p be a prime number and
![]() $S = [p/3,2p/3)$
or
$S = [p/3,2p/3)$
or
![]() $S=\pm [p/6,p/3)$
to make S symmetric. Then the equation
$S=\pm [p/6,p/3)$
to make S symmetric. Then the equation
![]() $a+b \equiv c\quad \pmod p$
has no solutions in
$a+b \equiv c\quad \pmod p$
has no solutions in
![]() $a,b,c\in S$
. Further, we have
$a,b,c\in S$
. Further, we have
![]() $|S| \gg p$
but it is easy to see that
$|S| \gg p$
but it is easy to see that
![]() $\mathrm {cov}^\times (S)$
is unbounded. Indeed, if
$\mathrm {cov}^\times (S)$
is unbounded. Indeed, if
![]() $SX = {\mathbb {Z}}_p$
for a set X with
$SX = {\mathbb {Z}}_p$
for a set X with
![]() $|X| = O(1)$
, then we obtain a coloring of
$|X| = O(1)$
, then we obtain a coloring of
![]() ${\mathbb {Z}}_p$
with a finite number of colors and every color has no solutions to our equation
${\mathbb {Z}}_p$
with a finite number of colors and every color has no solutions to our equation
![]() $a+b \equiv c\quad \pmod p$
. It gives us a contradiction with the famous Schur theorem, (see [Reference Schur14]) (actually, it implies
$a+b \equiv c\quad \pmod p$
. It gives us a contradiction with the famous Schur theorem, (see [Reference Schur14]) (actually, it implies
![]() $\mathrm {cov}^\times (S) \gg \log p/\log \log p$
).
$\mathrm {cov}^\times (S) \gg \log p/\log \log p$
).
In particular, we see that
![]() $\mathrm {cov}^\times (X+s)$
can be much larger than
$\mathrm {cov}^\times (X+s)$
can be much larger than
![]() $\mathrm {cov}^\times (X)$
for a set X and a nonzero s.
$\mathrm {cov}^\times (X)$
for a set X and a nonzero s.
Proposition 5.2 implies that any syndetic set
![]() $S\subseteq \mathbb {F}_p$
,
$S\subseteq \mathbb {F}_p$
,
![]() $|S| \gg p$
has
$|S| \gg p$
has
![]() ${\mathrm {cov}^{\times } (S-S) = O(1)}$
. On the other hand, thanks to inclusion (5.2) any set of the form
${\mathrm {cov}^{\times } (S-S) = O(1)}$
. On the other hand, thanks to inclusion (5.2) any set of the form
![]() $A-A$
, where
$A-A$
, where
![]() $A\subseteq \mathbb {F}_p$
,
$A\subseteq \mathbb {F}_p$
,
![]() $|A| \gg p$
is syndetic (with the gap depending on A but not just on
$|A| \gg p$
is syndetic (with the gap depending on A but not just on
![]() $p/|A|$
, of course). Thus, it is natural to ask about a generalization of Theorem 5.4 to the family of syndetic sets. Nevertheless, taking
$p/|A|$
, of course). Thus, it is natural to ask about a generalization of Theorem 5.4 to the family of syndetic sets. Nevertheless, taking
![]() $S=\{ 1+ kM\}_{k\in [(p-1)/M]}$
,
$S=\{ 1+ kM\}_{k\in [(p-1)/M]}$
,
![]() $M\geqslant 5$
and
$M\geqslant 5$
and
![]() $p \equiv 2 \pmod M$
, say, we see that S is a syndetic set and S has no solutions to the equation
$p \equiv 2 \pmod M$
, say, we see that S is a syndetic set and S has no solutions to the equation
![]() $a+b \equiv c \pmod p$
. Thus, as in the example above, we see that
$a+b \equiv c \pmod p$
. Thus, as in the example above, we see that
![]() $\mathrm {cov}^\times (S)$
is unbounded.
$\mathrm {cov}^\times (S)$
is unbounded.
Remark 5.8 A dual form of Theorem 5.4 has no place, namely, there is a set
![]() ${A\subseteq {\mathbb {Z}}_p}$
,
${A\subseteq {\mathbb {Z}}_p}$
,
![]() $|A| \gg p$
such that
$|A| \gg p$
such that
![]() $\mathrm {cov}^{+} (A/A) \gg \log p$
. In other words,
$\mathrm {cov}^{+} (A/A) \gg \log p$
. In other words,
![]() $\mathrm {cov}^{+} (A/A)$
is close to the maximal possible value. To see this, just take A to be the set of all quadratic residues (see, e.g., [Reference Schoen and Shkredov13, Proposition 14]).
$\mathrm {cov}^{+} (A/A)$
is close to the maximal possible value. To see this, just take A to be the set of all quadratic residues (see, e.g., [Reference Schoen and Shkredov13, Proposition 14]).
Finally, let us give another proof of a variant of Theorem 1.1 via our covering Theorem 5.4. Notice that the number d below can be a non-divisor of q.
Theorem 5.9 Let q be a positive integer, let
![]() $A, B\subset {\mathbb {Z}}_q$
be sets,
$A, B\subset {\mathbb {Z}}_q$
be sets,
![]() $|A| = \alpha q$
,
$|A| = \alpha q$
,
![]() $|B|=\beta q$
, and let us assume that
$|B|=\beta q$
, and let us assume that
![]() $\alpha \geqslant \beta $
. Suppose that the least prime factor of q greater than
$\alpha \geqslant \beta $
. Suppose that the least prime factor of q greater than
![]() $2 \beta ^{-1}+3$
. Then, there is
$2 \beta ^{-1}+3$
. Then, there is
![]() $d\neq 0$
with
$d\neq 0$
with
and such that
Proof Applying Theorem 5.4 with
![]() $A=B$
, we find a set
$A=B$
, we find a set
![]() $X\subseteq {\mathbb {Z}}_q$
,
$X\subseteq {\mathbb {Z}}_q$
,
![]() $n:=|X| \leqslant \beta ^{-1} +1$
such that
$n:=|X| \leqslant \beta ^{-1} +1$
such that
![]() $X(B-B) = {\mathbb {Z}}_q$
. Let
$X(B-B) = {\mathbb {Z}}_q$
. Let
![]() $X = \{ x_1,\dots ,x_n\}$
and
$X = \{ x_1,\dots ,x_n\}$
and
![]() $\vec {x} = (x_1,\dots ,x_n) \in {\mathbb {Z}}^n_q$
. Considering the collection of the sets
$\vec {x} = (x_1,\dots ,x_n) \in {\mathbb {Z}}^n_q$
. Considering the collection of the sets
![]() $A^n + j \cdot \vec {x} \subseteq {\mathbb {Z}}^n_q$
,
$A^n + j \cdot \vec {x} \subseteq {\mathbb {Z}}^n_q$
,
![]() $j\geqslant 1$
, we see that there is
$j\geqslant 1$
, we see that there is
![]() $0<d \leqslant \alpha ^{-n}$
with
$0<d \leqslant \alpha ^{-n}$
with
![]() $d\cdot X \subseteq A-A$
. Hence
$d\cdot X \subseteq A-A$
. Hence
as required. It remains to notice that
This completes the proof.
6 Concluding remarks
Let us discuss other approaches to Theorem 1.1. First of all, recall the well-known Furstenberg’s result [Reference Furstenberg6].
Theorem 6.1 [Furstenberg]. Let n be a positive integer, let
![]() $\delta \in (0,1]$
be a real number, and let S be a set of size n. Then for all sufficiently large
$\delta \in (0,1]$
be a real number, and let S be a set of size n. Then for all sufficiently large
![]() $N \geqslant N(\delta ,n)$
an arbitrary set
$N \geqslant N(\delta ,n)$
an arbitrary set
![]() $A\subseteq [N]\times [N]$
,
$A\subseteq [N]\times [N]$
,
![]() $|A| \geqslant \delta N^2$
contains the set
$|A| \geqslant \delta N^2$
contains the set
![]() $\alpha +\beta \cdot S$
for some
$\alpha +\beta \cdot S$
for some
![]() $\alpha $
and
$\alpha $
and
![]() $\beta \neq 0$
.
$\beta \neq 0$
.
Quantitative bounds for
![]() $N(\delta ,n)$
from Theorem 6.1 can be found in [Reference Shelah15].
$N(\delta ,n)$
from Theorem 6.1 can be found in [Reference Shelah15].
Corollary 6.2 Let q be a prime number,
![]() $\mathcal {A} \subseteq \mathbb {F}^2_q$
,
$\mathcal {A} \subseteq \mathbb {F}^2_q$
,
![]() $|\mathcal {A}| = \delta q^2$
and
$|\mathcal {A}| = \delta q^2$
and
![]() $A,B \subseteq \mathbb {F}_q$
,
$A,B \subseteq \mathbb {F}_q$
,
![]() $|A| = \alpha _* q$
,
$|A| = \alpha _* q$
,
![]() $|B| = \beta _* q$
. Then, there is a decreasing positive function
$|B| = \beta _* q$
. Then, there is a decreasing positive function
![]() $\varphi $
such that if
$\varphi $
such that if
![]() $\min \{\alpha _*,\beta _*,\delta \} \geqslant \varphi (q)$
, then formula (3.1) takes place for
$\min \{\alpha _*,\beta _*,\delta \} \geqslant \varphi (q)$
, then formula (3.1) takes place for
![]() $\mathcal {B} = A\times B$
, any
$\mathcal {B} = A\times B$
, any
![]() $\lambda \in {\mathbb {Z}}^*_q$
and
$\lambda \in {\mathbb {Z}}^*_q$
and
![]() $d=1$
.
$d=1$
.
Proof Take
![]() $S=S_1=[k]\times [k]$
or
$S=S_1=[k]\times [k]$
or
![]() $S=S_2 = \{ (2j,2j) ~:~ j\in [k]\}$
for a certain positive integer k. Applying Theorem 6.1 with
$S=S_2 = \{ (2j,2j) ~:~ j\in [k]\}$
for a certain positive integer k. Applying Theorem 6.1 with
![]() $n=|S|$
and
$n=|S|$
and
![]() $A=\mathcal {A}$
, we see that for some
$A=\mathcal {A}$
, we see that for some
![]() $\alpha $
,
$\alpha $
,
![]() $\beta \neq 0$
the following holds
$\beta \neq 0$
the following holds
![]() $\alpha +\beta \cdot S \subseteq \mathcal {A}$
and hence to solve (3.1) with
$\alpha +\beta \cdot S \subseteq \mathcal {A}$
and hence to solve (3.1) with
![]() $d=1$
it is sufficiently to find for any
$d=1$
it is sufficiently to find for any
![]() $\lambda \in {\mathbb {Z}}^*_q$
some elements
$\lambda \in {\mathbb {Z}}^*_q$
some elements
![]() $a\in \beta ^{-1} (A-\alpha )$
,
$a\in \beta ^{-1} (A-\alpha )$
,
![]() $b\in \beta ^{-1} (B-\alpha )$
and
$b\in \beta ^{-1} (B-\alpha )$
and
![]() $(t_1,t_2) \in S$
such that
$(t_1,t_2) \in S$
such that
If for a certain absolute constant
![]() $C>0$
, one has
$C>0$
, one has
![]() $k \gg \min ^{-C} \{\alpha _* ,\beta _*,\delta \}$
, then for
$k \gg \min ^{-C} \{\alpha _* ,\beta _*,\delta \}$
, then for
![]() ${S=S_2}$
, the last equation has a solution thanks to the famous Bourgain–Gamburd machine [Reference Bourgain and Gamburd4] (see details in [Reference Shkredov18], say) and for
${S=S_2}$
, the last equation has a solution thanks to the famous Bourgain–Gamburd machine [Reference Bourgain and Gamburd4] (see details in [Reference Shkredov18], say) and for
![]() $S=S_1$
(actually, for any dense subset of
$S=S_1$
(actually, for any dense subset of
![]() $S_1$
), the latter fact was obtained in [Reference Shkredov18, Theorem 3]. This completes the proof.
$S_1$
), the latter fact was obtained in [Reference Shkredov18, Theorem 3]. This completes the proof.
The author does not know how to obtain Corollary 6.2 for composite q because there is no control over divisors of
![]() $\beta $
in Theorem 6.1. It would be interesting to say something about prime factors of the dilation
$\beta $
in Theorem 6.1. It would be interesting to say something about prime factors of the dilation
![]() $\beta $
.
$\beta $
.
We finish this section with a problem (it is interesting in its own right from a combinatorial point of view), which potentially gives another proof of Corollary 6.2 thanks to [Reference Shkredov18, Theorem 3].
Problem 6.3 Let n be a positive integer, and let
![]() $\delta ,\kappa \in (0,1]$
be real numbers. Then for all sufficiently large
$\delta ,\kappa \in (0,1]$
be real numbers. Then for all sufficiently large
![]() $N\geqslant N(\delta ,\kappa ,n)$
an arbitrary set
$N\geqslant N(\delta ,\kappa ,n)$
an arbitrary set
![]() $A\subseteq [N]\times [N]$
,
$A\subseteq [N]\times [N]$
,
![]() $|A| \geqslant \delta N^2$
contains the set
$|A| \geqslant \delta N^2$
contains the set
![]() $\alpha +\beta \cdot S$
for some
$\alpha +\beta \cdot S$
for some
![]() $\alpha $
and
$\alpha $
and
![]() $\beta $
, where
$\beta $
, where
![]() $S \subseteq [n]\times [n]$
is any set of size
$S \subseteq [n]\times [n]$
is any set of size
![]() $n^{1+\kappa }$
.
$n^{1+\kappa }$
.
Of course, some estimates on
![]() $N(\delta ,\kappa ,n)$
follow from Theorem 6.1 but maybe it is possible to obtain a better bound.
$N(\delta ,\kappa ,n)$
follow from Theorem 6.1 but maybe it is possible to obtain a better bound.
Acknowledgments
The author thanks Alexander Fish for very useful remarks (especially for Remark 5.5) and valuable comments. The author also expresses his deep gratitude to the referee for suggestions and comments.